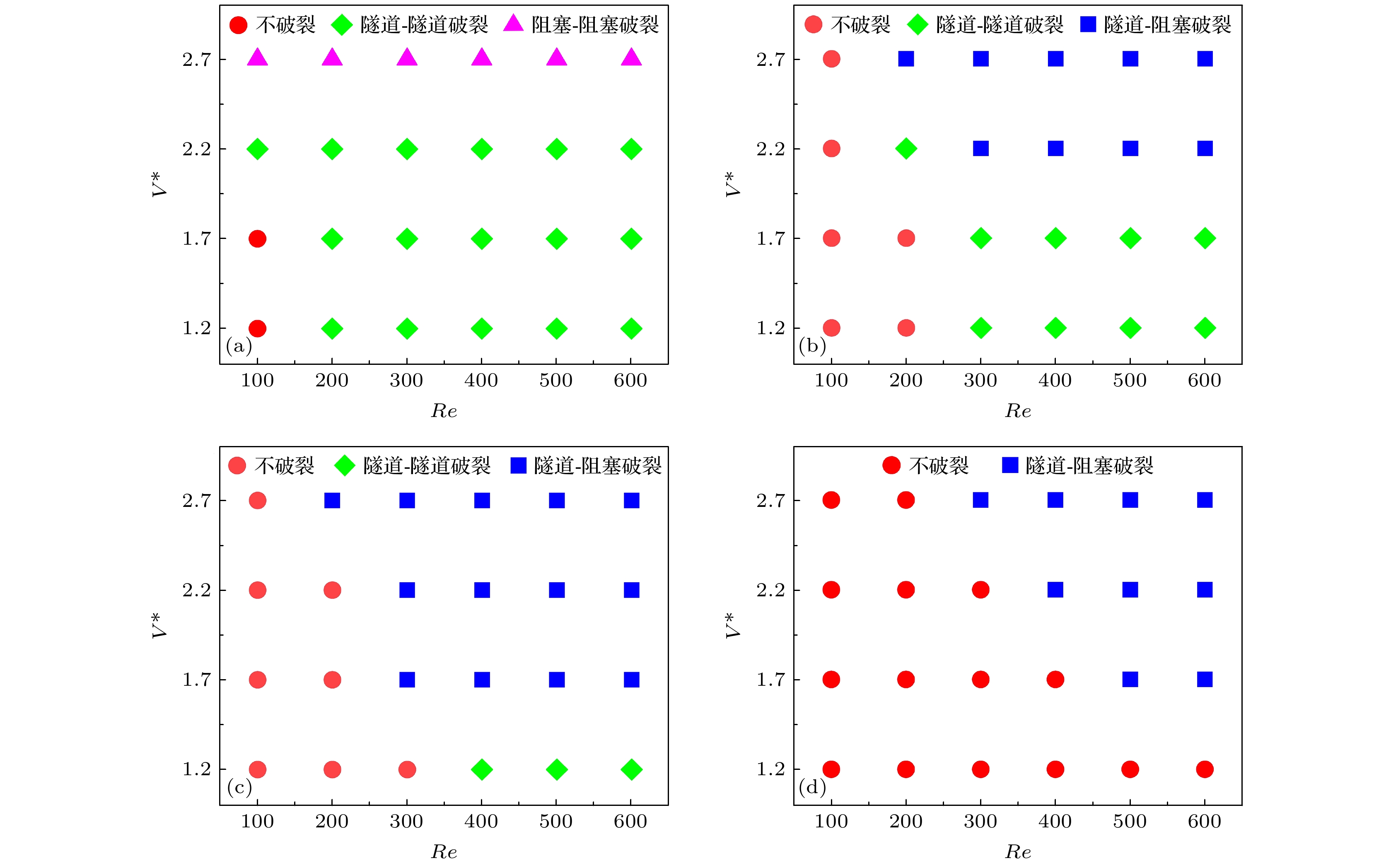
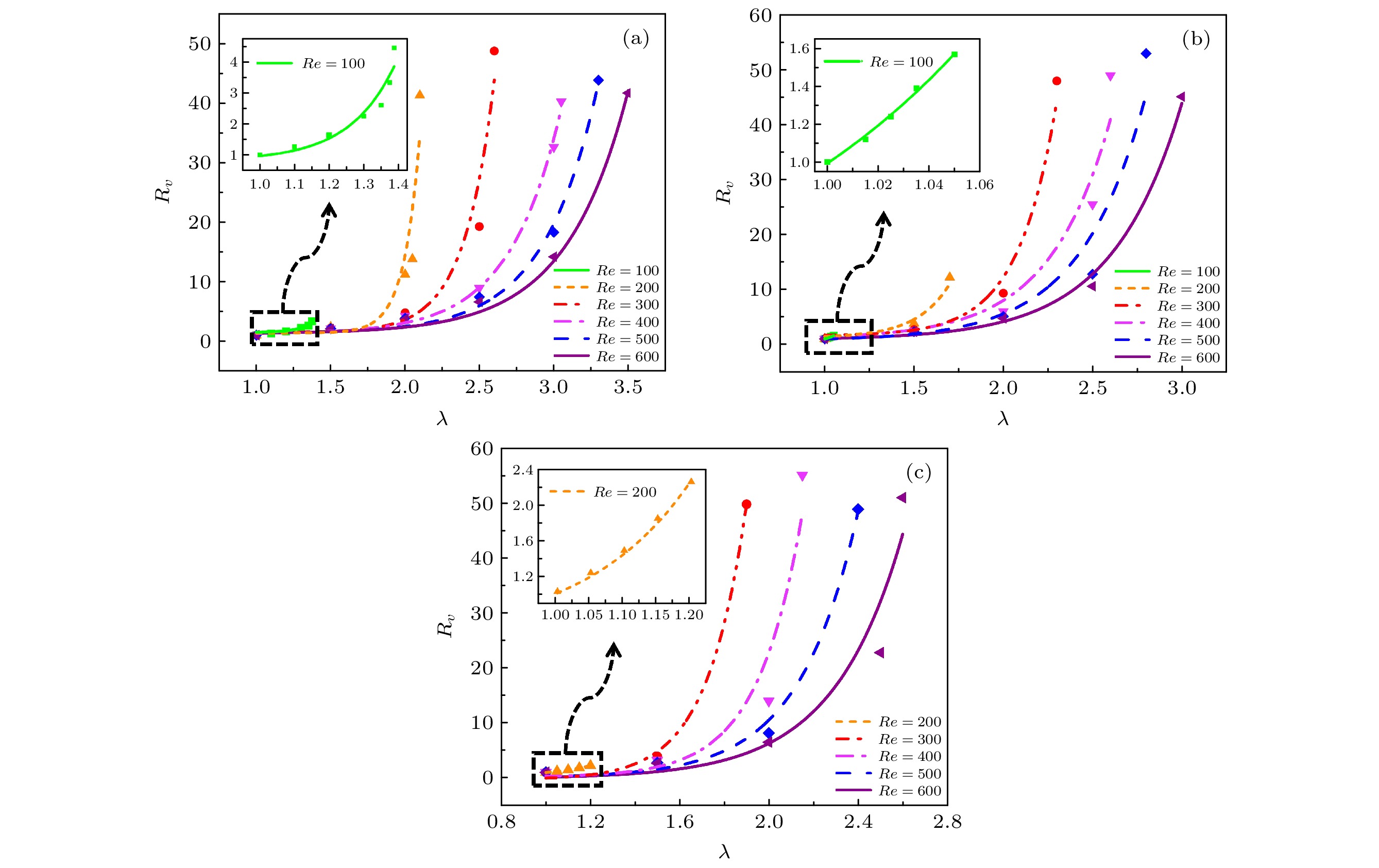
-
基于微通道两相流的微流控技术已得到广泛的应用, 精确控制通道中气泡或液滴的尺寸对相关微流控系统的设计起到至关重要的作用. 本文基于流体体积法重构Y型微通道内的气泡破裂行为, 系统研究了气泡无量纲尺寸(1.2—2.7)、出口流量比(1—4)以及主通道雷诺数(100—600)对气泡破裂行为的影响. 发现气泡非对称破裂过程分为3个阶段: 延伸阶段、挤压阶段和快速破裂阶段. 在气泡初始尺寸较小或出口流量较大的情况下, 气泡不破裂, 只经历延伸阶段和挤压阶段. 进一步针对不同尺寸和出口流量比揭示了气泡的4种破裂模式: 隧道-隧道破裂、阻塞-阻塞破裂、隧道-阻塞破裂和不破裂. 随着出口流量比的增大, 气泡的破裂过程逐渐变为非对称破裂, 其破裂模式沿隧道-隧道破裂/阻塞-阻塞破裂、逐渐向隧道-阻塞破裂和不破裂方向转变. 在此基础上获得了不同雷诺数和初始气泡尺寸下, 气泡破裂的临界流量比以及气泡破裂后子气泡体积比随出口流量比的变化规律并提炼了相应的准则关联式, 可为精确调控破裂后子气泡的尺寸提供理论指导.Microfluidic technology based on microchannel two-phase flow has been widely used. The precise control of the bubble or droplet size in the channel plays a crucial role in designing the microfluidic systems. In this work, the bubble breakup behavior in Y-shaped microchannel is reconstructed based on the volume of fluid method (VOF), and the effects of bubble dimensionless size (1.2–2.7), outlet flow ratio (1–4) and main channel Reynolds number (100–600) on the bubble breakup behavior are systematically investigated. The bubble asymmetric breakup process is found to be divided into three stages: extension stage, squeeze stage, and rapid pinch-off stage. In the case of small initial bubble size or relatively high outlet flow rate, the bubble does not break, but only experiences the extension stage and the squeezing stage. Four flow patterns of bubble breakup are further revealed for the bubbles with different sizes and outlet flow ratios: tunnel-tunnel breakup, obstruction-obstruction breakup, tunnel-obstruction breakup, and non-breakup. With the increase of outlet flow ratio, the breakup process of the bubble gradually becomes asymmetrical, and the flow pattern shifts along the tunnel-tunnel breakup and the obstruction-obstruction breakup, gradually turns toward the tunnel-obstruction breakup and non-breakup. On this basis, the critical flow ratio of bubble breakup and the variation of daughter bubble volume ratio with outlet flow ratio are obtained for different Reynolds numbers and initial bubble sizes, and the corresponding criterion correlation equation is refined, which can provide theoretical guidance for accurately regulating the daughter bubble size after breakup.
-
Keywords:
- outlet flow ratio /
- Y-shaped microchannel /
- bubble breakup /
- 3-dimensional numerical simulation
[1] 涂善东, 周帼彦, 于新海 2007 化工进展 26 2
Tu S T, Zhou G Y, Yu X H 2007 Chem. Ind. Eng. Prog. 26 2
[2] Teh S Y, Lin R, Hung L H, Lee A P 2008 Lab on Chip 8 198
Google Scholar
[3] 王琳琳, 李国君, 田辉, 叶阳辉 2011 西安交通大学学报 45 9
Wang L L, Li G J, Tian H, Ye Y H 2011 J. Xi'an Jiaotong. Univ. 45 9
[4] 王长亮, 靳遵龙, 王永庆, 王定标 2017 化工进展 36 S1
Wang C L, Jin Z L, Wang Y Q, Wang D B 2017 Chem. Ind. Eng. Prog 36 S1
[5] 乐军, 陈光文, 袁权, 罗灵爱, Hervé L G 2006 化工学报 57 6
Google Scholar
Le J, Chen G W, Yuan Q 2006 J. Chem. Ind. Eng. (China) 57 6
Google Scholar
[6] 梁宏, 柴振华, 施保昌 2016 65 204701
Google Scholar
Liang H, Cai Z H, Shi B C 2016 Acta Phys. Sin. 65 204701
Google Scholar
[7] Wang H 2019 Langmuir 35 32
[8] Zhang J Z, Li W 2016 Int. Commun. Heat Mass Transf. 74 1
Google Scholar
[9] Talimi V, Muzychka Y S, Kocabiyik S 2012 Int. J. Multiphase Flow 39 88104
[10] Seemann R, Brinkmann M, Pfohl T, Herminghaus S 2012 Rep. Prog. Phys. 75 016601
Google Scholar
[11] Squires T M, Quake S R 2005 Rev. Mod. Phys. 77 977
Google Scholar
[12] Stone H A, Kim S 2001 AIChE J. 47 1250
Google Scholar
[13] 侯璟鑫, 钱刚, 周兴贵 2013 化工学报 64 6
Hou J X, Qian G, Zhou X G 2013 J. Chem. Ind. Eng. (China) 64 6
[14] Tan J, Du L, Xu J H, Wang K, Luo G S 2011 AIChE J. 57 2647
Google Scholar
[15] 刘赵淼, 刘丽昆, 申峰 2014 机械工程学报 50 8
Liu Z M, Liu L K, Shen F 2014 Chin. J. Mech. Eng. 50 8
[16] 王维萌, 马一萍, 王澎, 陈斌 2015 工程热 36 2
Wang W M, Ma Y P, Wang P, Chen B 2015 J. Eng. Therm. 36 2
[17] Ma D F, Liang D, Zhu C Y, Fu T T, Ma Y G 2020 Chem. Eng. Sci. 10 1016
[18] Carlson A, Do-Quang M, Amberg G 2010 Int. J. Multiphase Flow 36 397
Google Scholar
[19] Samie M, Salari A, Shafii M B 2013 Phys. Rev. E 87 053003
Google Scholar
[20] Zheng M M, Ma Y L, Jin T M, Wang J T 2016 Microfluid Nanofluid 20 107
Google Scholar
[21] 温宇, 朱春英, 付涛涛, 马友光 2016 高校化学工程学报 30 19
Google Scholar
Wen Y, Zhu C Y, Fu T T, Ma Y G 2016 J. Chem. Eng. Chin. Univ. 30 19
Google Scholar
[22] Calderon A J, Heo Y S, Huh D, Futai N, Takayama S, Fowlkes J B 2006 Appl. Phys. Lett. 89 299
[23] 丛振霞, 朱春英, 付涛涛, 马友光 2014 化工学报 65 93
Google Scholar
Cong Z X, Zhu C Y, Fu T T, Ma Y G 2014 J. Chem. Ind. Eng. (China) 65 93
Google Scholar
[24] Menetrier-Deremble L, Tabeling P 2006 Phys Rev. E 74 035303
[25] Lou Q, Li T, Yang M 2019 J. Appl. Phys. 126 034301
Google Scholar
[26] Brackbill J U, Kothe D B, Zemach C 1992 J. Comput. Phys. 100 335
Google Scholar
[27] Fluent, ANSYS FLUENT 17.0: User's Guide, 2016, ANSYS-Fluent Inc., Canonsburg, PA.
[28] Xu Z Y, Fu T T, Zhu C Y, Jiang S K, Wang K 2019 Electrophoresis 40 376
Google Scholar
[29] Gupta R, Fletcher D F, Haynes B S 2009 Chem. Eng. Sci. 64 29
[30] Pan Z H, Weibel J A, Garimella S V 2015 Numerical Heat Transfer, Part A 67 1
[31] Wang X, Liu Z M, Pang Y 2019 Chem. Eng. Sci. 197 258
Google Scholar
[32] Wang X, Zhu C, Wu Y, Fu T, Ma Y 2015 Chem. Eng. Sci. 132 128
Google Scholar
[33] Jullien M C, Tsang Mui Ching M J, Cohen C, Menetrier L, Tabeling P 2009 Phys. Fluids 21 072001
Google Scholar
[34] Leshansky A M, Pismen L M 2009 Phys. Fluids 21 023303
Google Scholar
-
图 1 (a) 孤立气泡通过Y型分支微通道的示意图; (b) Y型结区域的网格模型
Fig. 1. (a) Schematic illustration of an isolated bubble traveling through a Y-shaped branching microchannel; (b) mesh generation in Y-junction region.
图 3 网格无关性检验:
$t^* $ = 27.8时的气泡轮廓Fig. 3. Mesh independence study: Bubble profile at the dimensionless time instant of 27.8.
图 4 Y型分支微通道中气泡轮廓的演化 (a) Re = 100, λ = 1; (b) Re = 300, λ = 1; (c) Re = 300, λ = 2; (d) Re = 300, λ = 4
Fig. 4. Evolution of bubble profile in Y-junction region: (a) Re = 100, λ = 1; (b) Re = 300, λ = 1; (c) Re = 300, λ = 2; (d) Re = 300, λ = 4.
图 5 气泡的4种破裂模式 (a) 隧道-隧道破裂; (b) 阻塞-阻塞破裂; (c) 隧道-阻塞破裂; (d) 不破裂
Fig. 5. Different flow patterns of bubble breakup: (a) Tunnel-tunnel breakup; (b) obstruction-obstruction breakup; (c) tunnel-obstruction breakup; (d) non-breakup.
图 6 液相的挤压力和剪切力分布 (a) 隧道-隧道破裂; (b) 阻塞-阻塞破裂; (c) 隧道-阻塞破裂
Fig. 6. Liquid-phase squeezing pressure and shear force distribution during bubble motion: (a) Tunnel-tunnel breakup; (b) obstruction-obstruction breakup; (c) tunnel-obstruction breakup.
图 7 不同流量比下气泡的破裂模式相图 (a) λ = 1.0; (b) λ = 1.5; (c) λ = 2.0; (d) λ = 2.5
Fig. 7. Bubble breakup modes at different flow ratios: (a) λ = 1.0; (b) λ = 1.5; (c) λ = 2.0; (d) λ = 2.5.
图 8 不同流量比(λ)下分支通道中子气泡的体积比(Rv) (a)
$V^* $ = 2.7; (b)$V^* $ = 2.2; (c)$V^* $ = 1.7Fig. 8. Volume ratio (Rv) of daughter bubbles in branching channel with different outlet flow ratio (λ): (a)
$V^* $ = 2.7; (b)$V^* $ = 2.2; (c)$V^* $ = 1.7.
图 9 不同气泡尺寸下的临界破裂流量比(λc)
Fig. 9. Critical fracture flow ratio (λc) at different bubble volume.
表 1 水和空气的物理性质(20 ℃)
Table 1. Physical parameters of water and air (20 ℃)
流体类型 密度/(kg·m–3) 黏性系
数/(Pa·s)表面张力
系数/(N·m–1)空气 1.225 0.000018 水 998.2 0.001005 0.07275 
下载: 导出CSV
表 2 网格无关性验证: 气泡长度对比
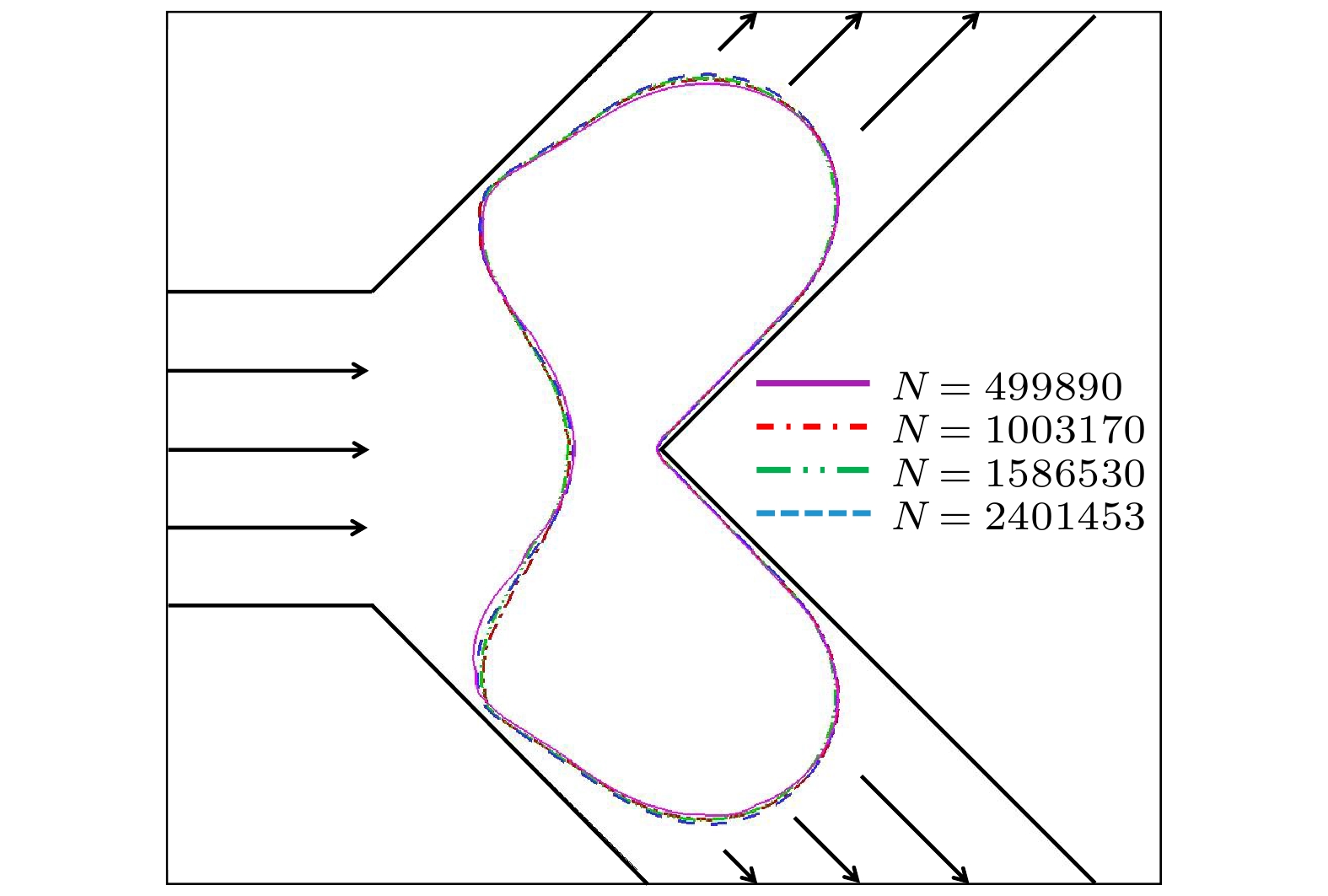
Table 2. Mesh-independent study: Comparison of bubble length.
算例 网格 1 网格 2 网格 3 网格 4 网格数 499890 1003170 1586530 2401453 雷诺数 100 600 100 600 100 600 100 600 气泡长度$l^* $ 2.122 2.563 2.196 2.595 2.187 2.589 2.190 2.592 |误差($l^* $)|/% 3.11 1.12 0.27 0.12 0.14 0.12 — —
下载: 导出CSV
Re 100 200 300 400 500 600 a1 1.47 1.45 1.35 1.30 1.25 1.22 b1 5.0 ×
10–71.1 ×
10–71.0 ×
10–45.8 ×
10–34.6 ×
10–32.2 ×
10–4c1 0.42 0.57 1.15 2.08 2.25 2.65 a2 0.001 0.001 0.001 0.02 0.02 0.02 b2 9.9 ×
10–51.20 1.58 1.07 0.66 0.81 c2 0.50 0.98 1.23 2.05 2.24 2.50 a3 — 0.001 0.001 0.001 0.005 0.010 b3 — 0.47 –0.36 0.15 0.03 –0.13 c3 — 0.85 1.00 1.20 1.63 1.98
下载: 导出CSV
i j $V^* $= 1.7 –0.79 0.025 $V^* $= 2.2 –0.44 0.034 $V^* $= 2.7 –1.54 0.054
下载: 导出CSV
-
[1] 涂善东, 周帼彦, 于新海 2007 化工进展 26 2
Tu S T, Zhou G Y, Yu X H 2007 Chem. Ind. Eng. Prog. 26 2
[2] Teh S Y, Lin R, Hung L H, Lee A P 2008 Lab on Chip 8 198
Google Scholar
[3] 王琳琳, 李国君, 田辉, 叶阳辉 2011 西安交通大学学报 45 9
Wang L L, Li G J, Tian H, Ye Y H 2011 J. Xi'an Jiaotong. Univ. 45 9
[4] 王长亮, 靳遵龙, 王永庆, 王定标 2017 化工进展 36 S1
Wang C L, Jin Z L, Wang Y Q, Wang D B 2017 Chem. Ind. Eng. Prog 36 S1
[5] 乐军, 陈光文, 袁权, 罗灵爱, Hervé L G 2006 化工学报 57 6
Google Scholar
Le J, Chen G W, Yuan Q 2006 J. Chem. Ind. Eng. (China) 57 6
Google Scholar
[6] 梁宏, 柴振华, 施保昌 2016 65 204701
Google Scholar
Liang H, Cai Z H, Shi B C 2016 Acta Phys. Sin. 65 204701
Google Scholar
[7] Wang H 2019 Langmuir 35 32
[8] Zhang J Z, Li W 2016 Int. Commun. Heat Mass Transf. 74 1
Google Scholar
[9] Talimi V, Muzychka Y S, Kocabiyik S 2012 Int. J. Multiphase Flow 39 88104
[10] Seemann R, Brinkmann M, Pfohl T, Herminghaus S 2012 Rep. Prog. Phys. 75 016601
Google Scholar
[11] Squires T M, Quake S R 2005 Rev. Mod. Phys. 77 977
Google Scholar
[12] Stone H A, Kim S 2001 AIChE J. 47 1250
Google Scholar
[13] 侯璟鑫, 钱刚, 周兴贵 2013 化工学报 64 6
Hou J X, Qian G, Zhou X G 2013 J. Chem. Ind. Eng. (China) 64 6
[14] Tan J, Du L, Xu J H, Wang K, Luo G S 2011 AIChE J. 57 2647
Google Scholar
[15] 刘赵淼, 刘丽昆, 申峰 2014 机械工程学报 50 8
Liu Z M, Liu L K, Shen F 2014 Chin. J. Mech. Eng. 50 8
[16] 王维萌, 马一萍, 王澎, 陈斌 2015 工程热 36 2
Wang W M, Ma Y P, Wang P, Chen B 2015 J. Eng. Therm. 36 2
[17] Ma D F, Liang D, Zhu C Y, Fu T T, Ma Y G 2020 Chem. Eng. Sci. 10 1016
[18] Carlson A, Do-Quang M, Amberg G 2010 Int. J. Multiphase Flow 36 397
Google Scholar
[19] Samie M, Salari A, Shafii M B 2013 Phys. Rev. E 87 053003
Google Scholar
[20] Zheng M M, Ma Y L, Jin T M, Wang J T 2016 Microfluid Nanofluid 20 107
Google Scholar
[21] 温宇, 朱春英, 付涛涛, 马友光 2016 高校化学工程学报 30 19
Google Scholar
Wen Y, Zhu C Y, Fu T T, Ma Y G 2016 J. Chem. Eng. Chin. Univ. 30 19
Google Scholar
[22] Calderon A J, Heo Y S, Huh D, Futai N, Takayama S, Fowlkes J B 2006 Appl. Phys. Lett. 89 299
[23] 丛振霞, 朱春英, 付涛涛, 马友光 2014 化工学报 65 93
Google Scholar
Cong Z X, Zhu C Y, Fu T T, Ma Y G 2014 J. Chem. Ind. Eng. (China) 65 93
Google Scholar
[24] Menetrier-Deremble L, Tabeling P 2006 Phys Rev. E 74 035303
[25] Lou Q, Li T, Yang M 2019 J. Appl. Phys. 126 034301
Google Scholar
[26] Brackbill J U, Kothe D B, Zemach C 1992 J. Comput. Phys. 100 335
Google Scholar
[27] Fluent, ANSYS FLUENT 17.0: User's Guide, 2016, ANSYS-Fluent Inc., Canonsburg, PA.
[28] Xu Z Y, Fu T T, Zhu C Y, Jiang S K, Wang K 2019 Electrophoresis 40 376
Google Scholar
[29] Gupta R, Fletcher D F, Haynes B S 2009 Chem. Eng. Sci. 64 29
[30] Pan Z H, Weibel J A, Garimella S V 2015 Numerical Heat Transfer, Part A 67 1
[31] Wang X, Liu Z M, Pang Y 2019 Chem. Eng. Sci. 197 258
Google Scholar
[32] Wang X, Zhu C, Wu Y, Fu T, Ma Y 2015 Chem. Eng. Sci. 132 128
Google Scholar
[33] Jullien M C, Tsang Mui Ching M J, Cohen C, Menetrier L, Tabeling P 2009 Phys. Fluids 21 072001
Google Scholar
[34] Leshansky A M, Pismen L M 2009 Phys. Fluids 21 023303
Google Scholar
下载:
计量
- 文章访问数: 4801
- PDF下载量: 79
- 被引次数: 0
