-
利用高精度微压计数据、卫星、地面观测数据和GDAS数据, 对2020年7月29日—30日东北冷涡暴雨过程重力波特征进行分析, 结果表明: 重力波激发了此次暴雨, 在暴雨发生前2—4 h, 出现了周期约128 min的重力波先兆活动; 在暴雨期间, 重力波周期集中在120—180 min; 在强对流发展期间出现了周期为128—256 min的重力波和8—64 min周期更短的重力波. 强对流与重力波相互影响、相互作用. 强对流发展时, 地面出现雷暴高压、冷池、出流边界, 在冷池前方形成气流辐合区; 气流辐合辐散区向前移动, 形成重力波传播, 最终激发暴雨. 重力波先兆活动这一特征对东北冷涡暴雨有一定的指示预警意义.Taking advantage of high-precision microbarograph data, satellite, ground measured data and GDAS data, the characteristics of gravity waves during the cold vortex heavy rainfall in Northeast China on 29−30 July 2020 are analyzed. The results show that the gravity waves initiate this heavy rainfall. In 2−4 h before the heavy rainfall, there appear the precursor activities of gravity waves with a period of about 128 min. During the heavy rainfall, the periods of gravity waves concentrate in a range of 120−180 min. During the development of convective storms, there occur gravity waves with periods in a range of 128−256 min. And severe storms spark the gravity waves with shorter periods (8−64 min). The relationship between rainstorm and gravity wave is interactive. When severe storms develop, there occur thunderstorm high pressure, cold pool and out-flow boundary. In the front of cold pool, there is formed a flow convergence. The convergence and divergence of air flow propagate forward, forming gravity waves and finally triggering the rainstorm. The characteristics of precursor activities of gravity waves may play a positive role in warning heavy rainfall during the cold vortex in Northeast China.
-
Keywords:
- gravity waves /
- microbarograph /
- cold vortex in Northeast China /
- heavy rainfall
[1] 张云, 雷恒池, 钱贞成 2008 大气科学 32 481
 Google Scholar
Google Scholar
Zhang Y, Lei H C, Qian Z C 2008 Chin. J. Atmos. Sci. 32 481
 Google Scholar
Google Scholar
[2] 钟水新, 王东海, 张人禾, 刘英 2011 高原气象 30 951
Zhong S X, Wang D H, Zhang R H, Liu Y 2011 Plateau Meteorol. 30 951
[3] Koch S E, Dorian P B 1988 Mon. Weather Rev. 116 2570
 Google Scholar
Google Scholar
[4] Schumacher R S, Johnson R H 2008 Mon. Weather Rev. 136 3964
 Google Scholar
Google Scholar
[5] Plougonven R, Zhang F Q 2014 Rev. Geophys. 52 33
 Google Scholar
Google Scholar
[6] Trexler C M, Koch S E 2000 Mon. Weather Rev. 128 2423
 Google Scholar
Google Scholar
[7] Sato K 1993 J. Atmos. Sci. 50 518
 Google Scholar
Google Scholar
[8] Balachandran N K 1980 Mon. Weather Rev. 108 804
 Google Scholar
Google Scholar
[9] 李麦村 1978 大气科学 2 201
 Google Scholar
Google Scholar
Li M C 1978 Chin. J. Atmos. Sci. 2 201
 Google Scholar
Google Scholar
[10] Uccellini L W 1975 Mon. Weather Rev. 103 497
 Google Scholar
Google Scholar
[11] Fovell R, Durran D, Holton J R 1992 J. Atmos. Sci. 49 1427
 Google Scholar
Google Scholar
[12] Piani C, Durran D, Alexander M J, Holton J R 2000 J. Atmos. Sci. 57 3689
 Google Scholar
Google Scholar
[13] Ruppert J H, Bosart L F 2014 Mon. Weather Rev. 142 1403
 Google Scholar
Google Scholar
[14] Liu L, Ran L K, Gao S T 2018 Adv. Atmos. Sci. 35 604
 Google Scholar
Google Scholar
[15] Huang X, Zhou Y S, Liu L 2020 Atmosphere. 11 752
 Google Scholar
Google Scholar
[16] Herron T J, Tolstoy I 1969 J. Atmos. Sci. 26 266
 Google Scholar
Google Scholar
[17] Stobie J G, Einaudi F, Uccellini L W 1983 J. Atmos. Sci. 40 2804
 Google Scholar
Google Scholar
[18] Rees J M, Denholm-Price J C W, King J C, Anderson P S 2000 J. Atmos. Sci. 57 511
 Google Scholar
Google Scholar
[19] Hauf T, Finke U, Neisser J, Bull G, Stangenberg J G 1996 J. Atmos. Oceanic Technol. 13 1001
 Google Scholar
Google Scholar
[20] Curry M J, Murty R C 1974 J. Atmos. Sci. 31 1402
 Google Scholar
Google Scholar
[21] Grachev A I, Kulichkov S N, Otezov A I 1997 Izv. Atmos. Oceanic Phys. 33 583
[22] Kulichkov S N, Chunchuzov I P, Popov O E, Perepelkin V G, Gorchakov G I 2019 Izv. Atmos. Oceanic Phys. 55 167
 Google Scholar
Google Scholar
[23] Blanc E, Farges T, Pichon A Le, Heinrich P 2014 J. Geophys. Res. Atmos. 119 6409
 Google Scholar
Google Scholar
[24] 李启泰, 谢金来, 杨训仁 1993 气象学报 51 361
Li Q T, Xie J L, Yang X R 1993 Acta Meteorol. Sin. 51 361
[25] Wu L J, Wen Z P, He H Y 2015 Atmos. Oceanic Sci. Lett. 8 78
 Google Scholar
Google Scholar
[26] Yang R, Liu Y, Ran L K, Zhang Y L 2018 Chin. Phys. B 27 059201
 Google Scholar
Google Scholar
[27] Torrence C G, Compo G P 1998 Bull. Am. Meteorol. Soc. 79 61
 Google Scholar
Google Scholar
[28] Wang X J, Lei H C, Feng L, Zhu J S, Li Z M, Jiang Z B 2020 Atmos. Oceanic Sci. Lett. 13 163
 Google Scholar
Google Scholar
[29] Rotunno R, Klemp J B, Weisman M L 1988 J. Atmos. Sci. 45 463
 Google Scholar
Google Scholar
[30] Weisman M L, Rotunno R 2004 J. Atmos. Sci. 61 361
 Google Scholar
Google Scholar
-
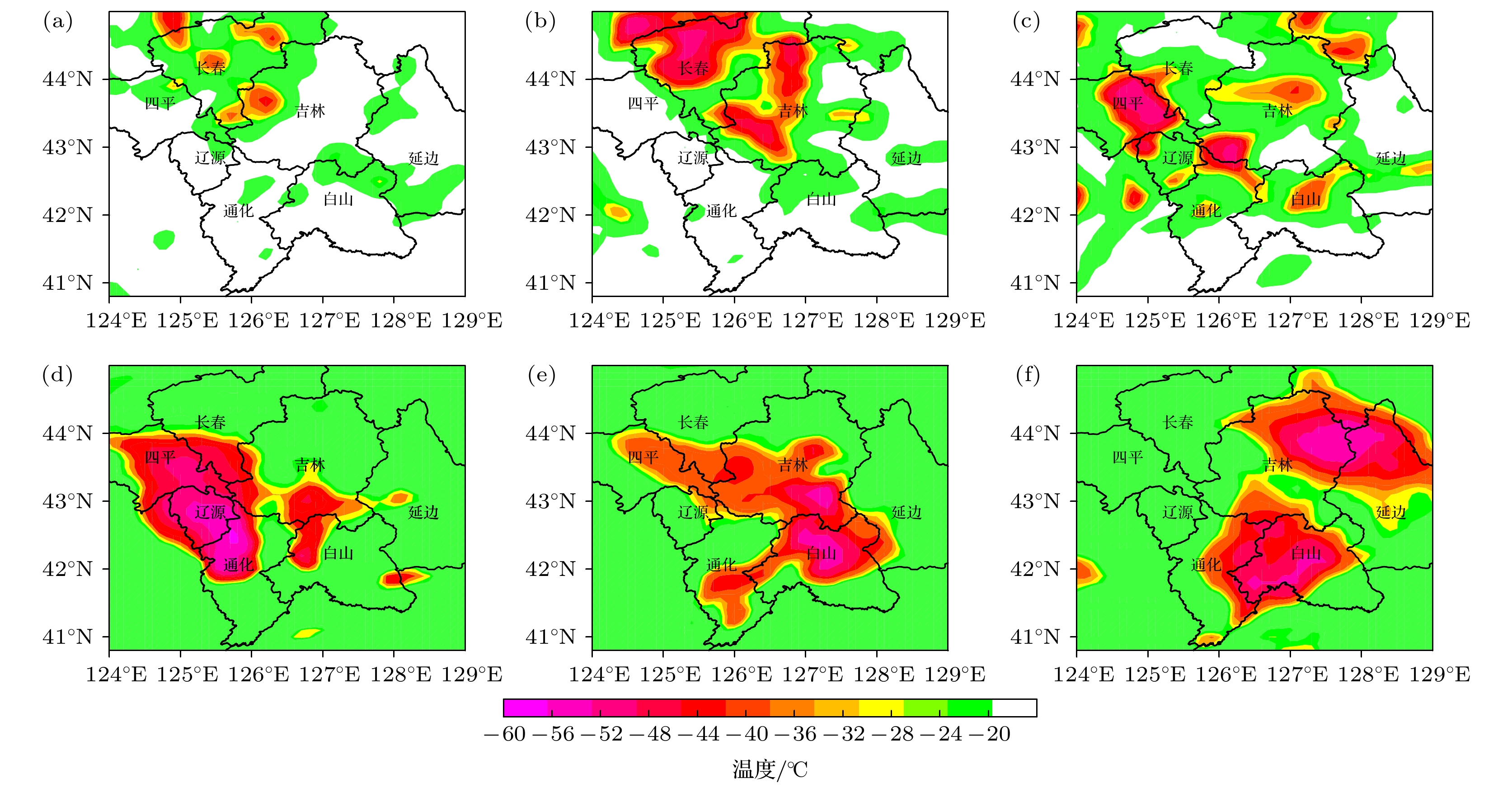
图 2 2020年7月29日7:00−30日5:00吉林省累计降雨量 (填色图, mm)、加密站E7215(黑色加号)、E9039(黑色圆点)、微压计1, 2, 3号站(黑色三角形)
Fig. 2. Accumulated heavy rainfall (shaded, mm) on 7 BJT 29−5 BJT 30 July 2020, the locations of surface automated mesonet station E7215 (black plus), E9039 (black dots) and microbarograph 1, 2, 3 (black triangle).
图 3 2020年7月29日20:00 (a) 200 hPa风场(风向杆, m/s); (b) 500 hPa位势高度场(黑色等值线, gpm)、温度场(红色等值线, ℃); (c) 850 hPa位势高度场(黑色等值线, gpm)、风矢; (d) 地面比湿(填色图, g/kg)、风矢
Fig. 3. (a) 200 hPa wind field (wind stick, m/s), (b) 500 hPa geopotential height field (black lines, gpm), temperature field (red lines, ℃), (c) 850 hPa geopotential height field (black lines, gpm), wind vector, (d) surface specific humidity (contour lines, g/kg), wind vector on 20 BJT 29.
图 5 2020年7月29日−30日 (a) E7215站(125.31°E, 43.04°N), (b) E9039 站(126.27°E, 41.95°N)1 h累积降雨量(黑色实线, mm)、气压(绿色实线, hPa)、温度(蓝色实线, ℃)
Fig. 5. The 1 h accumulated rainfall (black solid line, mm), pressure (green solid line, hPa), temperature (blue solid line, ℃) in (a) E7215 (125.31°E, 43.04°N), (b) E9039 (126.27°E, 41.95°N) on 29−30 July 2020.
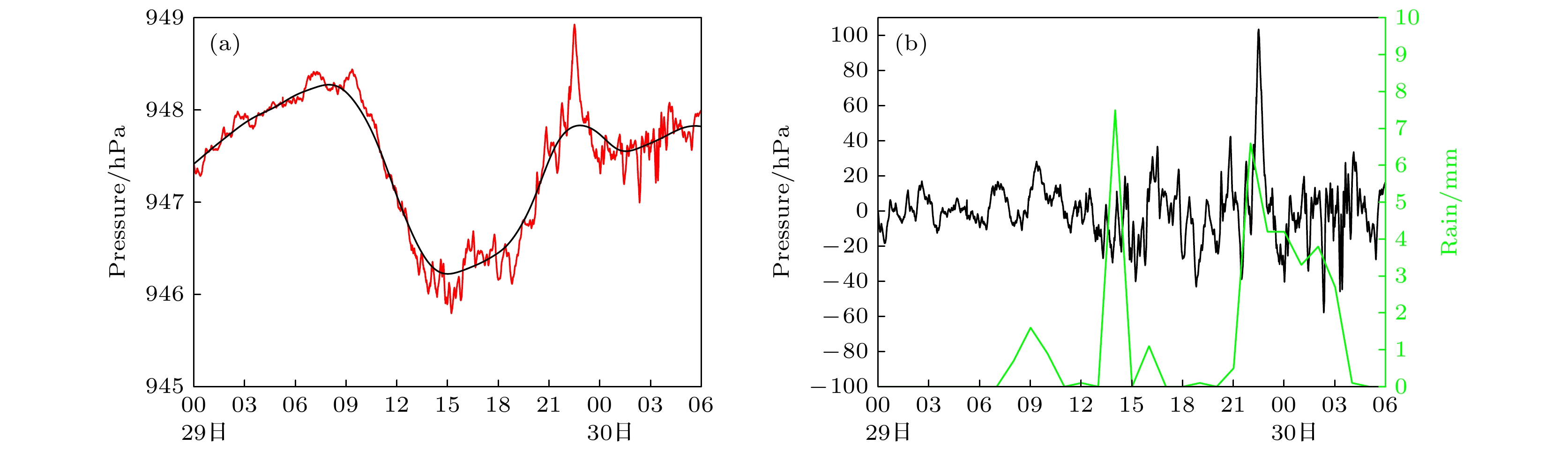
图 6 微压计3号站(126.61°E, 42.43°N) (a) 原始气压(红色实线, hPa)、气压日变化(黑色实线, hPa); (b) 扰动气压(黑色实线, Pa)、小时雨量(绿色实线, mm)
Fig. 6. Microbarograph 3 (126.61°E, 42.43°N): (a) Original pressure (red line, hPa), pressure daily variation (black line, hPa); (b) pressure disturbance (black line, Pa), hourly rain (green line, mm).
图 8 微压计2号站(126.8°E, 42.4°N) (a) 原始气压(红色实线, hPa)、气压日变化(黑色实线, hPa); (b) 扰动气压(黑色实线, Pa)、小时雨量(绿色实线, ℃)
Fig. 8. Microbarograph 2 (126.8°E, 42.4°N): (a) Original pressure data (red line, hPa), pressure daily variation (black line, hPa); (b) pressure disturbance (black line, Pa), hourly rain (green line, ℃).
图 7 微压计1号站(126.70°E, 42.41°N) (a) 原始气压(红色实线, hPa)、气压日变化(黑色实线, hPa); (b) 扰动气压(黑色实线, Pa)、小时雨量(绿色实线, ℃)
Fig. 7. Microbarograph 1 (126.70°E, 42.41°N): (a) Original pressure (red line, hPa), pressure daily variation (black line, hPa); (b) pressure disturbance (black line, Pa), hourly rain (green line, ℃).
图 9 2020年7月28日20:00−30日7:00重力波动态谱 ((a), (c), (e)) (填色图: 功率谱; 纵坐标: 周期; 横坐标: 时间; 黑色包络线显示了小波影响锥, 其外侧为边缘效应较大的区域, 绿色实线包围的是置信度在95%以上的区域; ▲表示降雨开始时间)与降雨量图 ((b), (d), (f)) (a), (b) 微压计3号站; (c), (d) 微压计1号站; (e), (f) 微压计2号站
Fig. 9. Gravity waves dynamic frequency spectra ((a), (c), (e)) (shaded: amplitudes; Y-axis: periods; X-axis: time; The black envelope line shows the cone of influence, outside of which edge effects might be large, and green solid lines designates the 95% confidence level, ▲ denotes the start time of rainfall) and precipitation figure ((b), (d), (f)): (a), (b) Microbarograph 3; (c), (d) microbarograph 1; (e), (f) microbarograph 2 on 20 BJT 28−07 BJT 30 July 2020.
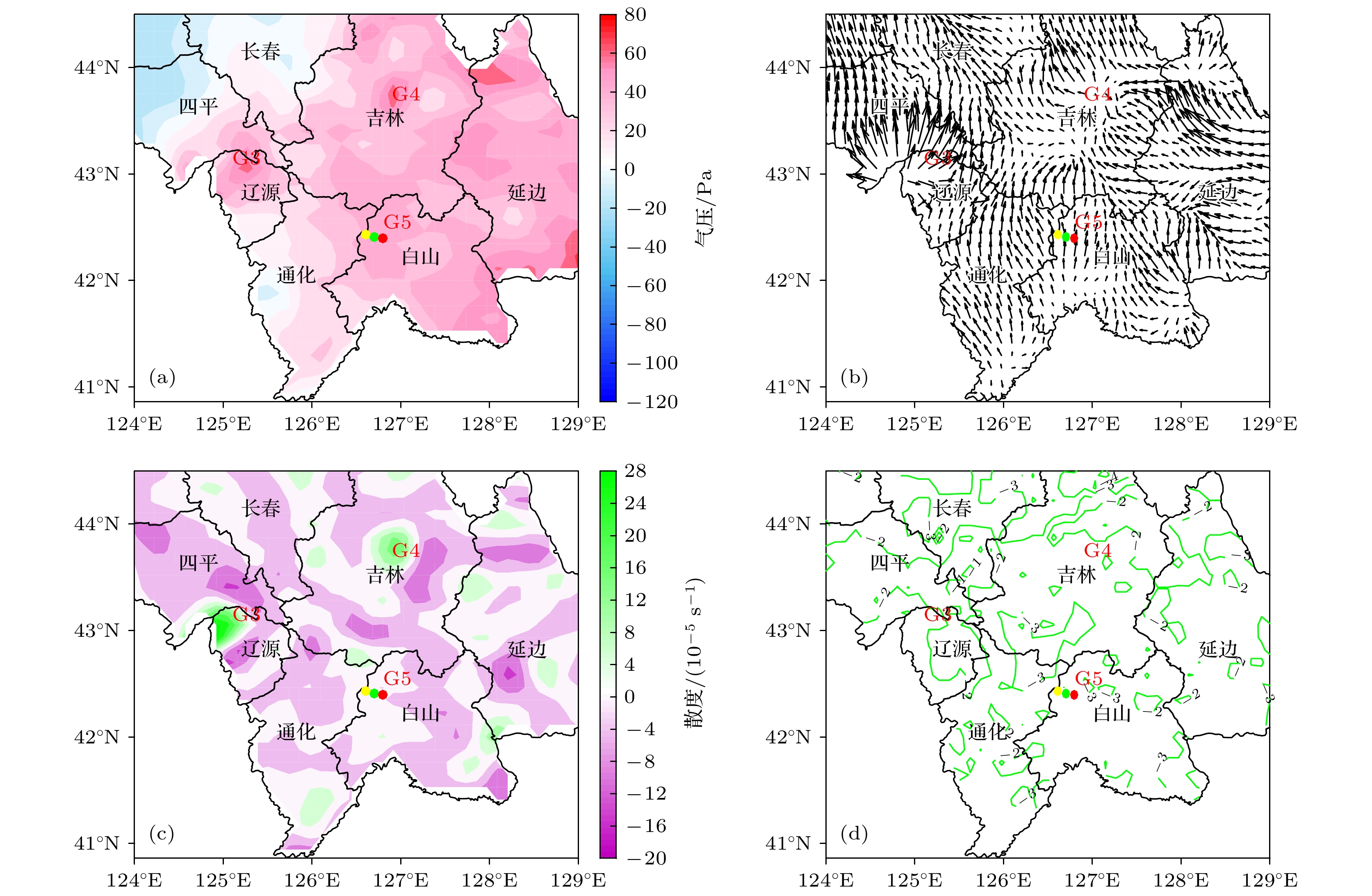
图 11 2020年7月29日14:00 (a) 地面气压扰动, (b) 风场, (c) 散度扰动, (d) 温度扰动(绿色等值线为正值, 红色虚线为负值, ℃). 绿色、红色、黄色圆点分别代表微压计1, 2, 3号站位置
Fig. 11. (a) Surface pressure disturbances, (b) surface wind filed, (c) divergence disturbances fields, (d) temperature disturbances (green solid lines denotes positive values, red dotted lines denotes negative values, units: ℃) on 14 BJT 29 July 2020. Green, red and yellow dot respectively denotes the locations of microbarograph 1, 2, 3.
图 12 2020年7月29日17:00 (a)地面气压扰动, (b) 风场, (c) 散度扰动, (d) 温度扰动(绿色等值线为正值, 红色虚线为负值, ℃). 绿色、红色、黄色圆点分别代表微压计1, 2, 3号站位置
Fig. 12. (a) Surface pressure disturbances, (b) surface wind filed, (c) divergence disturbances fields, (d) temperature disturbances (green solid lines denotes positive values, red dotted lines denotes negative values, units: ℃) on 17 BJT 29 July 2020. Green, red and yellow dot respectively denotes the locations of microbarograph 1, 2, 3.
图 13 2020年7月29日20:00 (a) 地面气压扰动, (b) 风场, (c) 散度扰动, (d) 温度扰动(绿色等值线为正值, 红色虚线为负值, ℃). 绿色、红色、黄色圆点分别代表微压计1, 2, 3号站位置
Fig. 13. (a) Surface pressure disturbances, (b) surface wind filed, (c) divergence disturbances fields, (d) temperature disturbances (green solid lines denotes positive values, red dotted lines denotes negative values, units: ℃) on 20 BJT 29 July 2020. Green, red and yellow dot respectively denotes the locations of microbarograph 1, 2, 3.
-
[1] 张云, 雷恒池, 钱贞成 2008 大气科学 32 481
 Google Scholar
Google Scholar
Zhang Y, Lei H C, Qian Z C 2008 Chin. J. Atmos. Sci. 32 481
 Google Scholar
Google Scholar
[2] 钟水新, 王东海, 张人禾, 刘英 2011 高原气象 30 951
Zhong S X, Wang D H, Zhang R H, Liu Y 2011 Plateau Meteorol. 30 951
[3] Koch S E, Dorian P B 1988 Mon. Weather Rev. 116 2570
 Google Scholar
Google Scholar
[4] Schumacher R S, Johnson R H 2008 Mon. Weather Rev. 136 3964
 Google Scholar
Google Scholar
[5] Plougonven R, Zhang F Q 2014 Rev. Geophys. 52 33
 Google Scholar
Google Scholar
[6] Trexler C M, Koch S E 2000 Mon. Weather Rev. 128 2423
 Google Scholar
Google Scholar
[7] Sato K 1993 J. Atmos. Sci. 50 518
 Google Scholar
Google Scholar
[8] Balachandran N K 1980 Mon. Weather Rev. 108 804
 Google Scholar
Google Scholar
[9] 李麦村 1978 大气科学 2 201
 Google Scholar
Google Scholar
Li M C 1978 Chin. J. Atmos. Sci. 2 201
 Google Scholar
Google Scholar
[10] Uccellini L W 1975 Mon. Weather Rev. 103 497
 Google Scholar
Google Scholar
[11] Fovell R, Durran D, Holton J R 1992 J. Atmos. Sci. 49 1427
 Google Scholar
Google Scholar
[12] Piani C, Durran D, Alexander M J, Holton J R 2000 J. Atmos. Sci. 57 3689
 Google Scholar
Google Scholar
[13] Ruppert J H, Bosart L F 2014 Mon. Weather Rev. 142 1403
 Google Scholar
Google Scholar
[14] Liu L, Ran L K, Gao S T 2018 Adv. Atmos. Sci. 35 604
 Google Scholar
Google Scholar
[15] Huang X, Zhou Y S, Liu L 2020 Atmosphere. 11 752
 Google Scholar
Google Scholar
[16] Herron T J, Tolstoy I 1969 J. Atmos. Sci. 26 266
 Google Scholar
Google Scholar
[17] Stobie J G, Einaudi F, Uccellini L W 1983 J. Atmos. Sci. 40 2804
 Google Scholar
Google Scholar
[18] Rees J M, Denholm-Price J C W, King J C, Anderson P S 2000 J. Atmos. Sci. 57 511
 Google Scholar
Google Scholar
[19] Hauf T, Finke U, Neisser J, Bull G, Stangenberg J G 1996 J. Atmos. Oceanic Technol. 13 1001
 Google Scholar
Google Scholar
[20] Curry M J, Murty R C 1974 J. Atmos. Sci. 31 1402
 Google Scholar
Google Scholar
[21] Grachev A I, Kulichkov S N, Otezov A I 1997 Izv. Atmos. Oceanic Phys. 33 583
[22] Kulichkov S N, Chunchuzov I P, Popov O E, Perepelkin V G, Gorchakov G I 2019 Izv. Atmos. Oceanic Phys. 55 167
 Google Scholar
Google Scholar
[23] Blanc E, Farges T, Pichon A Le, Heinrich P 2014 J. Geophys. Res. Atmos. 119 6409
 Google Scholar
Google Scholar
[24] 李启泰, 谢金来, 杨训仁 1993 气象学报 51 361
Li Q T, Xie J L, Yang X R 1993 Acta Meteorol. Sin. 51 361
[25] Wu L J, Wen Z P, He H Y 2015 Atmos. Oceanic Sci. Lett. 8 78
 Google Scholar
Google Scholar
[26] Yang R, Liu Y, Ran L K, Zhang Y L 2018 Chin. Phys. B 27 059201
 Google Scholar
Google Scholar
[27] Torrence C G, Compo G P 1998 Bull. Am. Meteorol. Soc. 79 61
 Google Scholar
Google Scholar
[28] Wang X J, Lei H C, Feng L, Zhu J S, Li Z M, Jiang Z B 2020 Atmos. Oceanic Sci. Lett. 13 163
 Google Scholar
Google Scholar
[29] Rotunno R, Klemp J B, Weisman M L 1988 J. Atmos. Sci. 45 463
 Google Scholar
Google Scholar
[30] Weisman M L, Rotunno R 2004 J. Atmos. Sci. 61 361
 Google Scholar
Google Scholar
计量
- 文章访问数: 6831
- PDF下载量: 90
- 被引次数: 0














 下载:
下载: