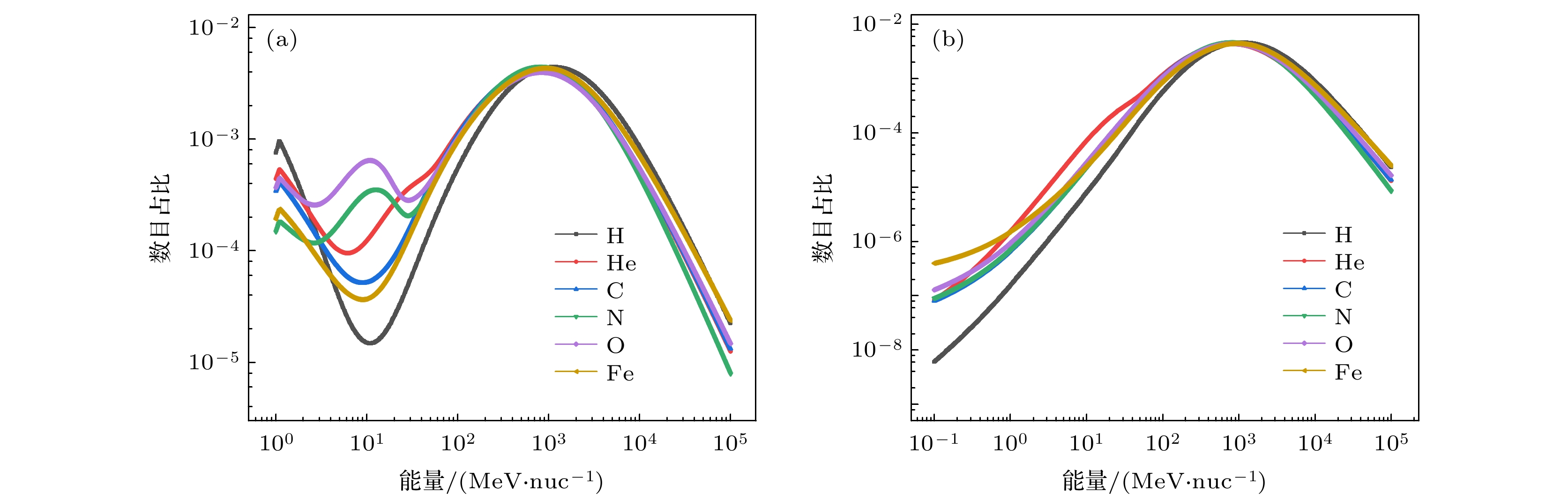
-
磷化铟(InP)具备电子迁移率高、禁带宽度大、耐高温、耐辐射等特性, 是制备空间辐射环境下电子器件的重要材料. 随着电子器件小型化, 单个重离子在器件灵敏体积内产生的位移损伤效应可能会导致其永久失效. 因此, 本文使用蒙特卡罗软件Geant4模拟空间重离子(碳、氮、氧、铁)在InP材料中的输运过程, 计算重离子的非电离能量损失(non-ionizing energy loss, NIEL), 得到重离子入射InP材料的位移损伤规律, 主要结论有: 1) NIEL值与原子序数的平方成正比, 重离子原子序数越大, 在InP材料中产生位移损伤的能力越强; 2)重离子NIEL比次级粒子NIEL大3—4个量级, 而NIEL与核弹性碰撞产生的反冲原子的非电离损伤能成正比, 说明重离子在材料中撞出的初级反冲原子是导致InP材料中产生位移损伤的主要原因; 3)空间辐射环境中重离子数目占比少, 一年中重离子在0.0125 mm3 InP中产生的总非电离损伤能占比为2.52%, 但重离子NIEL值是质子和α粒子的2—30倍, 仍需考虑单个空间重离子入射InP电子器件产生的位移损伤效应. 4)低能重离子在较厚材料中完全沉积导致平均非电离损伤能分布不均匀(前高后低), 使NIEL值随材料厚度的增大而略微减小, 重离子位移损伤严重区域分布在材料前端. 研究结果为InP材料在空间辐射环境中的应用打下基础.Indium phosphide (InP) has the characteristics of high electron mobility, large band gap, high temperature resistance, and radiation resistance. It is an important material of electronic devices in the space radiation environment. With the miniaturization of electronic devices, the displacement damage (DD) effect caused by a single heavy ion in the device may give rise to permanent failure. Therefore, this paper uses Monte Carlo software Geant4 to simulate the transportation process of space heavy ions(C, N, O, Fe) in InP. The non-ionizing energy loss (NIEL) of heavy ions is calculated for getting the information about displacement damage. Some conclusions are drawn as follows. 1) NIEL is proportional to the square of the atomic number, which means that single Fe can make severe displacement damage in InP. 2) The heavy ions NIEL is 3 to 4 orders of magnitude larger than PKA NIEL. The NIEL is proportional to the non-ionizing damage energy of recoil atoms produced by nuclear elastic collision, which indicates that the primary recoil atoms produced by heavy ions are the main cause of InP DD. 3) The number of heavy ions in space is small, so the proportion of total non-ionizing damage energy produced by heavy ions in 0.0125 mm3 InP is only 2.56% in one year. But the NIEL of heavy ions NIEL is 2–30 times that of protons and α particles, so the DD effect caused by single heavy ion incident on InP electronic device still needs to be considered. 4) NIEL decreases slightly with the increase of material thickness. The reason is that low-energy heavy ions are completely deposited in the front of InP, resulting in a non-uniform distribution of non-ionizing energy deposited in the material. Analyzing the dependence of mean DD energy with depth, we find that mean DD energy decreases with incident depth increasing, which means that the most severe DD region of heavy ions in InP is in the front of material.
-
Keywords:
- indium phosphide /
- displacement damage /
- Geant4 /
- non-ionizing energy loss
[1] Yamaguchi M, Araki K, Kojima N, Ohshita Y 2020 47th IEEE Photovoltaic Specialists Conference (PVSC) Calgary, OR, Canada, June 15–August 21, 2020 pp149–151
[2] O’Neill P M 2010 IEEE Trans. Nucl. Sci. 57 3148
Google Scholar
[3] Srour J R, Palko J W 2013 IEEE Trans. Nucl. Sci. 60 1740
Google Scholar
[4] Raine M, Jay A, Richard N, Goiffon V, Girard S, Gaillardin M, Paillet P 2017 IEEE Trans. Nucl. Sci. 64 133
Google Scholar
[5] Yamaguchi M, Uemura C, Yamamoto A 1984 J. Appl. Phys. 55 1429
Google Scholar
[6] Yamaguchi M, Ando K 1988 J. Appl. Phys. 63 5555
Google Scholar
[7] Walters R J, Messenger S R, Summers G P, Burke E A, Keavney C J 1991 IEEE Trans. Nucl. Sci. 38 1153
Google Scholar
[8] Keavney C J, Walters R J, Drevinsky P J 1993 J. Appl. Phys. 73 60
Google Scholar
[9] Walters R J 1995 Microelectronics J. 26 697
Google Scholar
[10] Messenger S R, County B, Road I H 1996 Solid. State. Electron. 39 797
Google Scholar
[11] Yamaguchi M, Takamoto T, Ohmori M 1997 J. Appl. Phys. 81 1116
Google Scholar
[12] Walters R J, Messenger S R, Summers G P, Romero M J, Al-Jassim M M, Araújo D, Garcia R 2001 J. Appl. Phys. 90 3558
Google Scholar
[13] Herre O, Wesch W, Wendler E, Gaiduk P, Komarov F 1998 Phys. Rev. B-Condens. Matter Mater. Phys. 58 4832
Google Scholar
[14] Gasparotto A, Carnera A, Frigeri C, Priolo F, Fraboni B, Camporese A, Rossetto G 1999 J. Appl. Phys. 85 753
Google Scholar
[15] Kamarou A, Wesch W, Wendler E, Undisz A, Rettenmayr M 2008 Phys. Rev. B - Condens. Matter Mater. Phys. 78 054111
Google Scholar
[16] Schnohr C S, Kluth P, Giulian R, Llewellyn D J, Byrne A P, Cookson D J, Ridgway M C 2010 Phys. Rev. B-Condens. Matter Mater. Phys. 81 1
Google Scholar
[17] Summers G P, Burke E A, Shapiro P, Messenger S R, Walters R J 1993 IEEE Trans. Nucl. Sci. 40 1372
Google Scholar
[18] Allison J, Amako K, Apostolakis J, et al. 2006 IEEE Trans. Nucl. Sci. 53 270
Google Scholar
[19] 申帅帅, 贺朝会, 李永宏 2018 67 182401
Google Scholar
Shen S S, He C H, Li Y H 2018 Acta Phys. Sin. 67 182401
Google Scholar
[20] 谢飞, 臧航, 刘方, 何欢, 廖文龙, 黄煜 2020 69 192401
Google Scholar
Xie F, Zang H, Liu F, He H, Liao W L, Huang Y 2020 Acta Phys. Sin. 69 192401
Google Scholar
[21] Garcia A R, Mendoza E, Cano-Ott D, Nolte R, Martinez T, Algora A, Tain J L, Banerjee K, Bhattacharya C 2017 Nucl. Instruments Methods Phys. Res. Sect. A: Accel. Spectrometers, Detect. Assoc. Equip. 868 73
Google Scholar
[22] 李兴冀, 刘超铭, 孙中亮, 兰慕杰, 肖立伊, 何世禹 2013 62 058502
Google Scholar
Li X J, Liu M C, Sun Z L, Lan M J, Xiao L Y, He S Y 2013 Acta Pyhs. Sin. 62 058502
Google Scholar
[23] Mendenhall M H, Weller R A 2005 Nucl. Instruments Methods Phys. Res. B. 227 420
Google Scholar
[24] Weller R A, Mendenhall M H, Fleetwod D M 2004 Trans. Nucl. Sci. 51 3669
Google Scholar
[25] Boberg P R, Brownstein B, Dietrich W F, Flueckiger E O, Petersen E L, Shea M A, Smart D F, Smith E C 1997 IEEE Trans. Nucl. Sci. 44 2150
Google Scholar
[26] Summers G P, Burke E A, Xapsos M A 1995 Radiat. Meas. 24 1
Google Scholar
[27] Jun I, Xapsos M A, Messenger S R, Burke E A, Walters R J, Summers G P, Jordan T 2003 IEEE Trans. Nucl. Sci. 50 1924
Google Scholar
[28] Robinson M T, Torrens L M 1974 Phys. Rev. B 8 15
Google Scholar
[29] Akkerman A, Barak J 2006 IEEE Trans. Nucl. Sci. 53 3667
Google Scholar
[30] Akkerman A, Barak J 2007 Nucl. Instrum. Methods Phys. Res. Sect. B: Beam Interact. Mater. Atoms 260 529
Google Scholar
[31] Jun I, Kim W, Evans R 2009 IEEE Trans. Nucl. Sci. 56 3229
Google Scholar
[32] 路伟, 王同权, 王兴功, 刘雪林 2011 核技术 34 529
Lu W, Wang T Q, Wang X G, Liu X L 2011 Nucl. Tech. 34 529
[33] Dale C G, Chen L, McNulty P J, Marshall P W, Burke E A 1994 IEEE Trans. Nucl. Sci. 41 197
Google Scholar
[34] Ziegler J F, Ziegler M D, Biersack J P 2010 Nucl. Instruments Methods Phys. Res. B 268 1818
Google Scholar
[35] Xapsos M A, Burke E A, Badavi F F, Townsend L W, Wilson J W, Jun I 2004 IEEE Trans. Nucl. Sci. 51 3250
Google Scholar
-
图 1 能谱图 (a)宇宙射线能谱图; (b) 100 mil Al屏蔽后的宇宙射线能谱图
Fig. 1. Energy spectrum: (a) Cosmic ray energy spectrum; (b) 100 mil Al shielded cosmic ray energy spectrum.
图 2 1−300 MeV质子入射(a) Si 和(b) InP的NIEL计算值
Fig. 2. 1−300 MeV proton NIEL for (a) Si and (b) InP.
图 3 不同种类重离子(a) C, (b) N, (c) O, (d) Fe入射5000 μm InP产生的平均非电离损伤能随深度分布图
Fig. 3. Distribution average non-ionization damage energy of different heavy ions (a) C, (b) N, (c) O, (d) Fe with depth in 5000 μm InP
表 1 Geant4模拟相关参数和NIEL计算值
Table 1. Geant4 Simulated parameters and NIEL.
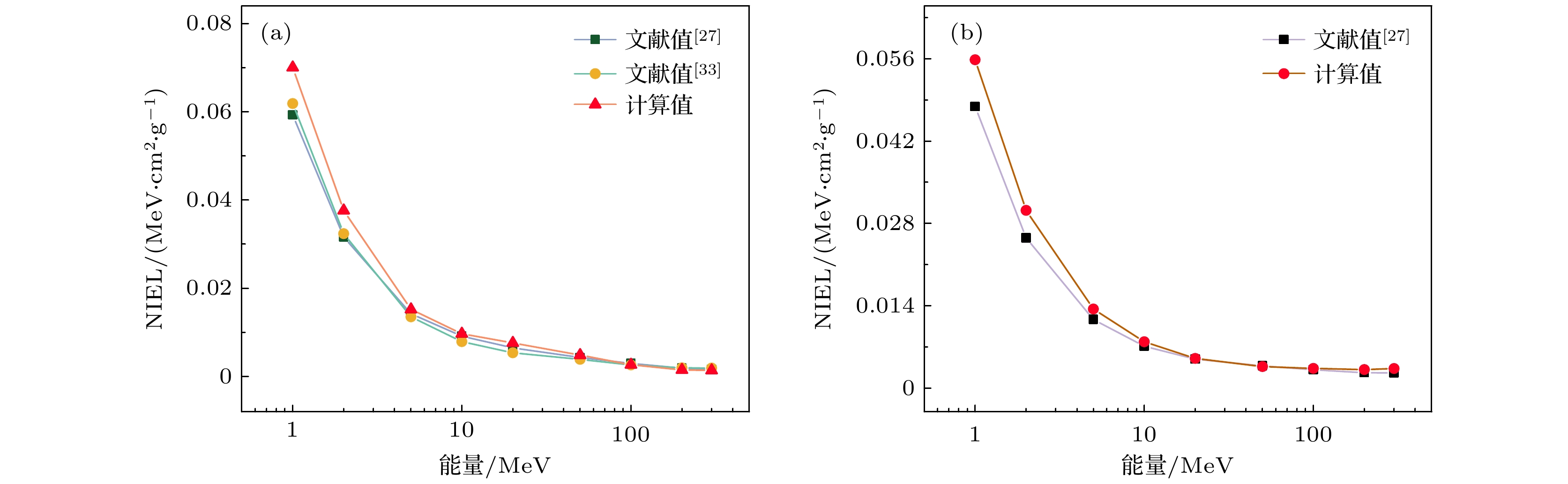
质子能量/MeV Si射程/mm Si厚度/mm NIEL/(MeV·cm2·g–1) InP射程/mm InP厚度/mm NIEL/(MeV·cm2·g–1) 1 0.016 0.0018 0.07004 0.013 0.0015 0.0558 2 0.048 0.0050 0.03763 0.038 0.0040 0.0302 5 0.216 0.0220 0.01519 0.164 0.0180 0.0135 10 0.709 0.0750 0.00968 0.518 0.0550 0.0079 20 2.390 0.2400 0.00759 1.680 0.2000 0.0051 50 12.180 1.2200 0.00483 8.320 1.0000 0.0037 100 41.620 4.1800 0.00265 27.530 3.0000 0.0034 200 138.630 14.0000 0.00148 90.270 9.5000 0.0032 300 273.570 28.0000 0.00138 176.860 18.0000 0.0033 
下载: 导出CSV
表 2 重离子入射InP材料的设计方案
Table 2. Design scheme of heavy ion incident on InP.
粒子种类 粒子数目 InP材料厚度/μm 方法一 H 106 500 He 106 500 C 106 500 N 106 500 O 106 500 Fe 106 500 方法二 H 12728631 500 He 1187039 500 C 30945 500 N 8389 500 O 29305 500 Fe 3200 500 方法三 C 106 500, 1000, 5000 N 106 500, 1000, 5000 O 106 500, 1000, 5000 Fe 106 500, 1000, 5000
下载: 导出CSV
表 3 宇宙射线粒子及其PKA在500 μm 厚的InP中产生的NIEL统计表
Table 3. NIEL of cosmic ray particles and their PKA produced in 500 μm InP.
粒子
种类统计
种类NIEL/
(MeV·cm2·g–1)NIEL
占比/%变异
系数H H 0.004316 98.365 0.03953 PKA 7.1739×10–5 1.635 0.08716 He He 0.00861 96.443 0.02208 PKA 3.17556×10–4 3.557 0.04532 C C 0.0165 99.906 0.01073 PKA 1.54785×10–5 0.094 0.20895 N N 0.01798 99.928 0.01309 PKA 1.2888×10–5 0.072 0.30657 O O 0.02132 99.936 0.01548 PKA 1.3566×10–5 0.064 0.20082 Fe Fe 0.11922 99.976 0.00507 PKA 2.9332×10–5 0.024 0.15543
下载: 导出CSV
表 4 不同粒子在0.125 mm3 InP产生的非电离损伤能统计表
Table 4. Total non-ionization damage energy produced by cosmic particles in 0.125 mm3 InP.
粒子
种类入射
数目非电离
损伤能/MeV非电离损
伤能占比/%变异
系数H 12728631 12380.55 82.14 0.01366 He 1187039 2312.76 15.34 0.02426 C 30945 116.995 0.78 0.07564 N 8389 33.99 0.23 0.01548 O 29304 142.74 0.95 0.05274 Fe 3200 86.27 0.56 0.01301
下载: 导出CSV
表 5 重离子在500, 1000, 5000 μm InP产生的NIEL统计表
Table 5. NIEL of heavy ion produced in 500, 1000, 5000 μm InP.
重离子种类 材料厚度/μm NIEL均值 变异系数 C 500 0.0165 0.01073 1000 0.01639 0.00631 5000 0.01539 0.00664 N 500 0.01798 0.01309 1000 0.01755 0.01031 5000 0.01628 0.00723 O 500 0.02132 0.01548 1000 0.02087 0.00724 5000 0.01878 0.00349 Fe 500 0.11922 0.00507 1000 0.11591 0.00382 5000 0.09486 0.00303
下载: 导出CSV
-
[1] Yamaguchi M, Araki K, Kojima N, Ohshita Y 2020 47th IEEE Photovoltaic Specialists Conference (PVSC) Calgary, OR, Canada, June 15–August 21, 2020 pp149–151
[2] O’Neill P M 2010 IEEE Trans. Nucl. Sci. 57 3148
Google Scholar
[3] Srour J R, Palko J W 2013 IEEE Trans. Nucl. Sci. 60 1740
Google Scholar
[4] Raine M, Jay A, Richard N, Goiffon V, Girard S, Gaillardin M, Paillet P 2017 IEEE Trans. Nucl. Sci. 64 133
Google Scholar
[5] Yamaguchi M, Uemura C, Yamamoto A 1984 J. Appl. Phys. 55 1429
Google Scholar
[6] Yamaguchi M, Ando K 1988 J. Appl. Phys. 63 5555
Google Scholar
[7] Walters R J, Messenger S R, Summers G P, Burke E A, Keavney C J 1991 IEEE Trans. Nucl. Sci. 38 1153
Google Scholar
[8] Keavney C J, Walters R J, Drevinsky P J 1993 J. Appl. Phys. 73 60
Google Scholar
[9] Walters R J 1995 Microelectronics J. 26 697
Google Scholar
[10] Messenger S R, County B, Road I H 1996 Solid. State. Electron. 39 797
Google Scholar
[11] Yamaguchi M, Takamoto T, Ohmori M 1997 J. Appl. Phys. 81 1116
Google Scholar
[12] Walters R J, Messenger S R, Summers G P, Romero M J, Al-Jassim M M, Araújo D, Garcia R 2001 J. Appl. Phys. 90 3558
Google Scholar
[13] Herre O, Wesch W, Wendler E, Gaiduk P, Komarov F 1998 Phys. Rev. B-Condens. Matter Mater. Phys. 58 4832
Google Scholar
[14] Gasparotto A, Carnera A, Frigeri C, Priolo F, Fraboni B, Camporese A, Rossetto G 1999 J. Appl. Phys. 85 753
Google Scholar
[15] Kamarou A, Wesch W, Wendler E, Undisz A, Rettenmayr M 2008 Phys. Rev. B - Condens. Matter Mater. Phys. 78 054111
Google Scholar
[16] Schnohr C S, Kluth P, Giulian R, Llewellyn D J, Byrne A P, Cookson D J, Ridgway M C 2010 Phys. Rev. B-Condens. Matter Mater. Phys. 81 1
Google Scholar
[17] Summers G P, Burke E A, Shapiro P, Messenger S R, Walters R J 1993 IEEE Trans. Nucl. Sci. 40 1372
Google Scholar
[18] Allison J, Amako K, Apostolakis J, et al. 2006 IEEE Trans. Nucl. Sci. 53 270
Google Scholar
[19] 申帅帅, 贺朝会, 李永宏 2018 67 182401
Google Scholar
Shen S S, He C H, Li Y H 2018 Acta Phys. Sin. 67 182401
Google Scholar
[20] 谢飞, 臧航, 刘方, 何欢, 廖文龙, 黄煜 2020 69 192401
Google Scholar
Xie F, Zang H, Liu F, He H, Liao W L, Huang Y 2020 Acta Phys. Sin. 69 192401
Google Scholar
[21] Garcia A R, Mendoza E, Cano-Ott D, Nolte R, Martinez T, Algora A, Tain J L, Banerjee K, Bhattacharya C 2017 Nucl. Instruments Methods Phys. Res. Sect. A: Accel. Spectrometers, Detect. Assoc. Equip. 868 73
Google Scholar
[22] 李兴冀, 刘超铭, 孙中亮, 兰慕杰, 肖立伊, 何世禹 2013 62 058502
Google Scholar
Li X J, Liu M C, Sun Z L, Lan M J, Xiao L Y, He S Y 2013 Acta Pyhs. Sin. 62 058502
Google Scholar
[23] Mendenhall M H, Weller R A 2005 Nucl. Instruments Methods Phys. Res. B. 227 420
Google Scholar
[24] Weller R A, Mendenhall M H, Fleetwod D M 2004 Trans. Nucl. Sci. 51 3669
Google Scholar
[25] Boberg P R, Brownstein B, Dietrich W F, Flueckiger E O, Petersen E L, Shea M A, Smart D F, Smith E C 1997 IEEE Trans. Nucl. Sci. 44 2150
Google Scholar
[26] Summers G P, Burke E A, Xapsos M A 1995 Radiat. Meas. 24 1
Google Scholar
[27] Jun I, Xapsos M A, Messenger S R, Burke E A, Walters R J, Summers G P, Jordan T 2003 IEEE Trans. Nucl. Sci. 50 1924
Google Scholar
[28] Robinson M T, Torrens L M 1974 Phys. Rev. B 8 15
Google Scholar
[29] Akkerman A, Barak J 2006 IEEE Trans. Nucl. Sci. 53 3667
Google Scholar
[30] Akkerman A, Barak J 2007 Nucl. Instrum. Methods Phys. Res. Sect. B: Beam Interact. Mater. Atoms 260 529
Google Scholar
[31] Jun I, Kim W, Evans R 2009 IEEE Trans. Nucl. Sci. 56 3229
Google Scholar
[32] 路伟, 王同权, 王兴功, 刘雪林 2011 核技术 34 529
Lu W, Wang T Q, Wang X G, Liu X L 2011 Nucl. Tech. 34 529
[33] Dale C G, Chen L, McNulty P J, Marshall P W, Burke E A 1994 IEEE Trans. Nucl. Sci. 41 197
Google Scholar
[34] Ziegler J F, Ziegler M D, Biersack J P 2010 Nucl. Instruments Methods Phys. Res. B 268 1818
Google Scholar
[35] Xapsos M A, Burke E A, Badavi F F, Townsend L W, Wilson J W, Jun I 2004 IEEE Trans. Nucl. Sci. 51 3250
Google Scholar
下载:
计量
- 文章访问数: 4890
- PDF下载量: 149
- 被引次数: 0
