-
This article proposed an equivalent model to calculate the magnetic field of a special multipole magnet. The special multipole magnet is formed when two permanent magnets of large dimension differences are forced into contact with the same polarity, after the removal of the small magnet, the large magnet becomes the multipole magnet. In the process, the interacting force between the two magnets changes from repulsive force to attractive force as the two magnets approach. Moreover, the reversed pole of the multipole magnet occupies an area roughly the same as the contact area of the two magnets. Qualitatively, the large magnet possesses a lower load line than the small magnet, which suggests that the large magnet is prone to irreversible demagnetization, whereas the small magnet tends to remain unperturbed. Quantitatively, taking axially magnetized cylindrical magnets as examples, the equations for the magnetic fields were derived based on the magnetizing current theory under the assumption that the magnetization of the multipole magnet is locally homogeneous. To validate our equivalent model, two special multipole magnets (model A and model B) have been studied both theoretically and experimentally. Model A was obtained by a large magnet (
$\Phi40\times2.5$ ) demagnetized at the center by a small magnet ($\Phi12\times18$ ), model B was obtained by demagnetizing model A with 4 extra small magnets ($\Phi6\times20$ ) at specific symmetrical positions around the center. Measurements for the magnetic induction intensity of the special multipole magnets are in good agreement with the theoretical calculations. The results suggested that the special multipole magnets of model A and B are equivalent to ring magnets and porous magnets, where the near field magnetic induction of the multipole magnets can be adjusted by the small magnets. In addition, a parameter analysis was carried out to study the influence of small magnets on the special multipole magnets. The results indicated that the reversed pole behavior of the special multipole magnet works mainly at positions near the magnet, and decreases rapidly as the observation point moves away from the reversed area. Our model may provide a theoretical basis and alternative solutions for electromechanical systems using multipole magnets.[1] Coey J M D 2002 J. Magn. Magn. Mater. 248 3
[2] Lu Z, Wang Z X, Zhou Y, Lu X L 2018 J. Sound Vib. 423 18
Google Scholar
[3] Fu H L, Theodossiades S, Gunn B, Abdallah I, Chatzi, E 2020 Nonlinear Dyn. 101 4
[4] Cottone F, Vocca H, Gammaitoni L 2009 Phys. Rev. Lett. 102 080601
Google Scholar
[5] Lai S K, Wang C, Zhang L H 2019 Mech. Syst. Signal Process 122 87
Google Scholar
[6] Leng Y G, Tan D, Liu J J, Zhang Y Y, Fan S B 2017 J. Sound Vib. 406 146
Google Scholar
[7] Schomburg W K, Reinertz O, Sackmann J, Schmitz K 2020 J. Magn. Magn. Mater. 506 166694
Google Scholar
[8] Peng Q L 2007 J. Magn. Magn. Mater. 309 1
Google Scholar
[9] Zhang Y Y, Leng Y G, Tan D, Liu J J 2018 J. Magn. 23 4
[10] Caciagli A, Baars R J, Philipse A P, Kuipers B W 2018 J. Magn. Magn. Mater. 456 423
Google Scholar
[11] Zhang Y Y, Leng Y G, Liu J J, Tan D 2019 J. Magn. 24 3
[12] Zhang Y Y, Leng Y G 2020 Int. J. Appl. Electromagn. Mech. 62 2
[13] Ruoho S, Arkkio A 2008 IEEE Trans. Magn. 44 7
[14] Campbell P 1996 Permanent Magnet Materials and Their Application (Cambridge, U.K.: Cambridge University Press) pp64–65
[15] 孙帅令, 冷永刚, 张雨阳, 苏徐昆, 范胜波 2020 69 140502
Google Scholar
Sun S L, Leng Y G, Zhang Y Y, Su X K, Fan S B 2020 Acta Phys. Sin. 69 140502
Google Scholar
[16] Shin H S, Jang G H, Choi J Y 2020 AIP Adv. 10 1
[17] Ponce E A, Leeb S B 2020 IEEE Trans. Magn. 56 3
Google Scholar
[18] O’Connell J L, Robertson W S, Cazzolato B S 2020 J. Magn. Magn. Mater. 510 166894
Google Scholar
[19] De Visschere P 2005 J. Phys. D: Appl. Phys. 38 3
[20] Nguyen V T, Lu T F 2019 J. Magn. Magn. Mater. 491 165569
Google Scholar
[21] Landau L D, Lifschitz E M 1975 The Classical Theory of Fields (Vol. 2) (Oxford: Pergamon Press) pp89−108
[22] Landau L D, Lifschitz E M 1984 Electrodynamics of Continuous Media (Vol. 8) (Oxford: Pergamon Press) pp105−113
-
图 1 圆柱形磁铁表面多处接触反磁化示意图
Fig. 1. Schematic diagram of a cylindrical magnet with multiple local demagnetization on the surface.
图 2 (a), (b) 磁显片下
$ \varPhi \;40\times2.5 $ 和$ \varPhi \;12\times18 $ 的正常磁铁; (c) 中心接触反磁化结果: 小磁铁被吸附在大磁铁中心; (d) 磁感应强度测量实验装置Fig. 2. (a), (b) Normal magnets of size
$ \varPhi \;40\times2.5 $ and$ \varPhi \;12\times18 $ under magnetic field viewing film; (c) the small magnet is observed to be attracted to the center of the large magnet after the local demagnetization process at the center of the large magnet; (d) experiment setup for the measurement of magnetic induction intensity.
图 3 正常磁铁轴向磁感应强度
$ B_z(z) $ . 红色圆点为实验测量结果, 蓝色直线为磁化电流模型计算结果 (a) 大磁铁$ \varPhi \;40\times2.5 $ ; (b) 小磁铁$ \varPhi \;12\times18 $ Fig. 3. The axial magnetic induction intensity
$ B_z(z) $ of a normal magnet. Red dot denotes the experimental data whereas the blue line denotes the theoretical results: (a) Larger magnet$ \varPhi \;40\times2.5 $ ; (b) smaller magnet$ \varPhi \;12\times18 $ .
图 4 模型A: 仅中心接触反磁化的圆柱磁铁 (a) 反常磁铁示意图; (b) 磁显片下的反常磁铁
Fig. 4. Model A: Cylindrical magnet with local demagnetization at the center: (a) Diagram of the abnormal magnet; (b) the abnormal magnet under magnetic field viewing film.
图 5 (a) 模型A反常磁铁轴向磁感应强度
$ B_z(z) $ . 红色圆点为实验测量结果, 蓝色直线为磁化电流模型计算结果. (b) 正常圆柱形磁铁, 反常磁铁和正常环形磁铁轴向磁感应强度, 其中三种磁铁外形尺寸均一致Fig. 5. (a) The axial magnetic induction intensity
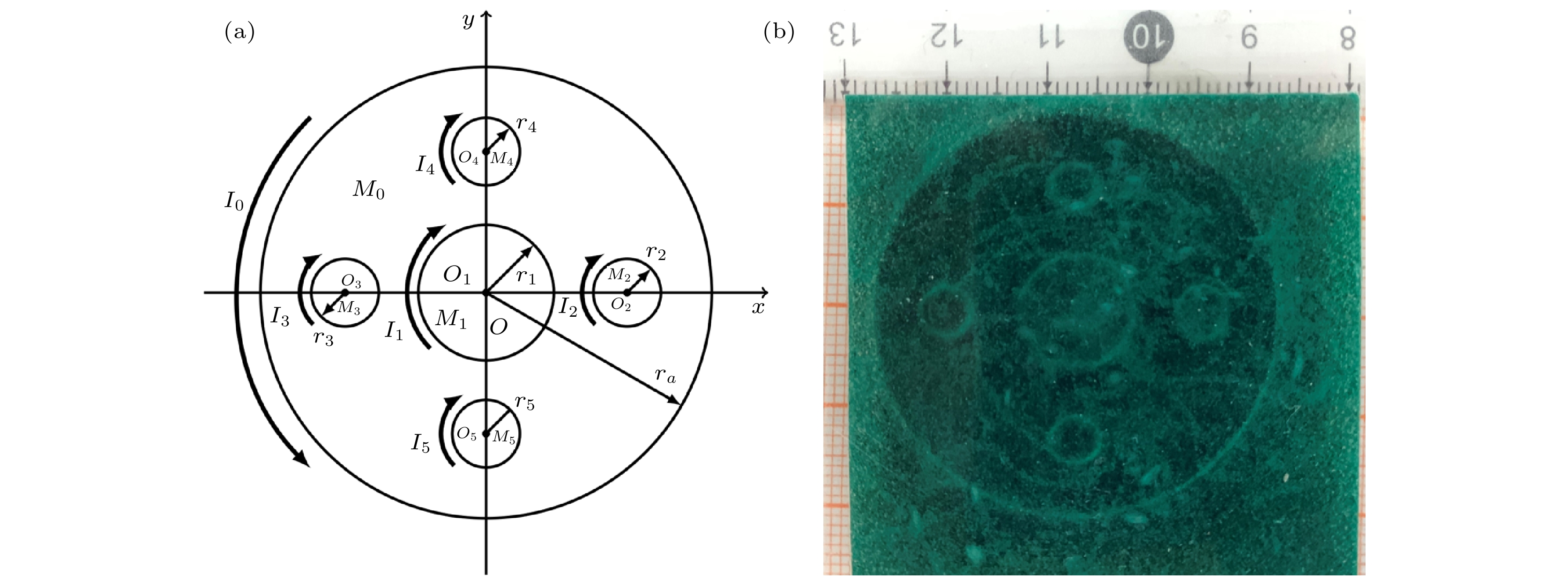
$ B_z(z) $ for the abnormal magnet. Red dot denotes the experimental data whereas the blue line denotes the theoretical results. (b) The axial magnetic induction intensity$ B_z(z) $ of a normal cylindrical magnet, an abnormal magnet and a normal ring magnet, of which the dimensions of the three are the same.
图 6 模型B: 中心及轨道反磁化的圆柱形磁铁 (a) 反常磁铁示意图. 为便于阅读, 图中四个小圆的半径放大了一倍. (b) 磁显片下的反常磁铁
Fig. 6. Model B: Cylindrical magnet with local demagnetization at both the center and the orbit: (a) Diagram of the abnormal magnet. For the convenience of reading, the radii of the four small circles in the figure are doubled. (b) The abnormal magnet under magnetic field viewing film.
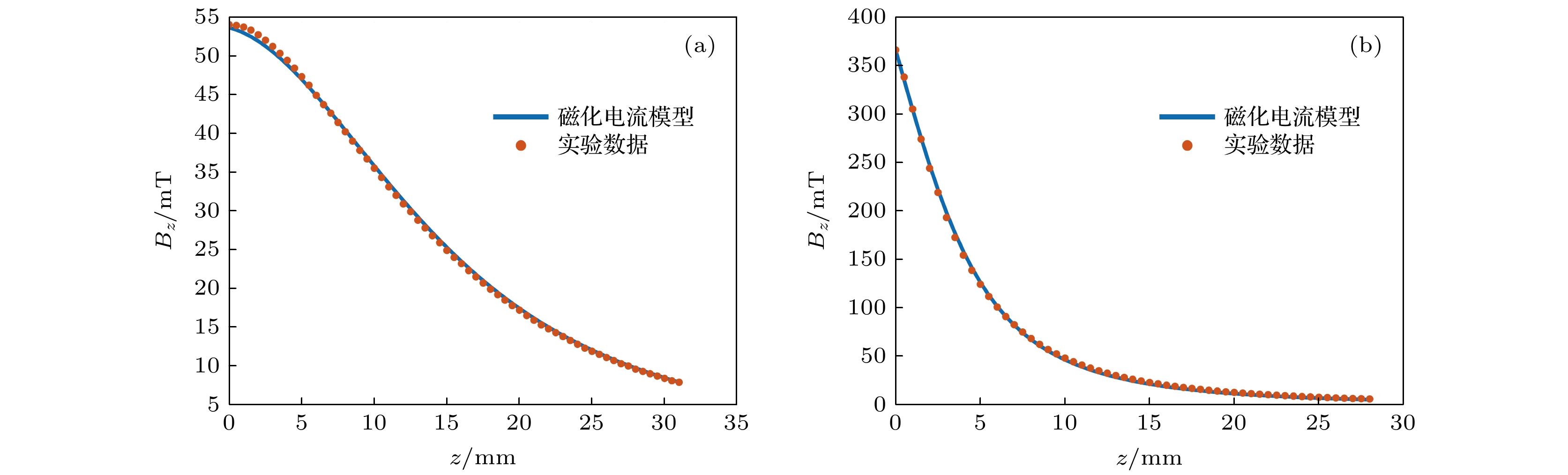
图 7 (a) 模型A, B反常磁铁轴向磁感应强度的实验数据; (b) 模型B反常磁铁轴向磁感应强度. 蓝色圆点为实验测量结果, 红色直线为磁化电流模型计算结果
Fig. 7. (a) Experimental data of axial magnetic induction for abnormal magnets of type A and B; (b) the axial magnetic induction intensity of the abnormal magnet of type B. Blue circle denotes the experimental data whereas the red line denotes the theoretical results.
图 8 (a) 在
$ z = 3 \;{\rm{mm}} $ 平面上, 模型B反常磁铁的水平磁感应强度$ B_x(x) $ , 红色圆点为实验测量结果, 蓝色直线为磁化电流模型计算结果; (b) 在$ z = 3 \;{\rm{mm}} $ 平面上, 模型A, B反常磁铁及正常磁铁的水平磁感应强度$ B_x(x) $ 的对比Fig. 8. (a) The horizontal magnetic induction intensity
$ B_x(x) $ of the abnormal magnet of type B at$ z = 3 \;{\rm{mm}} $ . Red circle denotes the experimental data whereas the blue line denotes the theoretical results. (b) Comparison of horizontal magnetic induction intensity$ B_x(x) $ of the abnormal magnets of type A and B and a normal magnet with the same size at$ z = 3 \;{\rm{mm}} $ .
图 9 不同厚度小磁铁 (a)
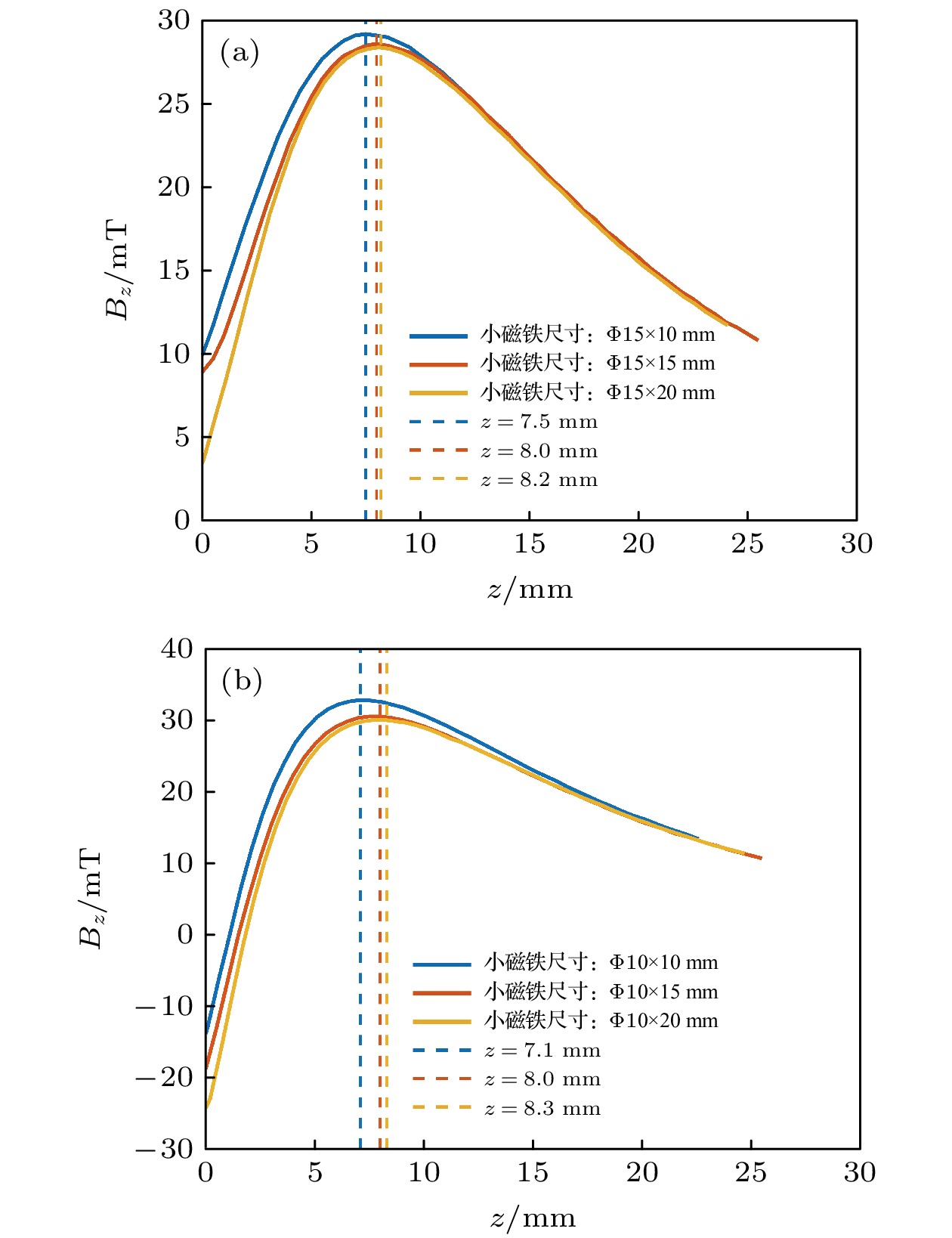
$ \Phi15\times10 $ ,$ \Phi15\times15 $ 和$ \Phi15\times20 $ 以及(b)$ \Phi10\times10 $ ,$ \Phi10\times15 $ 和$ \Phi10\times20 $ 接触反磁化大磁铁$ \Phi40\times2.5 $ 后得到的反常磁铁, 其沿$ z $ 轴的轴向磁感应强度$ B_z(z) $ Fig. 9. The axial magnetic induction
$ B_z(z) $ of larger magnet$ \Phi40\times2.5 $ demagnetized by smaller magnets of different heights with (a)$ \Phi15\times10 $ ,$ \Phi15\times15 $ ,$ \Phi15\times20 $ and (b)$ \Phi10\times10 $ ,$ \Phi10\times15 $ ,$ \Phi10\times20 $ .
图 10 由小磁铁
$\Phi15\times20$ 和$\Phi15\times10$ 接触反磁化形成的反常磁铁C, D, 在远离磁铁表面$ z = 5 \;{\rm{mm}} $ 处二者磁感应强度的差值: (a)反常磁铁C与D的切向磁感应强度差值$ B_{\rm t} $ ; (b) 反常磁铁C与D的法向磁感应强度差值$ B_{\rm n} $ ; (c) 反常磁铁C与D的磁感应强度的绝对值的差值$ B_{\rm{abs}} $ Fig. 10. Abnormal magnets C, D are locally demagnetized by smaller magnets of size
$\Phi 15\times20$ and$\Phi 15\times10$ at the center, the difference of magnetic induction between C and D at the plane$ 5 \;{\rm{mm}} $ away from the abnormal magnets' surface: (a) The tangential difference$ B_{t} $ ; (b) the axial difference$ B_{\rm n} $ ; (c) the difference of absolute value$ B_{\rm{abs}} $ .表 1 A, B两组模型材料参数
Table 1. Material parameters of the two models
组别 A B 大磁铁尺寸/mm $\varPhi \;40\times2.5$ $\varPhi \;40\times2.5$ 小磁铁a尺寸/mm $\varPhi \;12\times18$ $\varPhi \;12\times18$ 小磁铁b尺寸/mm Null $\varPhi \; 6\times20$ 
下载: 导出CSV
表 2 小磁铁尺寸、磁化强度及相应反常磁铁指标
Table 2. The size and magnetization of small magnet and the indicators of corresponding abnormal magnet
小磁铁尺寸/mm 小磁铁磁化强度/(A·m-1) 反常磁铁转变点位置/mm 反常磁铁的$B_{{\rm s}z}$/mT $\Phi15\times10$ $6.565\times10^5$ $7.5$ 9.9 $\Phi 15\times15$ $6.614\times10^5$ $8.0$ 8.9 $\Phi 15\times20$ $6.712\times10^5$ $8.2$ 3.4 $\Phi 10\times10$ $6.103\times10^5$ $7.1$ –13.9 $\Phi 10\times15$ $6.207\times10^5$ $8.0$ –18.8 $\Phi 10\times20$ $6.365\times10^5$ $8.3$ –24.3
下载: 导出CSV
-
[1] Coey J M D 2002 J. Magn. Magn. Mater. 248 3
[2] Lu Z, Wang Z X, Zhou Y, Lu X L 2018 J. Sound Vib. 423 18
Google Scholar
[3] Fu H L, Theodossiades S, Gunn B, Abdallah I, Chatzi, E 2020 Nonlinear Dyn. 101 4
[4] Cottone F, Vocca H, Gammaitoni L 2009 Phys. Rev. Lett. 102 080601
Google Scholar
[5] Lai S K, Wang C, Zhang L H 2019 Mech. Syst. Signal Process 122 87
Google Scholar
[6] Leng Y G, Tan D, Liu J J, Zhang Y Y, Fan S B 2017 J. Sound Vib. 406 146
Google Scholar
[7] Schomburg W K, Reinertz O, Sackmann J, Schmitz K 2020 J. Magn. Magn. Mater. 506 166694
Google Scholar
[8] Peng Q L 2007 J. Magn. Magn. Mater. 309 1
Google Scholar
[9] Zhang Y Y, Leng Y G, Tan D, Liu J J 2018 J. Magn. 23 4
[10] Caciagli A, Baars R J, Philipse A P, Kuipers B W 2018 J. Magn. Magn. Mater. 456 423
Google Scholar
[11] Zhang Y Y, Leng Y G, Liu J J, Tan D 2019 J. Magn. 24 3
[12] Zhang Y Y, Leng Y G 2020 Int. J. Appl. Electromagn. Mech. 62 2
[13] Ruoho S, Arkkio A 2008 IEEE Trans. Magn. 44 7
[14] Campbell P 1996 Permanent Magnet Materials and Their Application (Cambridge, U.K.: Cambridge University Press) pp64–65
[15] 孙帅令, 冷永刚, 张雨阳, 苏徐昆, 范胜波 2020 69 140502
Google Scholar
Sun S L, Leng Y G, Zhang Y Y, Su X K, Fan S B 2020 Acta Phys. Sin. 69 140502
Google Scholar
[16] Shin H S, Jang G H, Choi J Y 2020 AIP Adv. 10 1
[17] Ponce E A, Leeb S B 2020 IEEE Trans. Magn. 56 3
Google Scholar
[18] O’Connell J L, Robertson W S, Cazzolato B S 2020 J. Magn. Magn. Mater. 510 166894
Google Scholar
[19] De Visschere P 2005 J. Phys. D: Appl. Phys. 38 3
[20] Nguyen V T, Lu T F 2019 J. Magn. Magn. Mater. 491 165569
Google Scholar
[21] Landau L D, Lifschitz E M 1975 The Classical Theory of Fields (Vol. 2) (Oxford: Pergamon Press) pp89−108
[22] Landau L D, Lifschitz E M 1984 Electrodynamics of Continuous Media (Vol. 8) (Oxford: Pergamon Press) pp105−113
下载:
计量
- 文章访问数: 5974
- PDF下载量: 84
- 被引次数: 0
