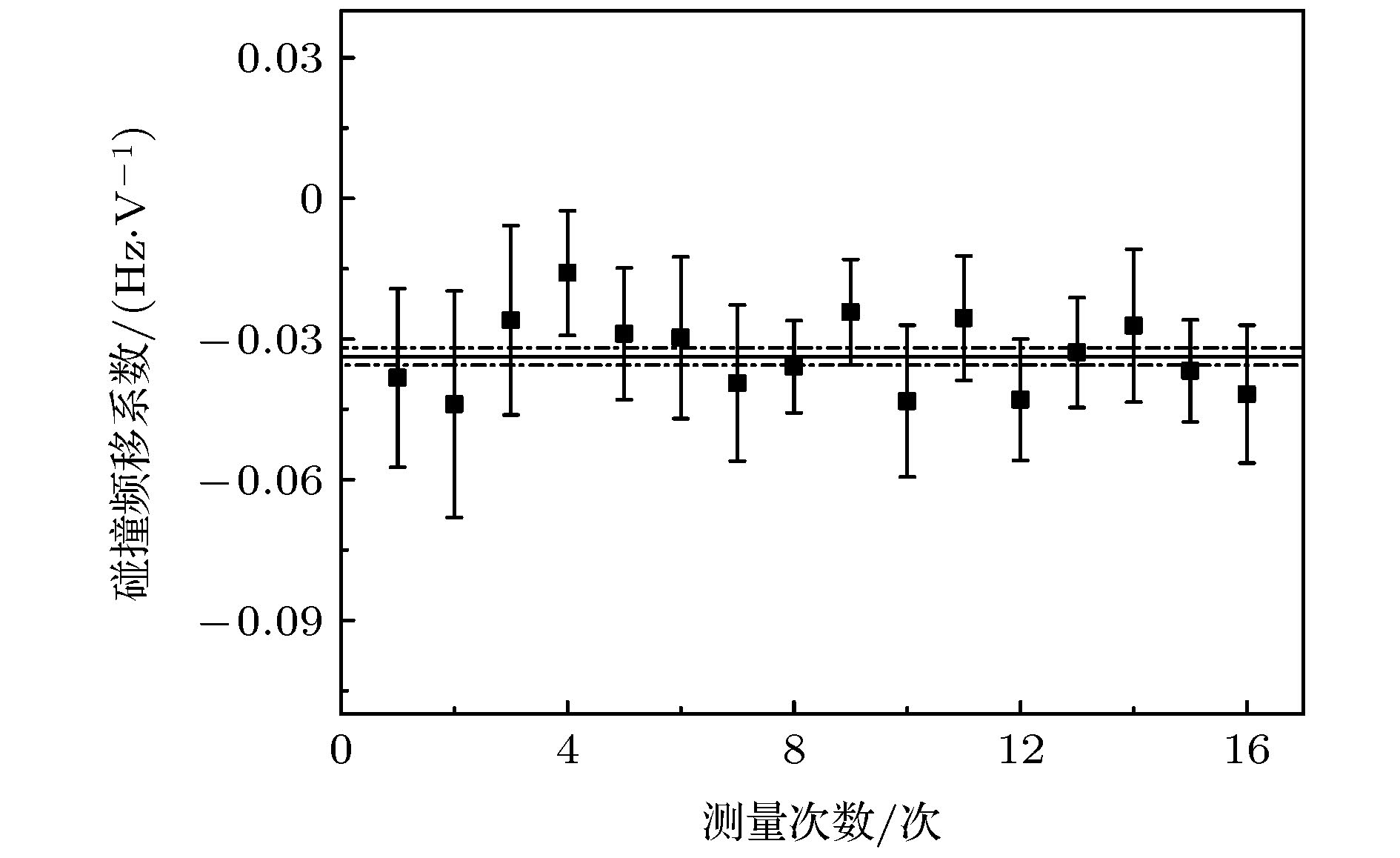
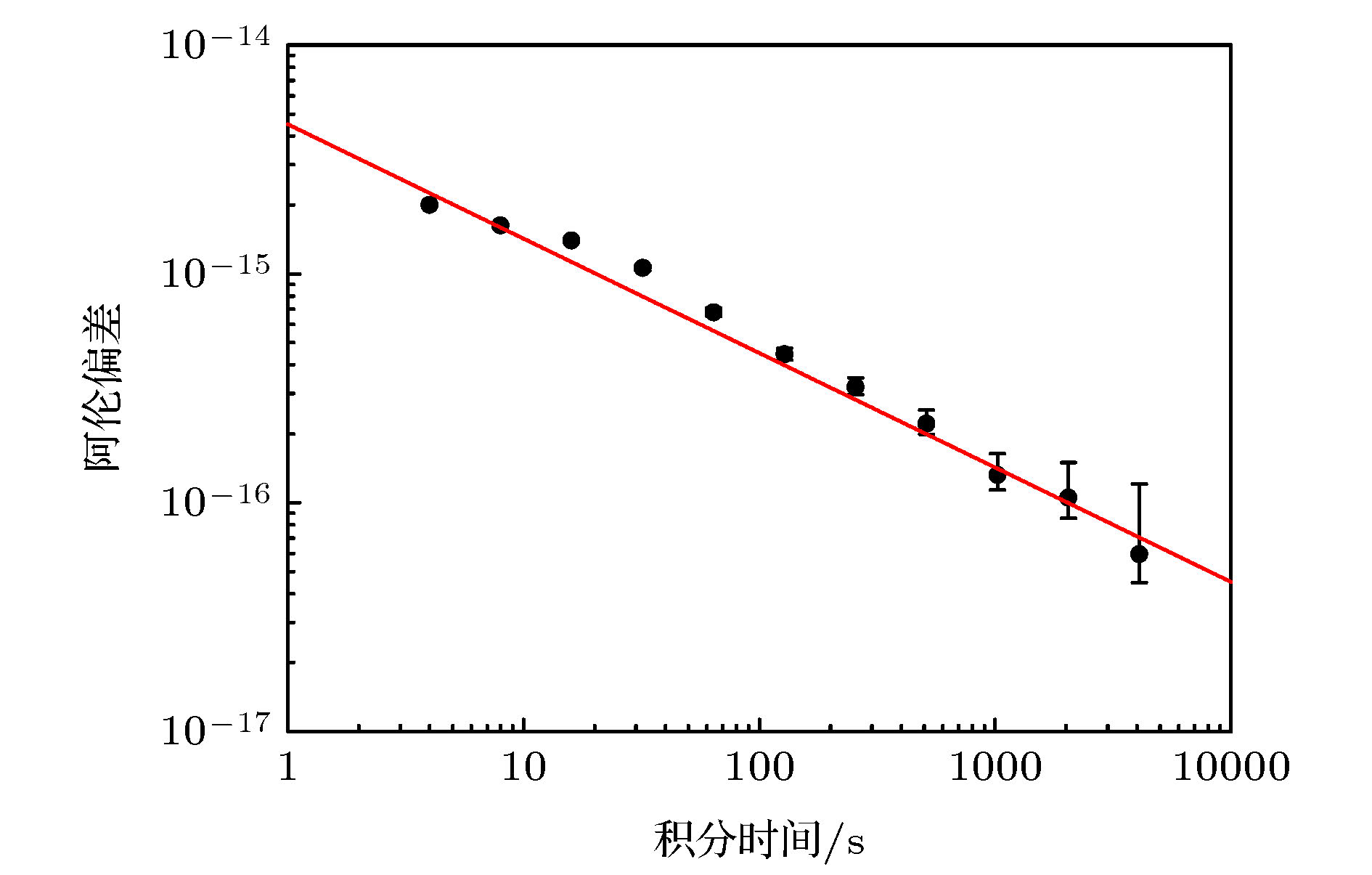
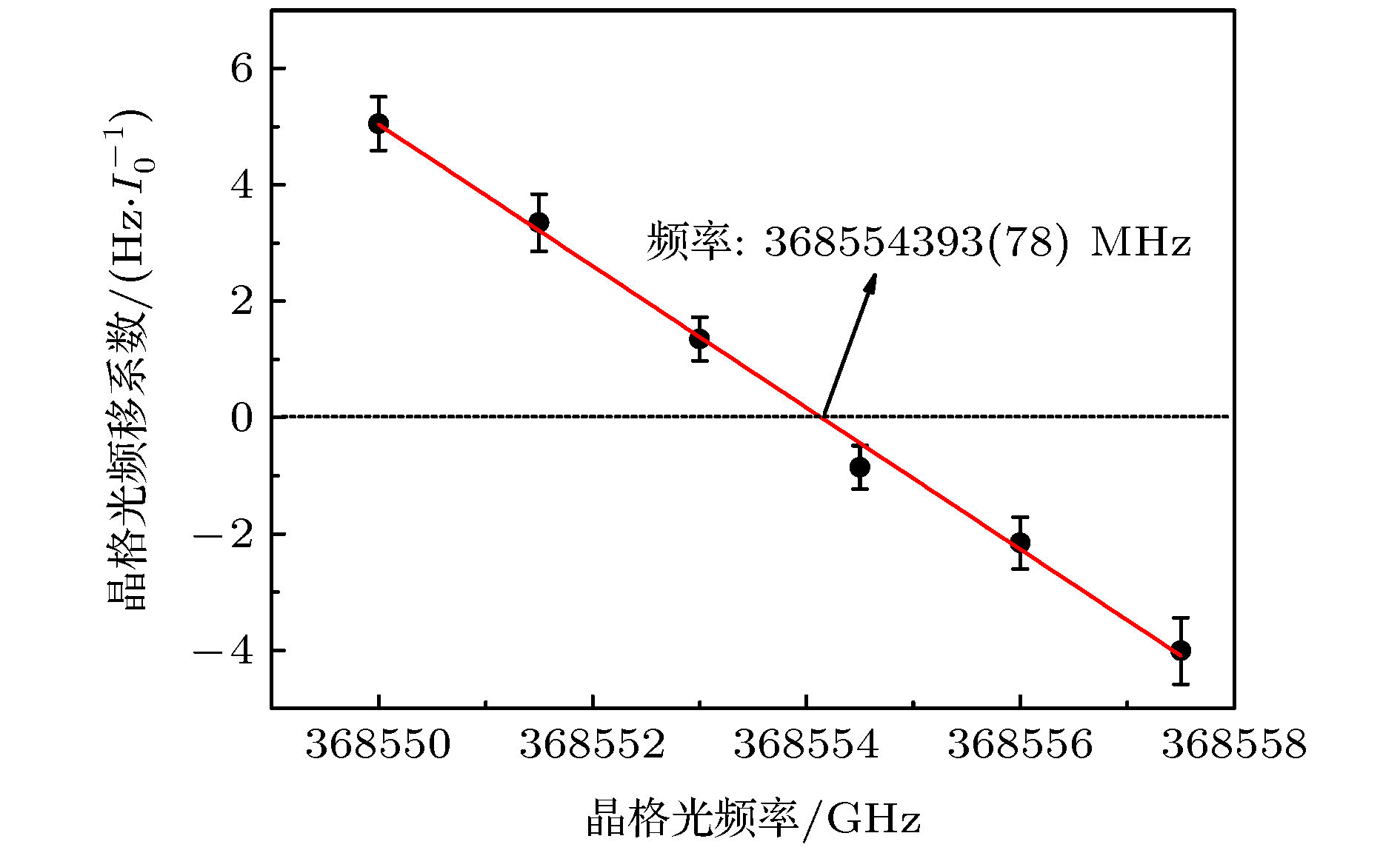
-
可搬运光学原子钟在科学研究和工程应用中具有重要意义. 本文测量了可搬运87Sr光晶格钟系统的主要频移, 包括黑体辐射频移、碰撞频移、晶格光交流斯塔克频移、二阶塞曼频移等. 首先实验上测量了磁光阱腔体表面的温度分布, 分析了不同热源对原子团的影响, 得到黑体辐射总的相对频移修正量为50.4 × 10–16, 相对不确定度为5.1 × 10–17. 然后利用分时自比对方法, 评估了碰撞频移、晶格光交流斯塔克频移和二阶塞曼频移. 结果表明, 由黑体辐射引起的频移量最大, 晶格光交流斯塔克频移的不确定度最大, 系统总的相对频移修正量为58.8 × 10–16, 总不确定度为2.3 × 10–16. 该工作为可搬运87Sr光晶格钟之后的性能提升和应用提供了条件.
-
关键词:
- 可搬运87Sr光晶格钟 /
- 系统频移 /
- 不确定度 /
- 分时自比对方法
Transportable optical clocks have broad applications in scientific research and engineering. Accurate evaluation of systematic uncertainty for the transportable 87Sr optical lattice clock is a prerequisite for the practical realization of the optical clock. Four main frequency shifts of the 87Sr optical lattice clock are measured, i.e. blackbody-radiation (BBR) shift, collision shift, lattice alternating current (AC) Stark shift, and second-order Zeeman shift. Firstly, by measuring the temperature distribution on the surface of the magneto-optical trap cavity and analyzing the influence of different heat sources on atomic cloud, the BBR shift correction is measured to be 50.4 × 10–16 Hz with an uncertainty of 5.1 × 10–17. Secondly, the time-interleaved self-comparison method is used under high and low atom density condition to evaluate the collision shift of the system. The correction of collision shift is 4.7 × 10–16 with an uncertainty of 5.6 × 10–17. Thirdly, the lattice AC Stark shift is evaluated by the time-interleaved self-comparison method. By measuring the dependence of the lattice AC Stark shift on the wavelength of the lattice light, the magic wavelength is measured to be 368554393(78) MHz. As a result, the lattice AC Stark shift correction is 3.0 × 10–16 with an uncertainty of 2.2 × 10–16. Finally, using the time-interleaved self-comparison technology, the second-order Zeeman frequency shift is evaluated by measuring the fluctuation of the difference in center frequency between the${m_{\text{F}}} = + {9 / 2} \to {m_{\text{F}}} = + {9 / 2}$ polarization spectrum and${m_{\text{F}}} = - {9 / 2} \to {m_{\text{F}}} = - {9 / 2}$ polarization spectrum. The correction of second-order Zeeman shift is calculated to be 0.7 × 10–16, and corresponding uncertainty is 0.2 × 10–17. Experimental results indicate that the frequency shift correction due to the blackbody radiation is the largest, while the uncertainty caused by the lattice AC Stark effect is the largest in the evaluated shifts. The systematic shift is 58.8 × 10–16, the total uncertainty is 2.3 × 10–16. In the next work, the magneto-optical trap cavity will be placed in a blackbody-radiation cavity to reduce the blackbody-radiation shift. The uncertainty of the collision shift will be reduced by increasing the beam waist of the lattice and reducing the potential well depth of the lattice, which will reduce the density of atoms. What is more, the light source for the optical lattice after spectral filtering will be measured by an optical frequency comb locked to the hydrogen clock signal to reduce the uncertainty of the lattice AC Stark frequency shift. The systematic uncertainty is expected to be on the order of 10–17. The evaluation of the systematic uncertainty for the transportable 87Sr optical lattice clock lays the foundation for the practical application.-
Keywords:
- transportable 87Sr optical lattice clock /
- systematic shift /
- uncertainty /
- time-interleaved self-comparison method
[1] Ludlow A D, Boyd M M, Ye J, Peik E, Schimidt P O 2015 Rev. Mod. Phys. 87 637
Google Scholar
[2] Rosenband T, Hume D B, Schmidt P O, Schmidt P O, Chou C W, Brusch A, Lorini L, Oskay W H, Drullinger R E, Fortier T M, Stalnaker J E, Diddams S A, Swann W C, Newbury N R, Itano W M, Wineland D J, Bergquist J C 2008 Science 319 1808
Google Scholar
[3] Diddams S A 2001 Science 293 825
Google Scholar
[4] Dube P, Madej A A, Zhou Z C, Bernard J E 2013 Phys. Rev. A 87 023806
Google Scholar
[5] Nicholson T L, Campbell S L, Hutson R B, Marti G E, Bloom B G, MacNally R L, Zhang W, Barrett M D, Safronova M S, Strouse G F, Tew W L, Ye J 2015 Nat. Commun. 6 6896
Google Scholar
[6] Campbell S L, Hutson R B, Marti G E, Goban A, Darkwah O N, MacNally R L, Souderhouse L, Robinson J M, Zhang W, Bloom B G, Ye J 2017 Science 358 90
Google Scholar
[7] Poli N, Schioppo M, Vogt S, Falke S, Sterr U, Lisdat C, Tino G M 2014 Appl. Phys. B 117 1107
Google Scholar
[8] Lin Y G, Wang Q, Li Y, Meng F, Lin B K, Zang E J, Sun Z, Fang F, Li T C, Fang Z J 2015 Chin. Phys. Lett. 32 090601
Google Scholar
[9] Ohmae N, Sakama S, Katori H 2019 Electr. Commun. JPN 102 43
Google Scholar
[10] Liu H, Zhang X, Jiang K L, Wang J Q, Zhou Q, Xiong Z X, He L X, Lü B L 2017 Chin. Phys. Lett. 34 020601
Google Scholar
[11] 周敏, 徐信业 2016 物理 45 431
Google Scholar
Zhou M, Xu X Y 2016 Physics 45 431
Google Scholar
[12] 管桦, 黄垚, 李承斌, 高克林 2018 67 164202
Google Scholar
Guan H, Huang Y, Li C B, Gao K L 2018 Acta Phys. Sin. 67 164202
Google Scholar
[13] Huang Y, Guan H, Zeng M, Tang L, Gao K 2019 Phys. Rev. A 99 011401
Google Scholar
[14] Brewer S M, Chen J S, Hankin A M, Clements E R, Chou C W, Wineland D J, Hume D B, Leibrandt D R 2019 Phys. Rev. Lett. 123 033201
Google Scholar
[15] Oelker E, Hutson R B, Kennedy C J, Sonderhouse L, Bothwell T, Goban A, Kedar D, Sanner C, Robinson J M, Marti G E, Matei D G, Legero T, Giunta M, Holzwarth R, Riehle F, Sterr U, Ye J 2019 Nat. Photon. 13 714
Google Scholar
[16] Paul S, Swanson T B, Hanssen J, Taylor J 2017 Metrologia 54 247
Google Scholar
[17] Derevianko A, Pospelov M 2014 Nat. Phys. 10 933
Google Scholar
[18] Kolkowitz S, Pikovski I, Langellier N, Lukin M D, Walsworth R L, Ye J 2016 Phys. Rev. D 94 124043
Google Scholar
[19] Chou C W, Hume D B, Rosenband T, Wineland D J 2010 Science 329 1630
Google Scholar
[20] Lopez O, Haboucha A, Chanteau B, Chardonnet Ch, Amy-Klein A, Santarelli G 2012 Opt. Express 20 23518
Google Scholar
[21] Bercy A, Lopez O, Pottie P E, Amy-Klein A 2016 Appl. Phys. B 122 189
Google Scholar
[22] Mcgrew W F, Zhang X, Fasano R J, Schäffer S A, Beloy K, Nicolodi D, Brown R C, Hinkley N, Milani G, Schioppo M, Yoon T H, Ludlow A D 2018 Nature 564 87
Google Scholar
[23] Grotti J, Koller S, Vogt S, Häfner S, Sterr U, Lisdat C, Denker H, Voigt C, Timmen L, Rolland A, Fred N B, Margolis H S, Zampaolo M, Thoumany P, Pizzocaro M, Rauf B, Bregolin F, Tampellini A, Barbieri P, Zucco M, Costanzo G A, Clivati C, Levi F, Calonico D 2018 Nat. Phys. 14 437
Google Scholar
[24] Shang H S, Zhang X G, Zhang S N, Pan D, Chen H J, Chen J B 2017 Opt. Express 25 30459
Google Scholar
[25] Zhang S, Zhang X, Cui J, Jiang Z J, Shang H S, Zhu C W, Chang P Y, Zhang L, Tu J H, Chen J B 2017 Rev. Sci. Instrum. 88 103106
Google Scholar
[26] Koller S B, Grotti J, Al-Masoudi A, Dörscher S, Häfner S, Sterr U, Lisdat C 2017 Phys. Rev. Lett. 118 073601
Google Scholar
[27] Cao J, Zhang P, Shang J, Cui K, Yuan J, Chao S, Wang S, Shu H, Huang X 2017 Appl. Phys. B 123 112
Google Scholar
[28] Takamoto M, Ushijima I, Ohmae N, Yahagi T, Kokado K, Shinkai H, Katori H 2020 Nat. Photon. 14 411
Google Scholar
[29] Wang Y B, Yin M J, Ren J, Xu Q F, Lu B Q, Han J X, Guo Y, Chang H 2018 Chin. Phys. B 27 023701
Google Scholar
[30] Kong D H, Wang Z H, Guo F, Zhang Q, Lu X T, Wang Y B, Chang H 2020 Chin. Phys. B 29 070602
Google Scholar
[31] Middlemann T, Falkes S, Listat C, Sterr U 2012 Phys. Rev. Lett. 109 263004
Google Scholar
[32] 李婷, 卢晓同, 张强, 孔德欢, 王叶兵, 常宏 2019 68 093701
Google Scholar
Li T, Lu X T, Zhang Q, Kong D H, Wang Y B, Chang H 2019 Acta Phys. Sin. 68 093701
Google Scholar
[33] Origlia S, Pramod M S, Schiller S, Singh Y, Bongs K, Schwarz R, Al-Masoudi A, Dörscher S, Häfner S, Sterr U, Lisdat C 2018 Phys. Rev. A 98 053443
Google Scholar
[34] Bureau International des Poids et Mesures (BIPM) Consultative Committee for Time and Frequency (CCTF) Report of the 21st Meeting (June 8-9, 2017) to the International Committee for Weights and Measures https://www.bipm.org/utils/common/pdf/CC/CCTF/CCTF21.pdf
[35] Lemke N D, von Stecher J, Sherman J A, Rey A M, Oates C W, Ludlow A D 2011 Phys. Rev. Lett. 107 103902
Google Scholar
[36] 卢晓同, 李婷, 孔德欢, 王叶兵, 常宏 2019 68 233401
Google Scholar
Lu X T, Li T, Kong D H, Wang Y B, Chang H 2019 Acta Phys. Sin. 68 233401
Google Scholar
[37] Katori H, Takamoto M, Pal’chikov V G, Ovsiannikov V D 2003 Phys. Rev. Lett. 91 173005
Google Scholar
[38] 林弋戈, 方占军 2018 67 160604
Google Scholar
Lin Y G, Fang Z J 2018 Acta Phys. Sin. 67 160604
Google Scholar
[39] Westergaard P G, Lodewyck J, Lorini L, Lecallier A, Burt E A, Zawada M, Millo J, Lemonde P 2011 Phys. Rev. Lett. 106 210801
Google Scholar
[40] Bloom B J, Nicholson T L, William J R, Campbell S L, Bishof M, Zhang X, Zhang W, Bromley S L 2014 Nature 506 71
Google Scholar
[41] Bailard X, Fouché M, Targat R L, Westergaard P G, Lecallier A, Coq Y L, Rovera G D, Bize S, Lemonde P 2007 Opt. Lett. 32 1812
Google Scholar
[42] Bothwell T, Kedar D, Oelker E, Robinson J M, Bromley S L, L Tew W L, Ye J, Kennedy C J 2019 Metrologia 56 065004
Google Scholar
-
图 1 可搬运87Sr光晶格钟的物理系统(PMT, 光电倍增管; EMCCD, 电子倍增电荷耦合相机)
Fig. 1. Physical system of the transportable strontium optical lattice clock (PMT, photo-multiplier tube; EMCCD, electron-multiplying charge coupled device)
图 2 磁光阱腔体上测量点的分布示意图
Fig. 2. Distribution of the temperature points on the magneto-optical trap cavity.
图 3 磁光阱腔体各测温点的温度波动
Fig. 3. Temperature fluctuations at the temperature points on the magneto-optical trap cavity
图 5 单次高低原子密度自比对的阿伦偏差
Fig. 5. The Allan deviation obtained by the time-interleaved self-comparison method between high and low atomic density
表 1 可搬运87Sr光晶格钟的系统不确定度评估
Table 1. Uncertainty budget for the transportable strontium optical lattice clock.
频移项 相对频移修正/10–16 相对不确定度/10–17 黑体辐射 50.4 5.1 碰撞 4.7 5.6 晶格光交流斯塔克 3.0 22.1 二阶塞曼 0.7 0.2 钟激光交流斯塔克 0 1.0 线牵引 0 1.0 总和 58.8 23.4 
下载: 导出CSV
-
[1] Ludlow A D, Boyd M M, Ye J, Peik E, Schimidt P O 2015 Rev. Mod. Phys. 87 637
Google Scholar
[2] Rosenband T, Hume D B, Schmidt P O, Schmidt P O, Chou C W, Brusch A, Lorini L, Oskay W H, Drullinger R E, Fortier T M, Stalnaker J E, Diddams S A, Swann W C, Newbury N R, Itano W M, Wineland D J, Bergquist J C 2008 Science 319 1808
Google Scholar
[3] Diddams S A 2001 Science 293 825
Google Scholar
[4] Dube P, Madej A A, Zhou Z C, Bernard J E 2013 Phys. Rev. A 87 023806
Google Scholar
[5] Nicholson T L, Campbell S L, Hutson R B, Marti G E, Bloom B G, MacNally R L, Zhang W, Barrett M D, Safronova M S, Strouse G F, Tew W L, Ye J 2015 Nat. Commun. 6 6896
Google Scholar
[6] Campbell S L, Hutson R B, Marti G E, Goban A, Darkwah O N, MacNally R L, Souderhouse L, Robinson J M, Zhang W, Bloom B G, Ye J 2017 Science 358 90
Google Scholar
[7] Poli N, Schioppo M, Vogt S, Falke S, Sterr U, Lisdat C, Tino G M 2014 Appl. Phys. B 117 1107
Google Scholar
[8] Lin Y G, Wang Q, Li Y, Meng F, Lin B K, Zang E J, Sun Z, Fang F, Li T C, Fang Z J 2015 Chin. Phys. Lett. 32 090601
Google Scholar
[9] Ohmae N, Sakama S, Katori H 2019 Electr. Commun. JPN 102 43
Google Scholar
[10] Liu H, Zhang X, Jiang K L, Wang J Q, Zhou Q, Xiong Z X, He L X, Lü B L 2017 Chin. Phys. Lett. 34 020601
Google Scholar
[11] 周敏, 徐信业 2016 物理 45 431
Google Scholar
Zhou M, Xu X Y 2016 Physics 45 431
Google Scholar
[12] 管桦, 黄垚, 李承斌, 高克林 2018 67 164202
Google Scholar
Guan H, Huang Y, Li C B, Gao K L 2018 Acta Phys. Sin. 67 164202
Google Scholar
[13] Huang Y, Guan H, Zeng M, Tang L, Gao K 2019 Phys. Rev. A 99 011401
Google Scholar
[14] Brewer S M, Chen J S, Hankin A M, Clements E R, Chou C W, Wineland D J, Hume D B, Leibrandt D R 2019 Phys. Rev. Lett. 123 033201
Google Scholar
[15] Oelker E, Hutson R B, Kennedy C J, Sonderhouse L, Bothwell T, Goban A, Kedar D, Sanner C, Robinson J M, Marti G E, Matei D G, Legero T, Giunta M, Holzwarth R, Riehle F, Sterr U, Ye J 2019 Nat. Photon. 13 714
Google Scholar
[16] Paul S, Swanson T B, Hanssen J, Taylor J 2017 Metrologia 54 247
Google Scholar
[17] Derevianko A, Pospelov M 2014 Nat. Phys. 10 933
Google Scholar
[18] Kolkowitz S, Pikovski I, Langellier N, Lukin M D, Walsworth R L, Ye J 2016 Phys. Rev. D 94 124043
Google Scholar
[19] Chou C W, Hume D B, Rosenband T, Wineland D J 2010 Science 329 1630
Google Scholar
[20] Lopez O, Haboucha A, Chanteau B, Chardonnet Ch, Amy-Klein A, Santarelli G 2012 Opt. Express 20 23518
Google Scholar
[21] Bercy A, Lopez O, Pottie P E, Amy-Klein A 2016 Appl. Phys. B 122 189
Google Scholar
[22] Mcgrew W F, Zhang X, Fasano R J, Schäffer S A, Beloy K, Nicolodi D, Brown R C, Hinkley N, Milani G, Schioppo M, Yoon T H, Ludlow A D 2018 Nature 564 87
Google Scholar
[23] Grotti J, Koller S, Vogt S, Häfner S, Sterr U, Lisdat C, Denker H, Voigt C, Timmen L, Rolland A, Fred N B, Margolis H S, Zampaolo M, Thoumany P, Pizzocaro M, Rauf B, Bregolin F, Tampellini A, Barbieri P, Zucco M, Costanzo G A, Clivati C, Levi F, Calonico D 2018 Nat. Phys. 14 437
Google Scholar
[24] Shang H S, Zhang X G, Zhang S N, Pan D, Chen H J, Chen J B 2017 Opt. Express 25 30459
Google Scholar
[25] Zhang S, Zhang X, Cui J, Jiang Z J, Shang H S, Zhu C W, Chang P Y, Zhang L, Tu J H, Chen J B 2017 Rev. Sci. Instrum. 88 103106
Google Scholar
[26] Koller S B, Grotti J, Al-Masoudi A, Dörscher S, Häfner S, Sterr U, Lisdat C 2017 Phys. Rev. Lett. 118 073601
Google Scholar
[27] Cao J, Zhang P, Shang J, Cui K, Yuan J, Chao S, Wang S, Shu H, Huang X 2017 Appl. Phys. B 123 112
Google Scholar
[28] Takamoto M, Ushijima I, Ohmae N, Yahagi T, Kokado K, Shinkai H, Katori H 2020 Nat. Photon. 14 411
Google Scholar
[29] Wang Y B, Yin M J, Ren J, Xu Q F, Lu B Q, Han J X, Guo Y, Chang H 2018 Chin. Phys. B 27 023701
Google Scholar
[30] Kong D H, Wang Z H, Guo F, Zhang Q, Lu X T, Wang Y B, Chang H 2020 Chin. Phys. B 29 070602
Google Scholar
[31] Middlemann T, Falkes S, Listat C, Sterr U 2012 Phys. Rev. Lett. 109 263004
Google Scholar
[32] 李婷, 卢晓同, 张强, 孔德欢, 王叶兵, 常宏 2019 68 093701
Google Scholar
Li T, Lu X T, Zhang Q, Kong D H, Wang Y B, Chang H 2019 Acta Phys. Sin. 68 093701
Google Scholar
[33] Origlia S, Pramod M S, Schiller S, Singh Y, Bongs K, Schwarz R, Al-Masoudi A, Dörscher S, Häfner S, Sterr U, Lisdat C 2018 Phys. Rev. A 98 053443
Google Scholar
[34] Bureau International des Poids et Mesures (BIPM) Consultative Committee for Time and Frequency (CCTF) Report of the 21st Meeting (June 8-9, 2017) to the International Committee for Weights and Measures https://www.bipm.org/utils/common/pdf/CC/CCTF/CCTF21.pdf
[35] Lemke N D, von Stecher J, Sherman J A, Rey A M, Oates C W, Ludlow A D 2011 Phys. Rev. Lett. 107 103902
Google Scholar
[36] 卢晓同, 李婷, 孔德欢, 王叶兵, 常宏 2019 68 233401
Google Scholar
Lu X T, Li T, Kong D H, Wang Y B, Chang H 2019 Acta Phys. Sin. 68 233401
Google Scholar
[37] Katori H, Takamoto M, Pal’chikov V G, Ovsiannikov V D 2003 Phys. Rev. Lett. 91 173005
Google Scholar
[38] 林弋戈, 方占军 2018 67 160604
Google Scholar
Lin Y G, Fang Z J 2018 Acta Phys. Sin. 67 160604
Google Scholar
[39] Westergaard P G, Lodewyck J, Lorini L, Lecallier A, Burt E A, Zawada M, Millo J, Lemonde P 2011 Phys. Rev. Lett. 106 210801
Google Scholar
[40] Bloom B J, Nicholson T L, William J R, Campbell S L, Bishof M, Zhang X, Zhang W, Bromley S L 2014 Nature 506 71
Google Scholar
[41] Bailard X, Fouché M, Targat R L, Westergaard P G, Lecallier A, Coq Y L, Rovera G D, Bize S, Lemonde P 2007 Opt. Lett. 32 1812
Google Scholar
[42] Bothwell T, Kedar D, Oelker E, Robinson J M, Bromley S L, L Tew W L, Ye J, Kennedy C J 2019 Metrologia 56 065004
Google Scholar
下载:
计量
- 文章访问数: 6589
- PDF下载量: 189
- 被引次数: 0
