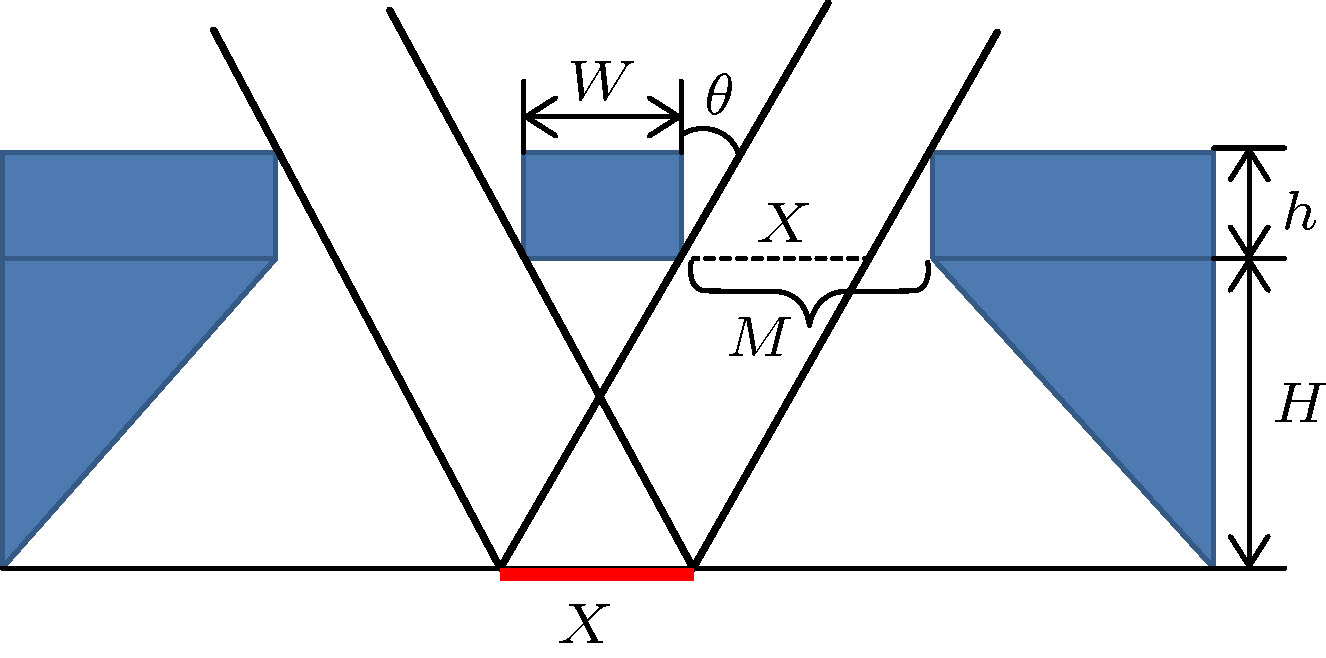
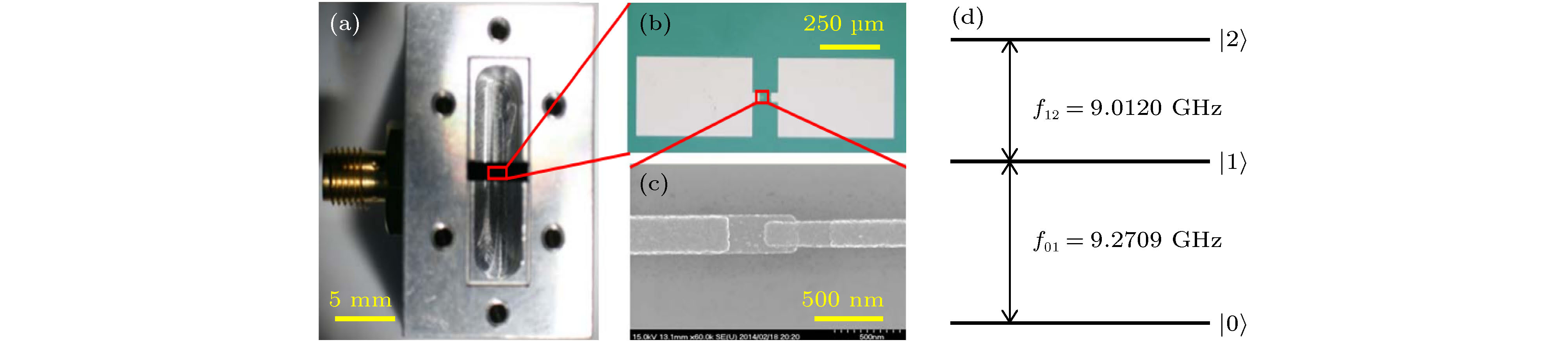
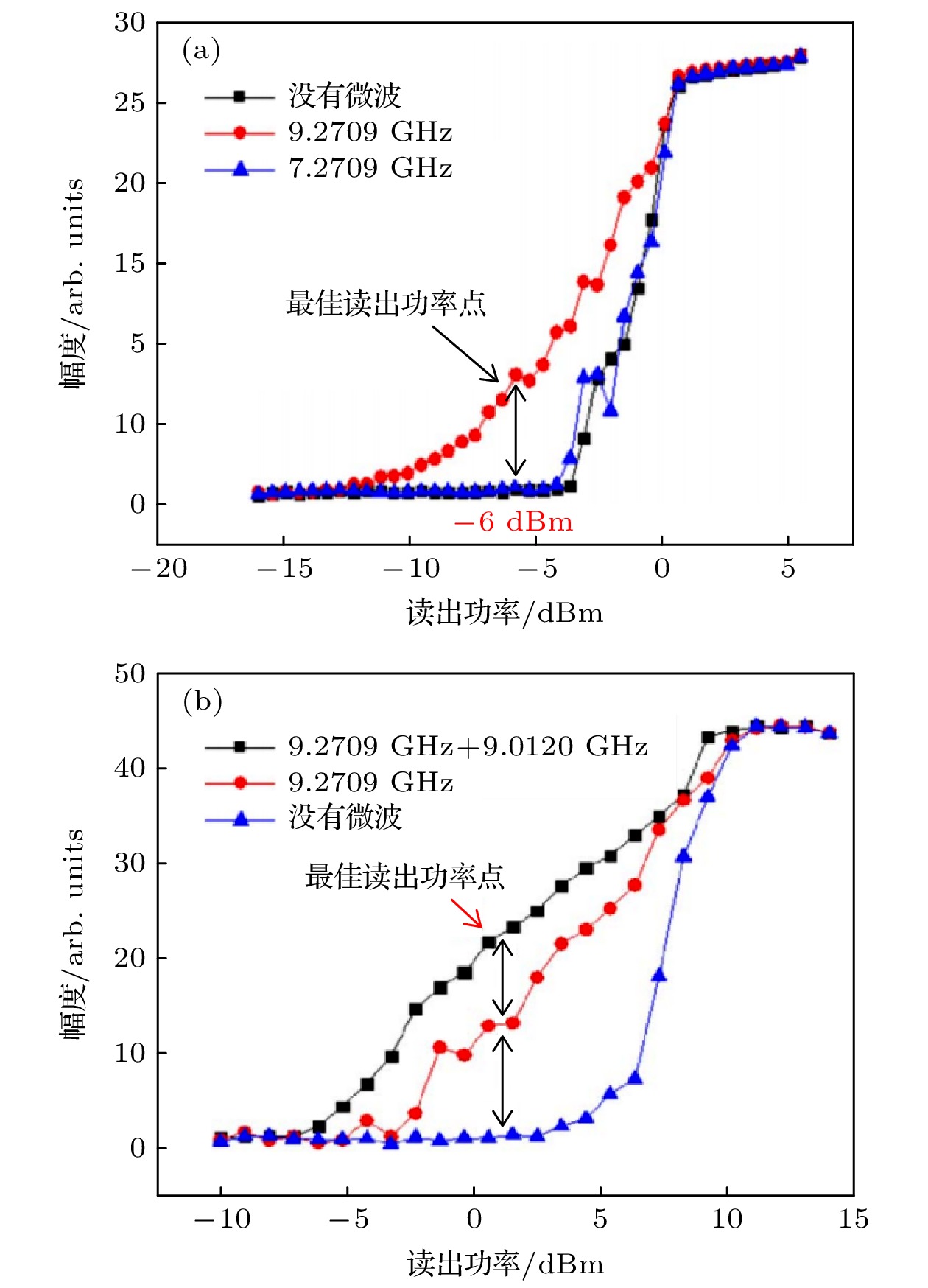
-
基于约瑟夫森结的超导量子比特是一个宏观的人工原子, 通过微纳米加工的方法, 可以改变人工原子的基本参数. 三维Transmon量子比特是目前已知退相干时间较长的一种量子比特, 该量子比特通过电容的方式与三维超导谐振腔进行耦合, 是一个人造的原子与腔场耦合系统, 可对原子物理、量子力学、量子光学、腔量子电动力学的各种效应进行实验验证. 本文制备并实现了3D Transmon量子比特, 通过Jaynes-Cummings方法寻找到了区分基态、第一激发态和第二激发态的最佳读出功率, 对共振条件下和非共振条件下的奥特-汤恩斯分裂效应进行了测试表征, 得到的测试结果与理论结果相符.
-
关键词:
- 三维 Transmon /
- Jaynes-Cummings读出法 /
- 奥特-汤恩斯分裂效应
The superconducting quantum bit(qubit) based on Josephson junction is a macroscopic artificial atom. The basic parameters of the artificial atom can be changed by micro and nano machining. The three-dimensional (3D) Transmon qubit is a kind of qubit with the longer decoherence time. It is coupled with a 3D superconducting cavity by means of capacitance. It is a man-made coupling system between atom and cavity field, which can verify the effects of atomic physics, quantum mechanics, quantum optics and cavity quantum electrodynamics. In this paper, transmon qubits are prepared by the double angle evaporation method, and coupled with aluminum based 3D superconducting resonator to form 3D transmon qubits. The basic parameters of 3D transmon are characterized at an ultra-low temperature of 10 mK. The 3D transmon parameters are EC = 348.74 MHz and EJ = 11.556 GHz. The coupling coefficient g2/Δ between qubit and the 3D cavity is 43 MHz, which is located in the dispersive regime. The first transition frequency of qubit is f01= 9.2709 GHz, and the second transition frequency is f12 = 9.0100 GHz. The 3D resonator is made of the material 6061T6 aluminum, the loaded quality factor is 4.8 × 105, and the bare frequency of the resonator is 8.108 GHz. The Jaynes-Cummings readout method is used to find the optimal readout power to distinguish among the qubit in the ground state $ \left| {\rm{0}} \right\rangle $ , qubit in the superposition state of$ \left| {\rm{0}} \right\rangle $ and$ \left| {\rm{1}} \right\rangle $ , and qubit in the superposition state of$ \left| {\rm{0}} \right\rangle $ ,$ \left| {\rm{1}} \right\rangle $ and$ \left| {\rm{2}} \right\rangle $ . Then, the Aulter-Townes splitting (ATS) experiment can be fulfilled in this system. Unlike the method given by Novikov et al. [Novikov S, Robinson J E, Keane Z K, et al. 2013 Phys. Rev. B 88 060503], our method only needs to apply continuous microwave excitation signal to the qubit, and does not need to carry out precise timing test on the qubit, thus reducing the test complexity of observing ATS effect. The ATS effect in resonance and non-resonance regime are observed. In the resonance ATS experiment, in order to obtain the peak value and frequency of resonance peak, Lorentz curve can be used for fitting peaks, and the ATS curve of double peak can be fitted by adding two Lorentz curves together. In the non-resonance ATS experiment, the detection signal is scanned, and the ATS double peak will shift with the different coupling signal detuning, forming an anti-crossing structure. The two curves formed by crossing free structure give two eigenvalues of Hamiltonian. By solving the equation, the experimental results can also be found to be consistent with the theoretical results.[1] You J Q, Nori F 2005 Phys. Today 58 42
[2] You J Q, Nori F 2011 Nature 474 589
Google Scholar
[3] Krantz P, Kjaergaard M, Yan F, et al. 2019 Appl. Phys. Rev. 6 021318
Google Scholar
[4] Rigetti C, Gambetta J M, Poletto S, et al. 2012 Phys. Rev. 86 100506
Google Scholar
[5] Neill C, Roushan P, Kechedzhi K, et al. 2018 Science 360 195
Google Scholar
[6] Wallraff A, Schuster D I, Blais A, et al. 2004 Nature 431 162
Google Scholar
[7] Blais A, Huang R S, Wallraff A, et al. 2004 Phys. Rev. A 69 6
[8] Murch K W, Weber S J, Macklin C, et al. 2013 Nature 502 211
Google Scholar
[9] Yoshihara F, Fuse T, Ashhab S, et al. 2017 Nat. Phys. 13 44
Google Scholar
[10] 赵虎, 李铁夫, 刘建设等 2012 61 154214
Google Scholar
Zhao H, Li T F, Liu J S, et al. 2012 Acta Phys. Sin. 61 154214
Google Scholar
[11] Wilson C M, Johansson G, Pourkabirian A, et al. 2011 Nature 479 376
Google Scholar
[12] Yoshihara F, Fuse T, Ao Z, et al. 2018 Phys. Rev. Lett. 120 183601
Google Scholar
[13] Lin Y H, Nguyen L B, Grabon N, et al. 2018 Phys. Rev. Lett. 120 150503
Google Scholar
[14] Wen P Y, Kockum A F, Ian H, et al. 2018 Phys. Rev. Lett. 120 063603
Google Scholar
[15] Magazzù L, Forn-Díaz P, Belyansky R, et al. 2018 Nat. Commun. 9 1403
Google Scholar
[16] Wang W, Wu Y, Ma Y, et al. 2019 Nat. Commun. 10 4382
Google Scholar
[17] Hu L, Ma Y, Cai W, et al. 2019 Nat. Phys. 15 503
Google Scholar
[18] Xu Y, Ma Y, Cai W, et al. 2020 Phys. Rev. Lett. 124 120501
Google Scholar
[19] Baur M, Filipp S, Bianchetti R, et al. 2009 Phys. Rev. Lett. 102 243602
Google Scholar
[20] Sillanpaa M A, Li J, Cicak K, et al. 2009 Phys. Rev. Lett. 103 193601
Google Scholar
[21] Abdumalikov A A, Astafiev O, Zagoskin A M, et al. 2010 Phys. Rev. Lett. 104 193601
Google Scholar
[22] Novikov S, Robinson J E, Keane Z K, et al. 2013 Phys. Rev. B 88 060503
Google Scholar
[23] 赵虎, 李铁夫, 刘其春等 2014 63 220305
Google Scholar
Zhao H, Li T F, Liu Q C, et al. 2014 Acta Phys. Sin. 63 220305
Google Scholar
[24] Zhao H, Li T F, Liu Q C, et al. 2014 Chin. Phys. Lett. 31 102101
Google Scholar
[25] Paik H, Schuster D I, Bishop L S, et al. 2011 Phys. Rev. Lett. 107 240501
Google Scholar
[26] Reed M D, DiCarlo L, Johnson B R, et al. 2010 Phys. Rev. Lett. 105 173601
Google Scholar
[27] Aulter S H, Townes C H 1955 Phys. Rev. 100 703
Google Scholar
-
图 6 (a)区分两个量子态的读出功率的优化; (b)区分三个能态的最佳读出功率优化
Fig. 6. (a) Optimization of readout power for distinguishing two quantum states; (b) optimization for distinguishing three energy states.
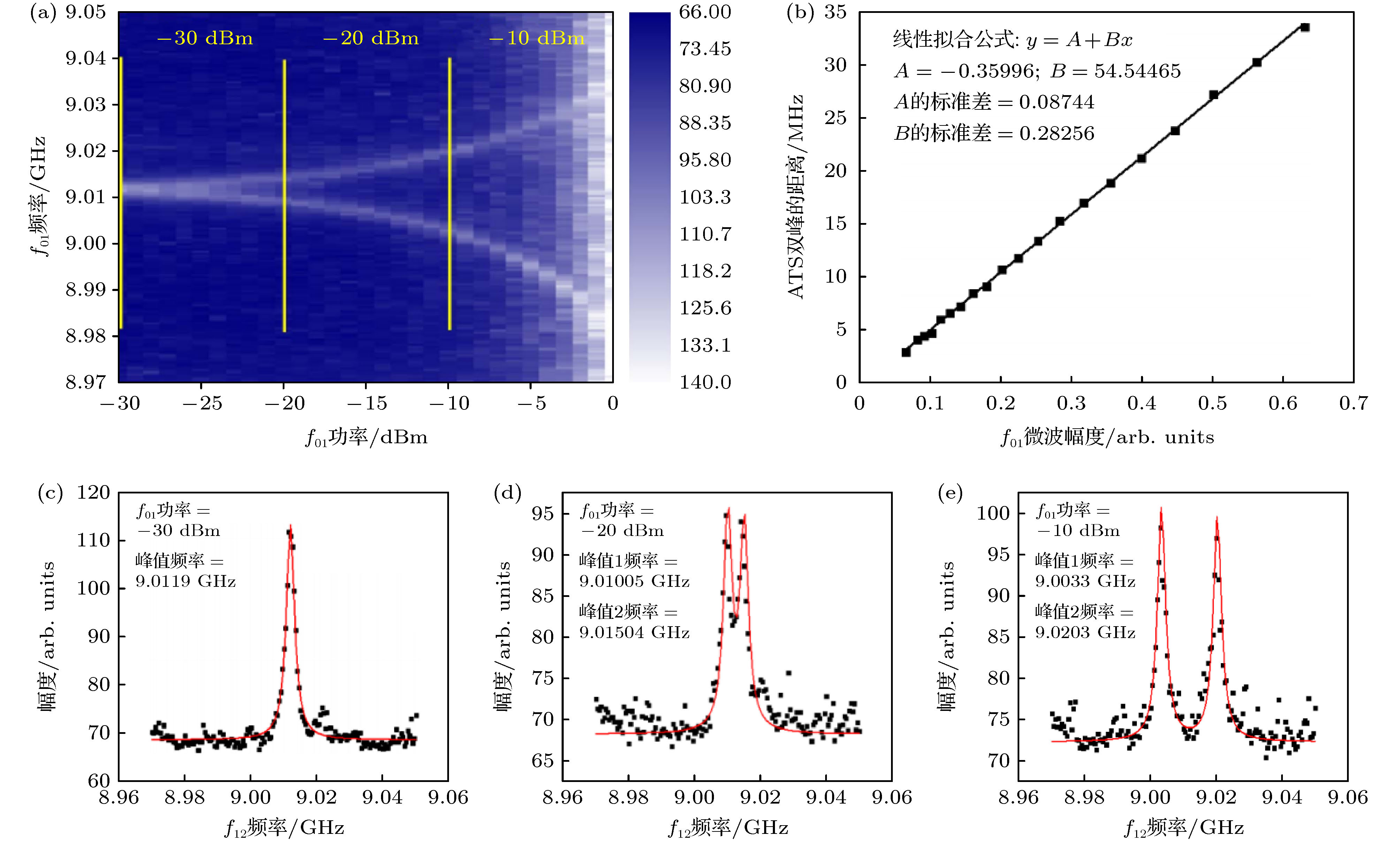
图 8 3D Transmon量子比特的奥特-汤恩斯分裂效应 (a)奥特-汤恩斯分裂效应测试强度图; (b)奥特-汤恩斯分裂效应双峰间距与微波幅度的关系; (c)耦合信号功率为–30 dBm时的奥特-汤恩斯分裂效应曲线; (d)耦合信号功率为–20 dBm时的奥特-汤恩斯分裂效应曲线; (e)耦合信号功率为–10 dBm时的奥特-汤恩斯分裂效应曲线.
Fig. 8. ATS effect of 3D transmon qubit: (a) ATS test intensity diagram; (b) relationship between ATS peak spacing and microwave amplitude; (c) ATS curve at –30 dBm coupling signal power; (d) ATS curve at –20 dBm coupling signal power; (e) ATS curve at –10 dBm coupling signal power.
图 9 非共振条件下的奥特-汤恩斯分裂效应免交叉测试强度图 (a)
${\omega _{\rm{C}}}$ 的功率为–20 dBm; (b)${\omega _{\rm{C}}}$ 的功率为–15 dBm; (c)${\omega _{\rm{C}}}$ 的功率为–10 dBmFig. 9. Anticrossing intensity test diagram of ATS under non resonance condition: (a) Power of
${\omega _{\rm{C}}}$ is –20 dBm; (b) power of${\omega _{\rm{C}}}$ is –15 dBm; (c) power of${\omega _{\rm{C}}}$ is –10 dBm. -
[1] You J Q, Nori F 2005 Phys. Today 58 42
[2] You J Q, Nori F 2011 Nature 474 589
Google Scholar
[3] Krantz P, Kjaergaard M, Yan F, et al. 2019 Appl. Phys. Rev. 6 021318
Google Scholar
[4] Rigetti C, Gambetta J M, Poletto S, et al. 2012 Phys. Rev. 86 100506
Google Scholar
[5] Neill C, Roushan P, Kechedzhi K, et al. 2018 Science 360 195
Google Scholar
[6] Wallraff A, Schuster D I, Blais A, et al. 2004 Nature 431 162
Google Scholar
[7] Blais A, Huang R S, Wallraff A, et al. 2004 Phys. Rev. A 69 6
[8] Murch K W, Weber S J, Macklin C, et al. 2013 Nature 502 211
Google Scholar
[9] Yoshihara F, Fuse T, Ashhab S, et al. 2017 Nat. Phys. 13 44
Google Scholar
[10] 赵虎, 李铁夫, 刘建设等 2012 61 154214
Google Scholar
Zhao H, Li T F, Liu J S, et al. 2012 Acta Phys. Sin. 61 154214
Google Scholar
[11] Wilson C M, Johansson G, Pourkabirian A, et al. 2011 Nature 479 376
Google Scholar
[12] Yoshihara F, Fuse T, Ao Z, et al. 2018 Phys. Rev. Lett. 120 183601
Google Scholar
[13] Lin Y H, Nguyen L B, Grabon N, et al. 2018 Phys. Rev. Lett. 120 150503
Google Scholar
[14] Wen P Y, Kockum A F, Ian H, et al. 2018 Phys. Rev. Lett. 120 063603
Google Scholar
[15] Magazzù L, Forn-Díaz P, Belyansky R, et al. 2018 Nat. Commun. 9 1403
Google Scholar
[16] Wang W, Wu Y, Ma Y, et al. 2019 Nat. Commun. 10 4382
Google Scholar
[17] Hu L, Ma Y, Cai W, et al. 2019 Nat. Phys. 15 503
Google Scholar
[18] Xu Y, Ma Y, Cai W, et al. 2020 Phys. Rev. Lett. 124 120501
Google Scholar
[19] Baur M, Filipp S, Bianchetti R, et al. 2009 Phys. Rev. Lett. 102 243602
Google Scholar
[20] Sillanpaa M A, Li J, Cicak K, et al. 2009 Phys. Rev. Lett. 103 193601
Google Scholar
[21] Abdumalikov A A, Astafiev O, Zagoskin A M, et al. 2010 Phys. Rev. Lett. 104 193601
Google Scholar
[22] Novikov S, Robinson J E, Keane Z K, et al. 2013 Phys. Rev. B 88 060503
Google Scholar
[23] 赵虎, 李铁夫, 刘其春等 2014 63 220305
Google Scholar
Zhao H, Li T F, Liu Q C, et al. 2014 Acta Phys. Sin. 63 220305
Google Scholar
[24] Zhao H, Li T F, Liu Q C, et al. 2014 Chin. Phys. Lett. 31 102101
Google Scholar
[25] Paik H, Schuster D I, Bishop L S, et al. 2011 Phys. Rev. Lett. 107 240501
Google Scholar
[26] Reed M D, DiCarlo L, Johnson B R, et al. 2010 Phys. Rev. Lett. 105 173601
Google Scholar
[27] Aulter S H, Townes C H 1955 Phys. Rev. 100 703
Google Scholar
下载:
计量
- 文章访问数: 5229
- PDF下载量: 77
- 被引次数: 0
