-
热电材料能够将废热能直接转换成电能, 近年来受到了科技工作者们的广泛关注. 本文采用非平衡格林函数方法系统地研究了晶界对石墨烯纳米带热电性能的调控作用. 研究结果表明: 晶界能有效地提高石墨烯纳米带的塞贝克系数, 同时可以极大地抑制其热导(包含电子和声子部分). 基于这两个积极的效应, 多晶石墨烯纳米带的热电转换性能得到了显著的增强. 在室温下, 多晶石墨烯纳米带的热电品质因子约为0.3, 较完美石墨烯纳米带(约为0.05)提升了6倍左右. 并且发现晶界的数量和系统的长度还能进一步提升多晶石墨烯纳米带的热电性能, 但系统的宽度对其影响有限. 这些结果表明, 多晶结构可以显著提升石墨烯纳米带的热电转换效率. 这将为设计和制备基于石墨烯纳米带的热电器件提供新的途径.Thermoelectric materials, which can convert heat energy into electric energy and also from electric energy into heat energy, have aroused widespread interest of both theoretical and technological researches recently. Graphene is a typical two-dimensional carbon nanomaterial and regarded as a competitive candidate for the next-generation micro/nano-devices. Unfortunately, graphene is an inefficient thermoelectric material due to the extremely high thermal conductivity. To overcome this drawback, exploring an effective way to improve the thermoelectric performance is of critical importance. In this paper, using the nonequilibrium Green’s function approach, we systematically investigate the effects of grain boundary on the thermoelectric properties of graphene nanoribbons. The results show that owing to the existence of grain boundary, the phonons and electrons encounter great scatterings when they transmit through the polycrystalline graphene nanoribbons. These scatterings cause the phononic and electronic transmission coefficient to decrease dramatically, and thus leading the thermal conductance (including both electron and phonon parts) of graphene nanoribbons to be evidently suppressed. Meanwhile, such scatterings induce more intense transmission peaks and pits in the electronic transmission spectrum of polycrystalline graphene nanoribbons. Generally, the Seebeck coefficient depends on the derivative of electronic transmission coefficient. The larger the logarithmic derivative of transmission, the higher the Seebeck coefficient can be obtained. Therefore Seebeck coefficient is improved obviously in the polycrystalline graphene nanoribbons. Based on such two positive effects, the thermoelectric performance of polycrystalline graphene nanoribbons is significantly enhanced. At room temperature, the thermoelectric figure of merit of polycrystalline graphene nanoribbons can approach to 0.3, which is about 6 times larger than that of pristine graphene nanoribbon (figure of merit is about 0.05). It is also found that the quantity of grain boundaries and length of system can further improve the thermoelectric properties of the polycrystalline graphene nanoribbons, while the width of system has a limited influence on it. This is because the quantity of grain boundaries and length of polycrystalline graphene nanoribbons can give rise to more intense phonon and electron scatterings and further decreasing of thermal conductance and enhancement of Seebeck coefficient. The results presented in this paper demonstrate that polycrystalline structure is indeed an effective way to improve the thermoelectric conversion efficiency of graphene nanoribbons, and provide a theoretical guideline for designing and preparing thermoelectric devices based on graphene nanoribbons.
[1] Tritt T M 2011 Annu. Rev. Mater. Res. 41 433
Google Scholar
[2] Dresselhaus M S, Chen G, Tang M Y, Yang R G, Lee H, Wang D Z, Ren Z F, Fleurial J P, Gogna P 2007 Adv. Mater. 19 1043
Google Scholar
[3] Snyder G J, Toberer E S 2008 Nat. Mater. 7 105
Google Scholar
[4] Zhu L, Li R, Yao K 2017 Phys. Chem. Chem. Phys. 19 4085
Google Scholar
[5] Zhu L, Li B, Yao K 2018 Nanotechnology 29 325206
Google Scholar
[6] Zhu L, Zou F, Gao G Y, Yao K 2017 Sci. Rep. 7 497
Google Scholar
[7] Geim A K, Novoselov K S 2007 Nat. Mater. 6 183
Google Scholar
[8] Geim A K 2009 Science 324 1530
Google Scholar
[9] Barone V, Hod O, Scuseria G E 2006 Nano Lett. 6 2748
Google Scholar
[10] Lee C, Wei X, Kysar J W, Hone J 2008 Science 321 385
Google Scholar
[11] Nan H Y, Ni Z H, Wang J, Zafar Z, Shi Z X, Wang Y Y 2013 J. Raman Spectrosc. 44 1018
Google Scholar
[12] Zhang H J, Zheng G G, Chen Y Y, Zou X J, Xu L H 2018 Chin. Phys. Lett. 35 038102
Google Scholar
[13] Bolotin K I, Sikes K J, Jiang Z, Klima M, Fudenberg G, Hone J, Kim P, Stormer H L 2008 Solid State Commun. 146 351
Google Scholar
[14] Dragoman D, Dragoman M 2007 Appl. Phys. Lett. 91 203116
Google Scholar
[15] Zhang H S, Guo Z X, Gong X G, Cao J X 2012 J. Appl. Phys. 112 123508
Google Scholar
[16] Nika D L, Pokatilov E P, Askerov A S, Balandin A A 2009 Phys. Rev. B 79 155413
Google Scholar
[17] Karamitaheri H, Pourfath M, Faez R, Kosina H 2011 J. Appl. Phys. 110 054506
Google Scholar
[18] Haskins J, Kınacı A, Sevik C, Sevinçli H, Cuniberti G, Çagǧın T 2011 ACS Nano 5 3779
Google Scholar
[19] 张嵛, 刘连庆, 焦念东, 席宁, 王越超, 董再励 2012 61 137101
Google Scholar
Zhang Y, Liu L Q, Jiao N D, Xi N, Wang Y C, Dong Z L 2012 Acta Phys. Sin. 61 137101
Google Scholar
[20] Tran V T, Saint Martin J, Dollfus P, Volz S 2017 Sci. Rep. 7 2313
Google Scholar
[21] 林琦, 陈余行, 吴建宝, 孔宗敏 2011 60 097103
Lin Q, Chen Y H, Wu J B, Kong Z M 2011 Acta Phys. Sin. 60 097103
[22] Huang P Y, Ruiz Vargas C S, van der Zande Arend M, Whitney W S, Levendorf M P, Kevek J W, Shivank G, Alden J S, Hustedt C J, Zhu Y, Park J W, McEuen P L, Muller D A 2011 Nature 469 389
Google Scholar
[23] Li X S, Cai W W, An J, Kim S, Nah J, Yang D X, Piner R, Velamakanni A, Jung I, Tutuc E, Banerjee S K, Colombo L, Ruoff R S 2009 Science 324 1312
Google Scholar
[24] Yazyev O V, Louie S G 2010 Nat. Mater. 9 806
Google Scholar
[25] Lee G H, Cooper R C, An S J, Lee S, Zande A, Petrone N, Hammerberg A G, Lee C, Crawford B, Oliver W, Kysar J W, Hone J 2013 Science 340 1073
Google Scholar
[26] Rassin G, Shenoy V B, Ruoff R S 2010 Science 330 946
Google Scholar
[27] Bagri A, Kim S P, Ruoff R S, Shenoy V B 2011 Nano Lett. 11 3917
Google Scholar
[28] Wang J S, Wang J, Lu J T 2008 Eur. Phys. J. B 62 381
Google Scholar
[29] Yamamoto T, Watanabe K 2006 Phys. Rev. Lett. 96 255503
Google Scholar
[30] Xiao H P, Cao W, Ouyang T, Xu X Y, Ding Y C, Zhong J X 2018 Appl. Phys. Lett. 112 233107
Google Scholar
[31] Ghosh S, Calizo I, Teweldebrhan D, Pokatilov E P, Nika D L, Balandin A A, Bao W, Miao F, Lau C N 2008 Appl. Phys. Lett. 92 151911
Google Scholar
[32] Li T C, Lu S P 2008 Phys. Rev. B 77 085408
Google Scholar
[33] Pereira V M, Neto A H C, Peres N M R 2009 Phys. Rev. B 80 045401
[34] Ouyang T, Hu M 2014 Nanotechnology 25 245401
Google Scholar
-
图 1 多晶石墨烯纳米带原子结构模型示意图
Fig. 1. Schematic diagram of polycrystalline graphene nanoribbons.
图 2 完美石墨烯纳米带和多晶石墨烯纳米带的(a)声子热导和(b)声子透射系数(其中L = 196.48 Å, W = 17.04Å); (c)−(e)多晶石墨烯纳米带(N = 5)在3个典型频率下(频率分别是160.68, 585.04, 951.72 Hz)的声子局域态密度
Fig. 2. Comparison of (a) the phonon conductance, (b) the phonon transmission for perfect graphene nanoribbons and polycrystalline graphene nanoribbons (where L = 196.48 Å, W = 17.04 Å); (c)−(e) the local density of states in polycrystalline graphene (N = 5) at three typical frequency (160.68, 585.04 and 951.72 Hz).
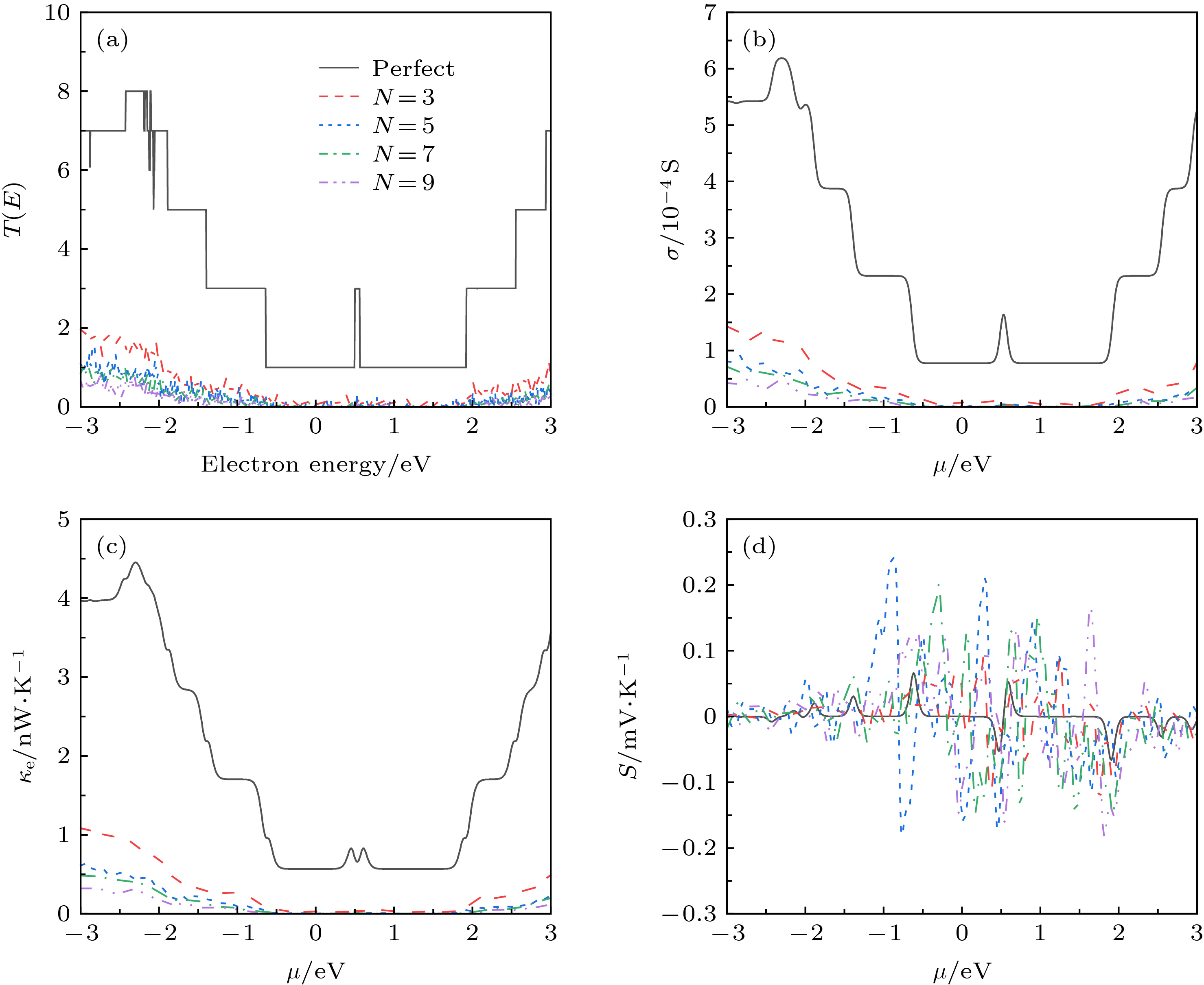
图 3 完美石墨烯纳米带和多晶石墨烯纳米带的电子性质 (a)电子透射系数; (b)电子电导; (c)电子热导; (d)塞贝克系数
Fig. 3. Electronic properties of perfect graphene and polycrystalline graphene: (a) The electronic transmission coefficient; (b) the electronic conductance; (c) the electronic contributed thermal conductance; (d) the Seebeck coefficient.
图 4 完美和多晶石墨烯纳米带的热电品质因子随温度的变化
Fig. 4. Peak values of ZT as a function of temperature for perfect graphene nanoribbons and polycrystalline graphene nanoribbons.
图 5 室温下(300 K), 完美与多晶石墨烯纳米带(N = 5)热电品质因子随系统(a)长度L和(b)宽度W的变化(阴影部分为标准偏差)
Fig. 5. Peak values of ZT of perfect and polycrystalline graphene nanoribbons (N = 5) at room temperature as a function of (a) nanoribbon length or (b) nanoribbon width. The shading part corresponds to the standard deviation.
-
[1] Tritt T M 2011 Annu. Rev. Mater. Res. 41 433
Google Scholar
[2] Dresselhaus M S, Chen G, Tang M Y, Yang R G, Lee H, Wang D Z, Ren Z F, Fleurial J P, Gogna P 2007 Adv. Mater. 19 1043
Google Scholar
[3] Snyder G J, Toberer E S 2008 Nat. Mater. 7 105
Google Scholar
[4] Zhu L, Li R, Yao K 2017 Phys. Chem. Chem. Phys. 19 4085
Google Scholar
[5] Zhu L, Li B, Yao K 2018 Nanotechnology 29 325206
Google Scholar
[6] Zhu L, Zou F, Gao G Y, Yao K 2017 Sci. Rep. 7 497
Google Scholar
[7] Geim A K, Novoselov K S 2007 Nat. Mater. 6 183
Google Scholar
[8] Geim A K 2009 Science 324 1530
Google Scholar
[9] Barone V, Hod O, Scuseria G E 2006 Nano Lett. 6 2748
Google Scholar
[10] Lee C, Wei X, Kysar J W, Hone J 2008 Science 321 385
Google Scholar
[11] Nan H Y, Ni Z H, Wang J, Zafar Z, Shi Z X, Wang Y Y 2013 J. Raman Spectrosc. 44 1018
Google Scholar
[12] Zhang H J, Zheng G G, Chen Y Y, Zou X J, Xu L H 2018 Chin. Phys. Lett. 35 038102
Google Scholar
[13] Bolotin K I, Sikes K J, Jiang Z, Klima M, Fudenberg G, Hone J, Kim P, Stormer H L 2008 Solid State Commun. 146 351
Google Scholar
[14] Dragoman D, Dragoman M 2007 Appl. Phys. Lett. 91 203116
Google Scholar
[15] Zhang H S, Guo Z X, Gong X G, Cao J X 2012 J. Appl. Phys. 112 123508
Google Scholar
[16] Nika D L, Pokatilov E P, Askerov A S, Balandin A A 2009 Phys. Rev. B 79 155413
Google Scholar
[17] Karamitaheri H, Pourfath M, Faez R, Kosina H 2011 J. Appl. Phys. 110 054506
Google Scholar
[18] Haskins J, Kınacı A, Sevik C, Sevinçli H, Cuniberti G, Çagǧın T 2011 ACS Nano 5 3779
Google Scholar
[19] 张嵛, 刘连庆, 焦念东, 席宁, 王越超, 董再励 2012 61 137101
Google Scholar
Zhang Y, Liu L Q, Jiao N D, Xi N, Wang Y C, Dong Z L 2012 Acta Phys. Sin. 61 137101
Google Scholar
[20] Tran V T, Saint Martin J, Dollfus P, Volz S 2017 Sci. Rep. 7 2313
Google Scholar
[21] 林琦, 陈余行, 吴建宝, 孔宗敏 2011 60 097103
Lin Q, Chen Y H, Wu J B, Kong Z M 2011 Acta Phys. Sin. 60 097103
[22] Huang P Y, Ruiz Vargas C S, van der Zande Arend M, Whitney W S, Levendorf M P, Kevek J W, Shivank G, Alden J S, Hustedt C J, Zhu Y, Park J W, McEuen P L, Muller D A 2011 Nature 469 389
Google Scholar
[23] Li X S, Cai W W, An J, Kim S, Nah J, Yang D X, Piner R, Velamakanni A, Jung I, Tutuc E, Banerjee S K, Colombo L, Ruoff R S 2009 Science 324 1312
Google Scholar
[24] Yazyev O V, Louie S G 2010 Nat. Mater. 9 806
Google Scholar
[25] Lee G H, Cooper R C, An S J, Lee S, Zande A, Petrone N, Hammerberg A G, Lee C, Crawford B, Oliver W, Kysar J W, Hone J 2013 Science 340 1073
Google Scholar
[26] Rassin G, Shenoy V B, Ruoff R S 2010 Science 330 946
Google Scholar
[27] Bagri A, Kim S P, Ruoff R S, Shenoy V B 2011 Nano Lett. 11 3917
Google Scholar
[28] Wang J S, Wang J, Lu J T 2008 Eur. Phys. J. B 62 381
Google Scholar
[29] Yamamoto T, Watanabe K 2006 Phys. Rev. Lett. 96 255503
Google Scholar
[30] Xiao H P, Cao W, Ouyang T, Xu X Y, Ding Y C, Zhong J X 2018 Appl. Phys. Lett. 112 233107
Google Scholar
[31] Ghosh S, Calizo I, Teweldebrhan D, Pokatilov E P, Nika D L, Balandin A A, Bao W, Miao F, Lau C N 2008 Appl. Phys. Lett. 92 151911
Google Scholar
[32] Li T C, Lu S P 2008 Phys. Rev. B 77 085408
Google Scholar
[33] Pereira V M, Neto A H C, Peres N M R 2009 Phys. Rev. B 80 045401
[34] Ouyang T, Hu M 2014 Nanotechnology 25 245401
Google Scholar
下载:
计量
- 文章访问数: 7881
- PDF下载量: 155
- 被引次数: 0
