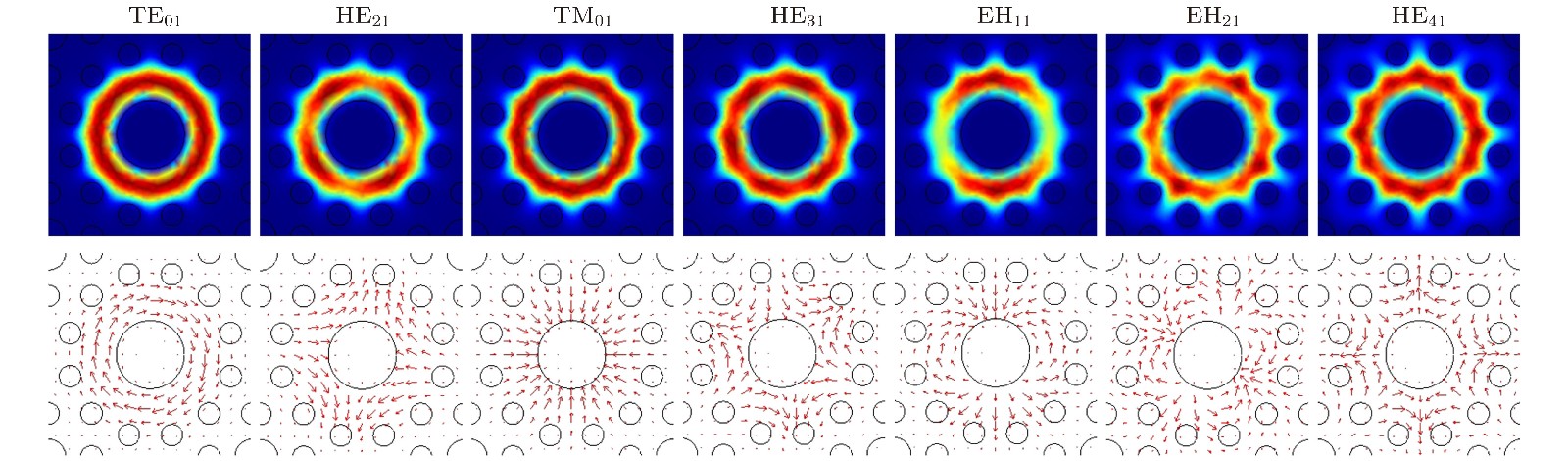
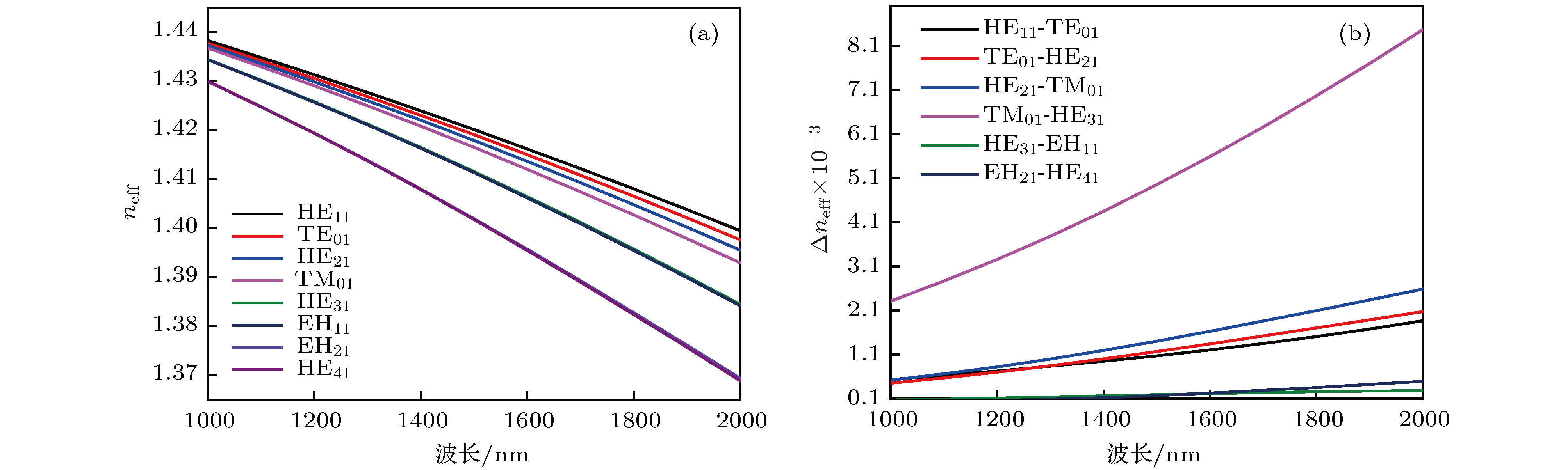
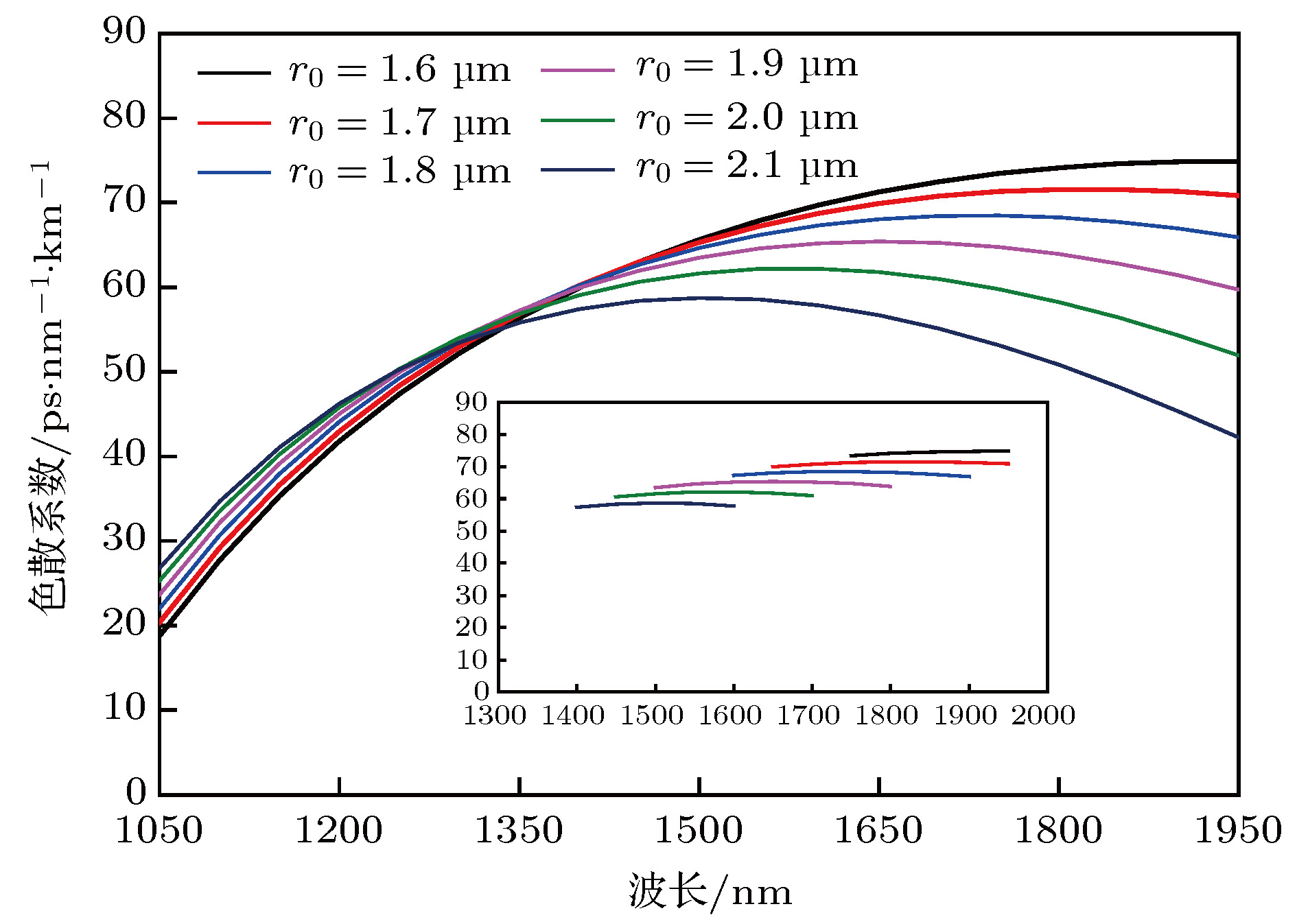
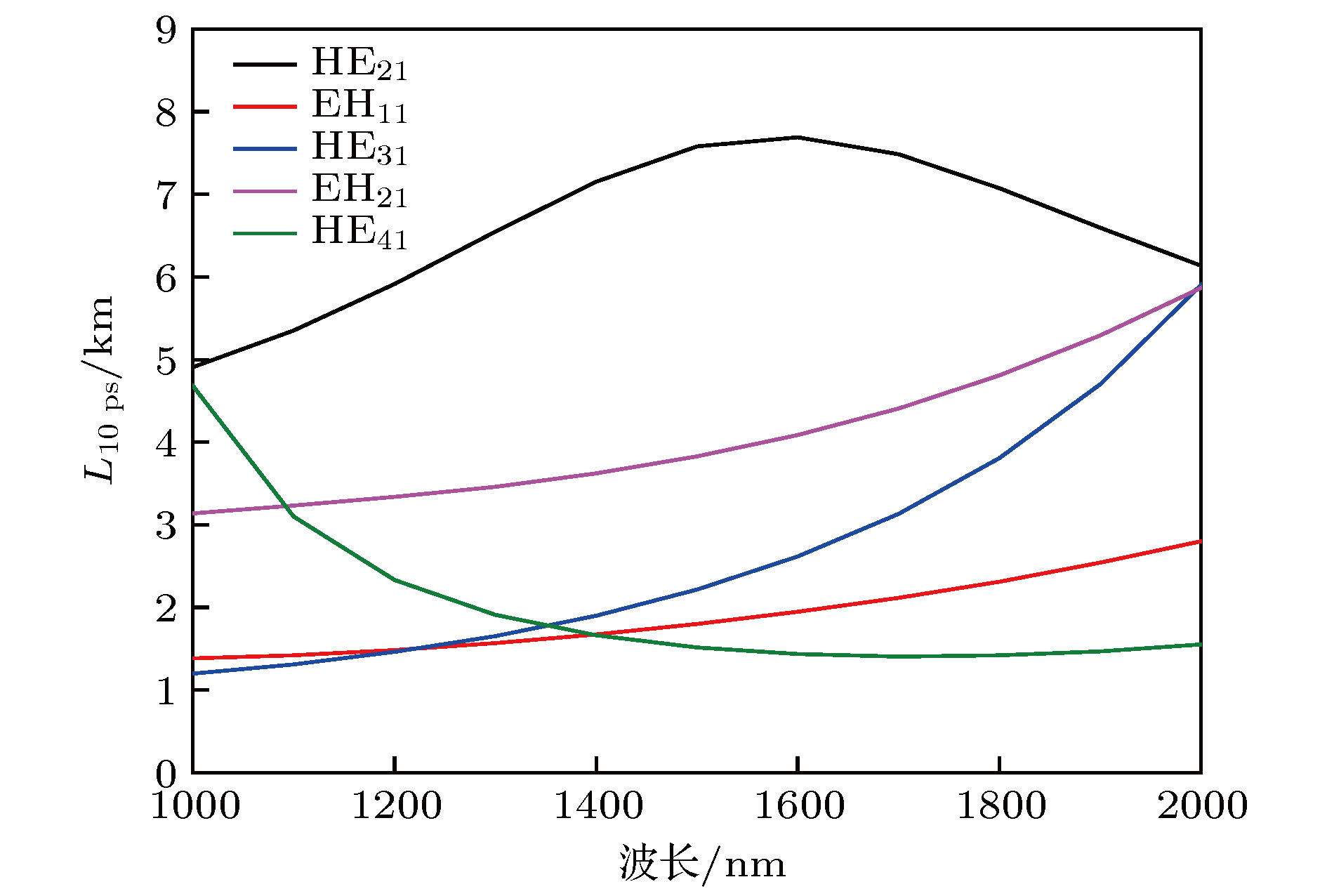
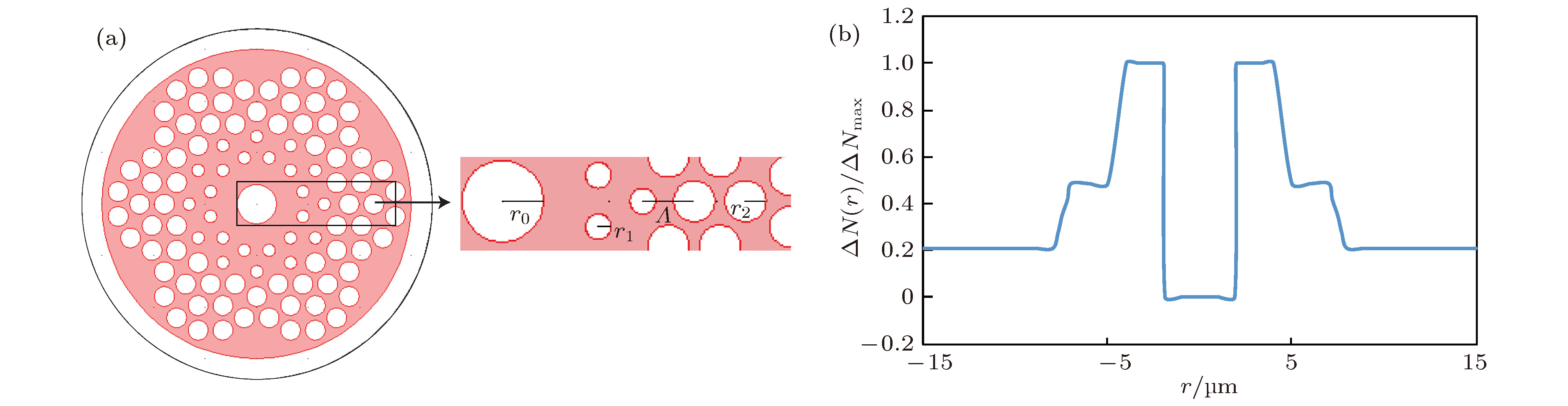
-
设计了一种新型的六重准晶涡旋光光子晶体光纤, 利用矢量有限元分析方法进行了数值模拟. 研究结果表明光纤中模式有效折射率差
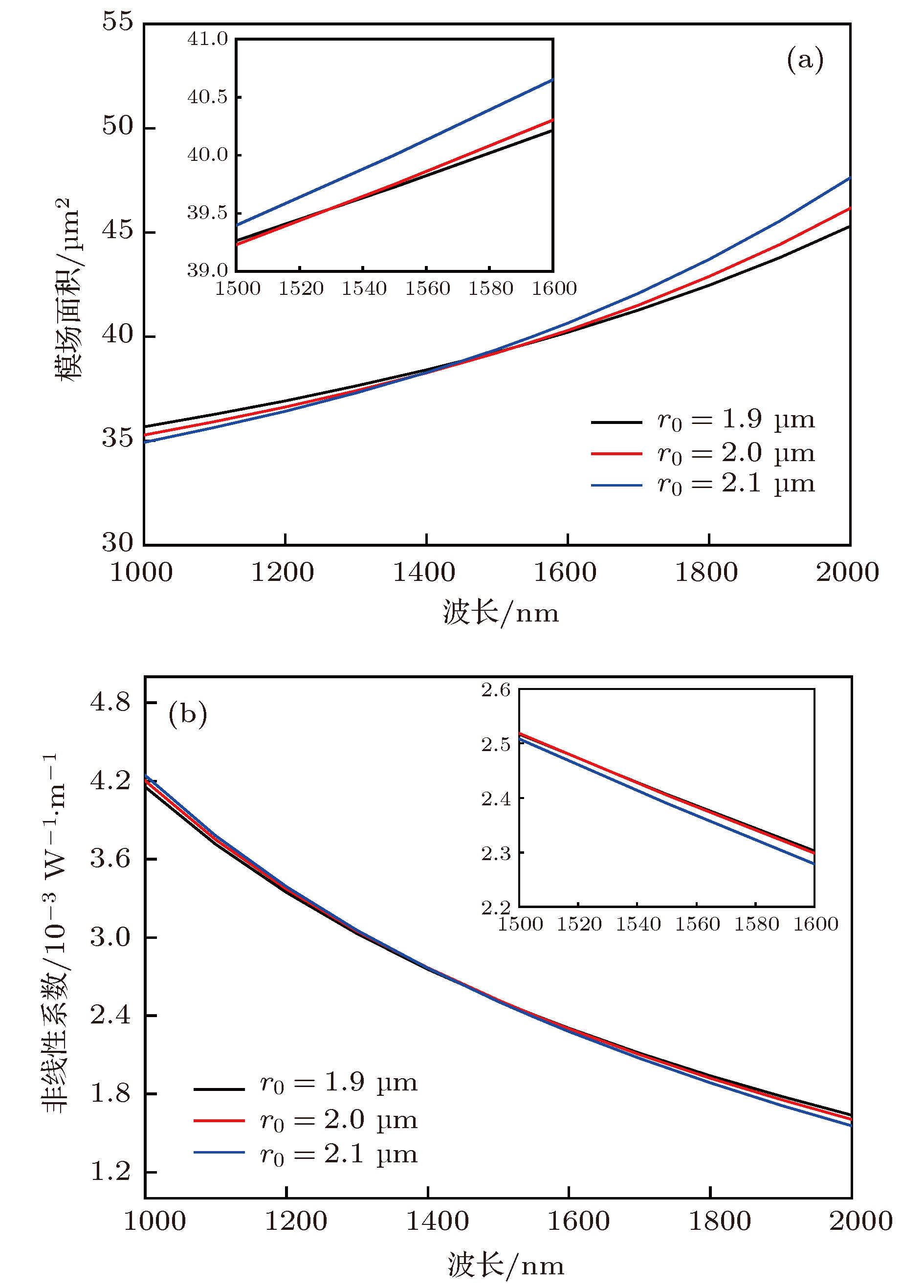
$ \Delta {n_{{\rm{eff}}}} > {10^{ - 4}}$ , 实现了7个本征矢量模(10个相位涡旋光)的稳定传输, 并以${\rm{H}}{{\rm{E}}_{21}}\normalsize$ 为对象, 对光纤模式的传输特性进行了分析研究. 研究结果表明, 在波段1500—1600 nm内, 涡旋光模式的限制性损耗在10–8—10–7量级, 模场面积保持在40 μm2, 非线性系数在10–3量级. 通过改变光纤中心空气孔的大小, 能够实现特定波段的色散平坦趋势, 当中心空气孔为1.9 μm时, 光纤能够在1500—1800 nm波段保持色散平坦, 色散系数维持在63.51—65.42 ps·nm–1·km–1之间.In an optical fiber communication system, vortex beams have aroused great interest in the last several decades. Vortex beams possess many intriguing properties. For example, they have the ability to carry orbital angular momentum (OAM) which is mutually orthogonal. The OAM is a fundamental physical quantity of light which can be used as information carriers for transmission channel of optical fiber. Combined with the existing multiplexing techniques such as wavelength division multiplexing technique, advanced multilevel amplitude modulation formats, etc., the vortex beams provide an alternative to the increase of the transmission capacity and spectral efficiency of the optical fiber transmission system. Recently, long-length transmission of vortex-beam in optical fiber has been realized and there have also occurred some new designs of optical fiber on vortex beams, such as air-core ring shaped fiber, graded index vortex fiber, multi-ring fiber, and supermode fiber. Photonic crystal fiber (PCF) is flexible in design. Therefore, it is easy to regulate the transmission performance of PCF by adjusting the radius and the pitch of the air holes and so on. In this paper, we propose a newly designed sixfold photonic quasi-crystal fiber (SPQCF) to transmit vortex beams stably. Transmission characteristics of this newly designed fiber are simulated and calculated by using COMSOL multiphysics software. When the wavelength of the incident light is 1550 nm, the effective index difference between the vortex modes in a group is more than 10–4 which is large enough to preclude the LP modes from being formed, and to transmit 7 vector modes (10 OAM modes). Changing the radius and pitch of the air holes, we can regulate the dispersion characteristic and confinement loss of the SPQCF flexibly. At 1550 nm, the confinement loss of the SPQCF maintains 10–8−10–7 which is low enough to confine the vortex beams in the fiber core. When the incident light wavelength of HE21 ranges from 1500 nm to 1800 nm (r0 = 1.9 μm), the dispersion coefficient of the SPQCF is between 63.51−65.42 ps·nm–1·km–1 which tends to be flat. By changing r0, the flat trend is adjusted to different wavelength range. This dispersion characteristic possesses great potential for the transmission of optical solitons. The effective mode area (HE21) is about 40 μm2 and the nonlinear coefficient (HE21) is maintained on the order of 10–3 between 1500−1600 nm. These features suppress the generation of nonlinear effect in the fiber and benefit the transmission of vortex beams. The stable transmission distance is longer than 1 km. In summary, we design a new type of PCF featuring quasi-crystal structure which has a ring shaped fiber core and supports the transmission of vortex beams stably.-
Keywords:
- photonic quasi-crystal fiber /
- vortex beams /
- dispersion /
- confinement loss
[1] Ramachandran S, Kristensen P 2013 Nanophotonics 2 455
[2] Curtis J E, Grier D G 2003 Opt. Lett. 28 872
Google Scholar
[3] Tabosa J W R, Petrov D V 1999 Phys. Rev. Lett. 83 4967
Google Scholar
[4] Vaziri A, Pan J W, Jennewein T, Weihs G, Zeilinger A 2003 Phys. Rev. Lett. 91 227902
Google Scholar
[5] Brunet C, Rusch L A 2016 Opt. Fiber Technol. 31 172
Google Scholar
[6] Wong K L G G, Xi X, Kang M S, Lee H W, Russell P 2012 Science 337 446
Google Scholar
[7] Bozinovic N, Yue Y, Ren Y, Tur M, Kristensen P, Huang H, Willner A E, Ramachandran S 2013 Science 340 1545
Google Scholar
[8] Coullet P, Gil L, Rocca F 1989 Opt. Commun. 73 403
Google Scholar
[9] Allen L, Beijersbergen M W, Spreeuw R, Woerdman J 1992 Phys. Rev. A 45 8185
Google Scholar
[10] McGloin D, Simpson N B, Padgett M J 1998 Appl. Opt. 37 469
Google Scholar
[11] Ramachandran S, Kristensen P, Yan M F 2009 Opt. Lett. 34 2525
Google Scholar
[12] Li S, Mo Q, Hu X, Du C, Wang J 2015 Opt. Lett. 40 4376
Google Scholar
[13] Yan Y, Zhang L, Wang J, Yang J Y, Fazal I M, Ahmed N, Willner A E, Dolinar S J 2012 Opt. Lett. 37 3294
Google Scholar
[14] Brunet C, Vaity P, Messaddeq Y, LaRochelle S, Rusch L A 2014 Opt. Express 22 26117
Google Scholar
[15] Brunet C, Ung B, Wang L, Messaddeq Y, LaRochelle S, Rusch L A 2015 Opt. Express 23 10553
Google Scholar
[16] Li S, Wang J 2013 IEEE Photon. J. 5 7101007
Google Scholar
[17] Li S, Wang J 2014 Sci. Rep. 4 3853
[18] Xia C, Bai N, Ozdur I, Zhou X, Li G 2011 Opt. Express 19 16653
Google Scholar
[19] Li S, Wang J 2015 Opt. Express 23 18736
Google Scholar
[20] Ung B, Vaity P, Wang L, Messaddeq Y, Rusch L, LaRochelle S 2014 Opt. Express 22 18044
Google Scholar
[21] Zhang Z, Gan J, Heng X, Wu Y, Li Q, Qian Q, Chen D, Yang Z 2015 Opt. Express 23 29331
Google Scholar
[22] Ferrando A, Silvestre E, Andres P, Miret J J, Andrés M V 2001 Opt. Express 9 687
Google Scholar
[23] Yue Y, Zhang L, Yan Y, Ahmed N, Yang J Y, Huang H, Ren Y, Dolinar S, Tur M, Willner A E 2012 Opt. Lett. 37 1889
Google Scholar
[24] Zhao C, Gan X, Li P, Fang L, Han L, Tu L, Zhao J 2016 J. Lightwave Technol. 34 1206
Google Scholar
[25] Zhang H, Zhang W, Xi L, Tang X, Zhang X, Zhang X 2016 IEEE Photon. Technol. Lett. 28 1426
Google Scholar
[26] Jin C, Cheng B, Man B, Li Z, Zhang D 2000 Phys. Rev. B 61 10762
Google Scholar
[27] Jin C, Meng X, Cheng B, Li Z, Zhang D 2001 Phys. Rev. B 63 195107
Google Scholar
-
图 1 六重准晶涡旋光光子晶体光纤 (a)光纤端面图; (b)折射率分布图
Fig. 1. The sixfold photonic quasi-crystal fiber: (a) Cross-section of SPQCF; (b) index profile of SPQCF.
图 3 (a)模式有效折射率(
${n_{{\rm{eff}}}}$ ); (b)模式间有效折射率差($\Delta {n_{{\rm{eff}}}}$ )随波长的变化曲线Fig. 3. (a) Effective refractive indices; (b) effective index difference as a function of wavelength for vector modes in SPQCF.
图 4 HE21模的限制性损耗随波长的变化(不同中心空气孔半径), 插图为1500—1600 nm波段内HE21模的限制性损耗
Fig. 4. Confinement loss as a function of wavelength for HE21 mode with different r0, the inset shows the loss between 1500−1600 nm.
图 5
${\rm{H}}{{\rm{E}}_{21}}$ 模色散系数随波长的变化(不同中心空气孔半径), 插图为${r_0}$ 对涡旋光平坦趋势的影响Fig. 5. Dispersion as a function of wavelength for
${\rm{H}}{{\rm{E}}_{21}}$ with different${r_0}$ , the inset shows the flat trend with di-fferent${r_0}$ 
图 6 (a) HE21模的模场面积; (b) HE21模的非线性系数随波长的变化 (不同中心空气孔半径); (a), (b) 的插图分别为 波段内的模场面积和非线性系数
Fig. 6. (a) Effective mode area of HE21, (b) nonlinear coefficient as a function of wavelength for HE21 mode with different r0, the inset shows the (a) effective modes area and (b) nonlinear coefficient between 1500−1600 nm.
图 7 光纤中不同本征模的
${L_{10\;{\rm{ps}}}}$ 随波长的变化Fig. 7.
${L_{10\;{\rm{ps}}}}$ as a function of wavelength for vortex modes in SPQCF. -
[1] Ramachandran S, Kristensen P 2013 Nanophotonics 2 455
[2] Curtis J E, Grier D G 2003 Opt. Lett. 28 872
Google Scholar
[3] Tabosa J W R, Petrov D V 1999 Phys. Rev. Lett. 83 4967
Google Scholar
[4] Vaziri A, Pan J W, Jennewein T, Weihs G, Zeilinger A 2003 Phys. Rev. Lett. 91 227902
Google Scholar
[5] Brunet C, Rusch L A 2016 Opt. Fiber Technol. 31 172
Google Scholar
[6] Wong K L G G, Xi X, Kang M S, Lee H W, Russell P 2012 Science 337 446
Google Scholar
[7] Bozinovic N, Yue Y, Ren Y, Tur M, Kristensen P, Huang H, Willner A E, Ramachandran S 2013 Science 340 1545
Google Scholar
[8] Coullet P, Gil L, Rocca F 1989 Opt. Commun. 73 403
Google Scholar
[9] Allen L, Beijersbergen M W, Spreeuw R, Woerdman J 1992 Phys. Rev. A 45 8185
Google Scholar
[10] McGloin D, Simpson N B, Padgett M J 1998 Appl. Opt. 37 469
Google Scholar
[11] Ramachandran S, Kristensen P, Yan M F 2009 Opt. Lett. 34 2525
Google Scholar
[12] Li S, Mo Q, Hu X, Du C, Wang J 2015 Opt. Lett. 40 4376
Google Scholar
[13] Yan Y, Zhang L, Wang J, Yang J Y, Fazal I M, Ahmed N, Willner A E, Dolinar S J 2012 Opt. Lett. 37 3294
Google Scholar
[14] Brunet C, Vaity P, Messaddeq Y, LaRochelle S, Rusch L A 2014 Opt. Express 22 26117
Google Scholar
[15] Brunet C, Ung B, Wang L, Messaddeq Y, LaRochelle S, Rusch L A 2015 Opt. Express 23 10553
Google Scholar
[16] Li S, Wang J 2013 IEEE Photon. J. 5 7101007
Google Scholar
[17] Li S, Wang J 2014 Sci. Rep. 4 3853
[18] Xia C, Bai N, Ozdur I, Zhou X, Li G 2011 Opt. Express 19 16653
Google Scholar
[19] Li S, Wang J 2015 Opt. Express 23 18736
Google Scholar
[20] Ung B, Vaity P, Wang L, Messaddeq Y, Rusch L, LaRochelle S 2014 Opt. Express 22 18044
Google Scholar
[21] Zhang Z, Gan J, Heng X, Wu Y, Li Q, Qian Q, Chen D, Yang Z 2015 Opt. Express 23 29331
Google Scholar
[22] Ferrando A, Silvestre E, Andres P, Miret J J, Andrés M V 2001 Opt. Express 9 687
Google Scholar
[23] Yue Y, Zhang L, Yan Y, Ahmed N, Yang J Y, Huang H, Ren Y, Dolinar S, Tur M, Willner A E 2012 Opt. Lett. 37 1889
Google Scholar
[24] Zhao C, Gan X, Li P, Fang L, Han L, Tu L, Zhao J 2016 J. Lightwave Technol. 34 1206
Google Scholar
[25] Zhang H, Zhang W, Xi L, Tang X, Zhang X, Zhang X 2016 IEEE Photon. Technol. Lett. 28 1426
Google Scholar
[26] Jin C, Cheng B, Man B, Li Z, Zhang D 2000 Phys. Rev. B 61 10762
Google Scholar
[27] Jin C, Meng X, Cheng B, Li Z, Zhang D 2001 Phys. Rev. B 63 195107
Google Scholar
下载:
计量
- 文章访问数: 8128
- PDF下载量: 71
- 被引次数: 0
