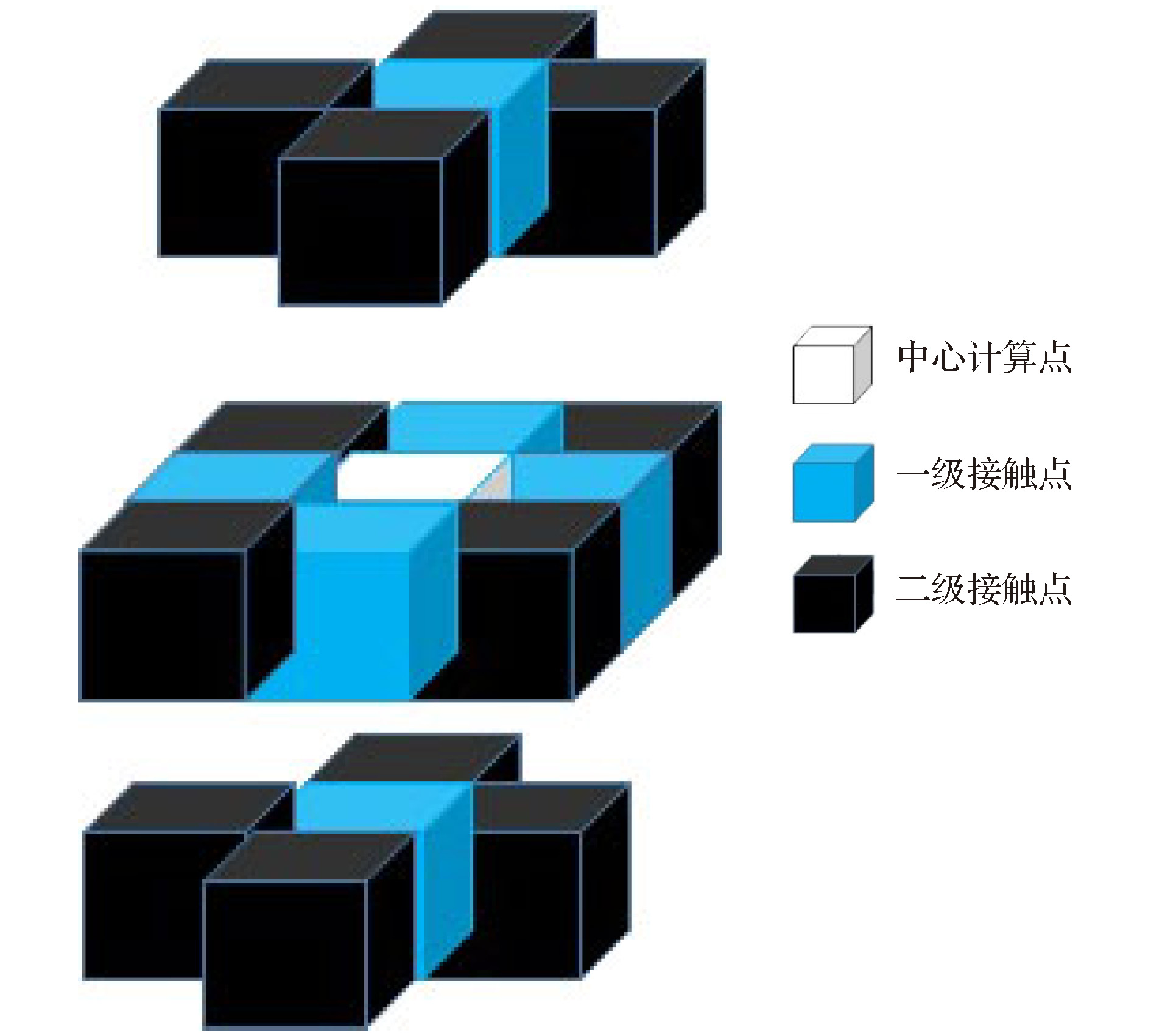
-
纳米流体液膜在自然状态下蒸发干燥后除了形成单一尺度的网状连续结构、枝状分形结构以及微尺寸环结构等沉积结构, 还会形成双尺度细胞网络沉积结构和双尺度纳米粒子环状沉积结构. 为了更直观地研究纳米流体液膜双尺度沉积结构的形成机理, 本文在二维动力学蒙特卡罗模型的基础上, 建立了三维动力学蒙特卡罗模型, 并加入了耦合溶剂蒸发率的动态化学势, 成功模拟出了双尺度细胞网络沉积结构和双尺度纳米粒子环状沉积结构, 并讨论了动态化学势中化学势锐度与流体临界蒸发率对纳米流体液膜蒸发自组装双尺度沉积结构的影响. 模拟结果与文献中的实验结果吻合良好.Self-assembly of nanomaterials from the drying of nanofluid films has aroused great interest due to its applications in micro/nano fabrication, ink-jet printing, and thin film coatings. Numerical models are developed to investigate the single-scale deposition structures from the drying of nanofluid films, including network structures, continuous labyrinthine, branched structures and micro-sized rings. In the case of the actual drying of nanofluid films, dual-scale cellular networks and nano-rings are also discovered. In order to study the formation mechanism of dual-scale deposition structures, a three-dimensional kinetic Monte Carlo model is developed based on two-dimensional lattice gas model, and the dynamic chemical potential which couples solvent evaporation rate is implemented. Different dynamic chemical potentials are defined for each layer of the thin-film in the model to mimic the real evaporation situation. Considering the Brownian motion and the interaction between particles, the formation of dual-scale cellular networks and nano-rings coexisting with small scale patternis achieved via coupling the chemical potential to the solvent evaporation rate. The simulation results accord well with the results from many experimentally studied de-wetting systems. The effects of the chemical potential sharpness and critical evaporation rate of fluids on the dual-scale deposition structures are discussed. It can be found that the evaporation mode of thin-film is dominated by nucleation and growth at the initial stage. If the spinodal point is passed, the residual solvent will evaporate suddenly, and the nanoparticles do not accumulate further but directly deposit into small-scale structures, thus forming a dual-scale deposition structures at the final stage of the evaporation. The simulation results also show that the chemical potential sharpness will affect the deposition structure after the mutation in a certain range. When the chemical potential sharpness equals zero, the sedimentary structure is the same as the single-scale sedimentary structure when the constant chemical potential is applied. When the chemical potential sharpness is small, the large-scale network structure interacts closely with the small-scale network structure. With the increase of chemical potential sharpness, the large-scale deposition structure remains unchanged, while the dense small-scale network structure becomes small-scale point structure. When the chemical potential sharpness exceeds a certain large value, the effect of chemical potential sharpness on the deposition structure will gradually decrease, and finally the dual-scale deposition structure will remain unchanged. The critical evaporation rate of fluids determines the area ratio of the two kind of structures in the dual-scale deposition. With the increase of the critical evaporation rate of fluids, the area ratio of small-scale structures decreases while that of the large-scale structure increases. When critical evaporation rate increases to a certain value, the final deposition structure will evolve into a single-scale deposition structure.
-
Keywords:
- 3-dimensional simulation /
- dynamic chemical potential /
- self-assembly of nanoparticles /
- dual-scale deposition structure
[1] Asha S K, Sunitha G 2019 J. Tai. Univ. Sci. 13 155
Google Scholar
[2] Chen P W, Lee N C, Chien Y H, Wu J Y, Wang P C, Hwu W L 2014 Clin. Chim. Acta 431 19
Google Scholar
[3] Shan Y G, Coyle T, Mostaghimi J 2007 J. Therm. Spray Technol. 16 736
Google Scholar
[4] Sou T, Kaminskas L M, Nguyen T H, Carlberg R, Mcintosh M P, Morton D A V 2013 Eur. J. Pharm. Biopharm. 83 234
Google Scholar
[5] Parsaiemehr M, Pourfattah F, Akbari O A, Toghraie D, Sheikhzadeh G 2017 Physica E 96 73
[6] Shan Y G, Wang Y L, Coyle T 2013 Appl. Therm. Eng. 51 690
Google Scholar
[7] Robbins M J, Archer A J, Thiele U 2011 J. Phys.: Condens. Matter 23 415102
Google Scholar
[8] Chan H C, Paik S, Tipton Jr J B, Kihm K D 2007 Langmuir 23 2953
Google Scholar
[9] Cui L, Zhang J, Zhang X 2012 Soft Matter. 8 10448
Google Scholar
[10] Bhardwaj R, Fang X, Somasundaran P, Attinger D 2010 Langmuir 26 7833
Google Scholar
[11] Chokprasombat K, Sirisathitkul C, Ratphonsan P 2014 Surf. Sci. 621 162
Google Scholar
[12] Zhong X, Crivoi A, Duan F 2015 Adv. Colloid Interface Sci. 217 13
Google Scholar
[13] Hamaker H C 1937 Phy. Sec. A 4 1058
[14] Hofman J A. M H, Stein H N 1992 J. Colloid Interface Sci. 154 359
Google Scholar
[15] Deegan R D, Bakajin O, Dupont T F, Huber G, Nagel S R, Witten T A 1997 Nature 389 827
Google Scholar
[16] Rabani E, Reichman D R, Geissler P L 2003 Nature 426 271
Google Scholar
[17] Seike W, Fisher M E 1980 Eur. Phys. J. B 40 71
[18] Crivoi A, Duan F 2012 Phys. Chem. Chem. Phys. 14 1449
Google Scholar
[19] Zhang H, Shan Y G, Li L, Lu M, Li R 2016 Appl. Therm. Eng. 94 650
Google Scholar
[20] Martin C P, Blunt M O, Moriary P 2004 Nano Lett. 4 2389
Google Scholar
[21] Stannard A, Martin C P, Pauliac V E, Philip M 2011 J. Phys. Chem. C 112 15195
[22] Vancea I, Thiele U, Pauliac E A, Stannard A, Martin C P, Blunt M O, Moriarty P J 2007 Phys. Rev. Lett. 99 116103
Google Scholar
[23] Yosef G, Rabani E 2006 J. Phys. Chem. B 110 20965
Google Scholar
[24] Lyushnin A V, Golovin A A, Pismen L M 2002 Phys. Rev. E 65 021602
[25] Vancea I, Thiele U 2008 Phys. Rev. E 78 041601
[26] Frastia L, Archer A J, Thiele U 2011 Phys. Rev. Lett. 106 077801
Google Scholar
[27] Zhang X, Crivoi A, Duan F 2015 Sci. Rep. 5 10926
Google Scholar
[28] Sztrum C G, Hod O, Rabani E 2005 J. Phys. Chem. B 109 6741
Google Scholar
[29] 曹进军, 单彦广 2018 化学通报 81 641
Cao J J, Shan Y G 2018 Chem. Bull. 81 641
[30] Stannard A 2011 J. Phys.: Condens. Matter 23 083001
Google Scholar
[31] Jacobs K, Seemann R, Herminghaus S 2008 Eprint Arxiv. 243
-
图 3 三维纳米流体液膜各层蒸发图
Fig. 3. Evaporation diagram of each layer of 3D nanofluid thin-film.
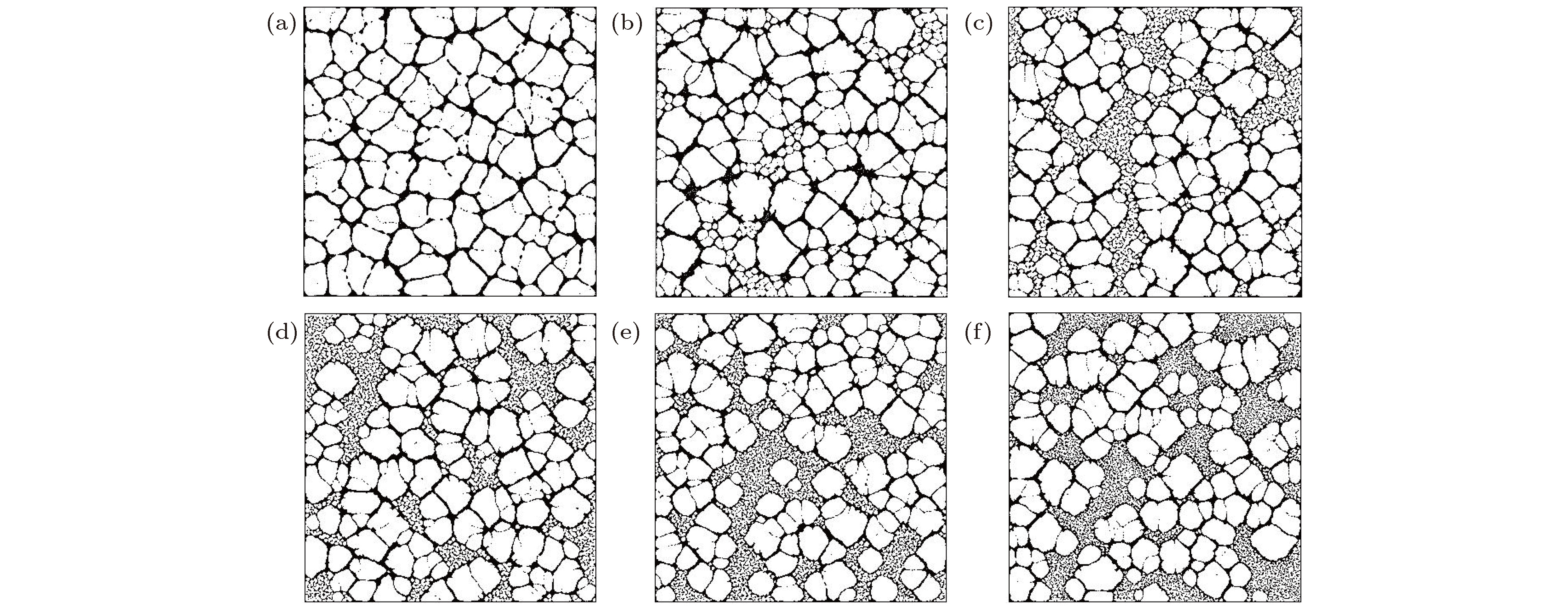
图 7 不同化学势锐度下封闭纳米流体液膜的沉积结构图 (a) Δμf = 0; (b) Δμf = 0.050; (c) Δμf = 0.100; (d) Δμf = 0.125; (e) Δμf = 0.150; (f) Δμf = 0.200
Fig. 7. Sedimentary pattern of nanofluid thin-film at different chemical potential sharpness: (a) Δμf = 0; (b) Δμf = 0.050; (c) Δμf = 0.100; (d) Δμf = 0.125; (e) Δμf = 0.150; (f) Δμf = 0.200
图 8 不同临界蒸发率下封闭纳米流体液膜的沉积结构图 (a) νs = 1%; (b) νs = 10%; (c) νs = 30%; (d) νs = 55%; (e) νs = 80%; (f) νs = 100%
Fig. 8. Sedimentary pattern of nanofluid thin-film at different critical evaporation rates: (a) νs = 1%; (b) νs = 10%; (c) νs = 30%; (d) νs = 55%; (e) νs = 80%; (f) νs = 100%.
-
[1] Asha S K, Sunitha G 2019 J. Tai. Univ. Sci. 13 155
Google Scholar
[2] Chen P W, Lee N C, Chien Y H, Wu J Y, Wang P C, Hwu W L 2014 Clin. Chim. Acta 431 19
Google Scholar
[3] Shan Y G, Coyle T, Mostaghimi J 2007 J. Therm. Spray Technol. 16 736
Google Scholar
[4] Sou T, Kaminskas L M, Nguyen T H, Carlberg R, Mcintosh M P, Morton D A V 2013 Eur. J. Pharm. Biopharm. 83 234
Google Scholar
[5] Parsaiemehr M, Pourfattah F, Akbari O A, Toghraie D, Sheikhzadeh G 2017 Physica E 96 73
[6] Shan Y G, Wang Y L, Coyle T 2013 Appl. Therm. Eng. 51 690
Google Scholar
[7] Robbins M J, Archer A J, Thiele U 2011 J. Phys.: Condens. Matter 23 415102
Google Scholar
[8] Chan H C, Paik S, Tipton Jr J B, Kihm K D 2007 Langmuir 23 2953
Google Scholar
[9] Cui L, Zhang J, Zhang X 2012 Soft Matter. 8 10448
Google Scholar
[10] Bhardwaj R, Fang X, Somasundaran P, Attinger D 2010 Langmuir 26 7833
Google Scholar
[11] Chokprasombat K, Sirisathitkul C, Ratphonsan P 2014 Surf. Sci. 621 162
Google Scholar
[12] Zhong X, Crivoi A, Duan F 2015 Adv. Colloid Interface Sci. 217 13
Google Scholar
[13] Hamaker H C 1937 Phy. Sec. A 4 1058
[14] Hofman J A. M H, Stein H N 1992 J. Colloid Interface Sci. 154 359
Google Scholar
[15] Deegan R D, Bakajin O, Dupont T F, Huber G, Nagel S R, Witten T A 1997 Nature 389 827
Google Scholar
[16] Rabani E, Reichman D R, Geissler P L 2003 Nature 426 271
Google Scholar
[17] Seike W, Fisher M E 1980 Eur. Phys. J. B 40 71
[18] Crivoi A, Duan F 2012 Phys. Chem. Chem. Phys. 14 1449
Google Scholar
[19] Zhang H, Shan Y G, Li L, Lu M, Li R 2016 Appl. Therm. Eng. 94 650
Google Scholar
[20] Martin C P, Blunt M O, Moriary P 2004 Nano Lett. 4 2389
Google Scholar
[21] Stannard A, Martin C P, Pauliac V E, Philip M 2011 J. Phys. Chem. C 112 15195
[22] Vancea I, Thiele U, Pauliac E A, Stannard A, Martin C P, Blunt M O, Moriarty P J 2007 Phys. Rev. Lett. 99 116103
Google Scholar
[23] Yosef G, Rabani E 2006 J. Phys. Chem. B 110 20965
Google Scholar
[24] Lyushnin A V, Golovin A A, Pismen L M 2002 Phys. Rev. E 65 021602
[25] Vancea I, Thiele U 2008 Phys. Rev. E 78 041601
[26] Frastia L, Archer A J, Thiele U 2011 Phys. Rev. Lett. 106 077801
Google Scholar
[27] Zhang X, Crivoi A, Duan F 2015 Sci. Rep. 5 10926
Google Scholar
[28] Sztrum C G, Hod O, Rabani E 2005 J. Phys. Chem. B 109 6741
Google Scholar
[29] 曹进军, 单彦广 2018 化学通报 81 641
Cao J J, Shan Y G 2018 Chem. Bull. 81 641
[30] Stannard A 2011 J. Phys.: Condens. Matter 23 083001
Google Scholar
[31] Jacobs K, Seemann R, Herminghaus S 2008 Eprint Arxiv. 243
下载:
计量
- 文章访问数: 7601
- PDF下载量: 76
- 被引次数: 0
