Persistency of tripartite nonlocality sharing with noise

Zhao Lin-Yang; He Kan; Zhang Yan-Fang

doi:10.7498/aps.73.20241150

Abstract

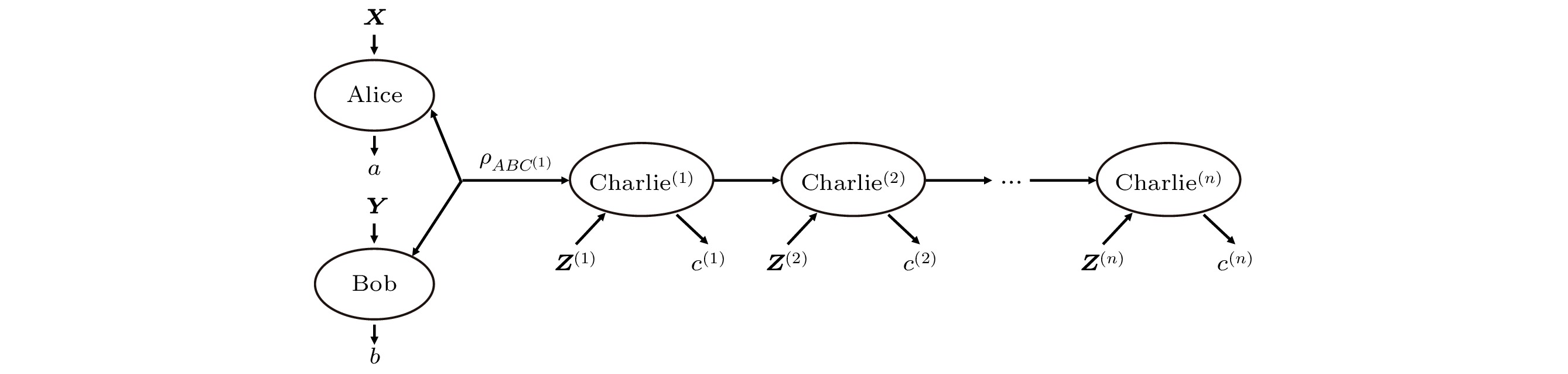
Recently, researchers have proven that an infinite number of Charlies and a pair of Alice and Bob can share standard tripartite nonlocality and genuinely nonsignal nonlocality by violating the Mermin and NS inequalities within tripartite systems. This discovery undoubtedly provides new perspectives and potential in quantum information science. However, it should be noted that the above-mentioned conclusion is derived on the highly idealized assumption that the quantum system is perfect and free from external disturbances. In reality, the realization of this ideal state is a challenging proposition. As a fundamental aspect of quantum mechanics, the phenomenon of quantum entanglement is susceptible to the influence of external factors, such as noise, during its practical implementation. Additionally, the process of quantum measurement can introduce potential errors, which may potentially diminish or even negate the observed quantum nonlocality. In light of the above situation, we investigate whether it is possible to share the corresponding quantum nonlocality, despite the inevitable occurrence of noise and error. This paper aims to study and discuss the persistency of nonlocality in noisy three-qubit systems. Firstly, the sufficient conditions are provided for Alice and Bob to share standard tripartite nonlocality with any number of Charlies, even when measurements are noisy and the initial three-qubit system is in a maximally entangled state with noise. This finding indicates that certain standard tripartite nonlocality can persist under non-ideal conditions as long as certain conditions are met. Moreover, this article elucidates the necessary conditions for multiple independent Charlies to share genuinely nonsignal nonlocality with a pair of Alice and Bob in a non-ideal state. This implies that despite the presence of noise and errors, this type of genuinely nonsignal nonlocality can still be securely shared among multiple parties as long as specific conditions are met. This research provides a new theoretical basis for the security and feasibility of quantum communication. The comprehensive analysis presented in this paper offers insights into the behavior of triple quantum nonlocality under noiseless conditions.

Keywords:

Authors and contacts

College of Mathematics, Taiyuan University of Technology, Taiyuan 030024, China

Corresponding author: He Kan, hekanquantum@163.com ; Zhang Yan-Fang, zhangyanfang@tyut.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant No. 12271394).

FullText HTML

References

[1]	Bell J S 1964 Phys. Phys. Fiz. 1 195 Google Scholar
[2]	Barrett J, Hardy L, Kent A 2005 Phys. Rev. Lett. 95 010503 Google Scholar
[3]	Acín A, Brunner N, Gisin N, Massar S, Pironio S, Scarani V 2007 Phys. Rev. Lett. 98 230501 Google Scholar
[4]	Li J J, Wang Y, Li H W, Bao W S 2020 Chin. Phys. B 29 030303 Google Scholar
[5]	周贤韬, 江英华 2023 72 020302 Google Scholar Zhou X T, Jiang Y H 2023 Acta Phys. Sin. 72 020302 Google Scholar
[6]	张沛, 周小清, 李智伟 2014 63 130301 Google Scholar Zhao P, Zhou X Q, Li Z W 2014 Acta Phys. Sin. 63 130301 Google Scholar
[7]	Dynes J F, Yuan Z L, Sharpe A W, Shields A J 2008 Appl. Phys. Lett. 93 031109 Google Scholar
[8]	Acín A, Masanes L 2016 Nature 540 213 Google Scholar
[9]	Curchod F J, Johansson M, Augusiak R, Hoban M J, Wittek P, Acín A 2017 Phys. Rev. A 95 020102 Google Scholar
[10]	Colbeck R, Renner R 2012 Nat. Phys. 8 450 Google Scholar
[11]	Colbeck R, Kent A 2011 J. Phys. A: Math. Theor. 44 095305 Google Scholar
[12]	李宏欣, 王相宾, 刘欣, 韩宇, 闫宝, 王伟 2017 现代物理 7 257 Google Scholar Li H X, Wang X B, Liu X, Han Y, Yan B, Wang W 2017 Modern Physics 7 257 Google Scholar
[13]	杜聪, 王金东, 秦晓娟, 魏正军, 於亚飞, 张智明 2020 69 190301 Google Scholar Du C, Wang J D, Qin X J, Wei Z J, Yu Y F, Zhang Z M 2020 Acta Phys. Sin. 69 190301 Google Scholar
[14]	东晨, 赵尚弘, 董毅, 赵卫虎, 赵静 2014 63 170303 Google Scholar Dong C, Zhao S H, Dong Y, Zhao W H, Zhao J 2014 Acta Phys. Sin. 63 170303 Google Scholar
[15]	Silva R, Gisin N, Guryanova Y, Popescu S 2015 Phys. Rev. Lett. 114 250401 Google Scholar
[16]	Mal S, Majumdar A, Home D 2016 Mathematics 4 48 Google Scholar
[17]	Shenoy H A, Designolle S, Hirsch F, Silva R, Gisin N, Brunner N 2019 Phys. Rev. A 99 022317 Google Scholar
[18]	Das D, Ghosal A, Sasmal S, Mal S, Majumdar A S 2019 Phys. Rev. A 99 022305 Google Scholar
[19]	Brown P J, Colbeck R 2020 Phys. Rev. Lett. 125 090401 Google Scholar
[20]	Zhang T G, Fei S M 2021 Phys. Rev. A 103 032216 Google Scholar
[21]	Mermin N D 1990 Phys. Rev. Lett. 65 1838 Google Scholar
[22]	Saha S, Das D, Sasmal S, Sarkar D, Mukherjee K, Roy K, Bhattacharya S S 2019 Quantum Inf. Process. 18 42 Google Scholar
[23]	Svetlichny G 1987 Phys. Rev. D 35 3066 Google Scholar
[24]	Bancal J D, Barrett J, Gisin N, Pironio S 2013 Phys. Rev. A 88 014102 Google Scholar
[25]	Xi Y, Li M S, Fu L B, Zheng Z J 2023 Phys. Rev. A 107 062419 Google Scholar
[26]	Mukherjee K, Chakrabarty I, Mylavarapu G 2023 Phys. Rev. A 107 032404 Google Scholar
[27]	Mukherjee K 2022 Phys. Rev. A 106 042206 Google Scholar
[28]	Ralston J P, Jain P, Nodland B 1998 Phys. Rev. Lett. 81 26 Google Scholar
[29]	Pearle P M 1970 Phys. Rev. D 2 1418 Google Scholar
[30]	Yang S S, Hou J C, He K 2024 Chin. Phys. B 33 010302 Google Scholar

Cited By

图 1 共享三体量子非局域场景

Figure 1. Scenario of sharing the genuine tripartite nonlocality.

DownLoad: Full-Size Img PowerPoint

map

[1]	Bell J S 1964 Phys. Phys. Fiz. 1 195 Google Scholar
[2]	Barrett J, Hardy L, Kent A 2005 Phys. Rev. Lett. 95 010503 Google Scholar
[3]	Acín A, Brunner N, Gisin N, Massar S, Pironio S, Scarani V 2007 Phys. Rev. Lett. 98 230501 Google Scholar
[4]	Li J J, Wang Y, Li H W, Bao W S 2020 Chin. Phys. B 29 030303 Google Scholar
[5]	周贤韬, 江英华 2023 72 020302 Google Scholar Zhou X T, Jiang Y H 2023 Acta Phys. Sin. 72 020302 Google Scholar
[6]	张沛, 周小清, 李智伟 2014 63 130301 Google Scholar Zhao P, Zhou X Q, Li Z W 2014 Acta Phys. Sin. 63 130301 Google Scholar
[7]	Dynes J F, Yuan Z L, Sharpe A W, Shields A J 2008 Appl. Phys. Lett. 93 031109 Google Scholar
[8]	Acín A, Masanes L 2016 Nature 540 213 Google Scholar
[9]	Curchod F J, Johansson M, Augusiak R, Hoban M J, Wittek P, Acín A 2017 Phys. Rev. A 95 020102 Google Scholar
[10]	Colbeck R, Renner R 2012 Nat. Phys. 8 450 Google Scholar
[11]	Colbeck R, Kent A 2011 J. Phys. A: Math. Theor. 44 095305 Google Scholar
[12]	李宏欣, 王相宾, 刘欣, 韩宇, 闫宝, 王伟 2017 现代物理 7 257 Google Scholar Li H X, Wang X B, Liu X, Han Y, Yan B, Wang W 2017 Modern Physics 7 257 Google Scholar
[13]	杜聪, 王金东, 秦晓娟, 魏正军, 於亚飞, 张智明 2020 69 190301 Google Scholar Du C, Wang J D, Qin X J, Wei Z J, Yu Y F, Zhang Z M 2020 Acta Phys. Sin. 69 190301 Google Scholar
[14]	东晨, 赵尚弘, 董毅, 赵卫虎, 赵静 2014 63 170303 Google Scholar Dong C, Zhao S H, Dong Y, Zhao W H, Zhao J 2014 Acta Phys. Sin. 63 170303 Google Scholar
[15]	Silva R, Gisin N, Guryanova Y, Popescu S 2015 Phys. Rev. Lett. 114 250401 Google Scholar
[16]	Mal S, Majumdar A, Home D 2016 Mathematics 4 48 Google Scholar
[17]	Shenoy H A, Designolle S, Hirsch F, Silva R, Gisin N, Brunner N 2019 Phys. Rev. A 99 022317 Google Scholar
[18]	Das D, Ghosal A, Sasmal S, Mal S, Majumdar A S 2019 Phys. Rev. A 99 022305 Google Scholar
[19]	Brown P J, Colbeck R 2020 Phys. Rev. Lett. 125 090401 Google Scholar
[20]	Zhang T G, Fei S M 2021 Phys. Rev. A 103 032216 Google Scholar
[21]	Mermin N D 1990 Phys. Rev. Lett. 65 1838 Google Scholar
[22]	Saha S, Das D, Sasmal S, Sarkar D, Mukherjee K, Roy K, Bhattacharya S S 2019 Quantum Inf. Process. 18 42 Google Scholar
[23]	Svetlichny G 1987 Phys. Rev. D 35 3066 Google Scholar
[24]	Bancal J D, Barrett J, Gisin N, Pironio S 2013 Phys. Rev. A 88 014102 Google Scholar
[25]	Xi Y, Li M S, Fu L B, Zheng Z J 2023 Phys. Rev. A 107 062419 Google Scholar
[26]	Mukherjee K, Chakrabarty I, Mylavarapu G 2023 Phys. Rev. A 107 032404 Google Scholar
[27]	Mukherjee K 2022 Phys. Rev. A 106 042206 Google Scholar
[28]	Ralston J P, Jain P, Nodland B 1998 Phys. Rev. Lett. 81 26 Google Scholar
[29]	Pearle P M 1970 Phys. Rev. D 2 1418 Google Scholar
[30]	Yang S S, Hou J C, He K 2024 Chin. Phys. B 33 010302 Google Scholar

[1]	Zhang Zhi-Da, Yi Kang-Yuan, Chen Yuan-Zhen, Yan Fei. Dynamic decoupling for multi-level systems. Acta Physica Sinica, 2023, 72(10): 100305. doi: 10.7498/aps.72.20222398
[2]	Lu Dao-Ming. Dynamics of nonlocality in an equidistance cavity coupled by fibers. Acta Physica Sinica, 2016, 65(10): 100301. doi: 10.7498/aps.65.100301
[3]	Leng Yong-Gang, Zhao Yue. Pulse response of a monostable system. Acta Physica Sinica, 2015, 64(21): 210503. doi: 10.7498/aps.64.210503
[4]	Ma Hong-Yang, Qin Guo-Qing, Fan Xing-Kui, Chu Peng-Cheng. Quantum network direct communication protocol over noisy channel. Acta Physica Sinica, 2015, 64(16): 160306. doi: 10.7498/aps.64.160306
[5]	Jin Jian-Guo, Di Zhi-Gang, Wei Ming-Jun. Potential risk of variable parameter cascade chaos system. Acta Physica Sinica, 2014, 63(12): 120509. doi: 10.7498/aps.63.120509
[6]	Tu Li-Lan, Liu Hong-Fang, Yu Le. Local adaptive H∞ consistency of delayed complex networks with noise. Acta Physica Sinica, 2013, 62(14): 140506. doi: 10.7498/aps.62.140506
[7]	Liu Hong-Mei, Yang Chun-Hua, Liu Xin, Zhang Jian-Qi, Shi Yun-Long. Noise characterization of quantum dot infrared photodetectors. Acta Physica Sinica, 2013, 62(21): 218501. doi: 10.7498/aps.62.218501
[8]	Guo Yong-Feng, Tan Jian-Guo. Suprathreshold stochastic resonance of a non-linear multilevel threshold neuronal networks system. Acta Physica Sinica, 2012, 61(17): 170502. doi: 10.7498/aps.61.170502
[9]	He Liang, Du Lei, Huang Xiao-Jun, Chen Hua, Chen Wen-Hao, Sun Peng, Han Liang. Non-Gaussian analysis of noise for metal interconnection electromigration. Acta Physica Sinica, 2012, 61(20): 206601. doi: 10.7498/aps.61.206601
[10]	Yang Lin-Jing, Dai Zu-Cheng. The effects of correlated time between noises on stability of unstable state in Logistic system. Acta Physica Sinica, 2012, 61(10): 100509. doi: 10.7498/aps.61.100509
[11]	Yang Lin-Jing. Effects of time delay on transition rate of state in an increasing process of Logistic system. Acta Physica Sinica, 2011, 60(5): 050502. doi: 10.7498/aps.60.050502
[12]	Liang Xiao-Bing, Liu Xi-Shun, Liu An-Zhi, Wang Bo-Liang. The transmission of weak signal in one-way coupled Hodgkin-Huxley neural system. Acta Physica Sinica, 2009, 58(7): 5065-5069. doi: 10.7498/aps.58.5065
[13]	Wang Bao-Hua, Lu Qi-Shao, Lü Shu-Juan. The spatio-temporal stochastic resonance of calcium in coupled hepatocytes systems affected by subthreshold stimuli and noise. Acta Physica Sinica, 2009, 58(11): 7458-7465. doi: 10.7498/aps.58.7458
[14]	Li Wei-Hua, Zhuang Yi-Qi, Du Lei, Bao Jun-Lin. Non-Gaussianity of noise in n-type metal oxide semiconductor field effect transistor. Acta Physica Sinica, 2009, 58(10): 7183-7188. doi: 10.7498/aps.58.7183
[15]	Zhou Ben-Yuan, Huang Hui, Li Gao-Xiang. Enhancement of three-mode Gaussian state light field nonlocality. Acta Physica Sinica, 2009, 58(3): 1679-1684. doi: 10.7498/aps.58.1679
[16]	. The effect of noise on the dynamical localization. Acta Physica Sinica, 2007, 56(12): 7207-7212. doi: 10.7498/aps.56.7207
[17]	Li Rui-Hong, Xu Wei, Li Shuang. Linear state feedback control for a new chaotic system. Acta Physica Sinica, 2006, 55(2): 598-604. doi: 10.7498/aps.55.598
[18]	Cheng Cheng, Zhang Hang. A semiconductor nanocrystal PbSe quantum dot fiber amplifier. Acta Physica Sinica, 2006, 55(8): 4139-4144. doi: 10.7498/aps.55.4139
[19]	Leng Yong-Gang, Wang Tai-Yong, Guo Yan, Wang Wen-Jin, Hu Shi-Guang. Stochastic resonance behaviors of bistable systems connected in series. Acta Physica Sinica, 2005, 54(3): 1118-1125. doi: 10.7498/aps.54.1118
[20]	Cheng Qing-Hua, Cao Li, Wu Da-Jin. Stochastic resonance in a single-mode laser driven by the colored pump noise with signal modulation and the quantum noise with cross-correlation between the real and imaginary parts. Acta Physica Sinica, 2004, 53(8): 2556-2562. doi: 10.7498/aps.53.2556

Catalog

Vol. 73, Issue 21 - 2024-11-05

Metrics

Abstract views: 1402
PDF Downloads: 46
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板