Stable solitons and their controllability in <inline-formula><tex-math id="M2">\begin{document}$ {\cal{PT}}$\end{document}</tex-math><alternatives><graphic xmlns:xlink="http://www.w3.org/1999/xlink" xlink:href="18-20220475_M2.jpg"/><graphic xmlns:xlink="http://www.w3.org/1999/xlink" xlink:href="18-20220475_M2.png"/></alternatives></inline-formula> symmetrical polariton condensates

Chen Li-Yuan; Gao Chao; Lin Ji; Li Hui-Jun

doi:10.7498/aps.71.20220475

Abstract

By constructing the spatial distribution of external potential and incoherent pumping, a $ {\cal{PT}} $ symmetrical model satisfied by the one-dimensional incoherent pumped exciton-polariton condensate system is designed. In the weakly nonlinear case, the $ {\cal{PT}} $ symmetrical phase transition point is found, and the linear spectrum is shown. In the normal nonlinear case, found are the bright soliton with the zero background, the multi-poles dark solitons with zero background, the symmetry breaking dark solitons and symmetrical dark soliton with the homogeneous background, and the dip- and hump-type dark solitons with the homogeneous background, and discussed are the effects of inhomogeneous pumping and the imaginary part of external potential on the profiles and the stability of solitons. Through these results, the competition between $ {\cal{PT}} $ symmetrical potential and the inhomogeneous pumping is understood, the scheme that how the bright and dark solitons are excited is presented, and the existence and stability regions of these solitons are determined. Finally, the symmetry breaking dark solitons are controlled by modulating the imaginary part of the $ {\cal{PT}} $ symmetrical potential, which reveals the potential applications of the polariton condensate system in optical information processing, such as the all-optical switches.

Keywords:

Authors and contacts

Department of Physics, Zhejiang Normal University, Jinhua 321004, China

Corresponding author: Li Hui-Jun, hjli@zjnu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 12074343, 11835011, 12074342) and the Natural Science Foundation of Zhejiang Province, China (Grant Nos. LZ22A050002, LY21A040004, LR22A040001)

FullText HTML : translate this paragraph

References

[1]	Cao H, Wiersig J 2015 Rev. Mod. Phys. 87 61 Google Scholar
[2]	Bender C M, Boettcher S 1998 Phys. Rev. Lett. 80 5243 Google Scholar
[3]	Musslimani Z H, Makris K G, El G R, Christodoulides D N 2008 Phys. Rev. Lett. 100 030402 Google Scholar
[4]	Rüter C, Makris K, El G R 2010 Nat. Phys. 6 192 Google Scholar
[5]	Regensburger A, Bersch C, Miri M A, Onishchukov G, Christodoulides D N, Peschel U 2012 Nature 488 167 Google Scholar
[6]	Guo A, Salamo G J, Duchesne D, Morandotti R, Volatier R M, Aimez V, Sililoglou G A, Christodoulides D N 2009 Phys. Rev. Lett. 103 093902 Google Scholar
[7]	Feng L, Wong Z J, Ma R, Wang Y, Zhang X 2014 Science 346 972 Google Scholar
[8]	Hodaei H, Miri M, Heinrich M, Christodoulides D N, Khajavikhan M 2014 Science 346 975 Google Scholar
[9]	Regensburger A, Miri M, Bersch C, Näger J, Onishchukov G, Christodoulides D N, Peschel U 2013 Phys. Rev. Lett. 110 223902 Google Scholar
[10]	Peng B, Özdemir S K, Lei F, Monifi F, Gianfreda M, Long G L, Fan S, Nori F, Bender C M, Yang L 2014 Nat. Phys. 10 394 Google Scholar
[11]	Chang L, Jiang X, Hua S, Yang C, Wen J, Jiang L, Li G, Wang G, Xiao M 2014 Nat. Photonics 8 524 Google Scholar
[12]	Ramezani H, Schindler J, Ellis F M, Gunther U, Kottos T 2012 Phys. Rev. A 85 062122 Google Scholar
[13]	Lin Z, Schindler J, Ellis F M, Kottos T 2012 Phys. Rev. A 85 050101 Google Scholar
[14]	Feng L, Xu Y L, Fegadolli W S, Lu M H, Oliveira J E B, Almeida V R, Chen Y F, Scherer A 2013 Nat. Mater. 12 108 Google Scholar
[15]	Sun Y, Tan W, Li H, Li J, Chen H 2014 Phys. Rev. Lett. 112 143903 Google Scholar
[16]	Li J, Harter A K, Liu J, Melo L, Joglekar Y N, Luo L 2019 Nat. Commun. 10 1 Google Scholar
[17]	Hang C, Huang G, Konotop V V 2013 Phys. Rev. Lett. 110 083604 Google Scholar
[18]	Sheng J, Miri M, Christodoulides D N, Xiao M 2013 Phys. Rev. A 88 041803 Google Scholar
[19]	Li H, Dou J, Huang G 2013 Opt. Express 21 32053 Google Scholar
[20]	Wu J, Artoni M, Rocca G 2014 Phys. Rev. Lett. 113 123004 Google Scholar
[21]	Zhang Z, Zhang Y, Sheng J, Yang L, Miri M, Christodoulides D N, He B, Zhang Y, Xiao M 2016 Phys. Rev. Lett. 117 123601 Google Scholar
[22]	Makris K G, El-Ganainy R, Christodoulides D N, Musslimani Z H 2008 Phys. Rev. Lett. 100 103904 Google Scholar
[23]	Sieberer L M, Buchhold M, Diehl S 2016 Rep. Prog. Phys. 79 096001 Google Scholar
[24]	Wouters M, Carusotto I 2007 Phys. Rev. Lett. 99 140402 Google Scholar
[25]	Szymanska M H, Keeling J, Littlewood P B 2006 Phys. Rev. Lett. 96 230602 Google Scholar
[26]	Byrnes T, Kim N Y, Yamamoto Y 2014 Nat. Phys. 10 803 Google Scholar
[27]	Anton C, Liew T C H, Cuadra J, Martin M D, Eldridge P S, Hatzopoulos Z, Stavrinidis G, Savvidis P G, Vina L 2013 Phys. Rev. B 88 245307 Google Scholar
[28]	Smirnov L A, Smirnova D A, Ostrovskaya E A, Kivshar Y S 2014 Phys. Rev. B 89 235310 Google Scholar
[29]	Xue Y, Matuszewski M 2014 Phys. Rev. Lett. 112 216401 Google Scholar
[30]	Liew T C H, Egorov O A, Matuszewski M, Kyriienko O, Ma X, Ostrovskaya E A 2015 Phys. Rev. B 91 085413 Google Scholar
[31]	Kol G R 2017 Opt. Quantum Electron. 49 385 Google Scholar
[32]	Cheng S, Chen T 2018 Phys. Rev. E 97 032212 Google Scholar
[33]	Yoon S, Sun M, Rubo Y G, Savenko I G 2019 Phys. Rev. A 100 023609 Google Scholar
[34]	Opala A, Pieczarka M, Bobrovska N, Matuszewski M 2018 Phys. Rev. B 97 155304 Google Scholar
[35]	Xue Y, Jiang Y, Wang G, Wang R, Feng S, Matuszewski M 2018 Opt. Express 26 6267 Google Scholar
[36]	Sigurdsson H, Liew T C H, Shelykh I A 2017 Phys. Rev. B 96 205406 Google Scholar
[37]	Ma X, Egorov O A, Schumacher S 2017 Phys. Rev. Lett. 118 157401 Google Scholar
[38]	Pinsker F, Flayac H 2016 Proc. R. Soc. A 472 20150592 Google Scholar
[39]	Kulczykowski M, Bobrovska N, Matuszewski M 2015 Phys. Rev. B 91 245310 Google Scholar
[40]	Ma X, Schumacher S 2017 Phys. Rev. B 95 235301 Google Scholar
[41]	Ostrovskaya E A, Abdullaev J, Desyatnikov A S, Fraser M D, Kivshar Y S 2012 Phys. Rev. A 86 013636 Google Scholar
[42]	Tanese D, Flayac H, Solnyshkov D, Amo A, Lemaître A, Galopin E, Braive R, Senellart P, Sagnes I, Malpuech G, Bloch J 2013 Nat. Commun. 4 1749 Google Scholar
[43]	Ostrovskaya E A, Abdullaev J, Fraser M D, Desyatnikov A S, Kivshar Y S 2013 Phys. Rev. Lett. 110 170407 Google Scholar
[44]	Pinsker F, Flayac H 2014 Phys. Rev. Lett. 112 140405 Google Scholar
[45]	Lien J, Chen Y, Ishida N, Chen H, Hwang C, Nori F 2015 Phys. Rev. B 91 024511 Google Scholar
[46]	Chestnov I Y, Demirchyan S S, Alodjants A P, Rubo Y G, Kavokin A V 2016 Sci. Rep. 6 19551 Google Scholar
[47]	Ma X K, Kartashov Y Y, Gao T, Schumacher S 2019 New J. Phys. 21 123008 Google Scholar
[48]	Jia C Y, Liang Z X 2020 Chin. Phys. Lett. 37 040502 Google Scholar
[49]	Zhang K, Wen W, Lin J, Li H 2021 New J. Phys. 23 033011 Google Scholar
[50]	Yang J 2011 Nonlinear Waves in Integrable and Nonintegrable Systems (Philadephia: PA: SIAM) p327
[51]	Carusotto I, Ciuti C 2013 Rev. Mod. Phys. 85 299 Google Scholar
[52]	Zhang K, Liang Y, Lin J, Li H 2018 Phys. Rev. A 97 023844 Google Scholar
[53]	Suchkov S V, Sukhorukov A A, Huang J H 2016 Laser Photonics Rev. 10 177 Google Scholar
[54]	Konotop V V, Yang J K, Zezyulin D A 2016 Rev. Mod. Phys. 88 035002 Google Scholar
[55]	Ozdemir S K, Rotter S, Nori F, Yang L 2019 Nat. Mater. 18 783 Google Scholar
[56]	Rodislav D, Boris A M 2011 Opt. Lett. 36 4323 Google Scholar
[57]	Yang J 2017 J. Opt. 19 054004 Google Scholar
[58]	Akhmediev N, Ankiewicz A 2005 Dissipative Solitons (Berlin: Springer) pp1–17
[59]	Bludov Y V, Hang C, Huang G, Konotop V V 2014 Opt. Lett. 39 3382 Google Scholar
[60]	Nixon S, Zhu Y, Yang J 2012 Opt. Lett. 37 4874 Google Scholar
[61]	党婷婷, 王娟芬, 安亚东, 刘香莲, 张朝霞, 杨玲珍 2015 64 064211 Google Scholar Dang T T, Wang J F, An Y D, Liu X L, Zhang Z X, Yang L Z 2015 Acta Phys. Sin. 64 064211 Google Scholar

Cited By

图 1 弱非线性激发的线性谱　(a) 参数取$ w=0.45 $, $ \xi_0=1 $, $ W=1 $时$ {\cal{PT}} $对称外势的轮廓图; (b) 线性谱中的虚部最大值随W的变化曲线; (c) $ W=0.1 $和(d) $ W=0.9 $时的线性谱以及离散本征值对应本征函数的虚实部

Figure 1. Linear spectrum of weakly nonlinear excitations: (a) Profile of $ {\cal{PT}} $ symmetrical potential. Here, $ w=0.45 $, $ \xi_0=1 $, and $ W=1 $; (b) Im$(\beta)_{{\rm{max}}}$ as a function of W; (c), (d) linear spectrum for $ W=0.1 $, $ W=0.9 $ and the discrete eigenvalues corresponding to the imaginary and real parts of the eigenfunction, respectively

DownLoad: Full-Size Img PowerPoint

图 2 非均匀泵浦强度$ \sigma_8 < 0 $时的暗孤子解　(a)—(c)分别为暗孤子的功率、稳定性和增益耗散强度随$ \sigma_8 $ 的变化曲线; (d1)—(g1)是取图(a)—(c)中字母d—g相应的W和$ \sigma_8 $时孤子的轮廓$ |\psi| $(红实线) 以及相位ϕ (蓝色点虚线)的图像; (d2)—(g2)是孤子演化的结果, 图中, 左侧是演化结果的投影, 右侧是特定时刻s的演化结果

Figure 2. Dark solitons for inhomogeneous pumping $ \sigma_8 < 0 $: (a)–(c) Power, stability and total gain loss intensity curves of the dark solitons as a function of $ \sigma_8 $, respectively; (d1)–(g1) profiles of the dark solitons with different $ \sigma_8 $ and W marked by the letter d–g in the panels (a)–(c) respectively, the red solid line (blue dashed-dotted line) denotes the profile $ |\psi| $ (phase ϕ); (d2)–(g2) projections and profiles of the evolution results. In the left panels, the projections of the evolution are shown. The profiles and phases of evolution at the special time s marked by the green line of the left panels are shown in the right panels

DownLoad: Full-Size Img PowerPoint

图 3 均匀泵浦情况下的暗孤子　(a)—(c)分别为孤子的功率、稳定性和增益耗散强度随W的变化曲线; (d1)—(f1)是取图(a)—(c)中字母d—f相应的W和$ \sigma_8 $时孤子的轮廓$ |\psi| $(红实线) 和相位ϕ(蓝色点虚线) 的图像; (d2)—(f2)是孤子演化的结果, 图中, 左侧是演化结果的投影, 右侧是特定时刻s的演化结果

Figure 3. Dark solitons for homogeneous pumping: (a)–(c) Power, stability and total gain loss intensity curves of the dark soliton as a function of W; (d1)–(f1) profiles of the dark soliton marked by the letter d–f in the panels (a)–(c) respectively, the red solid line (blue dashed-dotted line) denote the amplitude $ |\psi| $ (phase ϕ); (d2)–(f2) projections and profiles of the evolution results. In the left panels, the projections of the evolution are shown. The profiles and phases of evolution at the special time s marked by the green line of the left panels are shown in the right panels.

DownLoad: Full-Size Img PowerPoint

图 4 非均匀泵浦$ \sigma_8 > 0 $时的亮孤子　(a)—(c)分别为亮孤子的功率、稳定性和增益损耗强度随$ \sigma_8 $的变化曲线; (d1)—(g1)是取图(a)—(c)中字母d—g相应的W和$ \sigma_8 $时孤子的轮廓; (d2)—(g2) 是孤子演化的结果, 图中, 左侧是演化结果的投影, 右侧是特定时刻s的演化结果

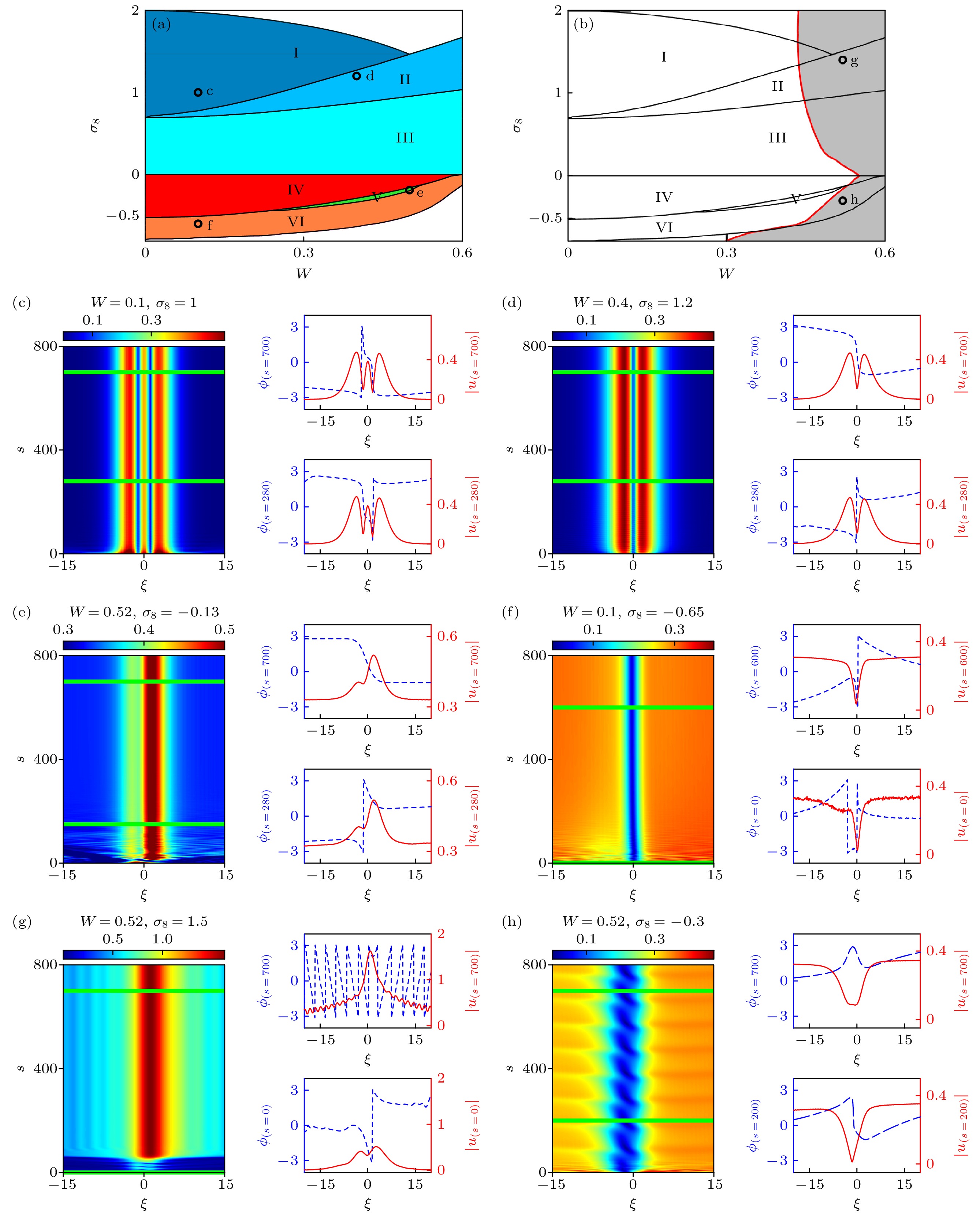
Figure 4. Bright solitons for inhomogeneous pumping $ \sigma_8 > 0 $: (a)–(c) Power, stability, and total gain loss intensity curves of the bright soliton as a function of $ \sigma_8 $; (d1)–(g1) profiles of the bright solitons marked by the letter d–g in the panels (a)–(c) respectively; (d2)–(g2) projections and profiles of the evolution results. In the left panel, the projections of the evolution results are shown. The profiles of evolution at the special times s marked by the green line of the left panels are shown in the right panels

DownLoad: Full-Size Img PowerPoint

图 5 孤子类型与孤子稳定区域分布图　(a) 7种孤子在参数W与$ \sigma_8 $区域的分布图; (b) 稳定孤子在参数W与$ \sigma_8 $区域的分布图; (c)—(h) 图 (a), (b) 中字母c—h相应的不同参数所对应孤子的演化结果, 图中, 左侧是演化结果的投影, 右侧是特定时刻s孤子轮廓和相位的演化结果

Figure 5. Phase diagram for soliton types and stability: (a) Phase diagram of seven types of solitons as the functions of W and $ \sigma_8 $; (b) phase diagram of stability regions for seven types of solitons; (c)–(h) projections and profiles of the evolution results of solitons marked by the letter c–h in the panels (a) and (b) respectively. In the left panels, the projections of the evolution are shown. The profiles and phases of evolution at the special time s marked by the green line of the left panels are shown in the right panels

DownLoad: Full-Size Img PowerPoint

图 6 调控外势虚部时对称破缺孤子的演化结果　(a) $ W= $$ 0.4, \;\sigma_8=-0.5 $时暗孤子的演化结果; (b) $ W=0.52, \; \sigma_8= $$ -0.13 $时暗孤子的演化结果; (c) $\xi_0 = 3, \;W = 0.1, \;\sigma_8 = -0.5$时暗孤子的演化结果

Figure 6. Evolution results of symmetry breaking solitons by controlling the imaginary part of $ {\cal{PT}} $ potential: (a) The evolution results of dark soliton with $ W=0.4, \;\sigma_8=-0.5 $; (b) the evolution results of dark soliton with $ W=0.52, $$ \sigma_8=-0.13 $; (c) the evolution results of dark soliton with $ \xi_0=3, \; W=0.1,\; \sigma_8=-0.5 $

DownLoad: Full-Size Img PowerPoint

map

[1]	Cao H, Wiersig J 2015 Rev. Mod. Phys. 87 61 Google Scholar
[2]	Bender C M, Boettcher S 1998 Phys. Rev. Lett. 80 5243 Google Scholar
[3]	Musslimani Z H, Makris K G, El G R, Christodoulides D N 2008 Phys. Rev. Lett. 100 030402 Google Scholar
[4]	Rüter C, Makris K, El G R 2010 Nat. Phys. 6 192 Google Scholar
[5]	Regensburger A, Bersch C, Miri M A, Onishchukov G, Christodoulides D N, Peschel U 2012 Nature 488 167 Google Scholar
[6]	Guo A, Salamo G J, Duchesne D, Morandotti R, Volatier R M, Aimez V, Sililoglou G A, Christodoulides D N 2009 Phys. Rev. Lett. 103 093902 Google Scholar
[7]	Feng L, Wong Z J, Ma R, Wang Y, Zhang X 2014 Science 346 972 Google Scholar
[8]	Hodaei H, Miri M, Heinrich M, Christodoulides D N, Khajavikhan M 2014 Science 346 975 Google Scholar
[9]	Regensburger A, Miri M, Bersch C, Näger J, Onishchukov G, Christodoulides D N, Peschel U 2013 Phys. Rev. Lett. 110 223902 Google Scholar
[10]	Peng B, Özdemir S K, Lei F, Monifi F, Gianfreda M, Long G L, Fan S, Nori F, Bender C M, Yang L 2014 Nat. Phys. 10 394 Google Scholar
[11]	Chang L, Jiang X, Hua S, Yang C, Wen J, Jiang L, Li G, Wang G, Xiao M 2014 Nat. Photonics 8 524 Google Scholar
[12]	Ramezani H, Schindler J, Ellis F M, Gunther U, Kottos T 2012 Phys. Rev. A 85 062122 Google Scholar
[13]	Lin Z, Schindler J, Ellis F M, Kottos T 2012 Phys. Rev. A 85 050101 Google Scholar
[14]	Feng L, Xu Y L, Fegadolli W S, Lu M H, Oliveira J E B, Almeida V R, Chen Y F, Scherer A 2013 Nat. Mater. 12 108 Google Scholar
[15]	Sun Y, Tan W, Li H, Li J, Chen H 2014 Phys. Rev. Lett. 112 143903 Google Scholar
[16]	Li J, Harter A K, Liu J, Melo L, Joglekar Y N, Luo L 2019 Nat. Commun. 10 1 Google Scholar
[17]	Hang C, Huang G, Konotop V V 2013 Phys. Rev. Lett. 110 083604 Google Scholar
[18]	Sheng J, Miri M, Christodoulides D N, Xiao M 2013 Phys. Rev. A 88 041803 Google Scholar
[19]	Li H, Dou J, Huang G 2013 Opt. Express 21 32053 Google Scholar
[20]	Wu J, Artoni M, Rocca G 2014 Phys. Rev. Lett. 113 123004 Google Scholar
[21]	Zhang Z, Zhang Y, Sheng J, Yang L, Miri M, Christodoulides D N, He B, Zhang Y, Xiao M 2016 Phys. Rev. Lett. 117 123601 Google Scholar
[22]	Makris K G, El-Ganainy R, Christodoulides D N, Musslimani Z H 2008 Phys. Rev. Lett. 100 103904 Google Scholar
[23]	Sieberer L M, Buchhold M, Diehl S 2016 Rep. Prog. Phys. 79 096001 Google Scholar
[24]	Wouters M, Carusotto I 2007 Phys. Rev. Lett. 99 140402 Google Scholar
[25]	Szymanska M H, Keeling J, Littlewood P B 2006 Phys. Rev. Lett. 96 230602 Google Scholar
[26]	Byrnes T, Kim N Y, Yamamoto Y 2014 Nat. Phys. 10 803 Google Scholar
[27]	Anton C, Liew T C H, Cuadra J, Martin M D, Eldridge P S, Hatzopoulos Z, Stavrinidis G, Savvidis P G, Vina L 2013 Phys. Rev. B 88 245307 Google Scholar
[28]	Smirnov L A, Smirnova D A, Ostrovskaya E A, Kivshar Y S 2014 Phys. Rev. B 89 235310 Google Scholar
[29]	Xue Y, Matuszewski M 2014 Phys. Rev. Lett. 112 216401 Google Scholar
[30]	Liew T C H, Egorov O A, Matuszewski M, Kyriienko O, Ma X, Ostrovskaya E A 2015 Phys. Rev. B 91 085413 Google Scholar
[31]	Kol G R 2017 Opt. Quantum Electron. 49 385 Google Scholar
[32]	Cheng S, Chen T 2018 Phys. Rev. E 97 032212 Google Scholar
[33]	Yoon S, Sun M, Rubo Y G, Savenko I G 2019 Phys. Rev. A 100 023609 Google Scholar
[34]	Opala A, Pieczarka M, Bobrovska N, Matuszewski M 2018 Phys. Rev. B 97 155304 Google Scholar
[35]	Xue Y, Jiang Y, Wang G, Wang R, Feng S, Matuszewski M 2018 Opt. Express 26 6267 Google Scholar
[36]	Sigurdsson H, Liew T C H, Shelykh I A 2017 Phys. Rev. B 96 205406 Google Scholar
[37]	Ma X, Egorov O A, Schumacher S 2017 Phys. Rev. Lett. 118 157401 Google Scholar
[38]	Pinsker F, Flayac H 2016 Proc. R. Soc. A 472 20150592 Google Scholar
[39]	Kulczykowski M, Bobrovska N, Matuszewski M 2015 Phys. Rev. B 91 245310 Google Scholar
[40]	Ma X, Schumacher S 2017 Phys. Rev. B 95 235301 Google Scholar
[41]	Ostrovskaya E A, Abdullaev J, Desyatnikov A S, Fraser M D, Kivshar Y S 2012 Phys. Rev. A 86 013636 Google Scholar
[42]	Tanese D, Flayac H, Solnyshkov D, Amo A, Lemaître A, Galopin E, Braive R, Senellart P, Sagnes I, Malpuech G, Bloch J 2013 Nat. Commun. 4 1749 Google Scholar
[43]	Ostrovskaya E A, Abdullaev J, Fraser M D, Desyatnikov A S, Kivshar Y S 2013 Phys. Rev. Lett. 110 170407 Google Scholar
[44]	Pinsker F, Flayac H 2014 Phys. Rev. Lett. 112 140405 Google Scholar
[45]	Lien J, Chen Y, Ishida N, Chen H, Hwang C, Nori F 2015 Phys. Rev. B 91 024511 Google Scholar
[46]	Chestnov I Y, Demirchyan S S, Alodjants A P, Rubo Y G, Kavokin A V 2016 Sci. Rep. 6 19551 Google Scholar
[47]	Ma X K, Kartashov Y Y, Gao T, Schumacher S 2019 New J. Phys. 21 123008 Google Scholar
[48]	Jia C Y, Liang Z X 2020 Chin. Phys. Lett. 37 040502 Google Scholar
[49]	Zhang K, Wen W, Lin J, Li H 2021 New J. Phys. 23 033011 Google Scholar
[50]	Yang J 2011 Nonlinear Waves in Integrable and Nonintegrable Systems (Philadephia: PA: SIAM) p327
[51]	Carusotto I, Ciuti C 2013 Rev. Mod. Phys. 85 299 Google Scholar
[52]	Zhang K, Liang Y, Lin J, Li H 2018 Phys. Rev. A 97 023844 Google Scholar
[53]	Suchkov S V, Sukhorukov A A, Huang J H 2016 Laser Photonics Rev. 10 177 Google Scholar
[54]	Konotop V V, Yang J K, Zezyulin D A 2016 Rev. Mod. Phys. 88 035002 Google Scholar
[55]	Ozdemir S K, Rotter S, Nori F, Yang L 2019 Nat. Mater. 18 783 Google Scholar
[56]	Rodislav D, Boris A M 2011 Opt. Lett. 36 4323 Google Scholar
[57]	Yang J 2017 J. Opt. 19 054004 Google Scholar
[58]	Akhmediev N, Ankiewicz A 2005 Dissipative Solitons (Berlin: Springer) pp1–17
[59]	Bludov Y V, Hang C, Huang G, Konotop V V 2014 Opt. Lett. 39 3382 Google Scholar
[60]	Nixon S, Zhu Y, Yang J 2012 Opt. Lett. 37 4874 Google Scholar
[61]	党婷婷, 王娟芬, 安亚东, 刘香莲, 张朝霞, 杨玲珍 2015 64 064211 Google Scholar Dang T T, Wang J F, An Y D, Liu X L, Zhang Z X, Yang L Z 2015 Acta Phys. Sin. 64 064211 Google Scholar

[1]	Wang Jin-Ling, Zhang Kun, Lin Ji, Li Hui-Jun. Generation and modulation of shock waves in two-dimensional polariton condensates. Acta Physica Sinica, 2024, 73(11): 119601. doi: 10.7498/aps.73.20240229
[2]	Li Xin-Yue, Qi Juan-Juan, Zhao Dun, Liu Wu-Ming. Soliton solutions of the spin-orbit coupled binary Bose-Einstein condensate system. Acta Physica Sinica, 2023, 72(10): 106701. doi: 10.7498/aps.72.20222319
[3]	Jia Rui-Yu, Fang Ping-Ping, Gao Chao, Lin Ji. Quenched solitons and shock waves in Bose-Einstein condensates. Acta Physica Sinica, 2021, 70(18): 180303. doi: 10.7498/aps.70.20210564
[4]	Wen Lin, Liang Yi, Zhou Jing, Yu Peng, Xia Lei, Niu Lian-Bin, Zhang Xiao-Fei. Effects of linear Zeeman splitting on the dynamics of bright solitons in spin-orbit coupled Bose-Einstein condensates. Acta Physica Sinica, 2019, 68(8): 080301. doi: 10.7498/aps.68.20182013
[5]	Liu Hao-Hua, Wang Shao-Hua, Li Bo-Bo, Li Hua-Lin. Defect induced asymmetric soliton transmission in the nonlinear circuit. Acta Physica Sinica, 2017, 66(10): 100502. doi: 10.7498/aps.66.100502
[6]	He Zhang-Ming, Zhang Zhi-Qiang. Controlling interactions between bright solitons in Bose-Einstein condensate. Acta Physica Sinica, 2016, 65(11): 110502. doi: 10.7498/aps.65.110502
[7]	Qiao Hai-Long, Jia Wei-Guo, Liu Bao-Lin, Wang Xu-Dong, Menke Neimule, Yang Jun, Zhang Jun-Ping. Effect of Ramam gain on the characteristic of soliton propagation. Acta Physica Sinica, 2013, 62(10): 104212. doi: 10.7498/aps.62.104212
[8]	Zhang Heng, Duan Wen-Shan. The modulational instability of the solition wave in two-dimensional Bose-Einstein condensates. Acta Physica Sinica, 2013, 62(4): 044703. doi: 10.7498/aps.62.044703
[9]	Lu Da-Quan, Hu Wei. Two-dimensional asynchronous fractional Fourier transform and propagation properties of beams in strongly nonlocal nonlinear medium with an elliptically symmetric response. Acta Physica Sinica, 2013, 62(8): 084211. doi: 10.7498/aps.62.084211
[10]	Lu Da-Quan, Hu Wei. Breather for weak beam induced by strong beam in strongly nonlocal nonlinear medium. Acta Physica Sinica, 2013, 62(3): 034205. doi: 10.7498/aps.62.034205
[11]	Yuan Zong-Qiang, Chu Min, Zheng Zhi-Gang. Energy carriers investigation in the Fermi-Pasta-Ulam β lattice. Acta Physica Sinica, 2013, 62(8): 080504. doi: 10.7498/aps.62.080504
[12]	Zhang Bo, Wang Deng-Long, She Yan-Chao, Zhang Wei-Xi. Soliton dynamical behavior of the condensates trapped in a square-well potential. Acta Physica Sinica, 2013, 62(11): 110501. doi: 10.7498/aps.62.110501
[13]	Yi Ding, Qin Wei, Xie Shi-Jie. Investigation of polarons in perovskite manganites. Acta Physica Sinica, 2012, 61(20): 207101. doi: 10.7498/aps.61.207101
[14]	Tao Feng, Chen Wei-Zhong, Xu Wen, Du Si-Dan. The study of asymmetric energy transmission based on the nonlinear supratransmission. Acta Physica Sinica, 2012, 61(13): 134103. doi: 10.7498/aps.61.134103
[15]	Liu Hai-Lan, Wen Shuang-Chun, Xiong Min, Dai Xiao-Yu. Formation and propagation of dark solitons in metamaterials. Acta Physica Sinica, 2007, 56(11): 6473-6479. doi: 10.7498/aps.56.6473
[16]	He Zhang-Ming, Wang Deng-Long. Evolvement between bright and dark soliton of condensates. Acta Physica Sinica, 2007, 56(6): 3088-3091. doi: 10.7498/aps.56.3088
[17]	Xu Quan, Tian Qiang. The interaction of excitons with phonons and solution of breathers in one-dimensional molecular chain. Acta Physica Sinica, 2004, 53(9): 2811-2815. doi: 10.7498/aps.53.2811
[18]	Xu Yan, Xue De-Sheng, Zuo Wei, Li Fa-Shen. Nonlinear surface spin waves on ferromagnetic media with inhomogeneous exchange anisotropy. Acta Physica Sinica, 2003, 52(11): 2896-2900. doi: 10.7498/aps.52.2896
[19]	Hong Xue-Ren, Duan Wen-Shan, Sun Jian-An, Shi Yu-Ren, Lü Ke-Pu. The propagation of solitons in an inhomogeneous dusty plasma. Acta Physica Sinica, 2003, 52(11): 2671-2677. doi: 10.7498/aps.52.2671
[20]	Wang Xiao-Sheng, She Wei-Long. . Acta Physica Sinica, 2002, 51(3): 573-577. doi: 10.7498/aps.51.573

Catalog

Vol. 71, Issue 18 - 2022-09-20

Metrics

Abstract views: 3489
PDF Downloads: 131
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板

Stable solitons and their controllability in $ {\cal{PT}}$ symmetrical polariton condensates

Abstract

Authors and contacts

Corresponding author: Li Hui-Jun, hjli@zjnu.cn

FullText HTML : translate this paragraph

References

Cited By

Catalog

Metrics

Authors

Referees

About Journal

About

Search

Article

留言板

Stable solitons and their controllability in $ {\cal{PT}}$ symmetrical polariton condensates

Abstract

Authors and contacts

Corresponding author: Li Hui-Jun, hjli@zjnu.cn

FullText HTML : translate this paragraph

References

Cited By

Catalog

Metrics

Publishing process

Authors

Referees

About Journal

About