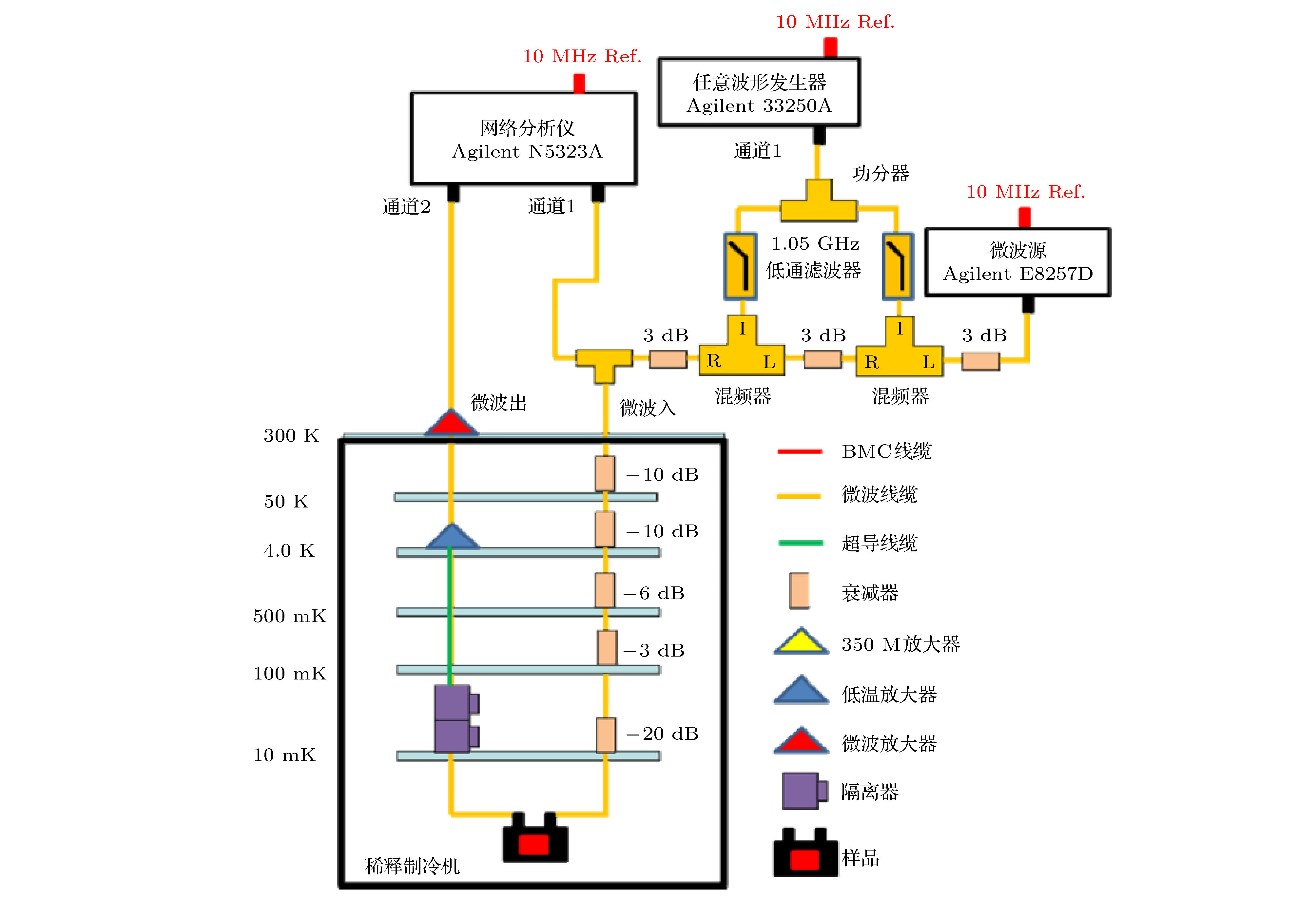
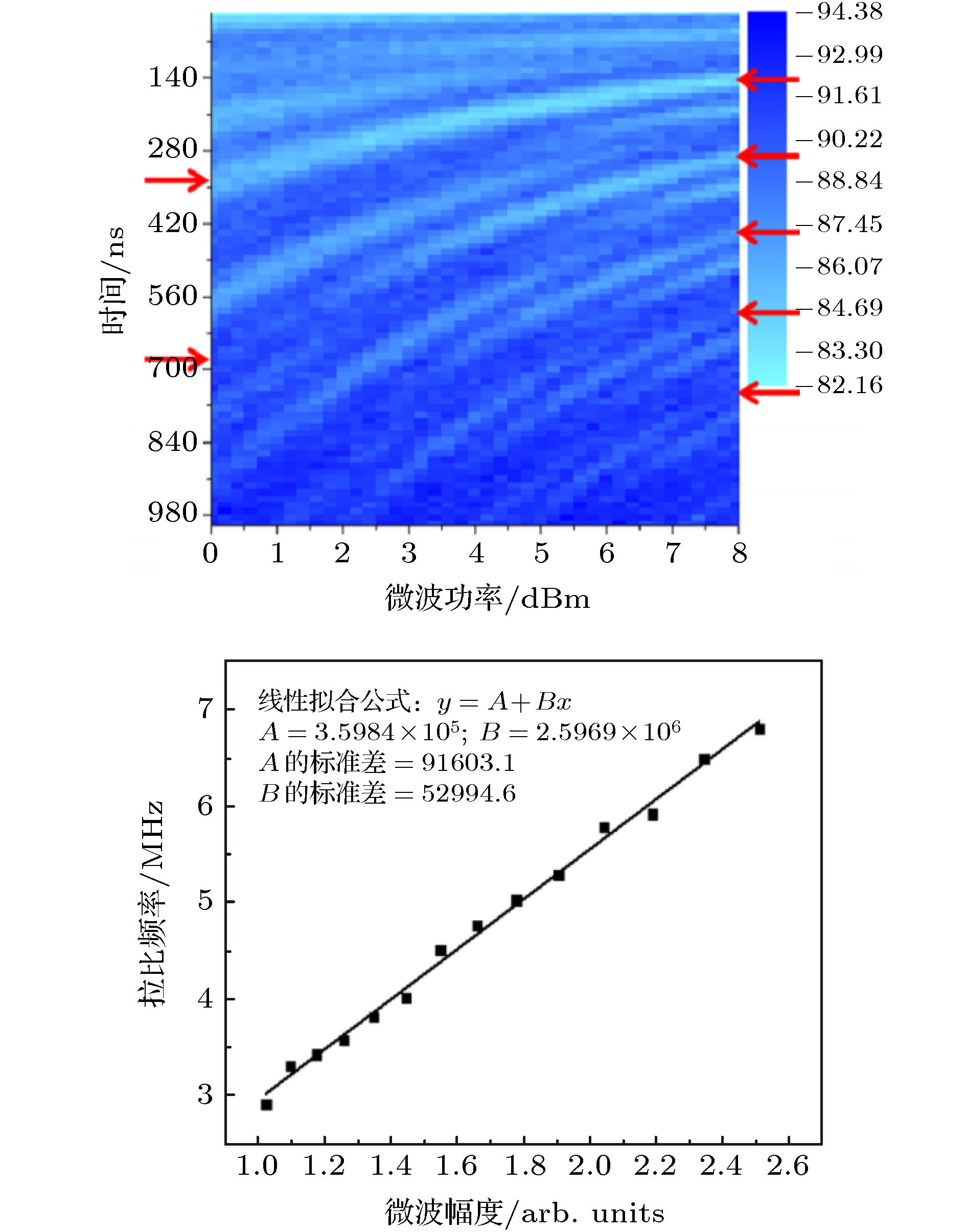
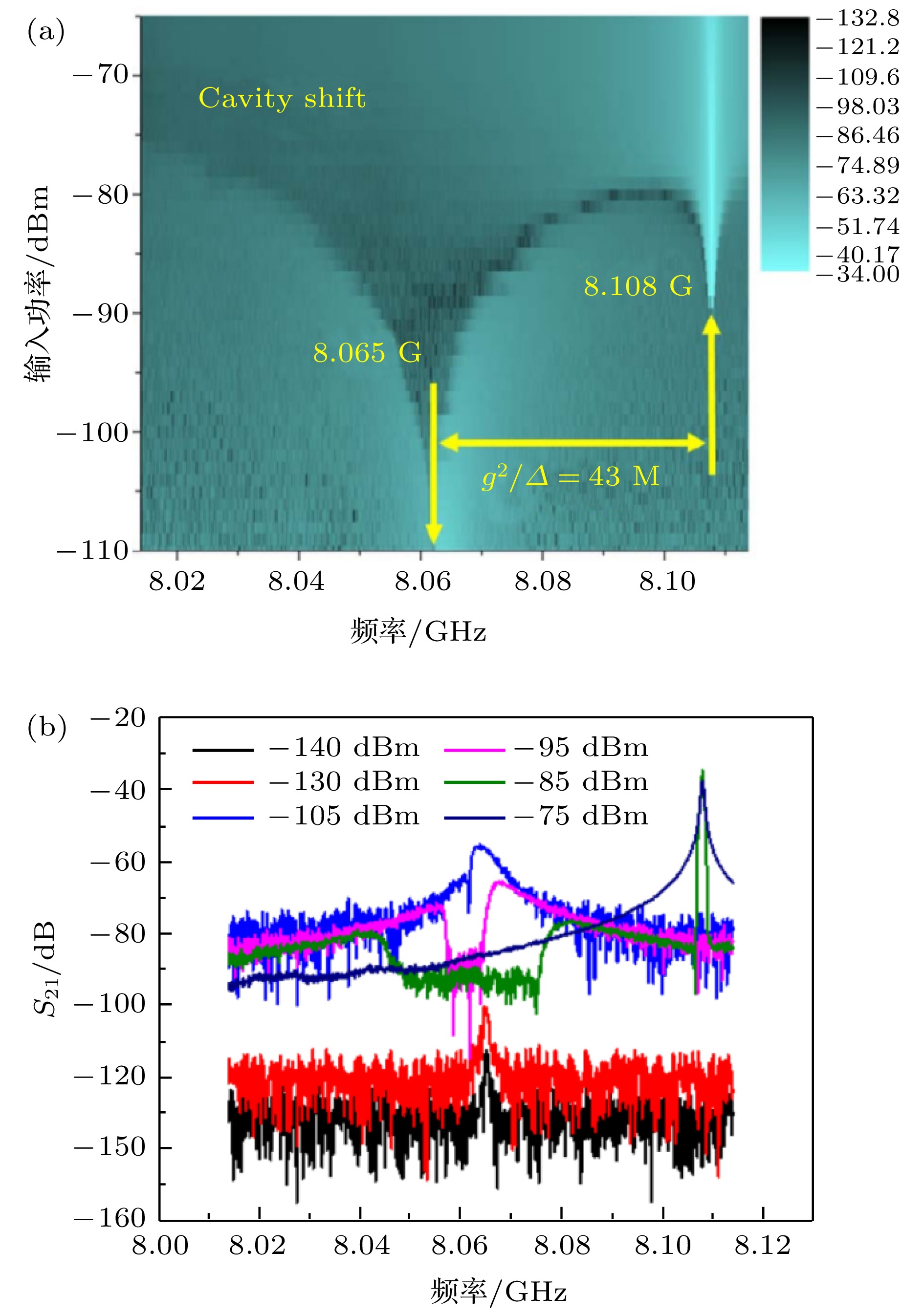
-
Three-dimensional(3D) transmon is a kind of superconducting qubit with long decoherence time, which has important applications in superconducting quantum computation, quantum optics, cavity quantum electrodynamics, et al. Rabi oscillation is a vital method to characterize the decoherence time of quantum system, and it is also a basic experiment to demonstrate the energy level evolution of quantum system. In order to test the Rabi oscillation of 3D transmon, strict timing control is necessary, and the process of testing and debugging is complicated. In this paper, 3D transmon samples are fabricated and their basic parameters EC = 348.74 MHz and EJ = 11.556 GHz are tested and characterized. The coupling coefficient g2/Δ between qubit and the 3D cavity is 43 MHz, which is located in the dispersive regime. The qubit’s first transition frequency f01 = 9.2709 GHz, and the second transition frequency f12 = 9.0100 GHz. The 3D resonator is fabricated by the material 6061T6 aluminum, the loaded quality factor is 4.8 × 105, and the bare frequency of the resonator is 8.108 GHz. Through comparison, it is found that the Rabi oscillation time obtained by the proposed method is shorter than by the Jaynes-Cummings method. The main reasons are as follows. First, the measurement of network analyzer is a continuous measurement, and the test signal always affects the decoherence process of 3D transmon. Second, the quantum bit is in the ground state after decoherence, and the ground state measured by the network analyzer accounts for a relatively high proportion, which causes the curve measured by the network analyzer to be one-sided attenuation oscillation. Third, the dispersive readout method is related to the quality factor of the superconducting cavity. The storage time of microwave photons in the superconducting cavity is longer than the decoherence time of 3D transmon, so the quantum information is partially decohered before leaving the superconducting cavity, which will shorten the Rabi oscillation time. An innovative Rabi oscillation test method based on network analyzer is presented. The test system based on this method is simple to build and can be used as a new way to quickly verify whether 3D transmon has quantum characteristics. This method can also be extended to other quantum systems for preliminarily verifying the time domain characteristics. -
Keywords:
- 3D Transmon /
- network analyzer /
- dispersive measurement /
- Rabi oscillation
[1] You J Q, Nori F 2005 Phys. Today 58 42
[2] You J Q, Nori F 2011 Nature 474 589
Google Scholar
[3] Krantz P, Kjaergaard M, Yan F, et al. 2019 Appl. Phys. Rev. 6 021318
Google Scholar
[4] 赵虎, 李铁夫, 刘建设, 陈炜 2012 61 154214
Google Scholar
Zhao H, Li T F, Liu J S, Chen W 2012 Acta Phys. Sin. 61 154214
Google Scholar
[5] Neill C, Roushan P, Kechedzhi K, et al. 2018 Science 360 6385
[6] Klimov P V, Kelly J, Chen J, et al. 2018 Phys. Rev. Lett. 121 090502
Google Scholar
[7] Blais A, Huang R S, Wallraff A, et al. 2004 Phys. Rev. A 69 666
[8] Bunyk P I, Hoskinson E M, Johnson M W, et al. 2014 IEEE Trans. Appl. Supercond. 24 4
Google Scholar
[9] Mooij J E, Orlando T P, Leviotv L, et al. 1999 Science 285 1036
Google Scholar
[10] Nakamura Y, Pashkin Y A, Tsai J S 1999 Nature 398 786
Google Scholar
[11] Friedman J R, Patel V, Chen W, et al. 2000 Nature 406 43
Google Scholar
[12] Martinis J M, Nam S, Aumentado J, Urbina C 2002 Phys. Rev. Lett. 89 117901
Google Scholar
[13] Wallraff A, Schuster D I, Blais A, et al. 2004 Nature 431 162
Google Scholar
[14] Paik H, Schuster D I, Bishop L S, et al. 2011 Phys. Rev. Lett. 107 240501
Google Scholar
[15] Chiorescu I, Nakamura Y, Harmans C J P M, et al. 2003 Science 299 1869
Google Scholar
[16] Vion D, Aassime A, Cottet A, et al. 2002 Science 296 886
Google Scholar
[17] Barends R, Kelly J, Megrant A, et al. 2013 Phys. Rev. Lett. 111 080502
Google Scholar
[18] Yan F, Gustavsson S, Kamal A, et al. 2016 Nat. Commun. 7 12964
Google Scholar
[19] You J Q, Hu X, Ashhab S, et al. 2006 Phys. Rev. B 75 140551
[20] Wallraff A, Schuster D I, Blais A, et al. 2005 Phys. Rev. Lett. 95 060501
Google Scholar
[21] Reed M D, DiCarlo L, Johnson B R, et al. 2010 Phys. Rev. Lett. 105 173601
Google Scholar
[22] Koch J, Yu T M, Gambetta J, et al. 2007 Phys. Rev. A 76 042319
Google Scholar
[23] Zhao H, Li T F, Liu Q C, et al. 2014 Chin. Phys. Lett. 31 102101
Google Scholar
[24] 赵虎, 李铁夫, 刘其春, 张颖珊, 刘建设, 陈炜 2014 63 220305
Google Scholar
Zhao H, Li T F, Liu Q C, Zhang Y S, Liu J S, Chen W 2014 Acta Phys. Sin. 63 220305
Google Scholar
-
图 1 3D Transmon的S21变功率扫描结果 (a) S21强度图; (b)部分S21曲线
Figure 1. The power change scan S21 of 3D Transmon: (a) Intensity graph of S21; (b) partial S21 curve.
图 2 3D Transmon测试系统
Figure 2. 3D Transmon measurement system.
图 3 微波信号连续激励量子比特时的S21曲线
Figure 3. The S21 curve for the qubits excited by continuously microwave.
图 4 网络分析仪测量相干振荡时序图
Figure 4. Time sequence diagram of coherent oscillation measured by network analyzer.
图 5 网络分析仪测量3D Transmon相干振荡幅度与相位图
Figure 5. Amplitude and phase diagram of 3D transmon coherent oscillation measured by network analyzer
图 6 (a) 不同微波功率下的相干振荡强度图; (b) 相干振荡与微波激励幅度关系
Figure 6. (a) Intensity diagram of coherent oscillation at different microwave power; (b) relationship between coherent oscillation and microwave excitation amplitude.
图 7 数据采集卡Jaynes-Cummings方法测试拉比振荡
Figure 7. Rabi oscillation by Jaynes-Cummings method based on data acquisition card.
-
[1] You J Q, Nori F 2005 Phys. Today 58 42
[2] You J Q, Nori F 2011 Nature 474 589
Google Scholar
[3] Krantz P, Kjaergaard M, Yan F, et al. 2019 Appl. Phys. Rev. 6 021318
Google Scholar
[4] 赵虎, 李铁夫, 刘建设, 陈炜 2012 61 154214
Google Scholar
Zhao H, Li T F, Liu J S, Chen W 2012 Acta Phys. Sin. 61 154214
Google Scholar
[5] Neill C, Roushan P, Kechedzhi K, et al. 2018 Science 360 6385
[6] Klimov P V, Kelly J, Chen J, et al. 2018 Phys. Rev. Lett. 121 090502
Google Scholar
[7] Blais A, Huang R S, Wallraff A, et al. 2004 Phys. Rev. A 69 666
[8] Bunyk P I, Hoskinson E M, Johnson M W, et al. 2014 IEEE Trans. Appl. Supercond. 24 4
Google Scholar
[9] Mooij J E, Orlando T P, Leviotv L, et al. 1999 Science 285 1036
Google Scholar
[10] Nakamura Y, Pashkin Y A, Tsai J S 1999 Nature 398 786
Google Scholar
[11] Friedman J R, Patel V, Chen W, et al. 2000 Nature 406 43
Google Scholar
[12] Martinis J M, Nam S, Aumentado J, Urbina C 2002 Phys. Rev. Lett. 89 117901
Google Scholar
[13] Wallraff A, Schuster D I, Blais A, et al. 2004 Nature 431 162
Google Scholar
[14] Paik H, Schuster D I, Bishop L S, et al. 2011 Phys. Rev. Lett. 107 240501
Google Scholar
[15] Chiorescu I, Nakamura Y, Harmans C J P M, et al. 2003 Science 299 1869
Google Scholar
[16] Vion D, Aassime A, Cottet A, et al. 2002 Science 296 886
Google Scholar
[17] Barends R, Kelly J, Megrant A, et al. 2013 Phys. Rev. Lett. 111 080502
Google Scholar
[18] Yan F, Gustavsson S, Kamal A, et al. 2016 Nat. Commun. 7 12964
Google Scholar
[19] You J Q, Hu X, Ashhab S, et al. 2006 Phys. Rev. B 75 140551
[20] Wallraff A, Schuster D I, Blais A, et al. 2005 Phys. Rev. Lett. 95 060501
Google Scholar
[21] Reed M D, DiCarlo L, Johnson B R, et al. 2010 Phys. Rev. Lett. 105 173601
Google Scholar
[22] Koch J, Yu T M, Gambetta J, et al. 2007 Phys. Rev. A 76 042319
Google Scholar
[23] Zhao H, Li T F, Liu Q C, et al. 2014 Chin. Phys. Lett. 31 102101
Google Scholar
[24] 赵虎, 李铁夫, 刘其春, 张颖珊, 刘建设, 陈炜 2014 63 220305
Google Scholar
Zhao H, Li T F, Liu Q C, Zhang Y S, Liu J S, Chen W 2014 Acta Phys. Sin. 63 220305
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 5967
- PDF Downloads: 93
- Cited By: 0
