Quantitative relations between fundamental nonlinear waves and modulation instability

Duan Liang; Liu Chong; Zhao Li-Chen; Yang Zhan-Ying

doi:10.7498/aps.69.20191385

Abstract

Nonlinear waves are ubiquitous in various physical systems, and they have become one of the research hotspots in nonlinear physics. For the experimental realization, observation and application of nonlinear waves, it is very important to understand the generation mechanism, and determine the essential excitation conditions of various nonlinear waves. In this paper, we first briefly review the experimental and theoretical research progress of nonlinear waves in recent years. Based on the exact nonlinear wave solutions and linear stability analysis results, we systemically discuss how to establish the quantitative relations between fundamental nonlinear waves and modulation instability. These relations would deepen our understanding on the mechanism of nonlinear waves. To solve the excitation conditions degenerations problem for some nonlinear waves, we further introduce the perturbation energy and relative phase to determine the excitation conditions of nonlinear waves. Finally, we present a set of complete parameters that can determine the excitation conditions of nonlinear waves, and give the excitation conditions and phase diagrams of the fundamental nonlinear waves. These results can be used to realize controllable excitation of nonlinear waves, and could be extended to many other nonlinear systems.

Keywords:

Authors and contacts

1.
School of Physics, Northwest University, Xi’an 710127, China
2.
Shaanxi Key Laboratory for Theoretical Physics Frontiers, Xi’an 710069, China

Corresponding author: Yang Zhan-Ying, zyyang@nwu.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 11875220, 11775176)

FullText HTML

References

[1]	Guo B, Pan X 1990 Chin. Phys. Lett. 7 241 Google Scholar
[2]	Lou S Y, Ni G J, Huang G X 1992 Commun. Theor. Phys. 17 67 Google Scholar
[3]	Chabchoub A, Hoffmann N P, Akhmediev N 2011 Phys. Rev. Lett. 106 204502 Google Scholar
[4]	Chabchoub A, Hoffmann N, Onorato M, et al. 2012 Phys. Rev. X 2 011015
[5]	Chabchoub A, Hoffmann N, Onorato M, et al. 2012 Phys. Rev. E 86 056601 Google Scholar
[6]	Chen Z, Segev M, et al 1996 Opt. Lett. 21 1821 Google Scholar
[7]	Chen Z, Segev M, et al 1997 J. Opt. Soc. Am. B 14 1407 Google Scholar
[8]	Guo Q, Luo B, Yi F, Chi S, Xie Y 2004 Phys. Rev. E 69 016602 Google Scholar
[9]	Deng D, Guo Q 2007 Opt. Lett. 32 3206 Google Scholar
[10]	Solli D R, Ropers C, Koonath P, et al. 2007 Nature 450 1054 Google Scholar
[11]	Kibler B, Fatome J, Finot C, et al. 2010 Nat. Phys. 6 790 Google Scholar
[12]	Dudley J M, Genty G, Dias F, et al. 2009 Opt. Express 17 21497 Google Scholar
[13]	Liu X 2011 Phys. Rev. A 84 053828 Google Scholar
[14]	Kibler B, Fatome J, Finot C, et al. 2012 Sci. Rep. 2 463 Google Scholar
[15]	Jia J, Lin J 2012 Opt. Express 20 7469 Google Scholar
[16]	Zhang Y, Belic M, Wu Z, Zheng H, Lu K, Li Y, Zhang Y 2013 Opt. Lett. 38 4585 Google Scholar
[17]	Lin J, Chen W W, Jia J 2014 J. Opt. Soc. Am. A 31 188 Google Scholar
[18]	Liu W, Pang L, Han H, Shen Z, Lei M, Teng H, Wei Z 2016 Photon. Research 4 111 Google Scholar
[19]	Liu W, Pang L, Yan H, Ma G, Lei M, Wei Z 2016 EuroPhys. Lett. 116 64002 Google Scholar
[20]	Liu X, Yao X, Cui Y 2018 Phys. Rev. Lett. 121 023905 Google Scholar
[21]	Liu X, Popa D, Akhmediev N 2019 Phys. Rev. Lett. 123 093901 Google Scholar
[22]	Bailung H, Sharma S K, Nakamura Y 2011 Phys. Rev. Lett. 107 255005 Google Scholar
[23]	Tsai Y Y, Tsai J Y, Lin I 2016 Nat. Phys. 12 573 Google Scholar
[24]	Zhang W, Walls D F 1994 Phys. Rev. Lett. 72 60 Google Scholar
[25]	Zhang W, Walls D F 1994 Phys. Rev. A 49 3799 Google Scholar
[26]	Burger S, Bongs K, Dettmer S, et al. 1999 Phys. Rev. Lett. 83 5198 Google Scholar
[27]	Denschlag J, Simsarian J E, Feder D L, et al. 2000 Science 287 97 Google Scholar
[28]	Huang G X 2001 Chin. Phys. Lett. 18 628 Google Scholar
[29]	Khaykovich L, Schreck F, Ferrari G, et al. 2002 Science 296 1290 Google Scholar
[30]	Strecker K E, Partridge G B, Truscott A G, et al. 2002 Nature 417 150 Google Scholar
[31]	Liang Z X, Zhang Z D, Liu W M 2005 Phys. Rev. Lett. 94 050402 Google Scholar
[32]	Bludov Y V, Konotop V V, Akhmediev N 2009 Phys. Rev. A 80 033610 Google Scholar
[33]	Zhao D, Luo H G, Chai H Y 2008 Phys. Lett. A 372 5644 Google Scholar
[34]	Feng B, Zhao D 2016 J. Differ. Equations 260 2973 Google Scholar
[35]	Zeng J, Malomed B A 2017 Phys. Rev. E 95 052214 Google Scholar
[36]	Yao Y Q, Han W, Li J, Liu W M 2018 J. Phys. B 51 105001 Google Scholar
[37]	Wang D S, Liu J, Wang L 2018 Phys. Lett. A 382 799 Google Scholar
[38]	He Z M, Wen L, Wang Y J, Chen G P, Tan R B, Dai C Q, Zhang X F 2019 Phys. Rev. E 99 062216 Google Scholar
[39]	Shats M, Punzmann H, Xia H 2010 Phys. Rev. Lett. 104 104503 Google Scholar
[40]	Daniel M, Kavitha L, Amuda R 1999 Phys. Rev. B 59 13774 Google Scholar
[41]	Daniel M, Beula J 2009 Chaos, Solitons Fractals 41 1842
[42]	Daniel M, Beula J 2009 Phys. Lett. A 373 2841 Google Scholar
[43]	Zhao F, Li Z D, Li Q Y, et al 2012 Ann. Phys. 327 2085 Google Scholar
[44]	Qi J W, Li Z D, Yang Z Y, et al. 2017 Phys. Lett. A 381 1874 Google Scholar
[45]	Yan Z 2010 Commun. Theor. Phys. 54 947 Google Scholar
[46]	Yan Z 2011 Phys. Lett. A 375 4274 Google Scholar
[47]	Wu Y, Zhao L C, Lei X K 2015 Eur. Phys. J. B 88 297 Google Scholar
[48]	Zheludev N I, Kivshar Y S 2012 Nat. Mater. 11 917 Google Scholar
[49]	Wen S, Wang Y, Su W, Xiang Y, Fu X, Fan D 2006 Phys. Rev. E 73 036617 Google Scholar
[50]	Xiong H, Gan J, Wu Y 2017 Phys. Rev. Lett. 119 153901 Google Scholar
[51]	Konotop V V, Yang J, Zezyulin D A 2016 Rev. Mod. Phys. 88 035002 Google Scholar
[52]	Lou S Y, Huang F 2017 Sci. Rep. 7 869 Google Scholar
[53]	Polo J, Ahufinger V 2013 Phys. Rev. A 88 053628 Google Scholar
[54]	McDonald G D, Kuhn C C N, Hardman K S, et al. 2014 Phys. Rev. Lett. 113 013002 Google Scholar
[55]	Helm J L, Cornish S L, Gardiner S A 2015 Phys. Rev. Lett. 114 134101 Google Scholar
[56]	Zhao L C, Ling L, Yang Z Y, Liu J 2016 Nonlinear Dyn. 83 659 Google Scholar
[57]	Solli D R, Ropers C, Jalali B 2008 Phys. Rev. Lett. 101 233902 Google Scholar
[58]	Frisquet B, Chabchoub A, Fatome J, et al. 2014 Phys. Rev. A 89 023821 Google Scholar
[59]	Gertjerenken B, Billam T P, Blackley C L, et al. 2013 Phys. Rev. Lett. 111 100406 Google Scholar
[60]	Fatome J, Kibler B, Finot C 2013 Opt. Lett. 38 1663 Google Scholar
[61]	Yang G, Wang Y, Qin Z, et al. 2014 Phys. Rev. E 90 062909 Google Scholar
[62]	Zhao L C 2018 Phys. Rev. E 97 062201 Google Scholar
[63]	Hasegawa A, Tappert F 1973 Appl. Phys. Lett. 23 171 Google Scholar
[64]	Zakharov V E, Shabat A B 1973 Sov. Phys. JETP 37 823
[65]	Kivshar Y S, Luther-Davies B 1998 Phys. Rep. 298 81 Google Scholar
[66]	Kivshar Y S 1991 Phys. Rev. A 43 1677 Google Scholar
[67]	Kivshar Y S, Afanasjev V V 1991 Phys. Rev. A 44 R1446 Google Scholar
[68]	Dong G, Liu Z 1996 Opt. Commun. 128 8 Google Scholar
[69]	Li Z, Li L, Tian H, et al. 2000 Phys. Rev. Lett. 84 4096 Google Scholar
[70]	Zhao L C, Li S C, Ling L 2014 Phys. Rev. E 89 023210 Google Scholar
[71]	Liu C, Yang Z Y, Zhao L C, et al. 2015 Phys. Rev. E 91 022904 Google Scholar
[72]	Ren Y, Yang Z Y, Liu C, et al. 2015 Phys. Lett. A 379 2991 Google Scholar
[73]	Liu C, Yang Z Y, Zhao L C, et al. 2016 Phys. Rev. E 94 042221 Google Scholar
[74]	Zhao L C, Li S C, Ling L 2016 Phys. Rev. E 93 032215 Google Scholar
[75]	Peregrine D H 1983 J. Australas. Math. Soc. Ser. B 25 16 Google Scholar
[76]	Akhmediev N N, Korneev V I 1986 Theor. Math. Phys. 1089
[77]	Kuznetsov E A 1977 Akademiia Nauk SSSR Doklady 236 575
[78]	Ma Y C 1979 Stud. Appl. Math. 60 43 Google Scholar
[79]	Tajiri M, Watanabe Y 1998 Phys. Rev. E 57 3510 Google Scholar
[80]	Priya N V, Senthilvelan M, Lakshmanan M 2013 Phys. Rev. E 88 022918 Google Scholar
[81]	刘冲 2016 博士学位论文(西安: 西北大学) Liu C 2016 Ph. D. Dissertation (Xi’an: Northwest University) (in Chinese)
[82]	Chowdury A, Ankiewicz A, Akhmediev N 2015 Proc. R. Soc. A 471 20150130 Google Scholar
[83]	Zakharov V E, Gelash A A 2013 Phys. Rev. Lett. 111 054101 Google Scholar
[84]	Kibler B, Chabchoub A, Gelash A, Akhmediev N, Zakharov V E 2015 Phys. Rev. X 5 041026
[85]	Zhang J H, Wang L, Liu C 2017 Proc. R. Soc. A 473 20160681 Google Scholar
[86]	Liu C, Ren Y, Yang Z Y, Yang W L 2017 Chaos 27 083120 Google Scholar
[87]	Liu C, Yang Z Y, Yang W L 2018 Chaos 28 083110 Google Scholar
[88]	Ren Y, Liu C, Yang Z Y, Yang W L 2018 Phys. Rev. E 98 062223 Google Scholar
[89]	Ren Y, Wang X, Liu C, Yang Z Y, Yang W L 2018 Commun. Nonlinear Sci. Numer. Simul. 63 161 Google Scholar
[90]	Xu G, Gelash A, Chabchoub A, Zakharov V, Kibler B 2019 Phys. Rev. Lett. 122 084101 Google Scholar
[91]	Liu C, Yang Z Y, Yang W L, Akhmediev N 2019 J. Opt. Soc. Am. B 36 1294 Google Scholar
[92]	Guo B, Ling L 2011 Chin. Phys. Lett. 28 110202 Google Scholar
[93]	Guo B, Ling L, Liu Q P 2012 Phys. Rev. E 85 026607 Google Scholar
[94]	Ling L, Zhao L C 2013 Phys. Rev. E 88 043201
[95]	Ling L, Guo B, Zhao L C 2014 Phys. Rev. E 89 041201(R)
[96]	Zhao L C, Liu J 2012 J. Opt. Soc. Am. B 29 3119 Google Scholar
[97]	Zhao L C, Liu J 2013 Phys. Rev. E 87 013201 Google Scholar
[98]	Zhao L C, Xin G G, Yang Z Y 2014 Phys. Rev. E 90 022918 Google Scholar
[99]	Baronio F, Conforti M, Degasperis A, et al. 2013 Phys. Rev. Lett. 111 114101 Google Scholar
[100]	Baronio F, Conforti M, Degasperis A, et al. 2014 Phys. Rev. Lett. 113 034101 Google Scholar
[101]	Zhao L C, Ling L, Yang Z Y, Liu J 2015 Commun. Nonlinear Sci. Numer. Simul. 23 21 Google Scholar
[102]	Chen S, Cai X M, Grelu P, et al. 2016 Opt. Express 24 5886 Google Scholar
[103]	Ling L, Zhao L C, Yang Z Y, et al. 2017 Phys. Rev. E 96 022211 Google Scholar
[104]	Yan Z 2015 Nonlinear Dyn. 79 2515 Google Scholar
[105]	Wen X Y, Yang Y, Yan Z 2015 Phys. Rev. E 92 012917 Google Scholar
[106]	Zhao L C, Guo B, Ling L 2016 J. Math. Phys. 57 043508 Google Scholar
[107]	Zhang G, Yan Z, Wen X Y, Chen Y 2017 Phys. Rev. E 95 042201 Google Scholar
[108]	Li M, Shui J J, Xu T 2018 Appl. Math. Lett. 83 110 Google Scholar
[109]	Zhao L C, Ling L 2014 arXiv: 1410.7536; 2016 J. Opt. Soc. Am. B 33 850
[110]	Baronio F, Chen S, Grelu P, et al. 2015 Phys. Rev. A 91 033804 Google Scholar
[111]	He J S, Xu S, Porsezian K 2012 J. Phys. Soc. Jpn. 81 124007 Google Scholar
[112]	Xu S, He J S, Cheng Y, Porseizan K 2015 Math. Meth. Appl. Sci. 38 1106?Google Scholar
[113]	Xu S, He J S 2012 J. Math. Phys. 53 063507 Google Scholar
[114]	Zhao L C, Yang Z Y, Ling L 2014 J. Phys. Soc. Jpn. 83 104401 Google Scholar
[115]	Zhao L C, Liu C, Yang Z Y 2015 Commun. Nonlinear Sci. Numer. Simul. 20 9 Google Scholar
[116]	Akhmediev N, Soto-Crespo J M, Ankiewicz A 2009 Phys. Rev. A 80 043818 Google Scholar
[117]	He J S, Zhang H R, Wang L H, Porsezian K, Fokas A S 2013 Phys. Rev. E 87 052914 Google Scholar
[118]	Wang L, He J S, Xu H, Wang J, Porsezian K 2017 Phys. Rev. E 95 042217 Google Scholar
[119]	Zakharov V E, Ostrovsky L A 2009 Physica D 238 540 Google Scholar
[120]	Hammani K, Wetzel B, Kibler B, et al. 2011 Opt. Lett. 36 2140 Google Scholar
[121]	Soto-Crespo J M, Ankiewicz A, Devine N, et al. 2012 J. Opt. Soc. Am. B 29 1930 Google Scholar
[122]	Forest M G, McLaughlin D W, Muraki D J, et al. 2000 J. Nonlinear Sci. 10 291 Google Scholar
[123]	Hirota R 1973 J. Math. Phys. 14 805 Google Scholar
[124]	Sasa N, Satsuma J 1991 J. Phys. Soc. Jpn. 60 409 Google Scholar
[125]	Duan L, Zhao L C, Xu W H, et al. 2017 Phys. Rev. E 95 042212 Google Scholar
[126]	Li P, Wang L, Kong L Q, et al. 2018 Appl. Math. Lett. 85 110 Google Scholar
[127]	Erkintalo M, Genty G, Wetzel B, et al. 2011 Phys. Lett. A 375 2029 Google Scholar
[128]	Agrawal G P 2007 Nonlinear Fiber Optics (Massachusetts: Academic Press)
[129]	Kevrekidis P G, Frantzeskakis D, Carretero-Gonzalez R 2007 Emergent Nonlinear Phenomena in BoseEinstein Condensates: Theory and Experiment (New York: Springer Science and Business Media)
[130]	Kawaguchi Y, Ueda M 2012 Phys. Rep. 520 253 Google Scholar
[131]	Gao P, Duan L, Zhao L C, et al. 2019 Chaos 29 083112 Google Scholar
[132]	Duan L, Yang Z Y, Zhao L C, et al. 2016 J. Mod. Opt. 63 1397 Google Scholar
[133]	Liu X S, Zhao L C, Duan L, et al. 2017 Chin. Phys. B 26 120503 Google Scholar
[134]	Liu C, Yang Z Y, Zhao L C, et al. 2015 Ann. Phys. 362 130 Google Scholar
[135]	Wang L, Li S, Qi F H 2016 Nonlinear Dyn. 85 389 Google Scholar
[136]	Wang X, Liu C, Wang L 2017 Chaos 27 093106 Google Scholar
[137]	Blanco-Redondo A, De Sterke C M, Sipe J E, et al. 2016 Nat. Commun. 7 10427 Google Scholar
[138]	Daniel M, Kavitha L 2001 Phys. Rev. B 63 172302 Google Scholar
[139]	Tao Y, He J S 2012 Phys. Rev. E 85 026601 Google Scholar
[140]	Wang L H, Porsezian K, He J S 2013 Phys. Rev. E 87 053202 Google Scholar
[141]	Ankiewicz A, Wang Y, Wabnitz S, et al. 2014 Phys. Rev. E 89 012907 Google Scholar
[142]	Chowdury A, Kedziora D J, Ankiewicz A, et al. 2014 Phys. Rev. E 90 032922 Google Scholar
[143]	Yang Y, Yan Z, Malomed B A 2015 Chaos 25 103112 Google Scholar
[144]	Chowdury A, Kedziora D J, Ankiewicz A, et al. 2015 Phys. Rev. E 91 032928 Google Scholar
[145]	Ankiewicz A, Kedziora D J, Chowdury A, et al. 2016 Phys. Rev. E 93 012206 Google Scholar
[146]	Wang L, Zhang J H, Wang Z Q, et al. 2016 Phys. Rev. E 93 012214 Google Scholar
[147]	Zhao L C, Ling L, Yang Z Y 2018 Phys. Rev. E 97 022218 Google Scholar
[148]	Duan L, Yang Z Y, Gao P, et al. 2019 Phys. Rev. E 99 012216 Google Scholar
[149]	Wen X Y, Yan Z, Boris A, Malomed 216 Chaos 26 123110
[150]	Wen X Y, Yan Z 2018 J. Math. Phys. 59 073511 Google Scholar
[151]	Wen X Y, Yan Z 2017 Commun. Nonlinear. Sci. Numer. Simul. 43 311 Google Scholar
[152]	Ohta Y, Yang J 2012 Phys. Rev. E 86 036604 Google Scholar
[153]	Yang B, Chen Y 2018 Appl. Math. Lett. 82 43 Google Scholar
[154]	Li M, Xu T, Meng D 2016 J. Phys. Soc. Jpn. 85 124001 Google Scholar
[155]	Xu T, Li H, Zhang H, et al. 2017 Appl. Math. Lett. 63 88 Google Scholar
[156]	Dai C Q, Zhu H P 2013 J. Opt. Soc. Am. B 30 3291 Google Scholar
[157]	Chen J, Chen Y, Feng B F, Maruno K 2015 Phys. Lett. A 379 1510 Google Scholar
[158]	Zhang X, Chen Y, Tang X 2018 Comput. Math. Appl. 76 1938 Google Scholar
[159]	Qian C, Rao J G, Liu Y B, He J S 2016 Chin. Phys. Lett. 33 110201 Google Scholar
[160]	Zhang X, Chen Y 2017 Commun. Nonlinear. Sci. Numer. Simul. 52 24 Google Scholar
[161]	Liu Y K, and Li B 2017 Chin. Phys. Lett. 34 010202 Google Scholar
[162]	Charalampidis E G, Wang W, Kevrekidis P G, Frantzeskakis D J, Cuevas-Maraver J 2016 Phys. Rev. A 93 063623 Google Scholar
[163]	Wang W, Kevrekidis P G, Carretero-Gonzalez R, Frantzeskakis D J 2016 Phys. Rev. A 93 023630 Google Scholar
[164]	Rao J, Porsezian K, He J S 2017 Chaos 27 083115??Google Scholar
[165]	Rao J, Porsezian K, He J S, Kanna T 2018 Proc. R. Soc. A 474 20170627
[166]	Zeng L, Zeng J, Kartashov Y V, Malomed B A 2019 Opt. Lett. 44 1206 Google Scholar

Cited By

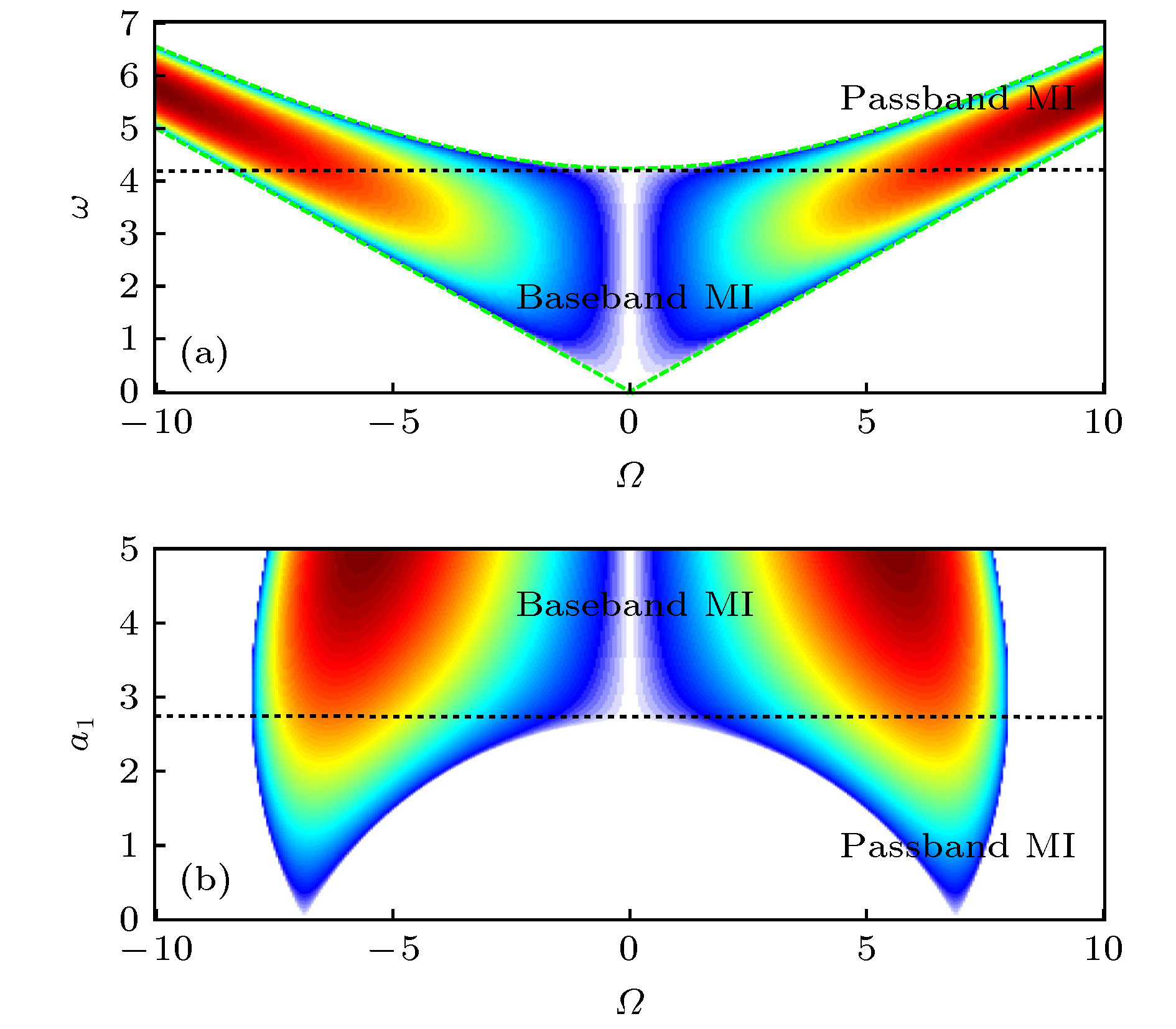
图 1 自散焦的两组分耦合非线性薛定谔系统的调制不稳定增益的分布　(a)调制不稳定增益在$(\varOmega, \omega)$平面的分布, 绿色点状曲线表示调制不稳定区的边界; (b)调制不稳定性在$(\varOmega, a_{1})$平面的分布

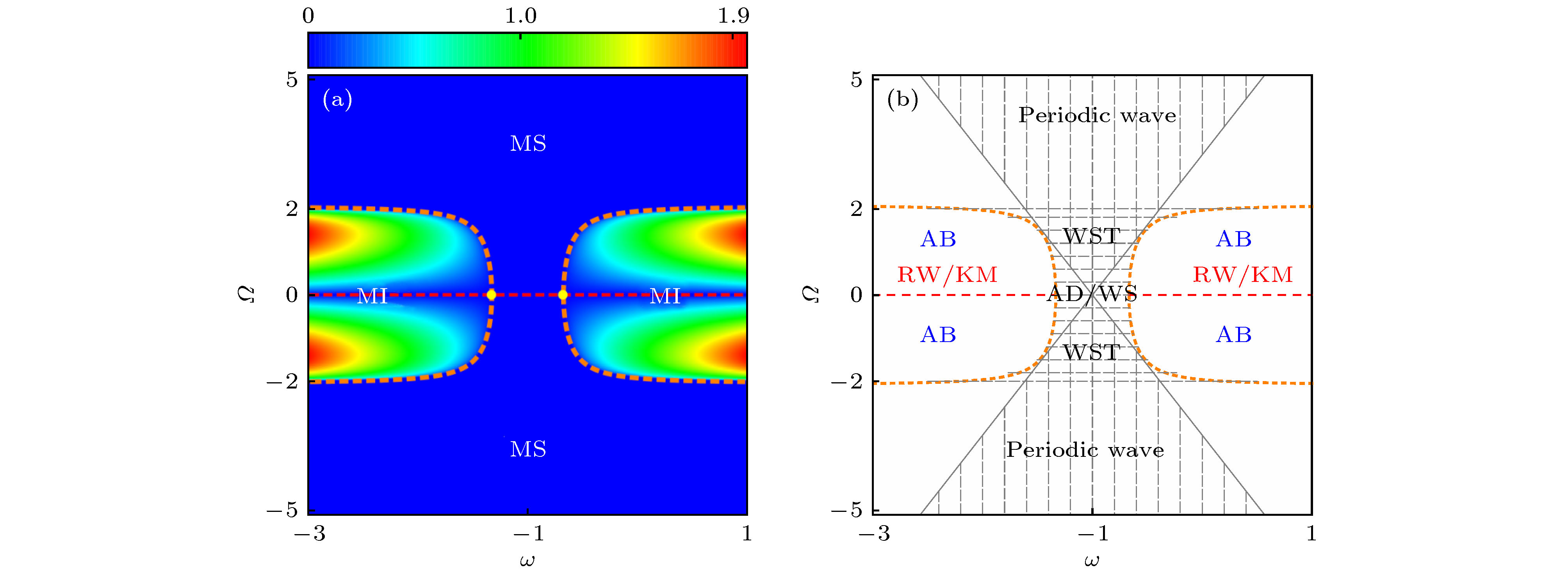
Figure 1. Modulation instability distributions of the defocusing two component coupled nonlinear Schrödinger system: (a) Modulation instability distribution in the $(\varOmega, \omega)$ plane, green dot curves are the boundary of the modulation instability regime; (b) modulation instability distribution in the $(\varOmega, a_{1})$ plane.

DownLoad: Full-Size Img PowerPoint

图 2 标准非线性薛定谔系统的调制不稳定增益分布和基本非线性波激发的相图　(a1)和(b1)分别为调制不稳定增益在$(\omega, \varOmega)$平面和$(a, \varOmega)$平面的分布. “MI”和“MS”分别表示调制不稳定性和调制稳定性, 红色虚线是共振线; (a2)和(b2)分别为基本非线性波在(a1)和(b1)中调制不稳定增益分布平面的相图. “AB”,“RW”和“KM”分别为Akhmediev呼吸子、怪波和Kuznetsov-Ma呼吸子

Figure 2. Modulation instability distributions and phase diagrams of fundamental nonlinear waves in standard nonlinear Schrödinger system: (a1) and (b1) are the distributions of the modulation instability gain in the $(\omega, \varOmega)$ plane and the $(a, \varOmega)$, respectively. “MI” and “MS” denote modulation instability and modulation stability, respectively. the red dotted line is the resonance line; (a2) and (b2) are the phase diagrams of fundamental nonlinear waves on the modulation instability gain distribution planes correspond to (a1) and (b1), respectively. "AB", "RW" and "KM" denote Akhmediev breather, rogue wave and Kuznetsov-Ma breather, respectively.

DownLoad: Full-Size Img PowerPoint

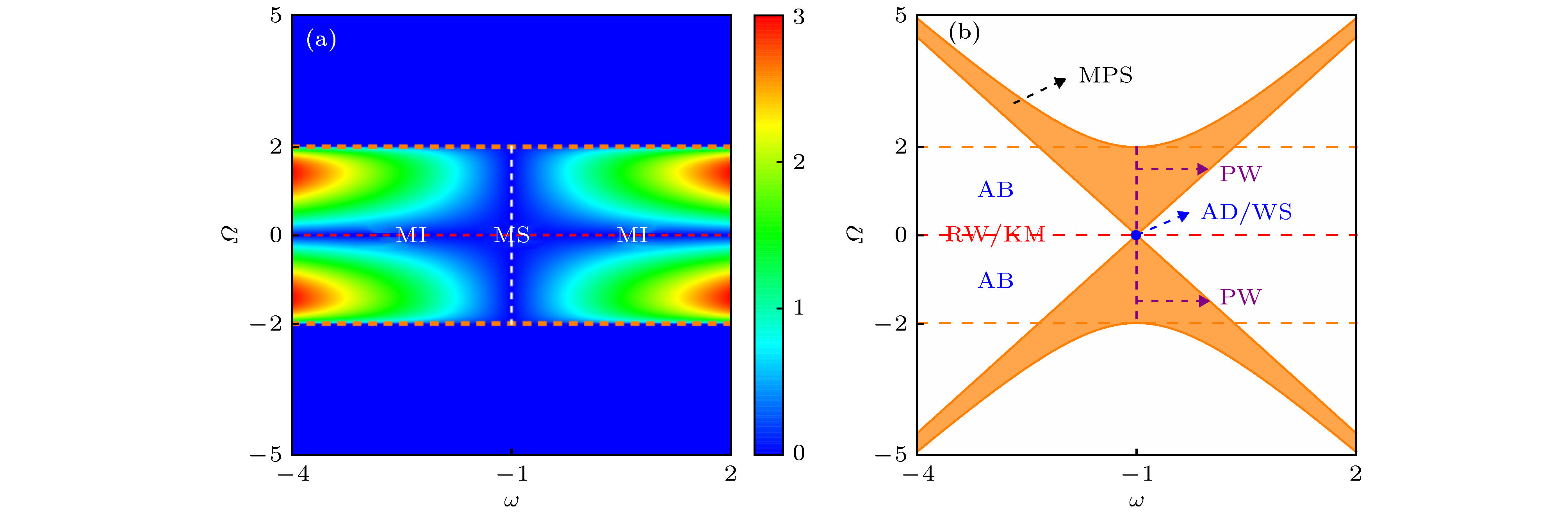
图 3 Sasa-Satsuma系统的调制不稳定增益分布和基本非线性波激发的相图　(a) Sasa-Satsuma系统中调制不稳定增益在背景频率$\omega$和扰动频率$\varOmega$平面的分布. “MI”和“MS”分别表示调制不稳定和调制稳定, 黄颜色圆点为共振线上临界点; (b)非线性波在调制不稳定增益分布平面的相图. “AB”, “RW” 和“KM” 分别为Akhmediev呼吸子、怪波和Kuznetsov-Ma呼吸子; “WS”, “WST”, “AD”和Periodic wave分别表示W形孤子、W形孤子链、反暗孤子和周期波

Figure 3. Modulation instability distributions and phase diagrams of fundamental nonlinear waves in Sasa-Satsuma system: (a) Distributions of the modulation instability gain in the background frequency $\omega$ and perturbation frequency$\varOmega$ plane. “MI” and “MS” denote modulation instability and modulation stability, respectively. The yellow dots are the critical points on the resonance line; (b) phase diagrams of nonlinear waves in the modulation instability gain distribution planes. “AB”, “RW” and “KM” denote Akhmediev breather, rogue wave and Kuznetsov-Ma breather, respectively; “WS”, “WST” and “AD” denote the W-shaped soliton, W-shaped soliton train and anti-dark soliton, respectively.

DownLoad: Full-Size Img PowerPoint

图 4 Hirota系统中的调制不稳定增益分布和基本非线性波激发的相图　(a) Hirota系统中调制不稳定增益在背景频率$\omega$和扰动频率$\varOmega$平面的分布. “MI”和“MS”分别表示调制不稳定和调制稳定; (b)非线性波在调制不稳定增益分布平面的相图. “AB”, “RW” 和“KM” 分别为Akhmediev呼吸子、怪波和Kuznetsov-Ma呼吸子; “WS”, “AD”, “PW”和“MPS”分别表示W形孤子、反暗孤子、周期波和多峰孤子

Figure 4. Modulation instability distributions and phase diagrams of fundamental nonlinear waves in Hirota system; (a) Distributions of the modulation instability gain in the background frequency $\omega$ and perturbation frequency$\varOmega$ plane. “MI” and “MS” denote modulation instability and modulation stability, respectively; (b) phase diagrams of nonlinear waves in the modulation instability gain distribution planes. “AB”, “RW” and “KM” denote Akhmediev breather, rogue wave and Kuznetsov-Ma breather, respectively; “WS”, “AD”, “PW” and “MPS” denote the W-shaped soliton, anti-dark soliton, periodic wave and multi-peak soliton, respectively.

DownLoad: Full-Size Img PowerPoint

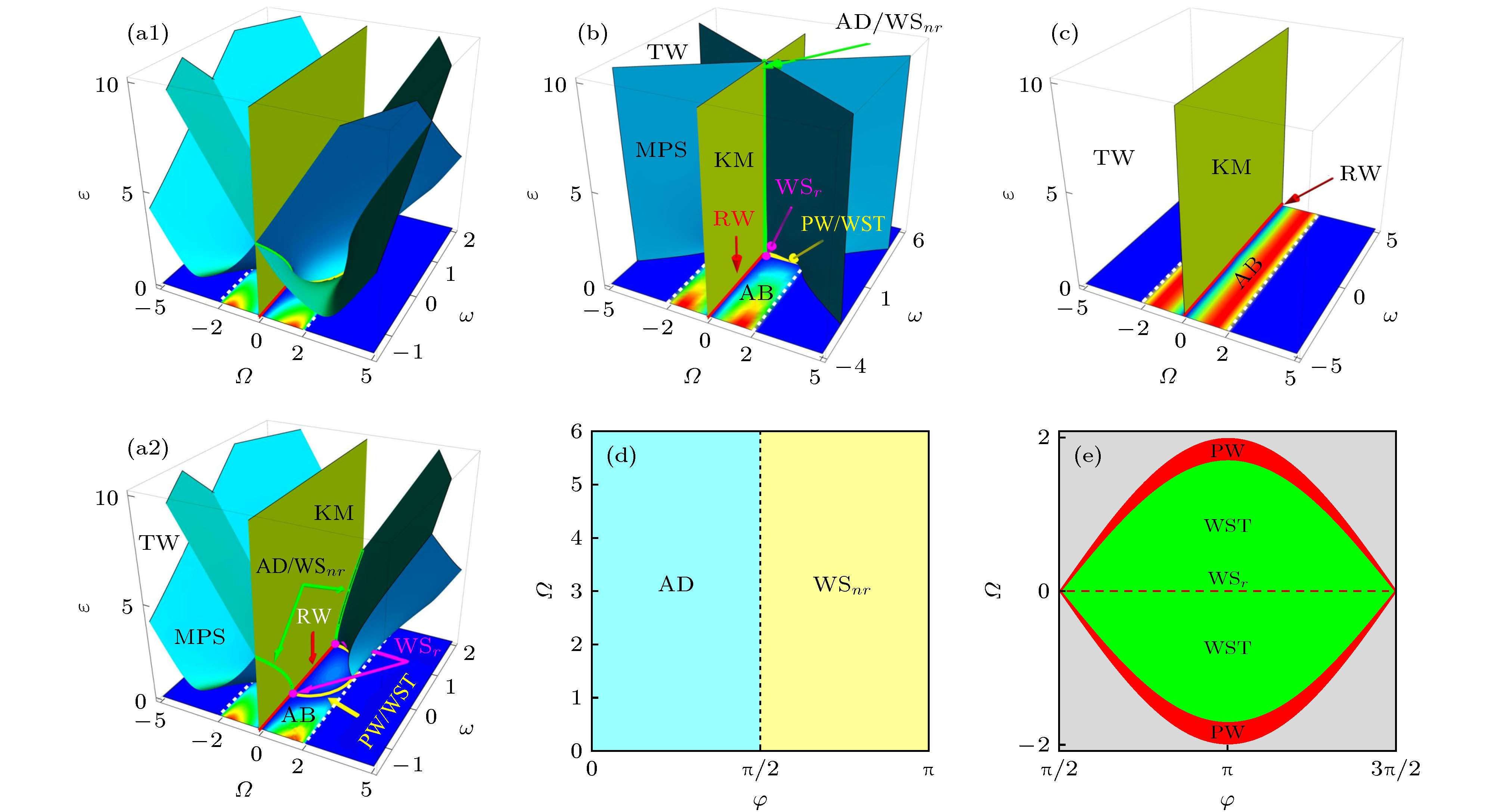
图 5 四阶非线性薛定谔系统调制不稳定增益分布和基本非线性波激发的相图　(a) 调制不稳定增益在背景频率$\omega$和扰动频率$\varOmega$平面的分布, “MI”和“MS” 分别表示调制不稳定性和调制稳定性; (b),(c) 基本非线性波在背景频率$\omega$和扰动频率$\varOmega$平面的相图, “AB”, “RW”, “KM”、“PW”, “WST”, “WS$_{\rm r}$”, “$\rm WS_{ {nr}}$” 和“AD”分别为Akhmediev呼吸子、怪波、Kuznetsov-Ma呼吸子、周期波、W形孤子链、有理的W形孤子、非有理的W形孤子和反暗孤子

Figure 5. Modulation instability distributions and phase diagrams of fundamental nonlinear waves in fourth-order nonlinear Schrödinger system: (a) Distributions of the modulation instability gain in the background frequency $\omega$ and perturbation frequency$\varOmega$ plane. “MI” and “MS” denote modulation instability and modulation stability, respectively; (b), (c) phase diagrams of nonlinear waves in the background frequency $\omega$ and perturbation frequency $\varOmega$ plane. “AB”, “RW”, “KM”, “PW”, “WST”, “WS$_{\rm r}$”, “WS$\rm WS_{{nr}}$” and “AD” denote Akhmediev breather, rogue wave, Kuznetsov-Ma breather, periodic wave, W-shaped soliton train, rational W-shaped soliton, nonrational W-shaped soliton and anti-dark soliton, respectively.

DownLoad: Full-Size Img PowerPoint

图 6 不同系统中平面波背景上基本非线性波在背景频率$\omega$, 扰动频率$\varOmega$, 扰动能量$\varepsilon$和相对相位$\varphi$空间的相图　(a) 四阶非线性薛定谔系统, 参数取$\beta={1}/{12}$, $\gamma=-{1}/{36}$, $a=1$; (b) Hirota系统, 参数取$\beta={1}/{12}$, $\gamma=0$, $a=1$; (c)非线性薛定谔系统, 参数取$\beta={1}/{12}$, $\gamma=0$, $a=1$; (d)反暗孤子和非有理W形孤子依赖于相对相位的相图; (e)周期波, W形孤子链和有理W形孤子在$(\varphi, \varOmega)$平面的相图. 图中“TW”, “KM”, “AB”, “RW”, “MPS”, “AD”, “WS$_{\rm {nr}}$”, “PW”, “WST”和“WS$_{\rm r}$”分别表示Tajiri-Watanabe呼吸子、Kuznetsov-Ma呼吸子、Akhmediev呼吸子、怪波、多峰孤子、反暗孤子、非有理W形孤子、周期波、W形孤子链和有理W形孤子

Figure 6. Phase diagrams of nonlinear waves in the background frequency $\omega$, perturbation frequency $\varOmega$, perturbation energy $\varepsilon$ and relative phase $\varphi$ space for different systems: (a) Fourth-order nonlinear Schrödinger system. Parameters are $\beta={1}/{12}$, $\gamma=-{1}/{36}$, $a=1$; (b) hirota system. Parameters are $\beta={1}/{12}$, $\gamma=0$, $a=1$; (c) nonlinear Schrödinger system. Parameters are $\beta=\gamma=0$, $a=1$; (d) phase diagram of anti-dark soliton and nonrational W-shaped soliton in relative phase space; (e) phase diagram of periodic wave, W-shaped soliton train and rational W-shaped soliton in the $(\varphi, \varOmega)$ plane. “TW”, “KM”, “AB”, “RW”, “MPS”, “AD”, “WS$_{\rm {nr}}$”, “PW”, “WST” and “WS$_{\rm r}$” denote Tajiri-Watanabe breather, Kuznetsov-Ma breather, Akhmediev breather, rogue wave, multi-peak soliton, anti-dark soliton, nonrational W-shaped soliton, periodic wave, W-shaped soliton train and rational W-shaped soliton.

DownLoad: Full-Size Img PowerPoint

图 7 不同非线性波的转换关系　(a) 呼吸子和怪波之间的转换关系； (b) 孤子和周期波之间的转换关系. 图中“TW”, “KM”, “AB”, “RW”分别为Tajiri-Watanabe呼吸子、Kuznetsov-Ma呼吸子、Akhmediev呼吸子和怪波, “MPS”, “AD”, “WS$_{\rm {nr}}$”, “PW”, “WST”和“WS$_{\rm r}$” 分别表示多峰孤子、反暗孤子、非有理W形孤子、周期波、W形孤子链和有理W形孤子

Figure 7. Conversion relationship of different nonlinear waves: (a) Conversion relationship between breathers and rogue wave; (b) conversion relationship between the solitons and periodic waves. “TW”, “KM”, “AB”, “RW”, “MPS”, “AD”, “WS$_{\rm {nr}}$”, “PW”, “WST” and “WS$_{\rm r}$” denote Tajiri-Watanabe breather, Kuznetsov-Ma breather, Akhmediev breather, rogue wave, multi-peak soliton, anti-dark soliton, nonrational W-shaped soliton, periodic wave, W-shaped soliton train and rational W-shaped soliton.

DownLoad: Full-Size Img PowerPoint

表 1 基本非线性波的激发条件

Table 1. Excitation conditions of fundamental nonlinear waves.

激发条件				非线性波类型
$\varOmega$	$\omega$	$\varepsilon$	$\varphi$	非线性波类型
0	$\omega^{2}-\alpha\neq0 $	0	$\varphi\in \left(\dfrac{{\text{π}}}{2}, \dfrac{3{\text{π}}}{2}\right)+2 n{\text{π}}$	怪波
0	$\omega^{2}-\alpha=0$, $\alpha\geqslant 0$	0		有理W形孤子
0	$\omega^{2}-\dfrac{\varepsilon^{2}}{24}-\alpha\neq0$, $\varepsilon>0$		$\varphi\in\mathbb{R}$	Kuznetsov-Ma呼吸子
	$\omega^{2}-\dfrac{\varepsilon^{2}}{24}-\alpha=0$, $\varepsilon>0$		$\varphi\in\left(\dfrac{{\text{π}}}{2},\right. \left.\dfrac{3{\text{π}}}{2}\right]+2 n{\text{π}}$	非有理W形孤子
	$\omega^{2}-\dfrac{\varepsilon^{2}}{24}-\alpha=0$, $\varepsilon > 0$		$\varphi\in \left(-\dfrac{{\text{π}}}{2},\right. \left.\dfrac{{\text{π}}}{2}\right]+2 n{\text{π}}$	反暗孤子
$\omega^{2}+\dfrac{\varOmega^{2} }{6}-\alpha\neq0, \varOmega\in(0, 2)$		0	$\varphi\in \left(\dfrac{{\text{π}}}{2},\dfrac{3{\text{π}}}{2}\right)+2 n{\text{π}}$	Akhmediev呼吸子
$\omega^{2}+\dfrac{\varOmega^{2} }{6}-\alpha=0$			$0<\|\varOmega\|<\dfrac{\sqrt{3}}{\|\sec\varphi\|}$	W形孤子链
$\omega^{2}+\dfrac{\varOmega^{2} }{6}-\alpha=0$			$\dfrac{\sqrt{3}}{\|\sec\varphi\|}<\|\varOmega\|<\dfrac{2}{\|\sec\varphi\|}$	周期波
$1+2\beta\left(\pm\sqrt{\varDelta}-3\omega\right)+2\gamma\nabla\neq0$			$\varphi\in \mathbb{\rm R}$	Tajiri-Watanabe呼吸子
$1+2\beta\left(\pm\sqrt{\varDelta}-3\omega\right)+2\gamma\nabla=0$			$\varphi\in \mathbb{\rm R}$	多峰孤子
注1: $\omega$, $\varOmega$, $\varepsilon$和$\varphi$分别为背景频率、扰动频率、扰动能量和相对相位. 参数$\alpha=\dfrac{\beta^{2}}{16\gamma^{2}}+\dfrac{1}{12\gamma}+a^{2}$, $\varDelta = {\bigg[ {\dfrac{ {\sqrt { { {({\varepsilon ^2} - 4{\varOmega ^2} + 16{a^2})}^2} + 16{\varepsilon ^2}{\varOmega ^2} } - ({\varepsilon ^2} - 4{\varOmega ^2} + 16{a^2})} }{8} } \bigg]^{1/2} }$, $\nabla=-2\varDelta\pm8\omega\sqrt{\varDelta}-6\omega^{2}+6 a^{2}+\dfrac{1}{4}\varepsilon^{2}-\varOmega^{2}$.

DownLoad: CSV

map

[1]	Guo B, Pan X 1990 Chin. Phys. Lett. 7 241 Google Scholar
[2]	Lou S Y, Ni G J, Huang G X 1992 Commun. Theor. Phys. 17 67 Google Scholar
[3]	Chabchoub A, Hoffmann N P, Akhmediev N 2011 Phys. Rev. Lett. 106 204502 Google Scholar
[4]	Chabchoub A, Hoffmann N, Onorato M, et al. 2012 Phys. Rev. X 2 011015
[5]	Chabchoub A, Hoffmann N, Onorato M, et al. 2012 Phys. Rev. E 86 056601 Google Scholar
[6]	Chen Z, Segev M, et al 1996 Opt. Lett. 21 1821 Google Scholar
[7]	Chen Z, Segev M, et al 1997 J. Opt. Soc. Am. B 14 1407 Google Scholar
[8]	Guo Q, Luo B, Yi F, Chi S, Xie Y 2004 Phys. Rev. E 69 016602 Google Scholar
[9]	Deng D, Guo Q 2007 Opt. Lett. 32 3206 Google Scholar
[10]	Solli D R, Ropers C, Koonath P, et al. 2007 Nature 450 1054 Google Scholar
[11]	Kibler B, Fatome J, Finot C, et al. 2010 Nat. Phys. 6 790 Google Scholar
[12]	Dudley J M, Genty G, Dias F, et al. 2009 Opt. Express 17 21497 Google Scholar
[13]	Liu X 2011 Phys. Rev. A 84 053828 Google Scholar
[14]	Kibler B, Fatome J, Finot C, et al. 2012 Sci. Rep. 2 463 Google Scholar
[15]	Jia J, Lin J 2012 Opt. Express 20 7469 Google Scholar
[16]	Zhang Y, Belic M, Wu Z, Zheng H, Lu K, Li Y, Zhang Y 2013 Opt. Lett. 38 4585 Google Scholar
[17]	Lin J, Chen W W, Jia J 2014 J. Opt. Soc. Am. A 31 188 Google Scholar
[18]	Liu W, Pang L, Han H, Shen Z, Lei M, Teng H, Wei Z 2016 Photon. Research 4 111 Google Scholar
[19]	Liu W, Pang L, Yan H, Ma G, Lei M, Wei Z 2016 EuroPhys. Lett. 116 64002 Google Scholar
[20]	Liu X, Yao X, Cui Y 2018 Phys. Rev. Lett. 121 023905 Google Scholar
[21]	Liu X, Popa D, Akhmediev N 2019 Phys. Rev. Lett. 123 093901 Google Scholar
[22]	Bailung H, Sharma S K, Nakamura Y 2011 Phys. Rev. Lett. 107 255005 Google Scholar
[23]	Tsai Y Y, Tsai J Y, Lin I 2016 Nat. Phys. 12 573 Google Scholar
[24]	Zhang W, Walls D F 1994 Phys. Rev. Lett. 72 60 Google Scholar
[25]	Zhang W, Walls D F 1994 Phys. Rev. A 49 3799 Google Scholar
[26]	Burger S, Bongs K, Dettmer S, et al. 1999 Phys. Rev. Lett. 83 5198 Google Scholar
[27]	Denschlag J, Simsarian J E, Feder D L, et al. 2000 Science 287 97 Google Scholar
[28]	Huang G X 2001 Chin. Phys. Lett. 18 628 Google Scholar
[29]	Khaykovich L, Schreck F, Ferrari G, et al. 2002 Science 296 1290 Google Scholar
[30]	Strecker K E, Partridge G B, Truscott A G, et al. 2002 Nature 417 150 Google Scholar
[31]	Liang Z X, Zhang Z D, Liu W M 2005 Phys. Rev. Lett. 94 050402 Google Scholar
[32]	Bludov Y V, Konotop V V, Akhmediev N 2009 Phys. Rev. A 80 033610 Google Scholar
[33]	Zhao D, Luo H G, Chai H Y 2008 Phys. Lett. A 372 5644 Google Scholar
[34]	Feng B, Zhao D 2016 J. Differ. Equations 260 2973 Google Scholar
[35]	Zeng J, Malomed B A 2017 Phys. Rev. E 95 052214 Google Scholar
[36]	Yao Y Q, Han W, Li J, Liu W M 2018 J. Phys. B 51 105001 Google Scholar
[37]	Wang D S, Liu J, Wang L 2018 Phys. Lett. A 382 799 Google Scholar
[38]	He Z M, Wen L, Wang Y J, Chen G P, Tan R B, Dai C Q, Zhang X F 2019 Phys. Rev. E 99 062216 Google Scholar
[39]	Shats M, Punzmann H, Xia H 2010 Phys. Rev. Lett. 104 104503 Google Scholar
[40]	Daniel M, Kavitha L, Amuda R 1999 Phys. Rev. B 59 13774 Google Scholar
[41]	Daniel M, Beula J 2009 Chaos, Solitons Fractals 41 1842
[42]	Daniel M, Beula J 2009 Phys. Lett. A 373 2841 Google Scholar
[43]	Zhao F, Li Z D, Li Q Y, et al 2012 Ann. Phys. 327 2085 Google Scholar
[44]	Qi J W, Li Z D, Yang Z Y, et al. 2017 Phys. Lett. A 381 1874 Google Scholar
[45]	Yan Z 2010 Commun. Theor. Phys. 54 947 Google Scholar
[46]	Yan Z 2011 Phys. Lett. A 375 4274 Google Scholar
[47]	Wu Y, Zhao L C, Lei X K 2015 Eur. Phys. J. B 88 297 Google Scholar
[48]	Zheludev N I, Kivshar Y S 2012 Nat. Mater. 11 917 Google Scholar
[49]	Wen S, Wang Y, Su W, Xiang Y, Fu X, Fan D 2006 Phys. Rev. E 73 036617 Google Scholar
[50]	Xiong H, Gan J, Wu Y 2017 Phys. Rev. Lett. 119 153901 Google Scholar
[51]	Konotop V V, Yang J, Zezyulin D A 2016 Rev. Mod. Phys. 88 035002 Google Scholar
[52]	Lou S Y, Huang F 2017 Sci. Rep. 7 869 Google Scholar
[53]	Polo J, Ahufinger V 2013 Phys. Rev. A 88 053628 Google Scholar
[54]	McDonald G D, Kuhn C C N, Hardman K S, et al. 2014 Phys. Rev. Lett. 113 013002 Google Scholar
[55]	Helm J L, Cornish S L, Gardiner S A 2015 Phys. Rev. Lett. 114 134101 Google Scholar
[56]	Zhao L C, Ling L, Yang Z Y, Liu J 2016 Nonlinear Dyn. 83 659 Google Scholar
[57]	Solli D R, Ropers C, Jalali B 2008 Phys. Rev. Lett. 101 233902 Google Scholar
[58]	Frisquet B, Chabchoub A, Fatome J, et al. 2014 Phys. Rev. A 89 023821 Google Scholar
[59]	Gertjerenken B, Billam T P, Blackley C L, et al. 2013 Phys. Rev. Lett. 111 100406 Google Scholar
[60]	Fatome J, Kibler B, Finot C 2013 Opt. Lett. 38 1663 Google Scholar
[61]	Yang G, Wang Y, Qin Z, et al. 2014 Phys. Rev. E 90 062909 Google Scholar
[62]	Zhao L C 2018 Phys. Rev. E 97 062201 Google Scholar
[63]	Hasegawa A, Tappert F 1973 Appl. Phys. Lett. 23 171 Google Scholar
[64]	Zakharov V E, Shabat A B 1973 Sov. Phys. JETP 37 823
[65]	Kivshar Y S, Luther-Davies B 1998 Phys. Rep. 298 81 Google Scholar
[66]	Kivshar Y S 1991 Phys. Rev. A 43 1677 Google Scholar
[67]	Kivshar Y S, Afanasjev V V 1991 Phys. Rev. A 44 R1446 Google Scholar
[68]	Dong G, Liu Z 1996 Opt. Commun. 128 8 Google Scholar
[69]	Li Z, Li L, Tian H, et al. 2000 Phys. Rev. Lett. 84 4096 Google Scholar
[70]	Zhao L C, Li S C, Ling L 2014 Phys. Rev. E 89 023210 Google Scholar
[71]	Liu C, Yang Z Y, Zhao L C, et al. 2015 Phys. Rev. E 91 022904 Google Scholar
[72]	Ren Y, Yang Z Y, Liu C, et al. 2015 Phys. Lett. A 379 2991 Google Scholar
[73]	Liu C, Yang Z Y, Zhao L C, et al. 2016 Phys. Rev. E 94 042221 Google Scholar
[74]	Zhao L C, Li S C, Ling L 2016 Phys. Rev. E 93 032215 Google Scholar
[75]	Peregrine D H 1983 J. Australas. Math. Soc. Ser. B 25 16 Google Scholar
[76]	Akhmediev N N, Korneev V I 1986 Theor. Math. Phys. 1089
[77]	Kuznetsov E A 1977 Akademiia Nauk SSSR Doklady 236 575
[78]	Ma Y C 1979 Stud. Appl. Math. 60 43 Google Scholar
[79]	Tajiri M, Watanabe Y 1998 Phys. Rev. E 57 3510 Google Scholar
[80]	Priya N V, Senthilvelan M, Lakshmanan M 2013 Phys. Rev. E 88 022918 Google Scholar
[81]	刘冲 2016 博士学位论文(西安: 西北大学) Liu C 2016 Ph. D. Dissertation (Xi’an: Northwest University) (in Chinese)
[82]	Chowdury A, Ankiewicz A, Akhmediev N 2015 Proc. R. Soc. A 471 20150130 Google Scholar
[83]	Zakharov V E, Gelash A A 2013 Phys. Rev. Lett. 111 054101 Google Scholar
[84]	Kibler B, Chabchoub A, Gelash A, Akhmediev N, Zakharov V E 2015 Phys. Rev. X 5 041026
[85]	Zhang J H, Wang L, Liu C 2017 Proc. R. Soc. A 473 20160681 Google Scholar
[86]	Liu C, Ren Y, Yang Z Y, Yang W L 2017 Chaos 27 083120 Google Scholar
[87]	Liu C, Yang Z Y, Yang W L 2018 Chaos 28 083110 Google Scholar
[88]	Ren Y, Liu C, Yang Z Y, Yang W L 2018 Phys. Rev. E 98 062223 Google Scholar
[89]	Ren Y, Wang X, Liu C, Yang Z Y, Yang W L 2018 Commun. Nonlinear Sci. Numer. Simul. 63 161 Google Scholar
[90]	Xu G, Gelash A, Chabchoub A, Zakharov V, Kibler B 2019 Phys. Rev. Lett. 122 084101 Google Scholar
[91]	Liu C, Yang Z Y, Yang W L, Akhmediev N 2019 J. Opt. Soc. Am. B 36 1294 Google Scholar
[92]	Guo B, Ling L 2011 Chin. Phys. Lett. 28 110202 Google Scholar
[93]	Guo B, Ling L, Liu Q P 2012 Phys. Rev. E 85 026607 Google Scholar
[94]	Ling L, Zhao L C 2013 Phys. Rev. E 88 043201
[95]	Ling L, Guo B, Zhao L C 2014 Phys. Rev. E 89 041201(R)
[96]	Zhao L C, Liu J 2012 J. Opt. Soc. Am. B 29 3119 Google Scholar
[97]	Zhao L C, Liu J 2013 Phys. Rev. E 87 013201 Google Scholar
[98]	Zhao L C, Xin G G, Yang Z Y 2014 Phys. Rev. E 90 022918 Google Scholar
[99]	Baronio F, Conforti M, Degasperis A, et al. 2013 Phys. Rev. Lett. 111 114101 Google Scholar
[100]	Baronio F, Conforti M, Degasperis A, et al. 2014 Phys. Rev. Lett. 113 034101 Google Scholar
[101]	Zhao L C, Ling L, Yang Z Y, Liu J 2015 Commun. Nonlinear Sci. Numer. Simul. 23 21 Google Scholar
[102]	Chen S, Cai X M, Grelu P, et al. 2016 Opt. Express 24 5886 Google Scholar
[103]	Ling L, Zhao L C, Yang Z Y, et al. 2017 Phys. Rev. E 96 022211 Google Scholar
[104]	Yan Z 2015 Nonlinear Dyn. 79 2515 Google Scholar
[105]	Wen X Y, Yang Y, Yan Z 2015 Phys. Rev. E 92 012917 Google Scholar
[106]	Zhao L C, Guo B, Ling L 2016 J. Math. Phys. 57 043508 Google Scholar
[107]	Zhang G, Yan Z, Wen X Y, Chen Y 2017 Phys. Rev. E 95 042201 Google Scholar
[108]	Li M, Shui J J, Xu T 2018 Appl. Math. Lett. 83 110 Google Scholar
[109]	Zhao L C, Ling L 2014 arXiv: 1410.7536; 2016 J. Opt. Soc. Am. B 33 850
[110]	Baronio F, Chen S, Grelu P, et al. 2015 Phys. Rev. A 91 033804 Google Scholar
[111]	He J S, Xu S, Porsezian K 2012 J. Phys. Soc. Jpn. 81 124007 Google Scholar
[112]	Xu S, He J S, Cheng Y, Porseizan K 2015 Math. Meth. Appl. Sci. 38 1106?Google Scholar
[113]	Xu S, He J S 2012 J. Math. Phys. 53 063507 Google Scholar
[114]	Zhao L C, Yang Z Y, Ling L 2014 J. Phys. Soc. Jpn. 83 104401 Google Scholar
[115]	Zhao L C, Liu C, Yang Z Y 2015 Commun. Nonlinear Sci. Numer. Simul. 20 9 Google Scholar
[116]	Akhmediev N, Soto-Crespo J M, Ankiewicz A 2009 Phys. Rev. A 80 043818 Google Scholar
[117]	He J S, Zhang H R, Wang L H, Porsezian K, Fokas A S 2013 Phys. Rev. E 87 052914 Google Scholar
[118]	Wang L, He J S, Xu H, Wang J, Porsezian K 2017 Phys. Rev. E 95 042217 Google Scholar
[119]	Zakharov V E, Ostrovsky L A 2009 Physica D 238 540 Google Scholar
[120]	Hammani K, Wetzel B, Kibler B, et al. 2011 Opt. Lett. 36 2140 Google Scholar
[121]	Soto-Crespo J M, Ankiewicz A, Devine N, et al. 2012 J. Opt. Soc. Am. B 29 1930 Google Scholar
[122]	Forest M G, McLaughlin D W, Muraki D J, et al. 2000 J. Nonlinear Sci. 10 291 Google Scholar
[123]	Hirota R 1973 J. Math. Phys. 14 805 Google Scholar
[124]	Sasa N, Satsuma J 1991 J. Phys. Soc. Jpn. 60 409 Google Scholar
[125]	Duan L, Zhao L C, Xu W H, et al. 2017 Phys. Rev. E 95 042212 Google Scholar
[126]	Li P, Wang L, Kong L Q, et al. 2018 Appl. Math. Lett. 85 110 Google Scholar
[127]	Erkintalo M, Genty G, Wetzel B, et al. 2011 Phys. Lett. A 375 2029 Google Scholar
[128]	Agrawal G P 2007 Nonlinear Fiber Optics (Massachusetts: Academic Press)
[129]	Kevrekidis P G, Frantzeskakis D, Carretero-Gonzalez R 2007 Emergent Nonlinear Phenomena in BoseEinstein Condensates: Theory and Experiment (New York: Springer Science and Business Media)
[130]	Kawaguchi Y, Ueda M 2012 Phys. Rep. 520 253 Google Scholar
[131]	Gao P, Duan L, Zhao L C, et al. 2019 Chaos 29 083112 Google Scholar
[132]	Duan L, Yang Z Y, Zhao L C, et al. 2016 J. Mod. Opt. 63 1397 Google Scholar
[133]	Liu X S, Zhao L C, Duan L, et al. 2017 Chin. Phys. B 26 120503 Google Scholar
[134]	Liu C, Yang Z Y, Zhao L C, et al. 2015 Ann. Phys. 362 130 Google Scholar
[135]	Wang L, Li S, Qi F H 2016 Nonlinear Dyn. 85 389 Google Scholar
[136]	Wang X, Liu C, Wang L 2017 Chaos 27 093106 Google Scholar
[137]	Blanco-Redondo A, De Sterke C M, Sipe J E, et al. 2016 Nat. Commun. 7 10427 Google Scholar
[138]	Daniel M, Kavitha L 2001 Phys. Rev. B 63 172302 Google Scholar
[139]	Tao Y, He J S 2012 Phys. Rev. E 85 026601 Google Scholar
[140]	Wang L H, Porsezian K, He J S 2013 Phys. Rev. E 87 053202 Google Scholar
[141]	Ankiewicz A, Wang Y, Wabnitz S, et al. 2014 Phys. Rev. E 89 012907 Google Scholar
[142]	Chowdury A, Kedziora D J, Ankiewicz A, et al. 2014 Phys. Rev. E 90 032922 Google Scholar
[143]	Yang Y, Yan Z, Malomed B A 2015 Chaos 25 103112 Google Scholar
[144]	Chowdury A, Kedziora D J, Ankiewicz A, et al. 2015 Phys. Rev. E 91 032928 Google Scholar
[145]	Ankiewicz A, Kedziora D J, Chowdury A, et al. 2016 Phys. Rev. E 93 012206 Google Scholar
[146]	Wang L, Zhang J H, Wang Z Q, et al. 2016 Phys. Rev. E 93 012214 Google Scholar
[147]	Zhao L C, Ling L, Yang Z Y 2018 Phys. Rev. E 97 022218 Google Scholar
[148]	Duan L, Yang Z Y, Gao P, et al. 2019 Phys. Rev. E 99 012216 Google Scholar
[149]	Wen X Y, Yan Z, Boris A, Malomed 216 Chaos 26 123110
[150]	Wen X Y, Yan Z 2018 J. Math. Phys. 59 073511 Google Scholar
[151]	Wen X Y, Yan Z 2017 Commun. Nonlinear. Sci. Numer. Simul. 43 311 Google Scholar
[152]	Ohta Y, Yang J 2012 Phys. Rev. E 86 036604 Google Scholar
[153]	Yang B, Chen Y 2018 Appl. Math. Lett. 82 43 Google Scholar
[154]	Li M, Xu T, Meng D 2016 J. Phys. Soc. Jpn. 85 124001 Google Scholar
[155]	Xu T, Li H, Zhang H, et al. 2017 Appl. Math. Lett. 63 88 Google Scholar
[156]	Dai C Q, Zhu H P 2013 J. Opt. Soc. Am. B 30 3291 Google Scholar
[157]	Chen J, Chen Y, Feng B F, Maruno K 2015 Phys. Lett. A 379 1510 Google Scholar
[158]	Zhang X, Chen Y, Tang X 2018 Comput. Math. Appl. 76 1938 Google Scholar
[159]	Qian C, Rao J G, Liu Y B, He J S 2016 Chin. Phys. Lett. 33 110201 Google Scholar
[160]	Zhang X, Chen Y 2017 Commun. Nonlinear. Sci. Numer. Simul. 52 24 Google Scholar
[161]	Liu Y K, and Li B 2017 Chin. Phys. Lett. 34 010202 Google Scholar
[162]	Charalampidis E G, Wang W, Kevrekidis P G, Frantzeskakis D J, Cuevas-Maraver J 2016 Phys. Rev. A 93 063623 Google Scholar
[163]	Wang W, Kevrekidis P G, Carretero-Gonzalez R, Frantzeskakis D J 2016 Phys. Rev. A 93 023630 Google Scholar
[164]	Rao J, Porsezian K, He J S 2017 Chaos 27 083115??Google Scholar
[165]	Rao J, Porsezian K, He J S, Kanna T 2018 Proc. R. Soc. A 474 20170627
[166]	Zeng L, Zeng J, Kartashov Y V, Malomed B A 2019 Opt. Lett. 44 1206 Google Scholar

[1]	Hu Zhi, Li Jin-Hua, Li Meng-Meng, Ma You-Qiao, Ren Hai-Dong. Stability analysis of Fermi-Pasta-Ulam-Tsingou phenomenon in nonlinear optical fibers. Acta Physica Sinica, 2024, 73(23): 235201. doi: 10.7498/aps.73.20241380
[2]	Zheng Zhou, Li Jin-Hua, Ma You-Qiao, Ren Hai-Dong. Influence of perturbation amplitude and perturbation frequency on Fermi-Pasta-Ulam-Tsingou recurrence phenomenon. Acta Physica Sinica, 2022, 71(18): 185201. doi: 10.7498/aps.71.20220945
[3]	Li Zai-Dong, Guo Qi-Qi. Rogue wave solution in ferromagnetic nanowires. Acta Physica Sinica, 2020, 69(1): 017501. doi: 10.7498/aps.69.20191352
[4]	Pei Shi-Xin, Xu Hui, Sun Ting-Ting, Li Jin-Hua. Modulation instabilities in equilateral three-core optical fibers for isosceles-triangle symmetric continuous waves. Acta Physica Sinica, 2018, 67(5): 054203. doi: 10.7498/aps.67.20171650
[5]	Chen Hai-Jun. Modulational instability of a two-dimensional Bose-Einstein condensate in an optical lattice through a variational approach. Acta Physica Sinica, 2015, 64(5): 054702. doi: 10.7498/aps.64.054702
[6]	Teng Fei, Xie Zheng-Wei. Modulational instabilities of two-component Bose-Einstein condensates in the optical lattices. Acta Physica Sinica, 2013, 62(2): 026701. doi: 10.7498/aps.62.026701
[7]	Mao Jie-Jian, Yang Jian-Rong. Nonlinear intermodulation waves of large-scale shallow water equations. Acta Physica Sinica, 2013, 62(13): 130205. doi: 10.7498/aps.62.130205
[8]	Zhang Li-Sheng, Deng Min-Yi, Kong Ling-Jiang, Liu Mu-Ren, Tang Guo-Ning. The cellular automaton model for the nonlinear waves in the two-dimensional excitable media. Acta Physica Sinica, 2009, 58(7): 4493-4499. doi: 10.7498/aps.58.4493
[9]	Ding Wan-Shan, Xi Ling, Liu Lian-Hua. Simulation of the modulation instability in dual-core optical fiber based on complex Ginzburg-Landau equation. Acta Physica Sinica, 2008, 57(12): 7705-7711. doi: 10.7498/aps.57.7705
[10]	Huang Jin-Song, Chen Hai-Feng, Xie Zheng-Wei. Modulational instability of two-component dipolar Bose-Einstein condensates in an optical lattice. Acta Physica Sinica, 2008, 57(6): 3435-3439. doi: 10.7498/aps.57.3435
[11]	Dai Xiao-Yu, Wen Shuang-Chun, Xiang Yuan-Jiang. Influence of dispersive magnetic permeability on modulation instability in metamaterials. Acta Physica Sinica, 2008, 57(1): 186-193. doi: 10.7498/aps.57.186
[12]	Zhan Jie-Min, Lin Dong, Li Yok-Sheng. Chebyshev generalized finite spectral method for linear and nonlinear waves. Acta Physica Sinica, 2007, 56(7): 3649-3654. doi: 10.7498/aps.56.3649
[13]	Zhao Xing-Dong, Xie Zheng-Wei, Zhang Wei-Ping. Nonlinear spin waves in a Bose condensed atomic chain. Acta Physica Sinica, 2007, 56(11): 6358-6366. doi: 10.7498/aps.56.6358
[14]	Jia Wei-Guo, Shi Pei-Ming, Yang Xing-Yu, Zhang Jun-Ping, Fan Guo-Liang. Modulation instability of fiber Bragg gratings with Gaussian apodization. Acta Physica Sinica, 2007, 56(9): 5281-5286. doi: 10.7498/aps.56.5281
[15]	Liu Zhi-Fang, Zhang Shan-Yuan. A nonlinear wave equation and exact periodic solutions in circular-rod waveguide. Acta Physica Sinica, 2006, 55(2): 628-633. doi: 10.7498/aps.55.628
[16]	Jia Wei-Guo, Shi Pei-Ming, Yang Xing-Yu, Zhang Jun-Ping, Fan Guo-Liang. Modulation instability of near frequency propagation regime in polarization-maintaining fibers. Acta Physica Sinica, 2006, 55(9): 4575-4581. doi: 10.7498/aps.55.4575
[17]	Wu Kun, Wu Jian, Xu Han, Zeng He-Ping. Ultrashort laser pulse up-conversion amplification based on modulation instability. Acta Physica Sinica, 2005, 54(8): 3749-3756. doi: 10.7498/aps.54.3749
[18]	Wen Lei, Yang Jian-Hu, Hu Li-Li. Determination of upconversion state lifetime and upconversion mechanisms of Er3+ in tellurite glasses. Acta Physica Sinica, 2004, 53(12): 4378-4381. doi: 10.7498/aps.53.4378
[19]	Li Qi-Liang, Zhu Hai-Dong, Tang Xiang-Hong, Li Cheng-Jia, Wang Xiao-Jun, Lin Li-Bin. Cross-phase modulational instability in fiber link wit h an active optical amplifier. Acta Physica Sinica, 2004, 53(12): 4194-4201. doi: 10.7498/aps.53.4194*
[20]	Zhang Jie-Qiu, Liang Chang-Hong, Wang Geng-Guo, Zhu Jia-Zhen. Condition of Alfven Gauss wave-packet evolvement to solitons and criterion of Alfven wave modulation instability. Acta Physica Sinica, 2003, 52(4): 890-895. doi: 10.7498/aps.52.890

Catalog

Vol. 69, Issue 1 - 2020-01-05

Metrics

Abstract views: 11840
PDF Downloads: 332
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板