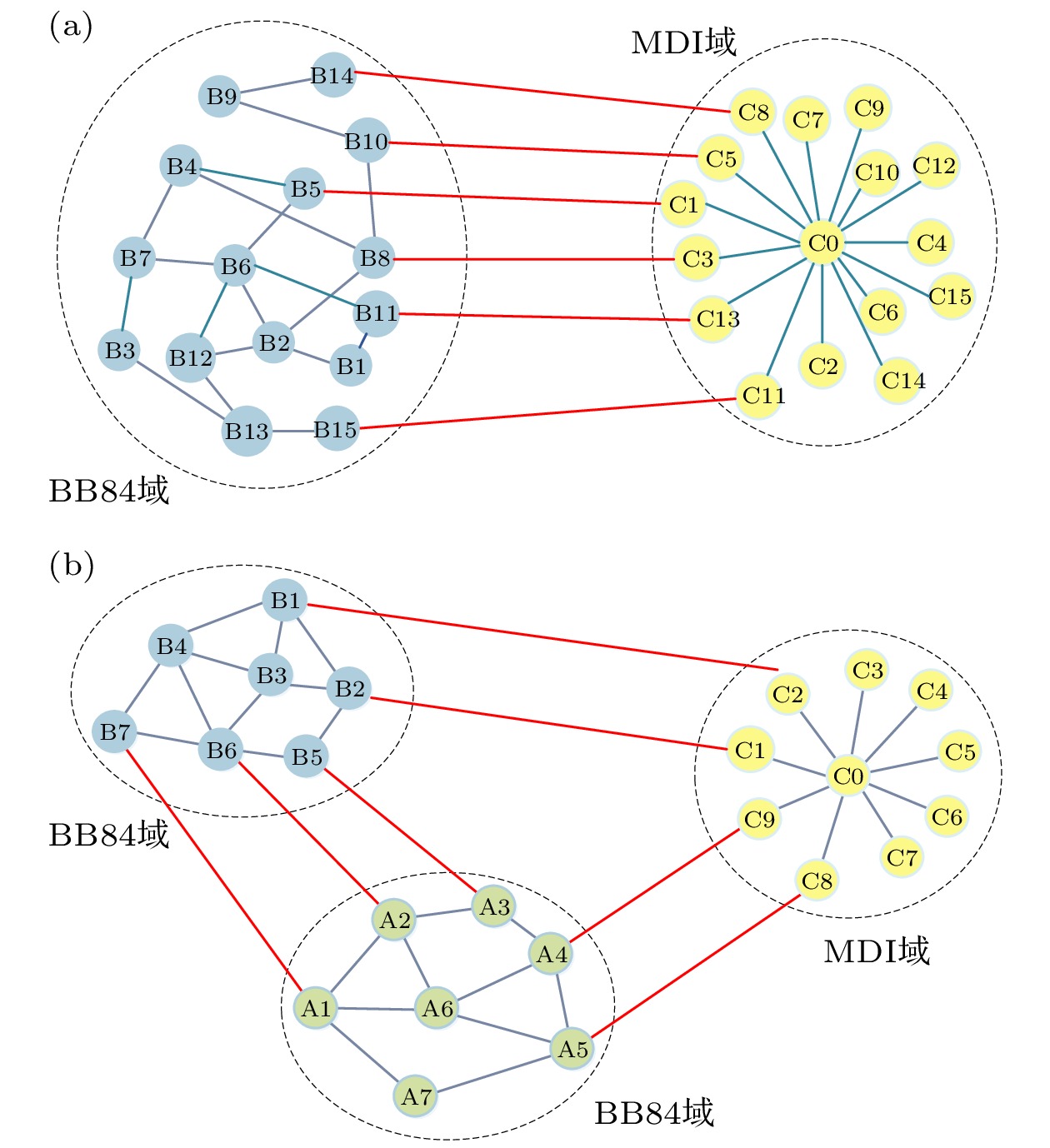
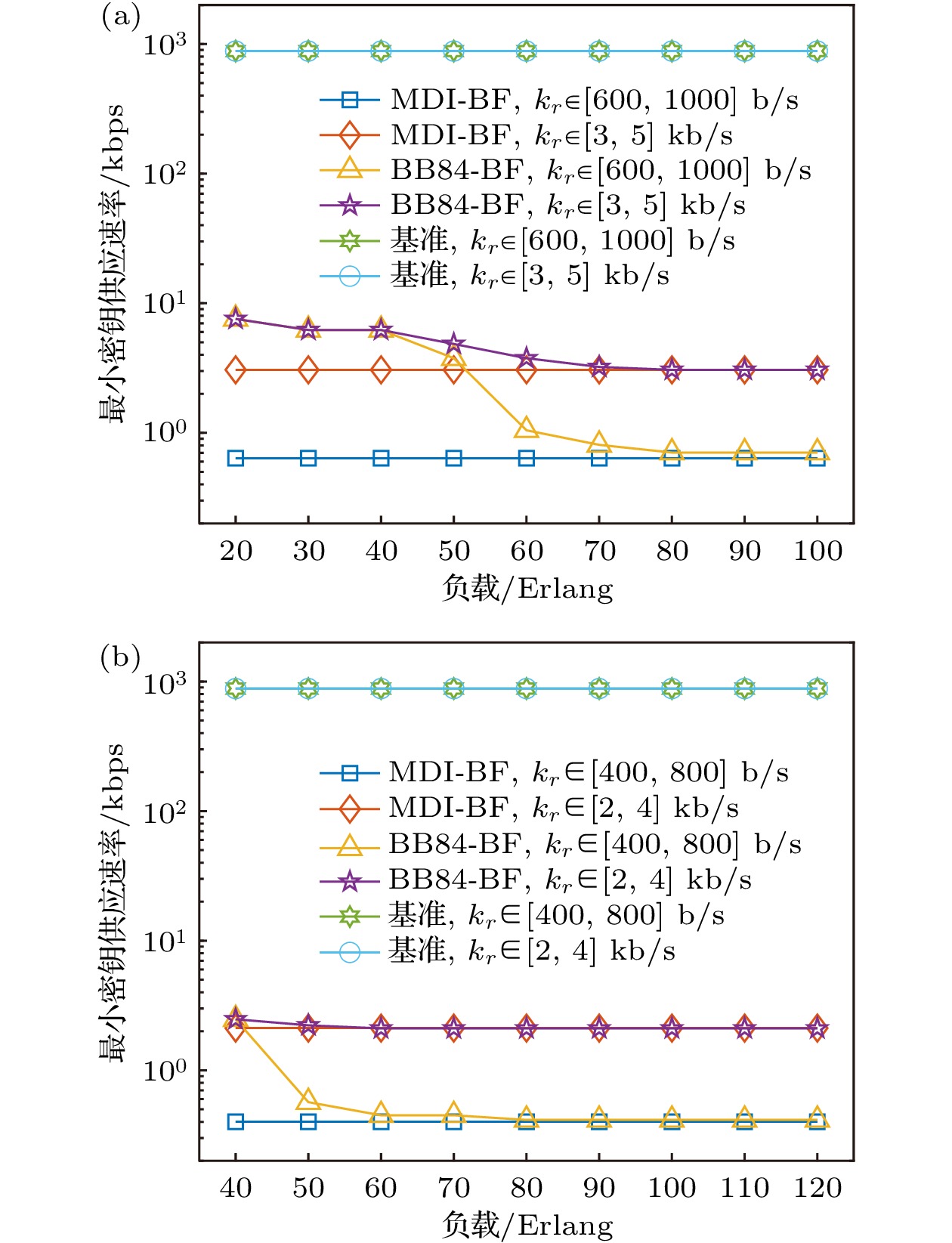
-
现有的城域量子网络大多基于单一的量子密钥分发协议实现, 将不同协议实现的城域量子网络进行互联是大规模量子网络的发展趋势, 但其域间密钥业务提供仍存在成功率低、密钥供需不适配等问题. 针对以上问题, 本文面向多域跨协议量子网络提出了两种域间密钥业务按需提供策略, 分别是基于BB84(Bennett-Brassard-1984)旁路优先的按需提供策略和基于测量设备无关(measurement-device-independent, MDI)旁路优先的按需提供策略. 同时, 设计了内嵌两种策略的域间密钥业务按需提供算法. 仿真结果表明, 所提策略能够在双域和三域量子网络中高效完成域间密钥业务的按需提供. 相比传统策略, 两种按需提供策略可将多域量子网络的密钥供需均衡度提高1个数量级以上, MDI旁路优先策略在低密钥率需求下可将域间密钥业务请求成功率提升30%. 此外, 所提策略可在一定程度上降低域间密钥业务提供的成本, 提高现实安全水平.Most of the existing metropolitan quantum networks are implemented based on a single quantum key distribution protocol, and interconnecting metropolitan quantum networks implemented by different protocols are the development trend of large-scale quantum networks, but there are still some problems in the provision of inter-domain key services, such as low possibility of success and mismatch between key supply and demand. To solve the above problems, this paper proposes two on-demand inter-domain key service provisioning strategies for multi-domain cross-protocol quantum networks, namely, on-demand provisioning strategy based on BB84 bypass first (BB84-BF) and on-demand provisioning strategy based on MDI bypass first (MDI-BF). Meanwhile, a service provisioning model for multi-domain cross-protocol quantum networks is constructed, and an on-demand inter-domain key service provisioning algorithm is designed. Moreover, numerical simulations and performance evaluation are carried out under two scenarios: high key rate demand and low key rate demand for two-domain and three-domain quantum network topologies. Simulation results verify that the proposed on-demand provisioning strategies have better applicability to different multi-domain quantum networks. In addition, for different key rate requirements, the MDI-BF strategy and BB84-BF strategys have different performance advantages under different performance indicators. For example, in terms of the success possibility of inter-domain key service requests, the MDI-BF strategy is more suitable for the low key rate requirements (~30% higher than the traditional strategies in two domain topologies), while the BB84-BF strategy is more suitable for the high key rate requirements (~19% higher than the traditional strategies under two domain topologies). In addition, compared with the traditional strategies, the proposed on-demand provisioning strategies can increase the balance degree between key supply and demand by more than one order of magnitude. Hence, the proposed strategies can reduce the cost of inter-domain key service provisioning and improve the realistic security level.
-
Keywords:
- multi-domain quantum networks /
- quantum key distribution /
- cross-protocol /
- key service provisioning
[1] Yang Z, Zolanvari M, Jain R 2023 IEEE Commun. Surveys Tuts. 25 1059
Google Scholar
[2] Gill S S, Kumar A, Singh H, Singh M, Kaur K, Usman M, Buyya R 2022 Softw. Pract. Exp. 52 66
Google Scholar
[3] Lo H K, Curty M, Tamaki K 2014 Nat. Photon. 8 595
Google Scholar
[4] Pirandola S, Andersen U L, Banchi L, Berta M, Bunandar D, Colbeck R, Englund D, Gehring T, Lupo C, Ottaviani C, Pereira J L, Razavi M, Shamsul S J, Tomamichel M, Usenko V C, Vallone G, Villoresi P, Wallden P 2020 Adv. Opt. Photon. 12 1012
Google Scholar
[5] Bennett C H, Brassard G 1984 IEEE Int. Conf. Comput. Syst. Signal Process. Bangalore, India, January, 1984 p175
[6] Lo H K, Curty M, Qi B 2012 Phys. Rev. Lett. 108 130503
Google Scholar
[7] Li W, Zhang L K, Tan H, Lu Y C, Liao S K, Huang J, Li H, Wang Z, Mao H K, Yan B Z, Li Q, Liu Y, Zhang Q, Peng C Z, You L X, Xu F H, Pan J W 2023 Nat. Photon. 17 416
Google Scholar
[8] Yin H L, Fu Y, Li C L, Weng C X, Li B H, Gu J, Lu Y S, Huang S, Chen Z B 2023 Nat. Sci. Rev. 10 nwac228
Google Scholar
[9] Cao Y, Zhao Y, Wang Q, Zhang J, Ng S X, Hanzo L 2022 IEEE Commun. Surveys Tuts. 24 839
Google Scholar
[10] Tang Y L, Yin H L, Zhao Q, Liu H, Sun X X, Huang M Q, Zhang W J, Chen S J, Zhang L, You L X, Wang Z, Liu Yang, Lu C Y, Jiang X, Ma X F, Zhang Q, Chen T Y, Pan J W 2016 Phys. Rev. X 6 011024
Google Scholar
[11] Joshi S K, Aktas D, Wengerowsky S, Lončarić M, Neumann S P, Liu B, Scheidl T, Lorenzo G C, Samec Ž, Kling L, Qiu A, Razavi M, Stipčević M, Rarity J G, Ursin R 2020 Sci. Adv. 6 eaba0959
Google Scholar
[12] Avesani M, Foletto G, Padovan M, Calderaro L, Agnesi C, Bazzani E, Berra F, Bertapelle T, Picciariello F, Santagiustina F, Scalcon D, Scriminich A, Stanco A, Vedovato F, Vallone G, Villoresi P 2023 Quantum Computing, Communication, and Simulation III San Francisco, United States, 2023 p112
[13] Cao Y, Zhao Y L, Zhang J, Wang Q, Niyato D, Hanzo L 2022 IEEE Netw. 36 14
Google Scholar
[14] Cao Y, Zhao Y L, Zhang J, Wang Q 2022 IEEE Commun. Mag. 60 38
Google Scholar
[15] Zhou L, Lin J P, Xie Y M, Lu Y S, Jing Y M, Yin H L, Yuan Z L 2023 Phys. Rev. Lett. 130 250801
Google Scholar
[16] Fan-Yuan G J, Lu F Y, Wang S, Yin Z Q, He D Y, Zhou Z, Teng J, Chen W, Guo G C, Han Z F 2021 Photonics Res. 9 1881
Google Scholar
[17] Tysowski P K, Ling X, Lütkenhaus N, Mosca M 2018 Quantum Sci. Technol. 3 024001
Google Scholar
[18] Li P, Yu X, Zhao Y, Zhang J 2023 Opto-Electronic and Communications Conference Shanghai, China, July 2–6, 2023 p1
[19] Gottesman D, Lo H K, Lutkenhaus N, Preskill J 2004 Quantum Inf. Comput. 4 325
Google Scholar
[20] Ma X F, Qi B, Zhao Y, Lo H K 2005 Phys. Rev. A 72 012326
Google Scholar
[21] Xu F H, Xu H, Lo H K 2014 Phys. Rev. A 89 052333
Google Scholar
[22] Ma X F, Fung C H F, Razavi M 2012 Phys. Rev. A 86 052305
Google Scholar
[23] Wang X B 2013 Phys. Rev. A 87 012320
Google Scholar
[24] Yu Z W, Zhou Y H, Wang X B 2013 Phys. Rev. A 88 062339
Google Scholar
[25] Curty M, Xu F, Cui W, Lim C C W, Tamaki K, Lo H K 2014 Nat. Commun. 5 3732
Google Scholar
[26] Wang Q, Wang X B 2014 Sci. Rep. 4 4612
Google Scholar
[27] Zhou Y H, Yu Z W, Wang X B 2016 Phys. Rev. A 93 042324
Google Scholar
-
图 2 多域跨协议量子网络的密钥中继结构示例
Fig. 2. Example of the key relay structure in a multi-domain cross-protocol quantum network.
图 3 密钥中继路径示例 (a) 传统策略; (b) MDI-BF策略; (c) BB84-BF策略
Fig. 3. Examples of QKD relay paths: (a) Traditional strategy; (b) MDI-BF strategy; (c) BB84-BF strategy.
图 4 仿真使用的多域量子网络拓扑 (a) 双域拓扑; (b) 三域拓扑
Fig. 4. Multi-domain quantum network topologies used for simulations: (a) Two-domain topology; (b) three-domain topology.
图 5 不同策略下域间密钥业务请求成功率随负载的变化关系 (a) 双域量子网络; (b) 三域量子网络
Fig. 5. Success possibility of inter-domain key service requests versus traffic load for different strategies: (a) Two-domain quantum network; (b) three-domain quantum network.
图 6 不同策略下域间密钥业务请求成功率随最大延迟时间的变化关系
Fig. 6. Success possibility of inter-domain key service requests versus maximum delay time for different strategies.
图 7 不同策略下全网最小密钥供应速率随负载的变化关系 (a) 双域量子网络; (b) 三域量子网络
Fig. 7. Minimum key supply rate versus traffic load for different strategies: (a) Two-domain quantum network; (b) three-domain quantum network.
图 8 不同策略下密钥供需均衡度随负载的变化关系 (a) 双域量子网络; (b) 三域量子网络
Fig. 8. Balance degree between key supply and demand versus traffic load for different strategies: (a) Two-domain quantum network; (b) three-domain quantum network.
图 9 不同策略下密钥供需均衡度随最大延迟时间的变化关系
Fig. 9. Balance degree between key supply and demand versus maximum delay time for different strategies.
图 10 不同策略下平均可信节点数量随负载的变化关系 (a) 双域量子网络; (b) 三域量子网络
Fig. 10. Average number of trusted nodes versus traffic load for different strategies: (a) Two-domain quantum network; (b) three-domain quantum network.
表 1 域间密钥业务按需提供算法
Table 1. Algorithm for on-demand provisioning of inter-domain key services.
输入: $G\left( {V, E} \right)$, ${V_{\text{B}}}$, ${V_{\text{M}}}$, ${V_{{\text{BJ}}}}$, ${V_{{\text{MJ}}}}$, $R$ 输出: 每个成功的域间密钥业务的密钥中继路径${p_r}\left( {{N_{{p_r}}}, {L_{{p_r}}}, {B_{{p_r}}}, {m_{{p_r}}}} \right)$, ${R_{\text{S}}}$ 1 初始化变量${R_{\text{S}}} \leftarrow \emptyset $; 2 for 每个域间密钥业务请求$r\left( {{s_r}, {d_r}, {k_r}, {t_r}} \right) \in R$ do 3 更新全网各节点设备占用状态; 4 如果源宿节点没有可用的QKD设备, 则该业务失败; 5 if 执行BB84-BF策略 then 6 for ${v_i} \in {V_{{\text{MJ}}}}$ do 7 if ${\lambda _{{v_i}}} < 2$ then 8 将${v_i}$从$V$中移除并更新$E$; 9 end if 10 end for 11 end if 12 基于K短路径算法计算源宿节点间的K条备选密钥中继路径, 路径集合为${P_r}$; 13 if ${P_r} = \emptyset $ then 14 域间密钥业务请求r失败; 15 end if 16 for 每条密钥中继路径${p_r}\left( {{N_{{p_r}}}, {L_{{p_r}}}, {B_{{p_r}}}, {m_{{p_r}}}} \right) \in {P_r}$ do 17 ${N_{{p_r}}} \leftarrow $${p_r}$经过的QKD节点集合, ${L_{{p_r}}} \leftarrow $${p_r}$经过的QKD链路集合, ${B_{{p_r}}} \leftarrow \emptyset $, ${m_{{p_r}}} \leftarrow {p_r}$的密钥供应
速率;18 for $n_{{p_r}}^i \in {N_{{p_r}}}$ do 19 if 执行MDI-BF策略 && $n_{{p_r}}^i \in {V_{{\text{MJ}}}}$ then 20 ${B_{{p_r}}} \leftarrow \{ {B_{{p_r}}}, n_{{p_r}}^i\} $; 21 end if 22 if $n_{{p_r}}^i \in {V_{\text{B}}}$ && $n_{{p_r}}^i \ne {d_r}$ && $n_{{p_r}}^i \ne {s_r}$ && (${\lambda _{n_{{p_r}}^i}} = 0~||~{\varepsilon _{n_{{p_r}}^i}} = 0 $) then 23 ${B_{{p_r}}} \leftarrow \{ {B_{{p_r}}}, n_{{p_r}}^i\} $; 24 end if 25 end for 26 ${m_{{p_r}}} \leftarrow $根据更新后的密钥中继路径重新计算${m_{{p_r}}}$; 27 if ${m_{{p_r}}} < {k_r}$ then 28 continue; 29 else 30 for $n_{{p_r}}^i \in {N_{{p_r}}}$do 31 if 旁路$n_{{p_r}}^i$后密钥中继路径密钥供应速率$ \geqslant {k_r}$ then 32 ${B_{{p_r}}} \leftarrow \{ {B_{{p_r}}}, n_{{p_r}}^i\} $, 更新${m_{{p_r}}}$; 33 end if 34 end for 35 将${p_r}$作为域间密钥业务r的最终密钥中继路径, ${R_{\text{S}}} \leftarrow \left\{ {{R_{\text{S}}}, r} \right\}$; 36 break; 37 end if 38 end for 39 如果${P_r}$中没有满足密钥率需求的密钥中继路径, 则该业务失败; 40 end for 41 return 每个成功的域间密钥业务的密钥中继路径${p_r}\left( {{N_{{p_r}}}, {L_{{p_r}}}, {B_{{p_r}}}, {m_{{p_r}}}} \right)$, ${R_{\text{S}}}$ 
下载: 导出CSV
表 2 密钥生成率仿真参数
Table 2. Simulation parameters for key rates.
参数 取值 真空态误码率${e_0}$ 0.5 本底误码${e_{\text{d}}}$/% 1 暗计数率${p_{\text{d}}}$ ${10^{ - 7}}$ 探测效率${\eta _{\text{d}}}$/% 40 纠错效率${f_{\text{e}}}$ 1.16 光纤衰减常数$\alpha $/(dB·km–1) 0.2 重复频率/GHz 1
下载: 导出CSV
-
[1] Yang Z, Zolanvari M, Jain R 2023 IEEE Commun. Surveys Tuts. 25 1059
Google Scholar
[2] Gill S S, Kumar A, Singh H, Singh M, Kaur K, Usman M, Buyya R 2022 Softw. Pract. Exp. 52 66
Google Scholar
[3] Lo H K, Curty M, Tamaki K 2014 Nat. Photon. 8 595
Google Scholar
[4] Pirandola S, Andersen U L, Banchi L, Berta M, Bunandar D, Colbeck R, Englund D, Gehring T, Lupo C, Ottaviani C, Pereira J L, Razavi M, Shamsul S J, Tomamichel M, Usenko V C, Vallone G, Villoresi P, Wallden P 2020 Adv. Opt. Photon. 12 1012
Google Scholar
[5] Bennett C H, Brassard G 1984 IEEE Int. Conf. Comput. Syst. Signal Process. Bangalore, India, January, 1984 p175
[6] Lo H K, Curty M, Qi B 2012 Phys. Rev. Lett. 108 130503
Google Scholar
[7] Li W, Zhang L K, Tan H, Lu Y C, Liao S K, Huang J, Li H, Wang Z, Mao H K, Yan B Z, Li Q, Liu Y, Zhang Q, Peng C Z, You L X, Xu F H, Pan J W 2023 Nat. Photon. 17 416
Google Scholar
[8] Yin H L, Fu Y, Li C L, Weng C X, Li B H, Gu J, Lu Y S, Huang S, Chen Z B 2023 Nat. Sci. Rev. 10 nwac228
Google Scholar
[9] Cao Y, Zhao Y, Wang Q, Zhang J, Ng S X, Hanzo L 2022 IEEE Commun. Surveys Tuts. 24 839
Google Scholar
[10] Tang Y L, Yin H L, Zhao Q, Liu H, Sun X X, Huang M Q, Zhang W J, Chen S J, Zhang L, You L X, Wang Z, Liu Yang, Lu C Y, Jiang X, Ma X F, Zhang Q, Chen T Y, Pan J W 2016 Phys. Rev. X 6 011024
Google Scholar
[11] Joshi S K, Aktas D, Wengerowsky S, Lončarić M, Neumann S P, Liu B, Scheidl T, Lorenzo G C, Samec Ž, Kling L, Qiu A, Razavi M, Stipčević M, Rarity J G, Ursin R 2020 Sci. Adv. 6 eaba0959
Google Scholar
[12] Avesani M, Foletto G, Padovan M, Calderaro L, Agnesi C, Bazzani E, Berra F, Bertapelle T, Picciariello F, Santagiustina F, Scalcon D, Scriminich A, Stanco A, Vedovato F, Vallone G, Villoresi P 2023 Quantum Computing, Communication, and Simulation III San Francisco, United States, 2023 p112
[13] Cao Y, Zhao Y L, Zhang J, Wang Q, Niyato D, Hanzo L 2022 IEEE Netw. 36 14
Google Scholar
[14] Cao Y, Zhao Y L, Zhang J, Wang Q 2022 IEEE Commun. Mag. 60 38
Google Scholar
[15] Zhou L, Lin J P, Xie Y M, Lu Y S, Jing Y M, Yin H L, Yuan Z L 2023 Phys. Rev. Lett. 130 250801
Google Scholar
[16] Fan-Yuan G J, Lu F Y, Wang S, Yin Z Q, He D Y, Zhou Z, Teng J, Chen W, Guo G C, Han Z F 2021 Photonics Res. 9 1881
Google Scholar
[17] Tysowski P K, Ling X, Lütkenhaus N, Mosca M 2018 Quantum Sci. Technol. 3 024001
Google Scholar
[18] Li P, Yu X, Zhao Y, Zhang J 2023 Opto-Electronic and Communications Conference Shanghai, China, July 2–6, 2023 p1
[19] Gottesman D, Lo H K, Lutkenhaus N, Preskill J 2004 Quantum Inf. Comput. 4 325
Google Scholar
[20] Ma X F, Qi B, Zhao Y, Lo H K 2005 Phys. Rev. A 72 012326
Google Scholar
[21] Xu F H, Xu H, Lo H K 2014 Phys. Rev. A 89 052333
Google Scholar
[22] Ma X F, Fung C H F, Razavi M 2012 Phys. Rev. A 86 052305
Google Scholar
[23] Wang X B 2013 Phys. Rev. A 87 012320
Google Scholar
[24] Yu Z W, Zhou Y H, Wang X B 2013 Phys. Rev. A 88 062339
Google Scholar
[25] Curty M, Xu F, Cui W, Lim C C W, Tamaki K, Lo H K 2014 Nat. Commun. 5 3732
Google Scholar
[26] Wang Q, Wang X B 2014 Sci. Rep. 4 4612
Google Scholar
[27] Zhou Y H, Yu Z W, Wang X B 2016 Phys. Rev. A 93 042324
Google Scholar
下载:
计量
- 文章访问数: 733
- PDF下载量: 39
- 被引次数: 0
