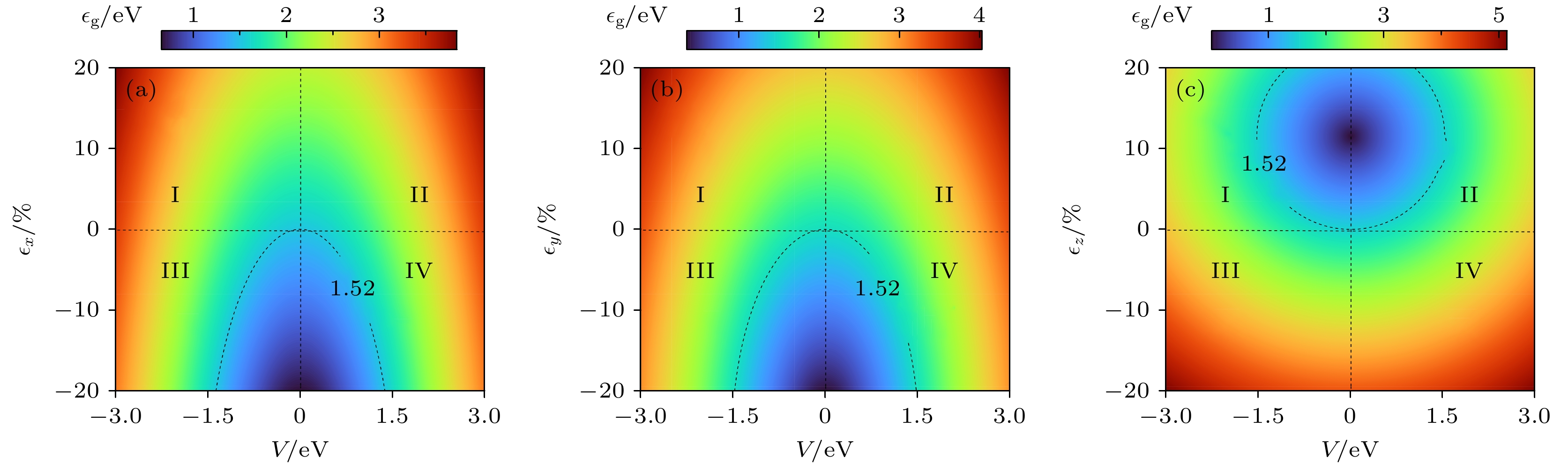
-
黑磷烯具有各向异性且独特的光电性能被广泛研究. 应变、电压等常用来调制能带结构, 进而调制其光电特性. 本文采用紧束缚近似哈密顿量, 考虑施加垂直磁场、电场、面内面外应变条件下的黑磷烯能带结构, 进一步利用Kubo公式研究了黑磷烯光电导率在多个调制因子下的特征, 并从能带结构进行了机理分析. 垂直磁场使能带劈裂, 产生多通道带间跃迁, 光电导率表现出多个峰. 随着面内拉伸应变增加能隙增加, 光电导峰位依赖于能隙. 而面外拉伸应变对能隙的调制区别于面内应变, 能隙表现出非单调变化. 电场通过带隙的变化, 调制光电导率峰值位置. 综合不同的调制因子, 能带和光电导表现出丰富的调制效果, 为研究基于黑磷烯光电器件的应用提供理论支持.Black phosphorene (BP) has been widely investigated for its anisotropic and unique photoelectric properties. Strain, voltage and so on are commonly used to modulate the energy band structure and accordingly its photoelectric characteristics. In this study, we consider the energy band structure of BP in the vertical magnetic field, electric field, and in-plane/out-of-plane strains by using the tight-binding approximate Hamiltonian. The anisotropic frequency-dependent interband optical conductivity (IOC) of BP is investigated by using the Kubo formula in these modulation factors. Inherent asymmetry in band dispersion along the armchair (AC) direction and the zigzag (ZZ) direction leads to anisotropic IOC. The introduction of a vertical magnetic field induces band splitting, thereby generating multiple interband transition channels. In this case, the IOC along both the AC direction and the ZZ direction exhibits three peaks around the original peak position, and the magnitudes of the peaks are also modulated. With the increase of in-plane strain (from –20% to 20%), the band gap increases monotonically, and both the position and magnitude of the peaks vary with band gap changing. However, the band gap of BP undergoes a non-monotonic change under out-of-plane strain (from –20% to 20%), which is different from the change under in-plane strain. The band gap reaches a minimum value when a tensile strain of 12% is applied. Along the AC direction, the modulation of the IOC by in-plane strain is opposite to the modulation of out-of-plane strain (εz < 12%), indicating a competitive effect when triaxial strains are applied. Along the ZZ direction, in-plane strain primarily modulates the peak magnitude, while out-of-plane strain effectively modulates not only the peak position but also the peak magnitude obviously. The modulation of the IOC by forward and reverse electric fields are symmetrical. The coefficient for the peak position shift due to the vertical electric field is 1/2 in the AC direction and 1/10 in the ZZ direction. By integrating various modulation factors, we achieve versatile control over the energy band and IOC of BP, providing theoretical support for the application of BP in optoelectronic devices.
-
Keywords:
- black phosphorene /
- band modulation /
- anisotropy /
- optical conductivity
[1] Novoselov K S, Geim A K, Morozov S V, et al. 2004 Science 306 666
Google Scholar
[2] Manzeli S, Ovchinnikov D, Pasquier D, Yazyev O V, Kis A 2017 Nat. Rev. Mater. 2 17033
Google Scholar
[3] Li L K, Yu Y J, Ye G J, Ge Q Q, Ou X D, Wu H, Feng D L, Chen X H, Zhang Y B 2014 Nat. Nanotechnol. 9 372
Google Scholar
[4] Liu H, Neal A T, Zhu Z, Luo Z, Xu X F, Tománek D, Ye P D 2014 ACS Nano 8 4033
Google Scholar
[5] Xia F N, Wang H, Jia Y C 2014 Nat. Commun. 5 4458
Google Scholar
[6] Jain A, McGaughey A J H 2015 Sci. Rep. 5 8501
Google Scholar
[7] Qiao J S, Kong X H, Hu Z X, Yang F, Ji W 2014 Nat. Commun. 5 4475
Google Scholar
[8] Ezawa M 2014 New J. Phys. 16 115004
Google Scholar
[9] Tran V, Soklaski R, Liang Y F, Yang L 2014 Phys. Rev. B 89 235319
Google Scholar
[10] Li L K, Kim J, Jin C H, et al. 2017 Nat. Nanotechnol. 12 21
Google Scholar
[11] Kim J, Baik S S, Ryu S H, Sohn Y, Park S, Park B G, Denlinger J, Yi Y, Choi H J, Kim K S 2015 Science 349 723
Google Scholar
[12] Peng X H, Wei Q, Copple A 2014 Phys. Rev. B 90 085402
Google Scholar
[13] Rodin A S, Carvalho A, Castro Neto A H 2014 Phys. Rev. Lett. 112 176801
Google Scholar
[14] Fei R, Yang L 2014 Nano Lett. 14 2884
Google Scholar
[15] Dai J, Zeng X C 2014 J. Phys. Chem. Lett. 5 1289
Google Scholar
[16] Chen X L, Lu X B, Deng B C, et al. 2017 Nat. Commun. 8 1672
Google Scholar
[17] Li L K, Yang F Y, Ye G J, et al. 2016 Nat. Nanotechnol. 11 593
Google Scholar
[18] Pereira Jr J M, Katsnelson M I 2015 Phys. Rev. B 92 075437
Google Scholar
[19] Zhou X Y, Lou W K, Zhai F, Chang K 2015 Phys. Rev. B 92 165405
Google Scholar
[20] Phuong L T T, Phong T C, Yarmohammadi M 2020 Sci. Rep. 10 9201
Google Scholar
[21] Keshtan M A M, Esmaeilzadeh M 2015 J. Phys. D: Appl. Phys. 48 485301
Google Scholar
[22] Wang Y, Guo Y L, Wang Z K, et al. 2021 ACS Nano 15 12069
Google Scholar
[23] Wang Y, Xu W, Fu L, et al. 2023 ACS Appl. Mater. Interfaces 15 54797
Google Scholar
[24] Wang Y, Xu W, Yang D Y, et al. 2023 ACS Nano 17 24320
Google Scholar
[25] Li P K, Appelbaum I 2014 Phys. Rev. B 90 115439
Google Scholar
[26] Le P T T, Yarmohammadi M 2019 J. Magn. Magn. Mater. 491 165629
Google Scholar
[27] Rudenko A N, Katsnelson M I 2014 Phys. Rev. B 89 201408
Google Scholar
[28] Yang C H, Zhang J Y, Wang G X, Zhang C 2018 Phys. Rev. B 97 245408
Google Scholar
[29] Jiang J W, Park H S 2015 Phys. Rev. B 91 235118
Google Scholar
[30] Khang P D, Davoudiniya M, Phuong L T T, Phong T C, Yarmohammadi M 2019 Phys. Chem. Chem. Phys. 21 15133
Google Scholar
[31] Yang C H, Zhang J Y, Wieser R, Xu W 2022 J. Phys. D: Appl. Phys. 55 085103
Google Scholar
[32] Le P T T, Mirabbaszadeh K, Yarmohammadi M 2019 J. Appl. Phys. 125 193101
Google Scholar
-
图 1 黑磷烯晶格结构示意图 (a) 三维; (b) 二维
Fig. 1. Schematic diagram of lattice structure of BP: (a) Three-dimensional; (b) two-dimensional.
图 2 黑磷烯能带示意图 (a) 磁场下; (b) 电场下; (c) x轴应变下
Fig. 2. Energy band structure of BP in the presence: (a) Magnetic field; (b) electric field; (c) x-axis strain.
图 3 同时施加磁场和应变下的黑磷烯带隙(虚线1.52对应的是本征带隙的位置) (a) 施加x轴应变 ; (b) 施加y轴应变; (c) 施加z轴应变
Fig. 3. Bandgap of BP in the presence of the magnetic field and strain applied along (Dashed line corresponds to the position of the intrinsic band gap): (a) x-axis; (b) y-axis; (c) z-axis.
图 4 同时施加电场和应变下的黑磷烯带隙(虚线1.52对应的是本征带隙的位置) (a) 施加x轴应变; (b) 施加y轴应变; (c) 施加z轴应变
Fig. 4. Bandgap of BP in the presence of the electric field and strain applied along (Dashed line corresponds to the position of the intrinsic band gap): (a) x-axis; (b) y-axis; (c) z-axis.
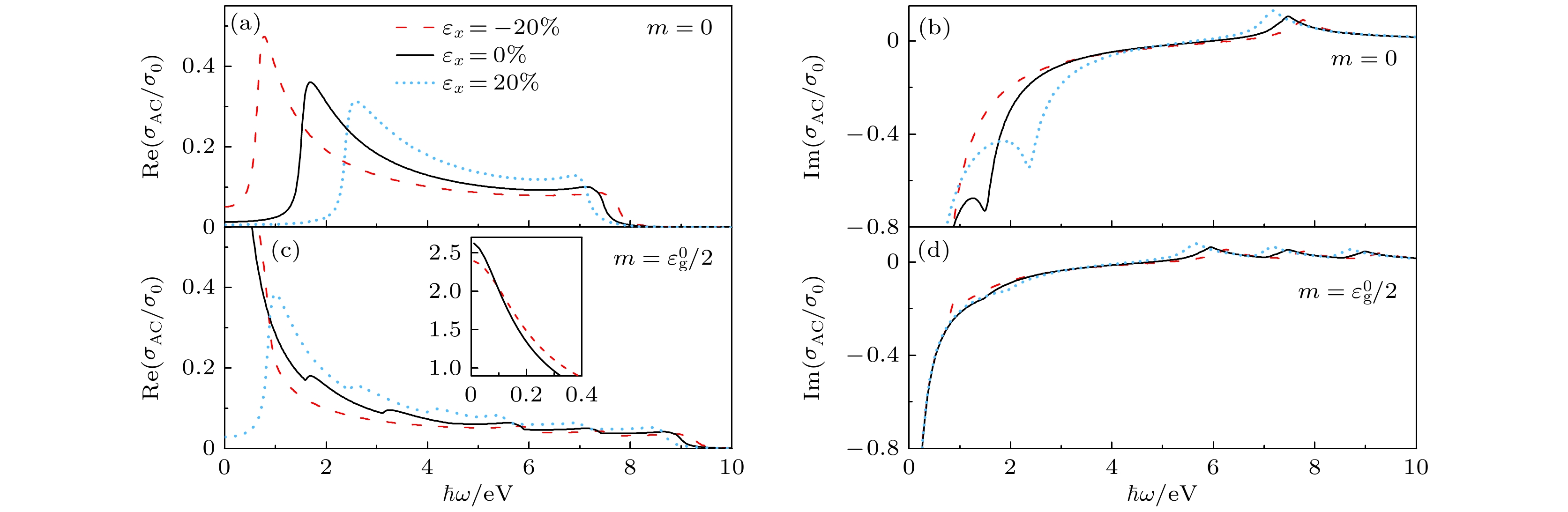
图 5 AC方向的带间光电导率在不同x轴应变条件下随入射光能量的变化 (a), (c) 不同磁场下的光电导实部; (b), (d) 不同磁场下的光电导虚部. 黑色、红色和蓝色曲线分别表示无应变、压缩应变和拉伸应变下的结果
Fig. 5. Interband optical conductivity along the AC direction as a function of the incident photon energy at different x-axial strain: (a), (c) Real part under different magnetic fields; (b), (d) the imaginary part under different magnetic fields. Black, red, and blue curves represent the results with no strain, compressive strain, and tensile strain, respectively.
图 8 带间光电导率实部在不同z轴应变条件下随入射光能量的变化 (a), (c)不同磁场下AC方向的结果; (b), (d)不同磁场下ZZ方向的结果
Fig. 8. Real part of the interband optical conductivity as a function of the incident photon energy at different z-axial strain: (a), (c) Results along the AC direction under different magnetic fields; (b), (d) results along the ZZ direction under different magnetic fields.
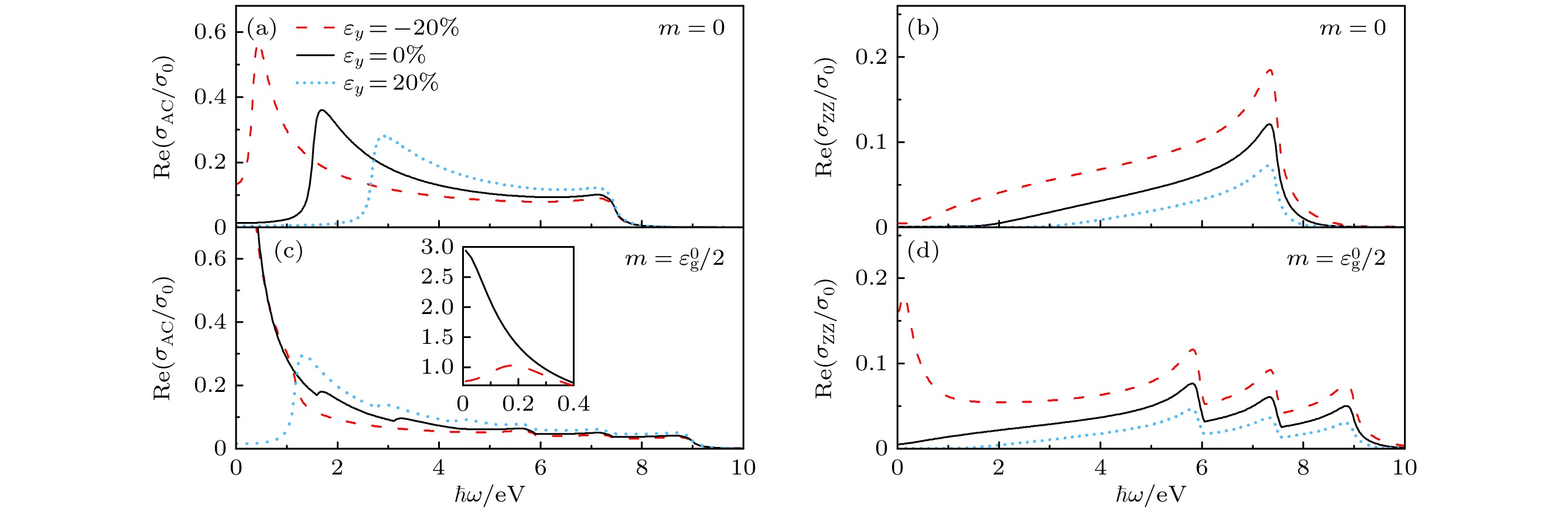
图 7 带间光电导率实部在不同y轴应变条件下随入射光能量的变化 (a), (c) 不同磁场下AC方向的结果; (b), (d)不同磁场下ZZ方向的结果
Fig. 7. Real part of the interband optical conductivity as a function of the incident photon energy at different y-axial strain: (a), (c) Results along the AC direction under different magnetic fields; (b), (d) the results along the ZZ direction under different magnetic fields.
图 6 ZZ方向的带间光电导率在不同x轴应变条件下随入射光能量的变化 (a), (c) 不同磁场下的光电导实部; (b), (d) 不同磁场下的光电导虚部
Fig. 6. Interband optical conductivity along the ZZ direction as a function of the incident photon energy at different x-axial strain: (a), (c) Real part under different magnetic fields; (b), (d) the imaginary part under different magnetic fields.
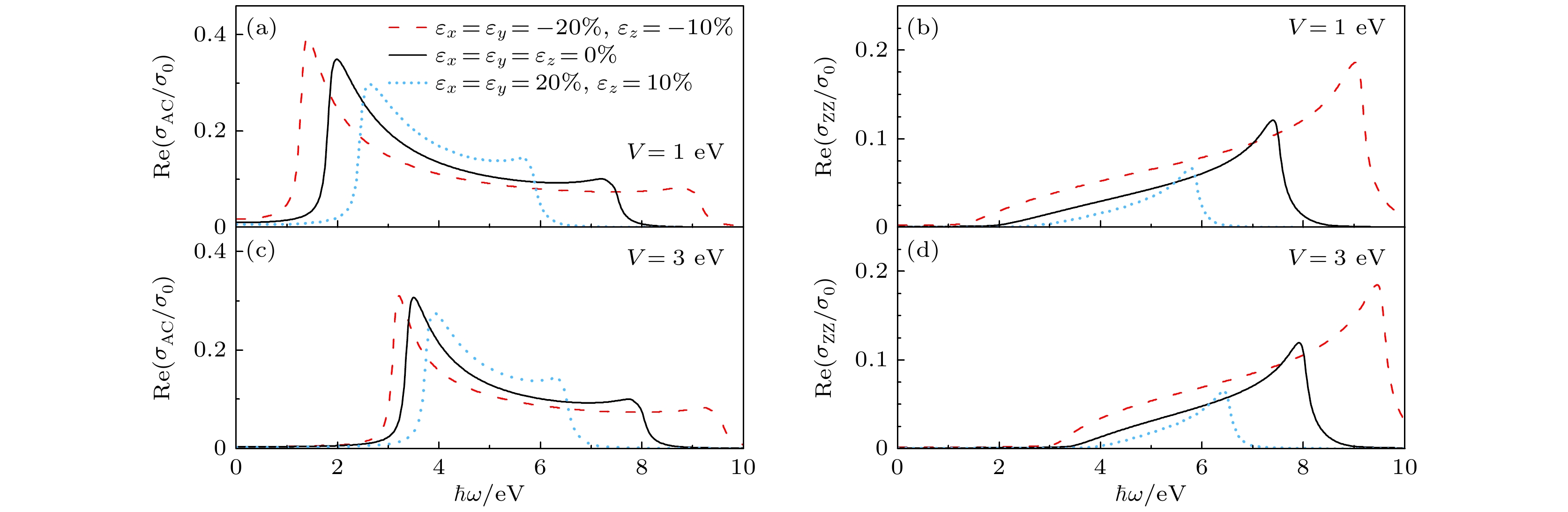
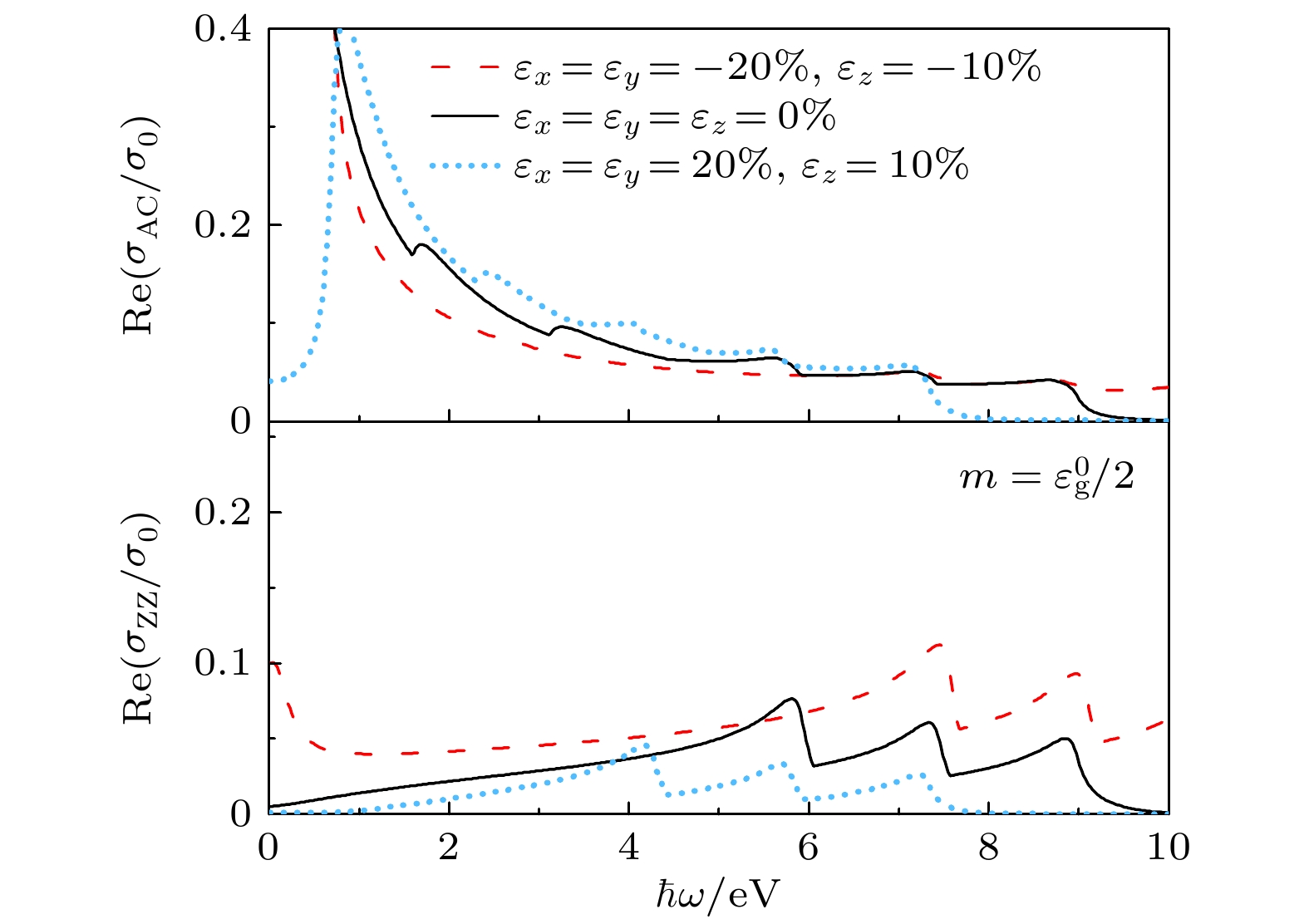
图 9 带间光电导率实部在三轴应变条件下随入射光能量的变化 (a), (c)不同磁场下AC方向的结果; (b), (d)不同磁场下ZZ方向的结果
Fig. 9. Real part of the interband optical conductivity as a function of the incident photon energy at different triaxial strains: (a), (c) Results along the AC direction under different magnetic fields; (b), (d) the results along the ZZ direction under different magnetic fields.
图 10 带间光电导率实部在三轴应变条件下随入射光能量的变化 (a), (c) 不同电场下AC方向的结果; (b), (d) 不同电场下ZZ方向的结果
Fig. 10. Real part of the interband optical conductivity as a function of the incident photon energy at different triaxial strains: (a), (c) Results along the AC direction under different electric fields; (b), (d) the results along the ZZ direction under different electric fields.
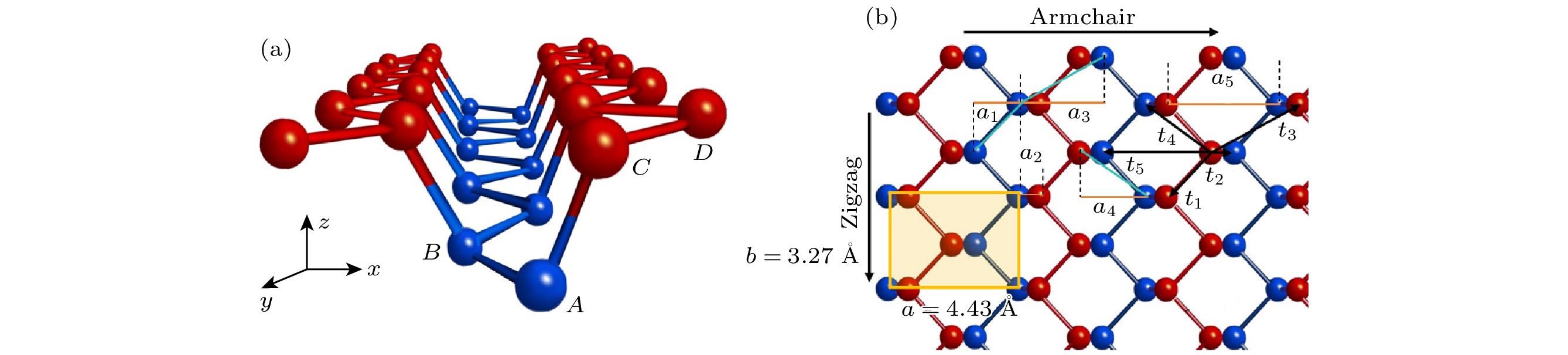
ti 具体数值/eV ai 具体数值/Å t1 –1.22 a1 1.41763 t2 3.665 a2 0.79732 t3 –0.205 a3 3.01227 t4 –0.105 a4 2.21468 t5 –0.055 a5 3.63258 
下载: 导出CSV
γ $ \alpha _{1}^\gamma $ $ \alpha _{2}^\gamma $ $ \alpha _{3}^\gamma $ $ \alpha _{4}^\gamma $ $ \alpha _{5}^\gamma $ x 0.4460 0.0992 0.7505 0.3976 0.7530 y 0.5571 0 0.2461 0.2280 0 z 0 0.9052 0 0.3722 0.2538
下载: 导出CSV
-
[1] Novoselov K S, Geim A K, Morozov S V, et al. 2004 Science 306 666
Google Scholar
[2] Manzeli S, Ovchinnikov D, Pasquier D, Yazyev O V, Kis A 2017 Nat. Rev. Mater. 2 17033
Google Scholar
[3] Li L K, Yu Y J, Ye G J, Ge Q Q, Ou X D, Wu H, Feng D L, Chen X H, Zhang Y B 2014 Nat. Nanotechnol. 9 372
Google Scholar
[4] Liu H, Neal A T, Zhu Z, Luo Z, Xu X F, Tománek D, Ye P D 2014 ACS Nano 8 4033
Google Scholar
[5] Xia F N, Wang H, Jia Y C 2014 Nat. Commun. 5 4458
Google Scholar
[6] Jain A, McGaughey A J H 2015 Sci. Rep. 5 8501
Google Scholar
[7] Qiao J S, Kong X H, Hu Z X, Yang F, Ji W 2014 Nat. Commun. 5 4475
Google Scholar
[8] Ezawa M 2014 New J. Phys. 16 115004
Google Scholar
[9] Tran V, Soklaski R, Liang Y F, Yang L 2014 Phys. Rev. B 89 235319
Google Scholar
[10] Li L K, Kim J, Jin C H, et al. 2017 Nat. Nanotechnol. 12 21
Google Scholar
[11] Kim J, Baik S S, Ryu S H, Sohn Y, Park S, Park B G, Denlinger J, Yi Y, Choi H J, Kim K S 2015 Science 349 723
Google Scholar
[12] Peng X H, Wei Q, Copple A 2014 Phys. Rev. B 90 085402
Google Scholar
[13] Rodin A S, Carvalho A, Castro Neto A H 2014 Phys. Rev. Lett. 112 176801
Google Scholar
[14] Fei R, Yang L 2014 Nano Lett. 14 2884
Google Scholar
[15] Dai J, Zeng X C 2014 J. Phys. Chem. Lett. 5 1289
Google Scholar
[16] Chen X L, Lu X B, Deng B C, et al. 2017 Nat. Commun. 8 1672
Google Scholar
[17] Li L K, Yang F Y, Ye G J, et al. 2016 Nat. Nanotechnol. 11 593
Google Scholar
[18] Pereira Jr J M, Katsnelson M I 2015 Phys. Rev. B 92 075437
Google Scholar
[19] Zhou X Y, Lou W K, Zhai F, Chang K 2015 Phys. Rev. B 92 165405
Google Scholar
[20] Phuong L T T, Phong T C, Yarmohammadi M 2020 Sci. Rep. 10 9201
Google Scholar
[21] Keshtan M A M, Esmaeilzadeh M 2015 J. Phys. D: Appl. Phys. 48 485301
Google Scholar
[22] Wang Y, Guo Y L, Wang Z K, et al. 2021 ACS Nano 15 12069
Google Scholar
[23] Wang Y, Xu W, Fu L, et al. 2023 ACS Appl. Mater. Interfaces 15 54797
Google Scholar
[24] Wang Y, Xu W, Yang D Y, et al. 2023 ACS Nano 17 24320
Google Scholar
[25] Li P K, Appelbaum I 2014 Phys. Rev. B 90 115439
Google Scholar
[26] Le P T T, Yarmohammadi M 2019 J. Magn. Magn. Mater. 491 165629
Google Scholar
[27] Rudenko A N, Katsnelson M I 2014 Phys. Rev. B 89 201408
Google Scholar
[28] Yang C H, Zhang J Y, Wang G X, Zhang C 2018 Phys. Rev. B 97 245408
Google Scholar
[29] Jiang J W, Park H S 2015 Phys. Rev. B 91 235118
Google Scholar
[30] Khang P D, Davoudiniya M, Phuong L T T, Phong T C, Yarmohammadi M 2019 Phys. Chem. Chem. Phys. 21 15133
Google Scholar
[31] Yang C H, Zhang J Y, Wieser R, Xu W 2022 J. Phys. D: Appl. Phys. 55 085103
Google Scholar
[32] Le P T T, Mirabbaszadeh K, Yarmohammadi M 2019 J. Appl. Phys. 125 193101
Google Scholar
下载:
计量
- 文章访问数: 1193
- PDF下载量: 38
- 被引次数: 0
