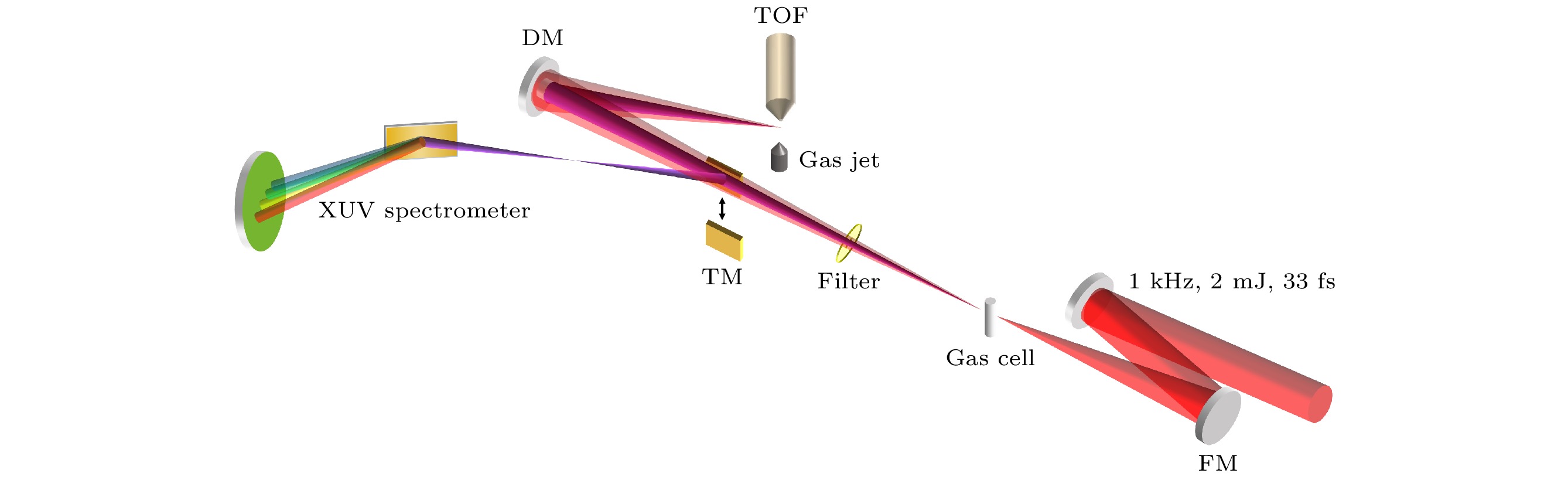
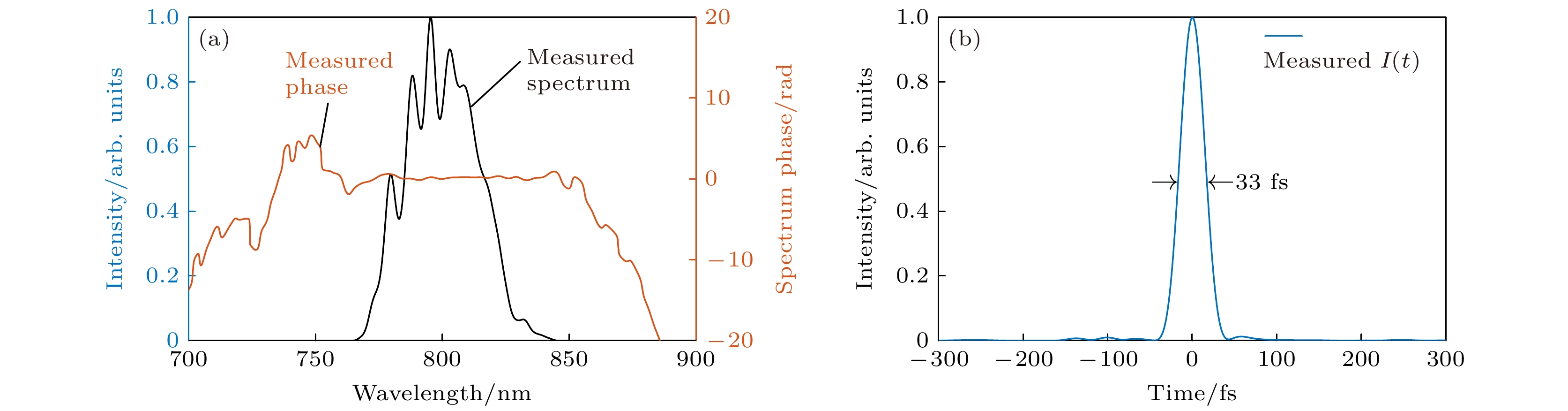
-
Attosecond pulses provide higher measurement precision for analyzing ultrafast dynamics in atoms, molecules, and electrons, laying the foundation for studying electronic motion in atomic and molecular systems. The most important method currently is to generate attosecond pulse trains and isolated attosecond pulses through the interaction of femtosecond lasers with gases. The temporal information of attosecond pulses and the dynamic information of electrons can be extracted from spectrograms by using attosecond streak camera or the reconstruction of attosecond beating by interference of two-photon transitions (RABBITT) method based on two-photon transition interference. Although the differences in phase among different high-order harmonics can be directly extracted from the oscillation frequencies of sidebands, the iterative algorithm of attosecond streak camera can provide complete phase information of attosecond pulse trains to better support the study of electron dynamics in atoms. Research purpose This work is dedicated to the investigation of the generation, measurement, and characterization of attosecond pulse train (APT), which are essential for probing ultrafast dynamics in atomic, molecular, and electronic systems. The focus is on the generation of APTs through interactions between femtosecond lasers and gases, as well as the extraction of temporal and dynamic information from these pulses by using advanced spectroscopic techniques such as the RABITT method. Methods The experimental approach involves the use of a homebuilt femtosecond titanium sapphire regenerative amplifier to produce high-order harmonics, leading to the generation of APTs. The setup includes the homebuilt titanium sapphire chirped pulse amplifier and a collinear attosecond pulse generation and measurement beamline, which are used to conduct RABITT experiments. The process requires the interaction of femtosecond lasers with gas targets to generate high-energy photons in the extreme ultraviolet and soft X-ray spectral ranges. By optimizing the phase-matching conditions within the gas target, strong high-order harmonic signals are observed on an XUV spectrometer. The temporal information of the attosecond pulses is indirectly measured through the photoelectron spectrum produced by the interaction of attosecond pulses with femtosecond lasers. The research also employs the FROG-CRAB algorithm and the extended phase retrieval and iterative engine (ePIE) algorithm for temporally reconstructing APTs and attempts to use a genetic algorithm to extract phase information. Results The study yields three sets of RABITT spectrograms, which are analyzed by using the RABITT sideband phase method to directly reconstruct APTs. Fourier transform analysis is used to extract phase differences between sidebands, offering insights into the phase differences between corresponding high-order harmonics. This method, however, provides an estimation of the phase in the center of each harmonic order, which does not fully represent the actual pulse shape. The FROG-CRAB algorithm and ePIE algorithm successfully reconstructs the attosecond pulse trains from the RABITT spectrograms, revealing similar temporal pulse train morphologies. In contrast, the genetic algorithm, despite its potential for high constraint optimization, does not yield satisfactory results, possibly due to the sensitivity of the algorithm to discrepancies between theoretical simulations and experimental data. Conclusions The research concludes that achieving ideal inversion results for APTs necessitates small time delay steps and a wide scanning range in the experimental data collection process to ensure a rich dataset for inversion. The FROG-CRAB algorithm and ePIE algorithm demonstrate their effective performance in reconstructing APTs, with ePIE showing higher computational efficiency. The genetic algorithm, while offering a high degree of constraint, faces challenges and requires to be further refined. The study underscores the importance of the signal-to-noise ratio in experimental data for the accuracy of inversion results. This work provides significant guidance for future measuring electron dynamics and explaining their evolution patterns, contributing valuable experimental methods and data analysis techniques to the field of attosecond science. -
Keywords:
- attosecond pulse trains /
- phase reconstruction /
- RABBITT method /
- attosecond streaking camera
[1] Borrego-Varillas R, Lucchini M, Nisoli M 2022 Rep. Prog. Phys. 85 066401
Google Scholar
[2] Zholents A, Zolotorev M 2008 New J. Phys. 10 025005
Google Scholar
[3] Nees J, Naumova N, Power E, Yanovsky V, Sokolov I, Maksimchuk A, Bahk S W, Chvykov V, Kalintchenko G, Hou B 2005 J. Mod. Opt. 52 305
Google Scholar
[4] Kaplan A 1994 Phys. Rev. Lett. 73 1243
Google Scholar
[5] McPherson A, Gibson G, Jara H, Johann U, Luk T S, McIntyre I, Boyer K, Rhodes C K 1987 JOSA B 4 595
Google Scholar
[6] Ferray M, L'Huillier A, Li X, Lompre L, Mainfray G, Manus C 1988 J. Phys. B: At. Mol. Opt. Phys. 21 L31
Google Scholar
[7] Chini M, Zhao K, Chang Z 2014 Nat. Photonics 8 178
Google Scholar
[8] Krausz F, Ivanov M 2009 Rev. Mod. Phys. 81 163
Google Scholar
[9] Antoine P, L'huillier A, Lewenstein M 1996 Phys. Rev. Lett. 77 1234
Google Scholar
[10] Sansone G, Benedetti E, Calegari F, Vozzi C, Avaldi L, Flammini R, Poletto L, Villoresi P, Altucci C, Velotta R 2006 Science 314 443
Google Scholar
[11] Hentschel M, Kienberger R, Spielmann C, Reider G A, Milosevic N, Brabec T, Corkum P, Heinzmann U, Drescher M, Krausz F 2001 Nature 414 509
Google Scholar
[12] Itatani J, Quéré F, Yudin G L, Ivanov M Y, Krausz F, Corkum P B 2002 Phys. Rev. Lett. 88 173903
Google Scholar
[13] Muller H G 2002 Appl. Phys. B 74 s17
Google Scholar
[14] Paul P M, Toma E S, Breger P, Mullot G, Augé F, Balcou P, Muller H G, Agostini P 2001 Science 292 1689
Google Scholar
[15] Mairesse Y, Quéré F 2005 Phys. Rev. A 71 011401
Google Scholar
[16] Gagnon J, Goulielmakis E, Yakovlev V S 2008 Appl. Phys. B 92 25
Google Scholar
[17] Chini M, Gilbertson S, Khan S D, Chang Z 2010 Opt. Express 18 13006
Google Scholar
[18] Zhao X, Wei H, Wu Y, Lin C D 2017 Phys. Rev. A 95 043407
Google Scholar
[19] Keathley P D, Bhardwaj S, Moses J, Laurent G, Kaertner F X 2016 New J. Phys. 18 073009
Google Scholar
[20] Månsson E P, Guénot D, Arnold C L, Kroon D, Kasper S, Dahlström J M, Lindroth E, Kheifets A S, L’huillier A, Sorensen S L 2014 Nat. Phys. 10 207
Google Scholar
[21] Jordan I, Huppert M, Pabst S, Kheifets A S, Baykusheva D, Wörner H J 2017 Phys. Rev. A 95 013404
Google Scholar
[22] Kotur M, Guenot D, Jiménez-Galán Á, Kroon D, Larsen E W, Louisy M, Bengtsson S, Miranda M, Mauritsson J, Arnold C 2016 Nat. Commun. 7 10566
Google Scholar
[23] Haessler S, Fabre B, Higuet J, Caillat J, Ruchon T, Breger P, Carré B, Constant E, Maquet A, Mével E 2009 Phys. Rev. A 80 011404
Google Scholar
[24] Klünder K, Dahlström J, Gisselbrecht M, Fordell T, Swoboda M, Guenot D, Johnsson P, Caillat J, Mauritsson J, Maquet A 2011 Phys. Rev. Lett. 106 143002
Google Scholar
[25] Zhan M J, Ye P, Teng H, He X K, Zhang W, Zhong S Y, Wang L F, Yun C X, Wei Z Y 2013 Chin. Phys. Lett. 30 093201
Google Scholar
[26] Jiang Y J, Liang Y Y, Gao Y T, Zhao K, Xu S Y, Wang J, He X K, Teng H, Zhu J F, Chen Y L, Wei Z Y 2020 Chin. Phys. B 29 013206
Google Scholar
[27] Zhong S Y, Teng H, Zhu X X, Gao Y T, Wang K J, Wang X Z, Wang Y M, Yu S, Zhao K, Wei Z Y 2023 Chin. Opt. Lett. 21 113201
Google Scholar
[28] Lucchini M, Brügmann M, Ludwig A, Gallmann L, Keller U, Feurer T 2015 Opt. Express 23 29502
Google Scholar
[29] Kheifets A S, Bray A W 2021 Phys. Rev. A 103 L011101
Google Scholar
[30] Cattaneo L, Vos J, Lucchini M, Gallmann L, Cirelli C, Keller U 2016 Opt. Express 24 29060
Google Scholar
-
图 1 Wizzler测量再生放大器输出光谱(a)及脉宽图(b)
Fig. 1. Spectrum (a) and pulse width (b) of the regenerative amplifier output measured by Wizzler.
图 2 阿秒脉冲串产生测量实验装置图
Fig. 2. Diagram of the experimental setup for attosecond pulse train generation measurement.
图 3 实验测到的3组RABBITT数据(a1), (b1), (c1)以及使用边带相位方法重建的阿秒脉冲串(a2), (b2), (c2), 图中标注了脉宽
Fig. 3. Experimental data of three sets of RABBITT (a1), (b1), (c1) and the reconstructed attosecond pulse train using the sideband phase method (a2), (b2), (c2), with the pulse width labeled.
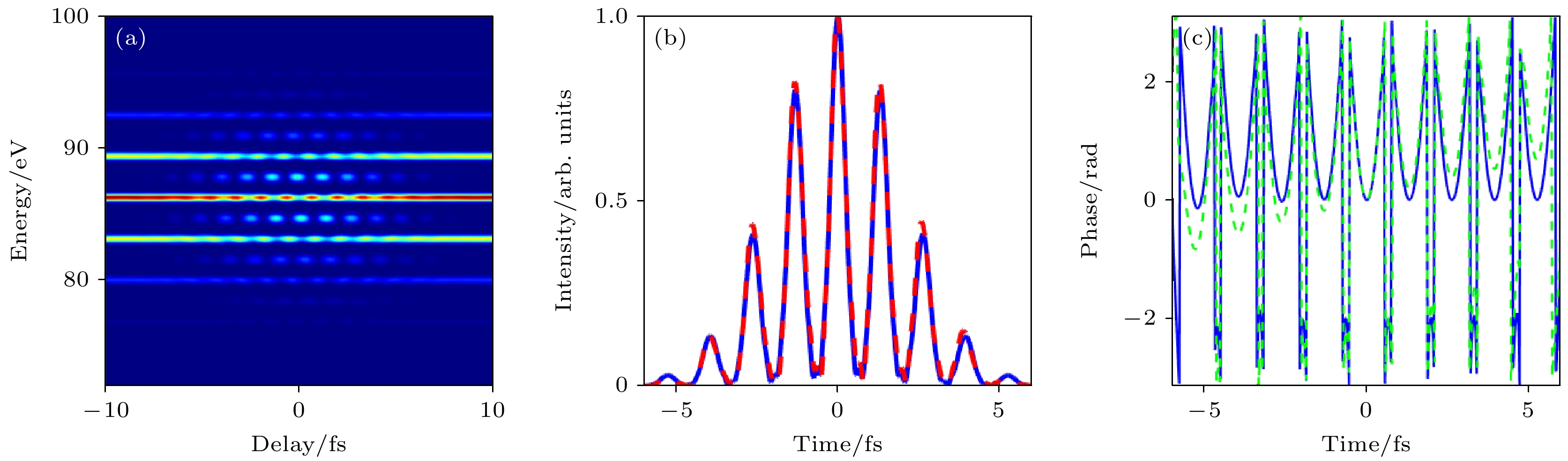
图 4 FROG-CRAB反演理论模拟的RABBITT能谱图重建阿秒脉冲串结果 (a)模拟的能谱图; (b), (c)中蓝线表示理论产生的阿秒脉冲串和相位, (b)中红线表示FROG-CRAB通过反演重建的阿秒脉冲串, (c)中绿线代表FROG-CRAB在RABBITT能谱图中提取到的相位
Fig. 4. Reconstruction results of RABBITT spectrogram using FROG-CRAB. The simulated spectrogram is displayed in panel (a). The blue lines in panel (b) and (c) represent the theoretically generated attosecond pulse train and phase, respectively. The red line in panel (b) indicates the attosecond pulse train reconstructed by FROG-CRAB, and the green line in panel (c) represents the phase extracted by FROG-CRAB from the RABBITT spectrogram.
图 5 FROG-CRAB反演实验RABBITT能谱图重建阿秒脉冲串结果 (a1), (b1), (c1)实验测到的3组RABBIT能谱图; (a2), (b2), (c2)根据反演脉冲重建的能谱图; (a3), (b3), (c3)反演得到的时域阿秒脉冲串和相位
Fig. 5. Results of reconstructing attosecond pulse trains using FROG-CRAB from RABBITT spectrogram: (a1), (b1), (c1) Three sets of RABBITT spectrograms measured in the experiment; (a2), (b2), (c2) the reconstructed spectrograms based on the inverted pulses; (a3), (b3), (c3) the time-domain attosecond pulse trains and phases obtained from the inversion.
图 6 ePIE反演理论模拟的RABBITT能谱图重建阿秒脉冲串结果 (a)模拟的能谱图; (b), (c)蓝线表示理论产生的阿秒脉冲串和相位, (b)中红线表示ePIE通过反演重建的阿秒脉冲串, (c)中绿线代表ePIE在RABBITT能谱图中提取到的相位
Fig. 6. Reconstruction results of RABBITT spectrogram using ePIE. The simulated spectrogram is displayed in panel (a). (b), (c) The blue lines represent the theoretically generated attosecond pulse train and phase, respectively. The red line in panel (b) indicates the attosecond pulse train reconstructed by ePIE, and the green line in panel (c) represents the phase extracted by ePIE from the RABBITT spectrogram.
图 7 ePIE反演实验RABBITT能谱图重建阿秒脉冲串结果 (a1), (b1), (c1)实验测到的3组RABBIT能谱图; (a2), (b2), (c2)根据反演脉冲重建的能谱图; (a3), (b3), (c3)反演得到的时域阿秒脉冲串和相位
Fig. 7. Results of reconstructing attosecond pulse trains using ePIE from RABBITT spectrogram: (a1), (b1), (c1) Three sets of RABBITT spectrograms measured in the experiment; (a2), (b2), (c2) the reconstructed spectrograms based on the inverted pulses; (a3), (b3), (c3) the time-domain attosecond pulse trains and phases obtained from the inversion.
图 8 遗传算法反演第3组实验RABBITT能谱图重建阿秒脉冲串结果 (a)第3组实验的能谱图; (b)遗传算法反演的能谱图; (c)遗传算法反演的时域脉冲串; (d)遗传算法在RABBITT能谱图中提取到的相位
Fig. 8. Results of reconstructing the attosecond pulse train of the third experimental RABBITT spectrogram using genetic algorithm: (a) The spectrogram of the third experimental RABBITT spectrogram; (b) the spectrogram inverted by the genetic algorithm; (c) the time-domain pulse train inverted by the genetic algorithm; (d) the phase extracted by the genetic algorithm from the RABBITT spectrogram.
-
[1] Borrego-Varillas R, Lucchini M, Nisoli M 2022 Rep. Prog. Phys. 85 066401
Google Scholar
[2] Zholents A, Zolotorev M 2008 New J. Phys. 10 025005
Google Scholar
[3] Nees J, Naumova N, Power E, Yanovsky V, Sokolov I, Maksimchuk A, Bahk S W, Chvykov V, Kalintchenko G, Hou B 2005 J. Mod. Opt. 52 305
Google Scholar
[4] Kaplan A 1994 Phys. Rev. Lett. 73 1243
Google Scholar
[5] McPherson A, Gibson G, Jara H, Johann U, Luk T S, McIntyre I, Boyer K, Rhodes C K 1987 JOSA B 4 595
Google Scholar
[6] Ferray M, L'Huillier A, Li X, Lompre L, Mainfray G, Manus C 1988 J. Phys. B: At. Mol. Opt. Phys. 21 L31
Google Scholar
[7] Chini M, Zhao K, Chang Z 2014 Nat. Photonics 8 178
Google Scholar
[8] Krausz F, Ivanov M 2009 Rev. Mod. Phys. 81 163
Google Scholar
[9] Antoine P, L'huillier A, Lewenstein M 1996 Phys. Rev. Lett. 77 1234
Google Scholar
[10] Sansone G, Benedetti E, Calegari F, Vozzi C, Avaldi L, Flammini R, Poletto L, Villoresi P, Altucci C, Velotta R 2006 Science 314 443
Google Scholar
[11] Hentschel M, Kienberger R, Spielmann C, Reider G A, Milosevic N, Brabec T, Corkum P, Heinzmann U, Drescher M, Krausz F 2001 Nature 414 509
Google Scholar
[12] Itatani J, Quéré F, Yudin G L, Ivanov M Y, Krausz F, Corkum P B 2002 Phys. Rev. Lett. 88 173903
Google Scholar
[13] Muller H G 2002 Appl. Phys. B 74 s17
Google Scholar
[14] Paul P M, Toma E S, Breger P, Mullot G, Augé F, Balcou P, Muller H G, Agostini P 2001 Science 292 1689
Google Scholar
[15] Mairesse Y, Quéré F 2005 Phys. Rev. A 71 011401
Google Scholar
[16] Gagnon J, Goulielmakis E, Yakovlev V S 2008 Appl. Phys. B 92 25
Google Scholar
[17] Chini M, Gilbertson S, Khan S D, Chang Z 2010 Opt. Express 18 13006
Google Scholar
[18] Zhao X, Wei H, Wu Y, Lin C D 2017 Phys. Rev. A 95 043407
Google Scholar
[19] Keathley P D, Bhardwaj S, Moses J, Laurent G, Kaertner F X 2016 New J. Phys. 18 073009
Google Scholar
[20] Månsson E P, Guénot D, Arnold C L, Kroon D, Kasper S, Dahlström J M, Lindroth E, Kheifets A S, L’huillier A, Sorensen S L 2014 Nat. Phys. 10 207
Google Scholar
[21] Jordan I, Huppert M, Pabst S, Kheifets A S, Baykusheva D, Wörner H J 2017 Phys. Rev. A 95 013404
Google Scholar
[22] Kotur M, Guenot D, Jiménez-Galán Á, Kroon D, Larsen E W, Louisy M, Bengtsson S, Miranda M, Mauritsson J, Arnold C 2016 Nat. Commun. 7 10566
Google Scholar
[23] Haessler S, Fabre B, Higuet J, Caillat J, Ruchon T, Breger P, Carré B, Constant E, Maquet A, Mével E 2009 Phys. Rev. A 80 011404
Google Scholar
[24] Klünder K, Dahlström J, Gisselbrecht M, Fordell T, Swoboda M, Guenot D, Johnsson P, Caillat J, Mauritsson J, Maquet A 2011 Phys. Rev. Lett. 106 143002
Google Scholar
[25] Zhan M J, Ye P, Teng H, He X K, Zhang W, Zhong S Y, Wang L F, Yun C X, Wei Z Y 2013 Chin. Phys. Lett. 30 093201
Google Scholar
[26] Jiang Y J, Liang Y Y, Gao Y T, Zhao K, Xu S Y, Wang J, He X K, Teng H, Zhu J F, Chen Y L, Wei Z Y 2020 Chin. Phys. B 29 013206
Google Scholar
[27] Zhong S Y, Teng H, Zhu X X, Gao Y T, Wang K J, Wang X Z, Wang Y M, Yu S, Zhao K, Wei Z Y 2023 Chin. Opt. Lett. 21 113201
Google Scholar
[28] Lucchini M, Brügmann M, Ludwig A, Gallmann L, Keller U, Feurer T 2015 Opt. Express 23 29502
Google Scholar
[29] Kheifets A S, Bray A W 2021 Phys. Rev. A 103 L011101
Google Scholar
[30] Cattaneo L, Vos J, Lucchini M, Gallmann L, Cirelli C, Keller U 2016 Opt. Express 24 29060
Google Scholar
下载:
计量
- 文章访问数: 1717
- PDF下载量: 101
- 被引次数: 0
