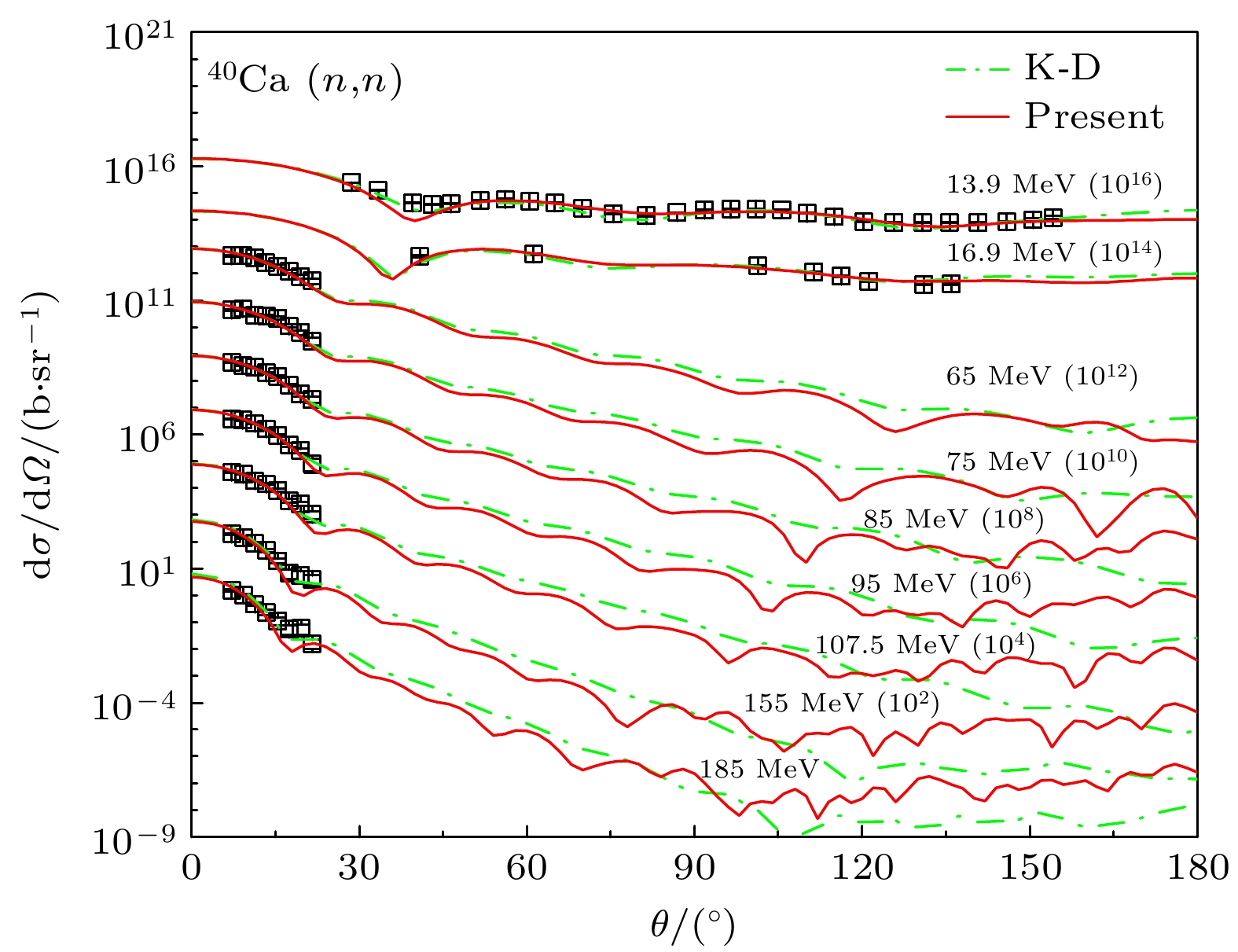
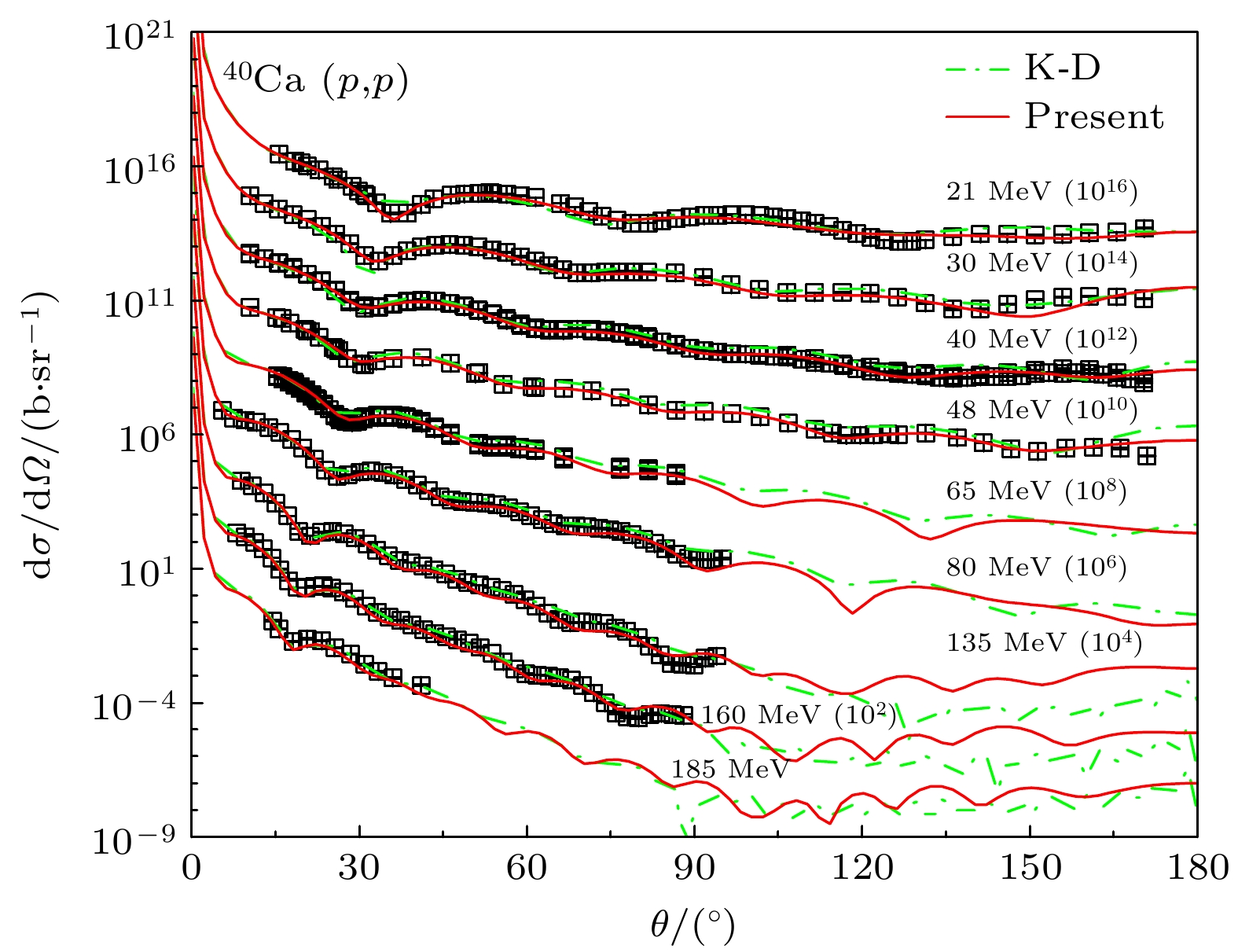
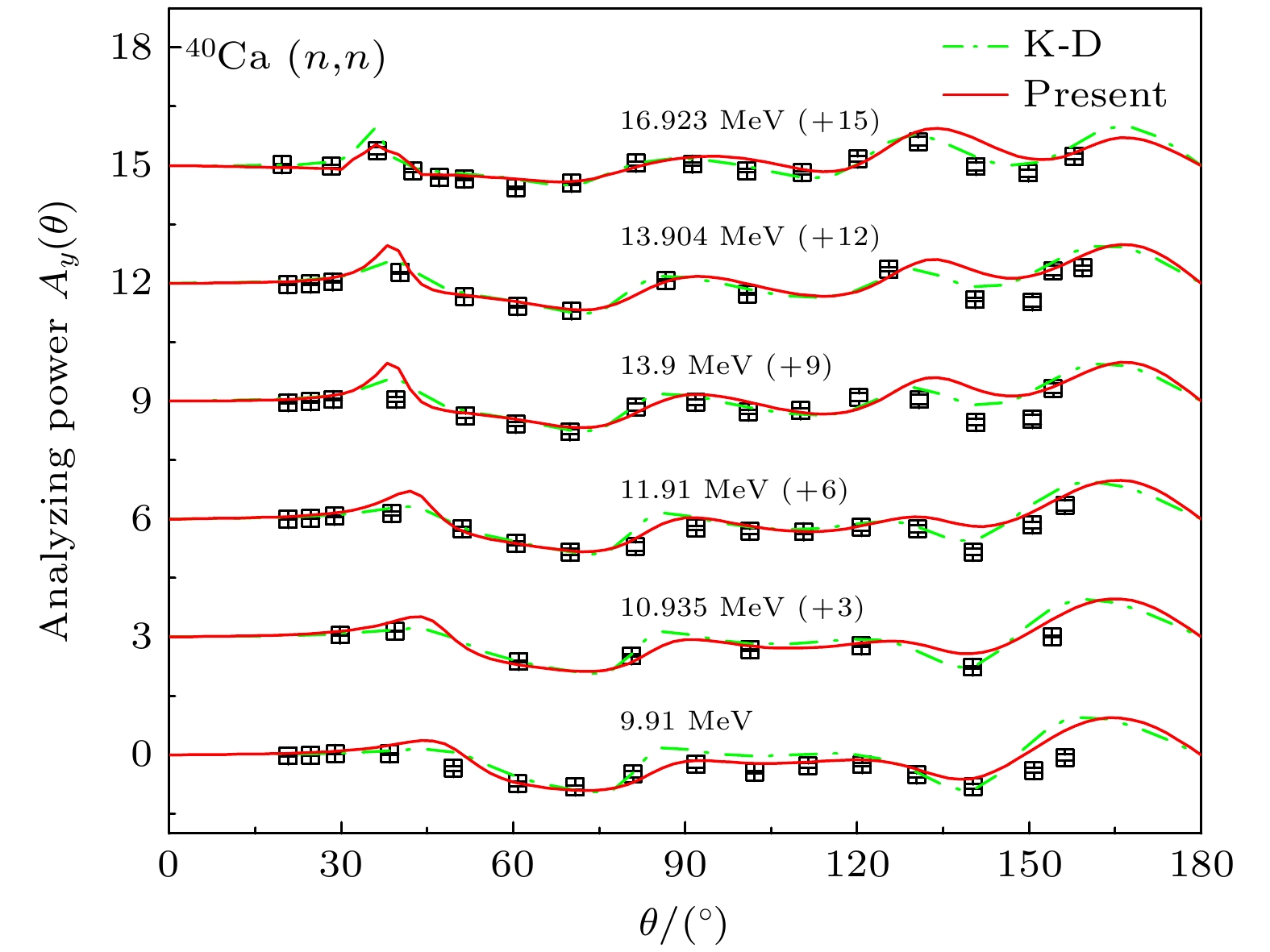
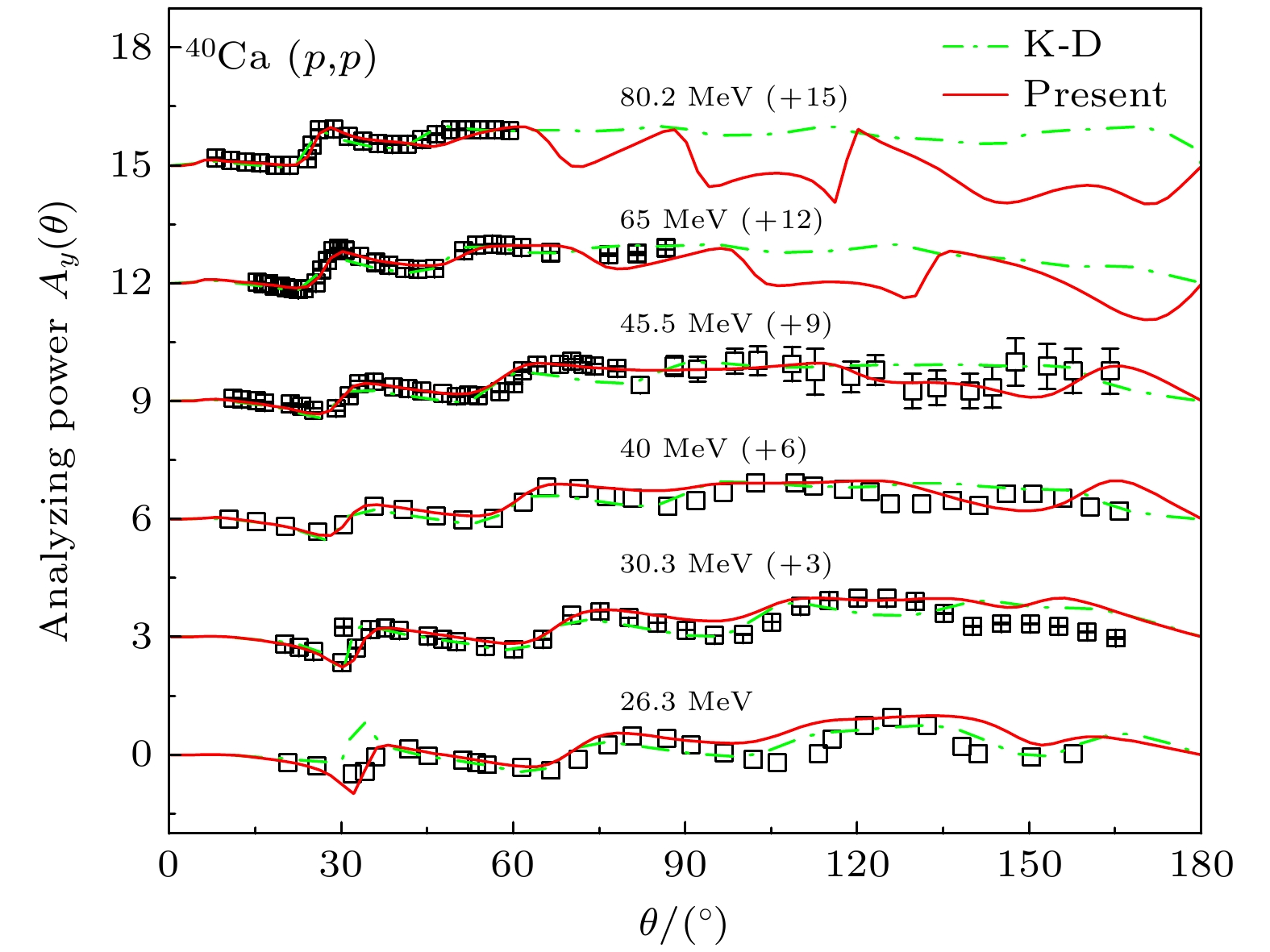
-
Spherical nucleus 40Ca is important structural and alloy material nucleus. Based on important theoretical value and application prospect of nuclear data of calcium isotopes, nucleon-nucleus scattering data on 40Ca nucleus, the main isotopes of natural calcium, are calculated by using dispersive optical model (DOM). The dispersive optical model potential is defined by energy-dependent real potentials, imaginary potentials, and also by the corresponding dispersive contributions to the real potential which are calculated analytically from the corresponding imaginary potentials by using a dispersion relation that follow from the requirement of causality. By fit simultaneously scattering experimental data for neutron and proton, an isospin-dependent dispersive optical model potential containing a dispersive term is derived. This derived potential in this work considers the nonlocality in the real “Hartree-Fock” potential
$ V_{\rm{HF}} $ and introduces the shell gap in the definition of nuclear imaginary volume, surface and spin-orbit potentials near the Fermi energy. This dispersive optical model potential shows a good description of nucleon-nucleus scattering data on 40Ca nucleus up to 200 MeV including neutron total cross sections, neutron elastic scattering angular distributions, proton elastic scattering angular distributions, neutron analyzing powers and proton analyzing powers. In addition, the energy dependencies of calculated real volume integrals of dispersive optical model potential is shown, and a typical dispersive hump is seen around the Fermi energy. This dispersive hump behavior naturally obtained from dispersion relations, and allows the dispersion optical potential to get rid of energy dependent geometry, thus avoiding the use of a radius dependent on energy.-
Keywords:
- nucleon scattering /
- dispersive optical model /
- elastic scattering
[1] Koning A J, Delaroche J P 2003 Nucl. Phys. A 713 231
Google Scholar
[2] Mahaux C, Sartor R 1986 Phys. Rev. Lett. 57 3015
Google Scholar
[3] Morillon B, Romain P 2004 Phys. Rev. C 70 014601
Google Scholar
[4] Morillon B, Romain P 2006 Phys. Rev. C 74 014601
Google Scholar
[5] Soukhovitskiĩ E Sh, Capote R, Quesada J M, Chiba S 2005 Phys. Rev. C 72 024604
Google Scholar
[6] Capote R, Soukhovitskiĩ E Sh, Quesada J M, Chiba S 2005 Phys. Rev. C 72 064610
Google Scholar
[7] Hao L J, Sun W L, Soukhovitskiĩ E Sh 2008 J. Phys. G: Nucl. Part. Phys. 35 095103
Google Scholar
[8] Capote R, Herman M, Obložinský P, Young P G, Goriely S, Belgya T, Ignatyuk A V, Koning A J, Hilaire S, Plujko V A, Avrigeanu M, Bersillon O, Chadwick M B, Fukahori T, Ge Z G, Han Y L, Kailas S, Kopecky J, Maslov V M, Reffo G, Sin G, Soukhovitskii E Sh, Talou P 2009 Nucl. Data Sheets 110 3107
Google Scholar
[9] Soukhovitskiĩ E Sh, Capote R, Quesada J M, Chiba S, Martyanov D S 2016 Phys. Rev. C 94 064605
Google Scholar
[10] Dickhoff W H, Charity R J 2019 Prog. Part. Nucl. Phys. 105 252
Google Scholar
[11] Zhao X N, Sun W L, Soukhovitskiĩ E Sh, Martyanov D S, Quesada J M, Capote R 2019 J. Phys. G: Nucl. Part. Phys. 46 055103
Google Scholar
[12] Zhao X N, Sun W L, Capote R, Soukhovitskiĩ E Sh, Martyanov D S, Quesada J M 2020 Phys. Rev. C 101 064618
Google Scholar
[13] Zhao X N, Du W Q, Capote R, Soukhovitskiĩ E Sh 2023 Phys. Rev. C 107 064606
Google Scholar
[14] Perey F, Buck B 1962 Nucl. Phys. 32 353
Google Scholar
[15] Brown G E, Rho M 1981 Nucl. Phys. A 372 397
Google Scholar
[16] Delaroche J P, Wang Y, Rapaport J 1989 Phys. Rev. C 39 391
Google Scholar
[17] Molina A, Capote R, Quesada J M, Lozano M 2002 Phys. Rev. C 65 034616
Google Scholar
[18] Mahaux C, Sartor R 1991 Nucl. Phys. A 528 253
Google Scholar
[19] Lane A M 1962 Phys. Rev. Lett. 8 171
Google Scholar
[20] Lane A M 1962 Nucl. Phys. 35 676
Google Scholar
[21] Sukhovitskiĩ E Sh, Lee Y O, Chang J, Chiba S, Iwamoto O 2000 Phys. Rev. C 62 044605
Google Scholar
[22] EXchange FORmat database (EXFOR) is maintained by the Network of Nuclear Reaction Data Centers (see www-nds.iaea.org/nrdc/). Data available online (e.g., at www-nds.iaea.org/exfor/).
-
图 1 40Ca中子诱发核反应计算中, 光学势各项深度随能量的变化情况
Fig. 1. Energy dependence of the DOM potential depths calculated for neutron induced reactions on 40Ca.
图 2 40Ca的中子总截面计算结果与K-D光学势给出的计算结果以及相关实验数据的比较
Fig. 2. Comparison of the calculated total cross section for 40Ca with calculations using the K-D potential and measurements.
图 3 40Ca的中子弹性散射角分布计算结果与K-D光学势给出的计算结果以及相关实验数据的比较
Fig. 3. Comparison of neutron elastic scattering angular distributions for 40Ca with calculations using the K-D potential and measurements.
图 4 40Ca的质子弹性散射角分布计算结果与K-D光学势给出的计算结果以及相关实验数据的比较
Fig. 4. Comparison of proton elastic scattering angular distributions for 40Ca with calculations using the K-D potential and measurements.
图 5 40Ca的中子弹性散射分析本领计算结果与K-D光学势给出的计算结果以及相关实验数据的比较
Fig. 5. Neutron elastic scattering analyzing powers for 40Ca compared with calculations using the K-D potential and the experimental data.
图 6 40Ca的质子弹性散射分析本领计算结果与K-D光学势给出的计算结果以及相关实验数据的比较
Fig. 6. Proton elastic scattering analyzing powers for 40Ca compared with calculations using the K-D potential and the experimental data.
图 7 40Ca的中子诱发核反应计算中DOM势实部体积分随能量的变化情况, 其中右边纵坐标度量
$V_{\rm{HF}}$ 势和总的实部势, 左边纵坐标度量色散修正项Fig. 7. Energy dependencies of calculated real volume integrals of dispersive optical model potential for neutron induced reactions on 40Ca. Volume integrals of and total real potential by using right Y-axis, the volume integrals of dispersive terms by using left Y-axis.
表 1 40Ca中子诱发核反应计算中的DOM势参数
Table 1. DOM potential parameters for nucleon induced reactions on 40Ca.
Volume Surface Spin-orbit couple Coulomb Real $V_{0}$ = 85.93$\rm{MeV}$ $ V_{\rm{so} } $ = 6.1$\rm{MeV}$ $C_{\rm{Coul}}$ = 1.0$\rm{MeV}$ potential $\lambda_{\rm{HF}}$ = 0.94$\rm{MeV}^{-1}$ Dispersive $ \lambda_{\rm{so} } $ = 0.004$\rm{MeV}^{-1}$ $ C_{\rm{viso} } $ = 24$\rm{MeV}$ Imaginary $A_{\rm{v}}$ = 13.36$\rm{MeV}$ $W_{0}$ = 17.67$\rm{MeV}$ $ W_{\rm{so} } $ = –3.1$\rm{MeV}$ potential $B_{\rm{v}}$ = 86.63$\rm{MeV}$ $B_{\rm{s}}$ = 11.74$\rm{MeV}$ $ B_{\rm{so} } $ = 160$\rm{MeV}$ $E_{\rm{a}}$ = 56$\rm{MeV}$ $C_{\rm{s}}$ = 0.02$\rm{MeV}^{-1}$ α = 0.380$\rm{MeV}^{1/2}$ $ C_{\rm{wiso} } $ = 22$\rm{MeV}$ Potential $r_{\rm{HF}}$ = 1.26$\rm{fm}$ $r_{\rm{s}}$ = 1.22$\rm{fm}$ $ r_{\rm{so} } $ = 1.35$\rm{fm}$ $r_{\rm{c}}$ = 1.03$\rm{fm}$ geometry $a_{\rm{HF}}$ = 0.622$\rm{fm}$ $a_{\rm{s}}$ = 0.502$\rm{fm}$ $ a_{\rm{so} } $ = 0.682$\rm{fm}$ $a_{\rm{c}}$ = 0.252$\rm{fm}$ $r_{\rm{v}}$ = 1.34$\rm{fm}$ $a_{\rm{v}}$ = 0.675$\rm{fm}$ 
下载: 导出CSV
-
[1] Koning A J, Delaroche J P 2003 Nucl. Phys. A 713 231
Google Scholar
[2] Mahaux C, Sartor R 1986 Phys. Rev. Lett. 57 3015
Google Scholar
[3] Morillon B, Romain P 2004 Phys. Rev. C 70 014601
Google Scholar
[4] Morillon B, Romain P 2006 Phys. Rev. C 74 014601
Google Scholar
[5] Soukhovitskiĩ E Sh, Capote R, Quesada J M, Chiba S 2005 Phys. Rev. C 72 024604
Google Scholar
[6] Capote R, Soukhovitskiĩ E Sh, Quesada J M, Chiba S 2005 Phys. Rev. C 72 064610
Google Scholar
[7] Hao L J, Sun W L, Soukhovitskiĩ E Sh 2008 J. Phys. G: Nucl. Part. Phys. 35 095103
Google Scholar
[8] Capote R, Herman M, Obložinský P, Young P G, Goriely S, Belgya T, Ignatyuk A V, Koning A J, Hilaire S, Plujko V A, Avrigeanu M, Bersillon O, Chadwick M B, Fukahori T, Ge Z G, Han Y L, Kailas S, Kopecky J, Maslov V M, Reffo G, Sin G, Soukhovitskii E Sh, Talou P 2009 Nucl. Data Sheets 110 3107
Google Scholar
[9] Soukhovitskiĩ E Sh, Capote R, Quesada J M, Chiba S, Martyanov D S 2016 Phys. Rev. C 94 064605
Google Scholar
[10] Dickhoff W H, Charity R J 2019 Prog. Part. Nucl. Phys. 105 252
Google Scholar
[11] Zhao X N, Sun W L, Soukhovitskiĩ E Sh, Martyanov D S, Quesada J M, Capote R 2019 J. Phys. G: Nucl. Part. Phys. 46 055103
Google Scholar
[12] Zhao X N, Sun W L, Capote R, Soukhovitskiĩ E Sh, Martyanov D S, Quesada J M 2020 Phys. Rev. C 101 064618
Google Scholar
[13] Zhao X N, Du W Q, Capote R, Soukhovitskiĩ E Sh 2023 Phys. Rev. C 107 064606
Google Scholar
[14] Perey F, Buck B 1962 Nucl. Phys. 32 353
Google Scholar
[15] Brown G E, Rho M 1981 Nucl. Phys. A 372 397
Google Scholar
[16] Delaroche J P, Wang Y, Rapaport J 1989 Phys. Rev. C 39 391
Google Scholar
[17] Molina A, Capote R, Quesada J M, Lozano M 2002 Phys. Rev. C 65 034616
Google Scholar
[18] Mahaux C, Sartor R 1991 Nucl. Phys. A 528 253
Google Scholar
[19] Lane A M 1962 Phys. Rev. Lett. 8 171
Google Scholar
[20] Lane A M 1962 Nucl. Phys. 35 676
Google Scholar
[21] Sukhovitskiĩ E Sh, Lee Y O, Chang J, Chiba S, Iwamoto O 2000 Phys. Rev. C 62 044605
Google Scholar
[22] EXchange FORmat database (EXFOR) is maintained by the Network of Nuclear Reaction Data Centers (see www-nds.iaea.org/nrdc/). Data available online (e.g., at www-nds.iaea.org/exfor/).
下载:
计量
- 文章访问数: 1967
- PDF下载量: 59
- 被引次数: 0
