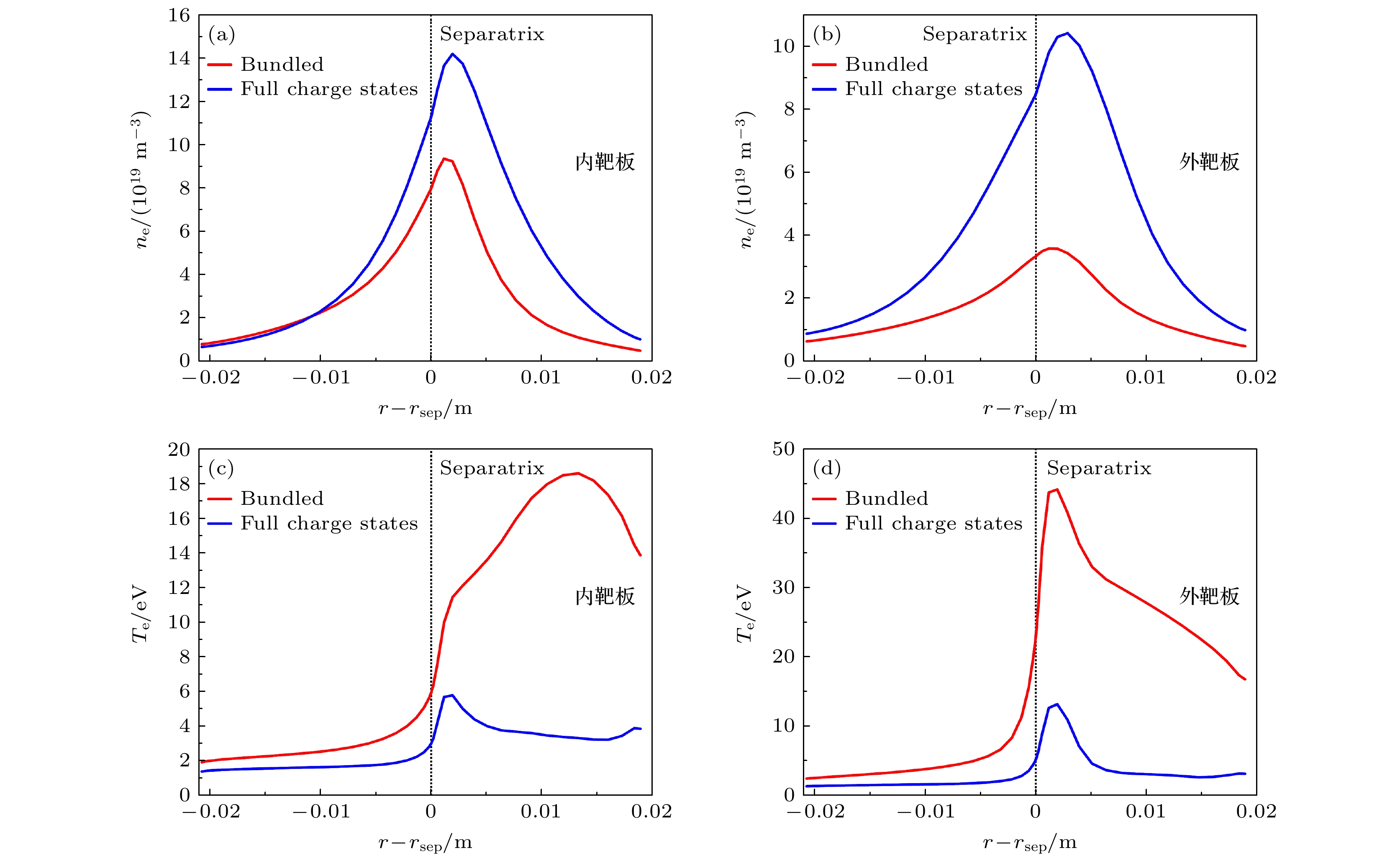
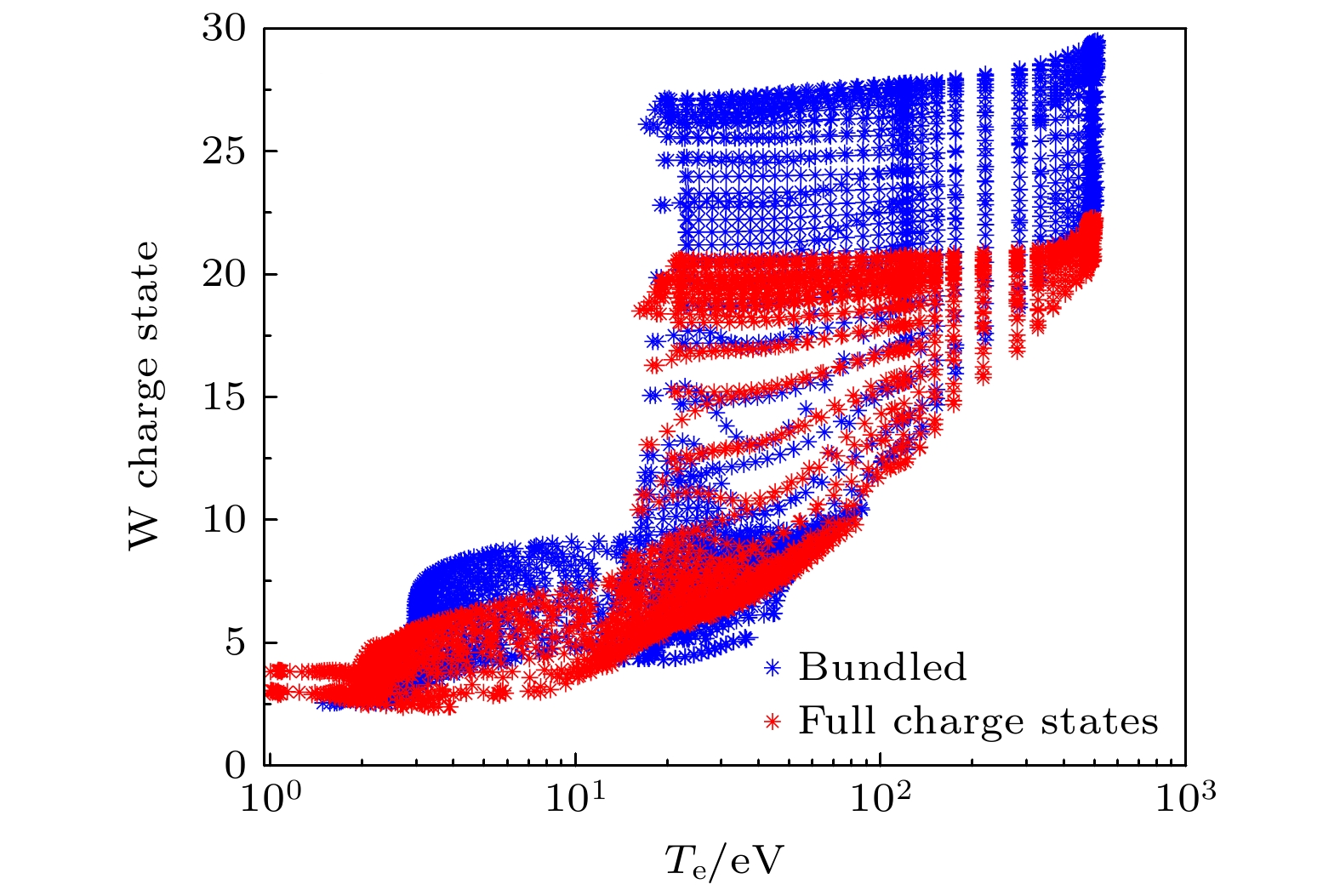
-
重杂质(如钨)聚芯是未来托卡马克反应堆中等离子体高性能运行所面临的严峻挑战. 开展多流体及动力学模拟以研究氖杂质注入条件下, 东方超环EAST托卡马克中等离子体高约束时的钨杂质边界输运特性. 结果表明, 低电离态钨离子主要聚集在碰撞率较高的偏滤器区域, 流体近似可很好地满足; 高电离态钨离子密度相对较低且主要位于碰撞率相对较低的芯部, 多流体与动力学模拟结果差异显著; 但二者计算的钨杂质总密度差异较小(< 30%). 多流体模拟中, 除将钨离子考虑为74种流体外, 还将电离能接近的钨离子进行价态捆绑. 比较发现, 价态捆绑可显著降低计算成本, 但在高再循环(或部分脱靶)运行机制下可显著高估(低估)偏滤器区域等离子体温度(密度), 从而大幅低估钨电离源及钨密度, 其根源在于价态捆绑对钨离子平均电离态和偏滤器区域辐射功率损失的显著影响. 模拟结果还表明, 氖杂质注入促进偏滤器脱靶可有效缓解钨杂质聚芯.Accumulation of tungsten (W) in core is a serious challenge for achieving high-performance plasmas in future tokamak reactors, thus W impurity transport is a highly concerned topic in the tokamak physics researches. Multi-fluid model and kinetic model are the numerical tools widely used for investigating and/or predicting impurity behaviors in the boundary of tokamak plasma. Generally, the applicability of multi-fluid model for impurity transport modeling requires that the collision mean-free-path should be smaller than the gradient scale lengths of particles, which may not be always satisfied. It is performed and comparatively investigated to evaluate the applicability of multi-fluid model for W impurity transport modeling, multi-fluid (SOLPS-ITER) modeling and kinetic (DIVIMP) modeling of W impurity transport in the edge of high-confinement plasma in Experimental Advanced Superconducting Tokamak (EAST) during neon impurity seeding. It is found that low-charge-state W ions are mainly located in the divertor region near the target plate where plasma collisionality is relatively high due to the relatively low/high local plasma temperature/density. Hence, the fluid assumption for transport of lowly-charged W ions can be well satisfied. Consequently, the density of lowly-charged W ions predicted by SOLPS-ITER and that calculated by DIVIMP are almost similar. Owing to the fact that the density of highly-charged W ions is relatively low and these particles mainly exist in the upstream (e.g. the main SOL and core) where plasma collisionality is relatively low, the fluid approximation cannot be well satisfied. However, the total W impurity density calculated by the kinetic code DIVIMP and the multi-fluid model SOLPS-ITER are found to be in agreement with each other within a factor of 1.5 for the simulation cases presented in this contribution. Besides, the multi-fluid simulation with bundled charge state model has also been performed, the obtained results are compared with those from the multi-fluid modeling with W ions treated as 74 fluids. It is revealed that in simulation cases with neon impurity seeding and with divertor plasmas in high-recycling or partially detached regimes, the bundling scheme, which is commonly used for saving the computation cost in multi-fluid modeling, tends to overestimate the average charge state of W ions and thus tends to underestimate the radiation power loss, especially in the divertor region. Consequently, under the circumstance that W impurity radiation dominates the radiative power loss in divertor region, plasma temperature/density can be largely overestimated/underestimated, leading to the underestimation of W ion ionization source and W impurity density. Moreover, simulation results demonstrate that W accumulation in core can decrease effectively during divertor detachment promoted by neon seeding.
-
Keywords:
- tokamak /
- tungsten impurity /
- multi-fluid model /
- kinetic modeling
[1] Ye D W, Ding F, Li K D, Hu Z H, Zhang L, Chen X H, Zhang Q, Zhao P A, He T, Meng L Y, Ye K X, Zhong F B, Duan Y M, Ding R, Wang L, Xu G S, Luo G N, EAST team 2022 Chin. Phys. B 31 065201
Google Scholar
[2] Wang F Q, Zha X J, Duan Y M, Mao S T, Wang L, Zhong F C, Liang L, Li L, Lu H W, Hu L Q, Chen Y P, Yang Z D 2018 Plasma Phys. Control. Fusion 60 125005
Google Scholar
[3] Yamoto S, Bonnin, X, Homma Y, Inoue H, Hoshino K, Hatayama A, Pitts RA 2017 Nucl. Fusion 57 116051
Google Scholar
[4] Kirschner A, Tskhakaya D, Brezinsek S, Borodin D, Romazanov J, Ding R, Eksaeva A, Linsmeier C 2018 Plasma Phys. Control. Fusion 60 014041
Google Scholar
[5] Shi S Y, Chen J L, Bourdelle C, Jian X, Odstrcil T, Garofalo A M, Cheng Y X, Chao Y, Zhang L, Duan Y M, Wu M Q, Ding F, Li Y Y, Huang J, Qian J P, Gao X, Wan Y X 2022 Nucl. Fusion 62 066031
Google Scholar
[6] Putterich T, Dux R, Neu R, Bernert M, Beurskens M N A, Bobkov V, Brezinsek S, Challis C, Coenen J W, Coffey I, Czarnecka A, Giroud C, Jacquet P, Joffrin E, Kallenbach A, Lehnen M, Lerche E, de la Luna E, Marsen S, Matthews G, Mayoral M L, McDermott R M, Meigs A, Mlynar J, Sertoli M, van Rooij G 2013 Plasma Phys. Control. Fusion 55 124036
Google Scholar
[7] Robert A 1997 IEEE Trans. Plasma Sci. 25 1187
Google Scholar
[8] Motojima O 2015 Nucl. Fusion 55 104023
Google Scholar
[9] Hinnov E, Mattioli M 1978 Phys. Lett. A 66 109
Google Scholar
[10] Isler R, Neidigh R, Cowan R 1977 Phys. Lett. A 63 295
Google Scholar
[11] Neu R, Dux R, Geier A, Greuner H, Krieger K, Maier H, Pugno R, Rohde V, Yoon S W 2003 J. Nucl. Mater. 313–316 116
[12] Zhang L, Morita S, Xu Z, Zhang P F, Zang Q, Duan Y M, Liu H Q, Zhao H L, Ding F, Ohishi T, Gao W, Huang J, Yang X D, Chen Y J, Wu Z W, Xu P, Ding B J, Hu C D, Gong X Z, Chen J L, Hu L Q 2017 Nucl. Mater. Energy 12 774
Google Scholar
[13] Wagner F, Becker G, Behringer K, Campbell D, Eberhagen A, Engelhardt W, Fussmann G, Gehre O, Gernhardt J, Vongierke G, Haas G, Huang M, Karger F, Keilhacker M, Klüber O, Kornherr M, Lackner K, Lisitano G, Lister G G, Mayer H M, Meisel D, Müller E R, Murmann H, Niedermeyer H, Poschenrieder W, Rapp H, Röhr H, Schneider F, Siller G, Speth E, Stäbler A, Steuer K H, Venus G, Vollmer O, Yü Z 1982 Phys. Rev. Lett. 49 1408
Google Scholar
[14] 杜海龙, 桑超峰, 王亮, 孙继忠, 刘少承, 汪惠乾, 张凌, 郭后扬, 王德真 2013 62 245206
Google Scholar
Du H L, Sang C F, Wang L, Sun J Z, Liu S C, Wang H Q, Zhang L, Guo H Y, Wang D Z 2013 Acta Phys. Sin. 62 245206
Google Scholar
[15] Dux R, Peeters A G, Gude A, Kallenbach A, Neu R, ASDEX Upgrade Team 1999 Nucl. Fusion 39 1509
Google Scholar
[16] 张文敏, 张凌, 程云鑫, 王正汹, 胡爱兰, 段艳敏, 周天富, 刘海庆 2022 71 115203
Google Scholar
Zhang W M, Zhang L, Cheng Y X, Wang Z X, Hu A L, Duan Y M, Zhou T F, Liu H Q 2022 Acta Phys. Sin. 71 115203
Google Scholar
[17] 沈勇, 董家齐, 徐红兵 2018 67 195203
Google Scholar
Shen Y, Dong J Q, Xu H B 2018 Acta Phys. Sin. 67 195203
Google Scholar
[18] Ciraolo G, Di Genova S, Yang H, Gallo A, Fedorczak N, Bufferand H, Gunn J P, Tamain P, Guirlet R, Guillemaut C, Desgranges C, Bourdelle C, Tsitrone E, Bucalossi J, D'Abusco M S, Serre E, Marandet Y, Raghunathan M, Sepetys A, Romazanov J, Kirschner A, Brezinsek S 2021 Nucl. Fusion 61 126015
Google Scholar
[19] Geier A, Krieger K, Elder J D, Pugno R, Rohde V 2003 J. Nucl. Mater. 313–316 1216
[20] Lim K, Garbet X, Sarazin Y, Grandgirard V, Obrejan K, Lesur M, Gravier E 2021 Nucl. Fusion 61 046037
Google Scholar
[21] van Vugt D C, Huijsmans G T A, Hoelzl M, Loarte A 2019 Phys. Plasmas 26 042508
Google Scholar
[22] Sinclair G, Maurizio R, Ma X, Abrams T, Elder J D, Guo H Y, Thomas D M, Leonard A W 2022 Nucl. Fusion 62 106024
Google Scholar
[23] Sang C F, Zhou Q R, Xu G S, Wang L, Wang Y L, Zhao X L, Zhang C, Ding R, Jia G Z, Yao D M, Liu X J, Si H, Wang D Z 2021 Nucl. Fusion 61 066004
Google Scholar
[24] Schmid K, Krieger K, Kukushkin A, Loarte A 2007 J. Nucl. Mater. 363–365 674
[25] Wiesen S, Reiter D, Kotov V, Baelmans M, Dekeyser W, Kukushkin A S, Lisgo S W, Pitts R A, Rozhansky V, Saibene G, Veselova I, Voskoboynikov S 2015 J. Nucl. Mater. 463 480
Google Scholar
[26] Bonnin X, Dekeyser W, Pitts R, Coster D, Voskoboynikov S, Wiesen S 2016 Plasma Fusion Res. 11 1403102
Google Scholar
[27] Strachan J D, Corrigan G, Harting D, Lauro-Taroni L, Maggi C F, Matthews G F, O'Mullane M, Reiter D, Seebacher J, Spence J, Summers H, Wiesen S 2011 J. Nucl. Mater. 415 S501
Google Scholar
[28] Harting D, Groth M, Beurskens M, Boerner P, Brix M, Coenen J W, Corrigan G, Lehnen M, Marsen S, van Rooij G, Reiter D, Wiesen S 2013 J. Nucl. Mater. 438 S480
Google Scholar
[29] Stangeby P C, Elder J D 1995 Nucl. Fusion 35 1391
Google Scholar
[30] Yamoto S, Homma Y, Hoshino K, Toma M, Hatayama A 2020 Comput. Phys. Commun. 248 106979
Google Scholar
[31] Wang J, Chen Y P, Wang L, Gao W, Wu Z W, Zhang L 2023 Phys. Plasmas 30 043905
Google Scholar
[32] Song Y T, Wan B N, Gong X Z, et al. 2022 IEEE Trans. Plasma Sci. 50 4330
Google Scholar
[33] Wang F Q, Liang Y, Zha X J, Zhong F C, Mao S T, Duan Y M, Hu L Q, Wang L, Liu J B, Yan N, Liu S C 2022 Nucl. Fusion 62 056021
Google Scholar
[34] Pan O, Bernert M, Lunt T, Cavedon M, Kurzan B, Wiesen S, Wischmeier M, Stroth U 2023 Nucl. Fusion 63 016001
Google Scholar
[35] Kaveeva E, Rozhansky V, Veselova I, Senichenkov I, Giroud C, Pitts R A, Wiesen S, Voskoboynikov S 2021 Nucl. Mater. Energy 28 101030
Google Scholar
[36] Koenders J T W, Wensing M, Ravensbergen T, Fevrier O, Perek A, van Berkel M 2022 Nucl. Fusion 62 066025
Google Scholar
[37] Kaveeva E, Rozhansky V, Senichenkov I, Sytova E, Veselova I, Voskoboynikov S, Bonnin X, Pitts R A, Kukushkin A S, Wiesen S, Coster D 2020 Nucl. Fusion 60 046019
Google Scholar
[38] Senichenkov I Y, Ding R, Molchanov P A, Kaveeva E G, Rozhansky V A, Voskoboynikov S P, Shtyrkhunov N V, Makarov S O, Si H, Liu X, Sang C, Mao S 2022 Nucl. Fusion 62 096010
Google Scholar
[39] Kumpulainen H A, Groth M, Fontell M, Jaervinen A E, Corrigan G, Harting D 2020 Nucl. Mater. Energy 25 100784
Google Scholar
[40] Jarvinen A, Giroud C, Groth M, Krieger K, Moulton D, Wiesen S, Brezinsek S 2011 Phys. Scr. 2011 014013
[41] Rozhansky V, Kaveeva E, Molchanov P, Veselova I, Voskoboynikov S, Coster D, Counsell G, Kirk A, Lisgo S 2009 Nucl. Fusion 49 025007
Google Scholar
[42] Reiter D, Baelmans M, Börner P 2005 Fusion Sci. Technol. 47 172
Google Scholar
[43] Schneider R, Bonnin X, Borrass K, Coster D P, Kastelewicz H, Reiter A, Rozhansky V A, Braams B J 2006 Contrib. Plasma Phys. 46 3
Google Scholar
[44] Wang R, Yang Z S, Li K D, Xu G S, Jia G Z, Niu G J, Nian F F, He T, Meng L Y, Lin X, Luo G N, Wang L 2022 Phys. Plasmas 29 112502
Google Scholar
[45] Hechtl E, Bohdansky J, Roth J 1981 J. Nucl. Mater. 103 333
Google Scholar
[46] Gao S L, Liu X J, Deng G Z, Ming T F, Li G Q, Zhang X X, Wu X D, Wu X H, Li B, Fan H C, Gao X 2022 Plasma Sci. Technol. 24 075104
Google Scholar
[47] 欧靖, 杨锦宏 2012 61 075201
Google Scholar
Ou J, Yang J H 2012 Acta Phys. Sin. 61 075201
Google Scholar
[48] Summers H P, O'Mullane M G 2000 AIP Conference Proceedings 543 304
Google Scholar
[49] Putterich T, Fable E, Dux R, O'Mullane M, Neu R, Siccinio M 2019 Nucl. Fusion 59 056013
Google Scholar
-
图 1 (a) 80443炮放电在6.5 s时刻的磁场位形; (b) SOLPS-ITER及DIVIMP计算网格
Fig. 1. (a) Magnetic configuration for shot #80443 at t = 6.5 s; (b) grid meshes for SOLPS-ITER and DIVIMP calculations.
图 2 模拟中设定的径向输运系数(
$ r-{r}_{{\rm{s}}{\rm{e}}{\rm{p}}} < 0 $ 代表芯部,$ r-{r}_{{\rm{s}}{\rm{e}}{\rm{p}}} > 0 $ 代表刮削层)Fig. 2. Radial transport coefficients specified in the simulations (
$ r-{r}_{{\rm{s}}{\rm{e}}{\rm{p}}} < 0 $ for core,$ r-{r}_{{\rm{s}}{\rm{e}}{\rm{p}}} > 0 $ for SOL).
图 3 SOLPS-ITER采用不同钨离子流体方案(蓝色代表方案1, 即74种流体; 红色代表方案2, —价态捆绑减为23种流体)计算所得外中平面处等离子体密度(a)和温度(b)的径向分布
Fig. 3. Radial profiles of plasma density (a) and temperature (b) at the outer mid-plane calculated by SOLPS-ITER using the full-charge-states (blue, 74 fluids) and bundled-charge-states (red, 23 fluids) fluid models.
图 4 SOLPS-ITER采用不同钨离子流体方案计算所得偏滤器靶板等离子体密度(a), (b)和温度(c), (d)分布
Fig. 4. Radial profiles of plasma density (a), (b) and temperature (c), (d) at the inner (a), (c) and outer (b), (d) target plates, calculated by SOLPS-ITER using the full-charge-states and bundled-charge-states fluid models.
图 5 不同模型计算得到的钨杂质密度二维分布 (a) 流体模型(SOLPS-ITER)将钨离子看作74种流体; (b) 流体模型(SOLPS-ITER)将部分价态钨离子捆绑(bundled) ; (c) 动力学模型(DIVIMP)
Fig. 5. Two-dimensional distribution of tungsten impurity density calculated by different models: (a) SOLPS-ITER using full-charge-states fluid model; (b) SOLPS-ITER using bundled-charge-states model; (c) DIVIMP.
图 6 不同模型计算所得钨杂质沿(a)内偏滤器靶板、(b)外偏滤器靶板、(c)中平面等处的径向分布, (d)及其在刮削层中第一个磁通管上的极向分布(横轴为距外靶板的极向距离). 注意: 图6(d)纵轴为对数坐标
Fig. 6. Calculated radial profiles of tungsten impurity density at the inner/outer target plate (a)/(b) and outer mid-plane (c) together with the poloidal profile of tungsten impurity density along the flux surface in the SOL near the separatrix (d). It is notable that the vertical axis is logarithmically scaled in panel (d).
图 7 SOLPS-ITER采用不同流体方案计算所得各网格中钨杂质平均电离态, 横轴为网格中的电子温度
Fig. 7. Average charge state of W ions in each grid cell plotted against the local electron temperature for SOLPS-ITER with full-charge-states fluid model and bundled-charge-states model.
图 8 SOLPS-ITER和DIVIMP计算所得不同价态钨杂质离子密度沿磁面的极向分布 (a) W1+—W10+; (b) W11+—W15+; (c) W16+—W20+; (d) W21+—W74+. 图中横轴为到外靶板的极向距离
Fig. 8. Poloidal profiles for W ions at different charge states calculated by SOLPS-ITER and DIVIMP: (a) W1+—W10+; (b) W11+—W15+; (c) W16+—W20+; (d) W21+—W74+. The vertical coordinates represent the poloidal distance from the outer target measured in the upstream direction.
图 9 当氖杂质注入率为2×1019 s–1 (a)和5×1019 s–1 (b)时, 计算得到的钨杂质含量(
$ {c}_{{\rm{W}}}={n}_{{\rm{W}}}/{n}_{{\rm{e}}} $ ).Fig. 9. W plasma content (
$ {c}_{{\rm{W}}}={n}_{{\rm{W}}}/{n}_{{\rm{e}}} $ ) for neon seeding level at 2×1019 s–1 (a) and 5×1019 s–1 (b).表 1 SOLPS-ITER采用不同流体方案计算所得+1价钨离子(W1+)电离源在外偏滤器区域(OD)、内偏滤器区域(ID)、刮削层(SOL)及芯部(core)的强度(单位: 1019 s–1)
Table 1. Strength of W1+ ionization source from neutrals in the outer divertor (OD), inner divertor (ID), scrape-off layer (SOL) and core calculated by SOLPS-ITER using different fluid models (in 1019 s–1).
Fluid models OD ID SOL Core Total Bundled-charge-states 9.31 0.93 0.002 $ \sim 0 $ 10.2 Full-charge-states 38.7 10.2 0.04 $ \sim 0 $ 48.9 
下载: 导出CSV
表 2 SOLPS-ITER采用不同流体模型计算所得不同离子在内/外靶板(IT/OT)的通量及靶板总通量(单位: s–1)
Table 2. Total target fluxes of deuterium (D), neon (Ne) and tungsten (W) together with the D, Ne and W ion fluxes at the inner/outer divertor target (IT/OT) (in s–1), calculated by SOLPS-ITER.
Fluid model Species OT/1019 IT/1018 Total/1020 Bundled-charge-states D 2280 26500 493 Ne 7.46 43.2 1.18 W 9.26 9.20 1.02 Full-charge-states D 2360 24800 484 Ne 6.13 44.7 1.06 W 37.8 98.7 4.76
下载: 导出CSV
表 3 SOLPS-ITER采用不同流体模型计算所得氘(D)、氖(Ne)以及钨(W)辐射功率损失在内偏滤器区域(ID)、外偏滤器区域(OD)、刮削层(SOL)及芯部(Core)的分布(单位: kW)
Table 3. Contributions of deuterium (D), neon (Ne) and tungsten (W) to radiation power loss in the inner/outer divertor region (ID/OD), scrape-off layer (SOL) and core calculated by SOLPS-ITER using the full-charge-states model and bundled-charge-states model (in kW).
Fluid model ID OD SOL Core Total Bundled-charge-states D 20.76 25.03 13.59 1.75 61.13 Ne 23.56 26.50 73.57 89.08 212.71 W 46.07 31.07 10.08 1.28 88.50 Full-charge-states D 21.59 26.54 14.10 1.72 63.95 Ne 28.51 28.81 79.43 94.10 230.85 W 386.26 237.45 32.11 6.69 662.51
下载: 导出CSV
-
[1] Ye D W, Ding F, Li K D, Hu Z H, Zhang L, Chen X H, Zhang Q, Zhao P A, He T, Meng L Y, Ye K X, Zhong F B, Duan Y M, Ding R, Wang L, Xu G S, Luo G N, EAST team 2022 Chin. Phys. B 31 065201
Google Scholar
[2] Wang F Q, Zha X J, Duan Y M, Mao S T, Wang L, Zhong F C, Liang L, Li L, Lu H W, Hu L Q, Chen Y P, Yang Z D 2018 Plasma Phys. Control. Fusion 60 125005
Google Scholar
[3] Yamoto S, Bonnin, X, Homma Y, Inoue H, Hoshino K, Hatayama A, Pitts RA 2017 Nucl. Fusion 57 116051
Google Scholar
[4] Kirschner A, Tskhakaya D, Brezinsek S, Borodin D, Romazanov J, Ding R, Eksaeva A, Linsmeier C 2018 Plasma Phys. Control. Fusion 60 014041
Google Scholar
[5] Shi S Y, Chen J L, Bourdelle C, Jian X, Odstrcil T, Garofalo A M, Cheng Y X, Chao Y, Zhang L, Duan Y M, Wu M Q, Ding F, Li Y Y, Huang J, Qian J P, Gao X, Wan Y X 2022 Nucl. Fusion 62 066031
Google Scholar
[6] Putterich T, Dux R, Neu R, Bernert M, Beurskens M N A, Bobkov V, Brezinsek S, Challis C, Coenen J W, Coffey I, Czarnecka A, Giroud C, Jacquet P, Joffrin E, Kallenbach A, Lehnen M, Lerche E, de la Luna E, Marsen S, Matthews G, Mayoral M L, McDermott R M, Meigs A, Mlynar J, Sertoli M, van Rooij G 2013 Plasma Phys. Control. Fusion 55 124036
Google Scholar
[7] Robert A 1997 IEEE Trans. Plasma Sci. 25 1187
Google Scholar
[8] Motojima O 2015 Nucl. Fusion 55 104023
Google Scholar
[9] Hinnov E, Mattioli M 1978 Phys. Lett. A 66 109
Google Scholar
[10] Isler R, Neidigh R, Cowan R 1977 Phys. Lett. A 63 295
Google Scholar
[11] Neu R, Dux R, Geier A, Greuner H, Krieger K, Maier H, Pugno R, Rohde V, Yoon S W 2003 J. Nucl. Mater. 313–316 116
[12] Zhang L, Morita S, Xu Z, Zhang P F, Zang Q, Duan Y M, Liu H Q, Zhao H L, Ding F, Ohishi T, Gao W, Huang J, Yang X D, Chen Y J, Wu Z W, Xu P, Ding B J, Hu C D, Gong X Z, Chen J L, Hu L Q 2017 Nucl. Mater. Energy 12 774
Google Scholar
[13] Wagner F, Becker G, Behringer K, Campbell D, Eberhagen A, Engelhardt W, Fussmann G, Gehre O, Gernhardt J, Vongierke G, Haas G, Huang M, Karger F, Keilhacker M, Klüber O, Kornherr M, Lackner K, Lisitano G, Lister G G, Mayer H M, Meisel D, Müller E R, Murmann H, Niedermeyer H, Poschenrieder W, Rapp H, Röhr H, Schneider F, Siller G, Speth E, Stäbler A, Steuer K H, Venus G, Vollmer O, Yü Z 1982 Phys. Rev. Lett. 49 1408
Google Scholar
[14] 杜海龙, 桑超峰, 王亮, 孙继忠, 刘少承, 汪惠乾, 张凌, 郭后扬, 王德真 2013 62 245206
Google Scholar
Du H L, Sang C F, Wang L, Sun J Z, Liu S C, Wang H Q, Zhang L, Guo H Y, Wang D Z 2013 Acta Phys. Sin. 62 245206
Google Scholar
[15] Dux R, Peeters A G, Gude A, Kallenbach A, Neu R, ASDEX Upgrade Team 1999 Nucl. Fusion 39 1509
Google Scholar
[16] 张文敏, 张凌, 程云鑫, 王正汹, 胡爱兰, 段艳敏, 周天富, 刘海庆 2022 71 115203
Google Scholar
Zhang W M, Zhang L, Cheng Y X, Wang Z X, Hu A L, Duan Y M, Zhou T F, Liu H Q 2022 Acta Phys. Sin. 71 115203
Google Scholar
[17] 沈勇, 董家齐, 徐红兵 2018 67 195203
Google Scholar
Shen Y, Dong J Q, Xu H B 2018 Acta Phys. Sin. 67 195203
Google Scholar
[18] Ciraolo G, Di Genova S, Yang H, Gallo A, Fedorczak N, Bufferand H, Gunn J P, Tamain P, Guirlet R, Guillemaut C, Desgranges C, Bourdelle C, Tsitrone E, Bucalossi J, D'Abusco M S, Serre E, Marandet Y, Raghunathan M, Sepetys A, Romazanov J, Kirschner A, Brezinsek S 2021 Nucl. Fusion 61 126015
Google Scholar
[19] Geier A, Krieger K, Elder J D, Pugno R, Rohde V 2003 J. Nucl. Mater. 313–316 1216
[20] Lim K, Garbet X, Sarazin Y, Grandgirard V, Obrejan K, Lesur M, Gravier E 2021 Nucl. Fusion 61 046037
Google Scholar
[21] van Vugt D C, Huijsmans G T A, Hoelzl M, Loarte A 2019 Phys. Plasmas 26 042508
Google Scholar
[22] Sinclair G, Maurizio R, Ma X, Abrams T, Elder J D, Guo H Y, Thomas D M, Leonard A W 2022 Nucl. Fusion 62 106024
Google Scholar
[23] Sang C F, Zhou Q R, Xu G S, Wang L, Wang Y L, Zhao X L, Zhang C, Ding R, Jia G Z, Yao D M, Liu X J, Si H, Wang D Z 2021 Nucl. Fusion 61 066004
Google Scholar
[24] Schmid K, Krieger K, Kukushkin A, Loarte A 2007 J. Nucl. Mater. 363–365 674
[25] Wiesen S, Reiter D, Kotov V, Baelmans M, Dekeyser W, Kukushkin A S, Lisgo S W, Pitts R A, Rozhansky V, Saibene G, Veselova I, Voskoboynikov S 2015 J. Nucl. Mater. 463 480
Google Scholar
[26] Bonnin X, Dekeyser W, Pitts R, Coster D, Voskoboynikov S, Wiesen S 2016 Plasma Fusion Res. 11 1403102
Google Scholar
[27] Strachan J D, Corrigan G, Harting D, Lauro-Taroni L, Maggi C F, Matthews G F, O'Mullane M, Reiter D, Seebacher J, Spence J, Summers H, Wiesen S 2011 J. Nucl. Mater. 415 S501
Google Scholar
[28] Harting D, Groth M, Beurskens M, Boerner P, Brix M, Coenen J W, Corrigan G, Lehnen M, Marsen S, van Rooij G, Reiter D, Wiesen S 2013 J. Nucl. Mater. 438 S480
Google Scholar
[29] Stangeby P C, Elder J D 1995 Nucl. Fusion 35 1391
Google Scholar
[30] Yamoto S, Homma Y, Hoshino K, Toma M, Hatayama A 2020 Comput. Phys. Commun. 248 106979
Google Scholar
[31] Wang J, Chen Y P, Wang L, Gao W, Wu Z W, Zhang L 2023 Phys. Plasmas 30 043905
Google Scholar
[32] Song Y T, Wan B N, Gong X Z, et al. 2022 IEEE Trans. Plasma Sci. 50 4330
Google Scholar
[33] Wang F Q, Liang Y, Zha X J, Zhong F C, Mao S T, Duan Y M, Hu L Q, Wang L, Liu J B, Yan N, Liu S C 2022 Nucl. Fusion 62 056021
Google Scholar
[34] Pan O, Bernert M, Lunt T, Cavedon M, Kurzan B, Wiesen S, Wischmeier M, Stroth U 2023 Nucl. Fusion 63 016001
Google Scholar
[35] Kaveeva E, Rozhansky V, Veselova I, Senichenkov I, Giroud C, Pitts R A, Wiesen S, Voskoboynikov S 2021 Nucl. Mater. Energy 28 101030
Google Scholar
[36] Koenders J T W, Wensing M, Ravensbergen T, Fevrier O, Perek A, van Berkel M 2022 Nucl. Fusion 62 066025
Google Scholar
[37] Kaveeva E, Rozhansky V, Senichenkov I, Sytova E, Veselova I, Voskoboynikov S, Bonnin X, Pitts R A, Kukushkin A S, Wiesen S, Coster D 2020 Nucl. Fusion 60 046019
Google Scholar
[38] Senichenkov I Y, Ding R, Molchanov P A, Kaveeva E G, Rozhansky V A, Voskoboynikov S P, Shtyrkhunov N V, Makarov S O, Si H, Liu X, Sang C, Mao S 2022 Nucl. Fusion 62 096010
Google Scholar
[39] Kumpulainen H A, Groth M, Fontell M, Jaervinen A E, Corrigan G, Harting D 2020 Nucl. Mater. Energy 25 100784
Google Scholar
[40] Jarvinen A, Giroud C, Groth M, Krieger K, Moulton D, Wiesen S, Brezinsek S 2011 Phys. Scr. 2011 014013
[41] Rozhansky V, Kaveeva E, Molchanov P, Veselova I, Voskoboynikov S, Coster D, Counsell G, Kirk A, Lisgo S 2009 Nucl. Fusion 49 025007
Google Scholar
[42] Reiter D, Baelmans M, Börner P 2005 Fusion Sci. Technol. 47 172
Google Scholar
[43] Schneider R, Bonnin X, Borrass K, Coster D P, Kastelewicz H, Reiter A, Rozhansky V A, Braams B J 2006 Contrib. Plasma Phys. 46 3
Google Scholar
[44] Wang R, Yang Z S, Li K D, Xu G S, Jia G Z, Niu G J, Nian F F, He T, Meng L Y, Lin X, Luo G N, Wang L 2022 Phys. Plasmas 29 112502
Google Scholar
[45] Hechtl E, Bohdansky J, Roth J 1981 J. Nucl. Mater. 103 333
Google Scholar
[46] Gao S L, Liu X J, Deng G Z, Ming T F, Li G Q, Zhang X X, Wu X D, Wu X H, Li B, Fan H C, Gao X 2022 Plasma Sci. Technol. 24 075104
Google Scholar
[47] 欧靖, 杨锦宏 2012 61 075201
Google Scholar
Ou J, Yang J H 2012 Acta Phys. Sin. 61 075201
Google Scholar
[48] Summers H P, O'Mullane M G 2000 AIP Conference Proceedings 543 304
Google Scholar
[49] Putterich T, Fable E, Dux R, O'Mullane M, Neu R, Siccinio M 2019 Nucl. Fusion 59 056013
Google Scholar
下载:
计量
- 文章访问数: 2544
- PDF下载量: 102
- 被引次数: 0
