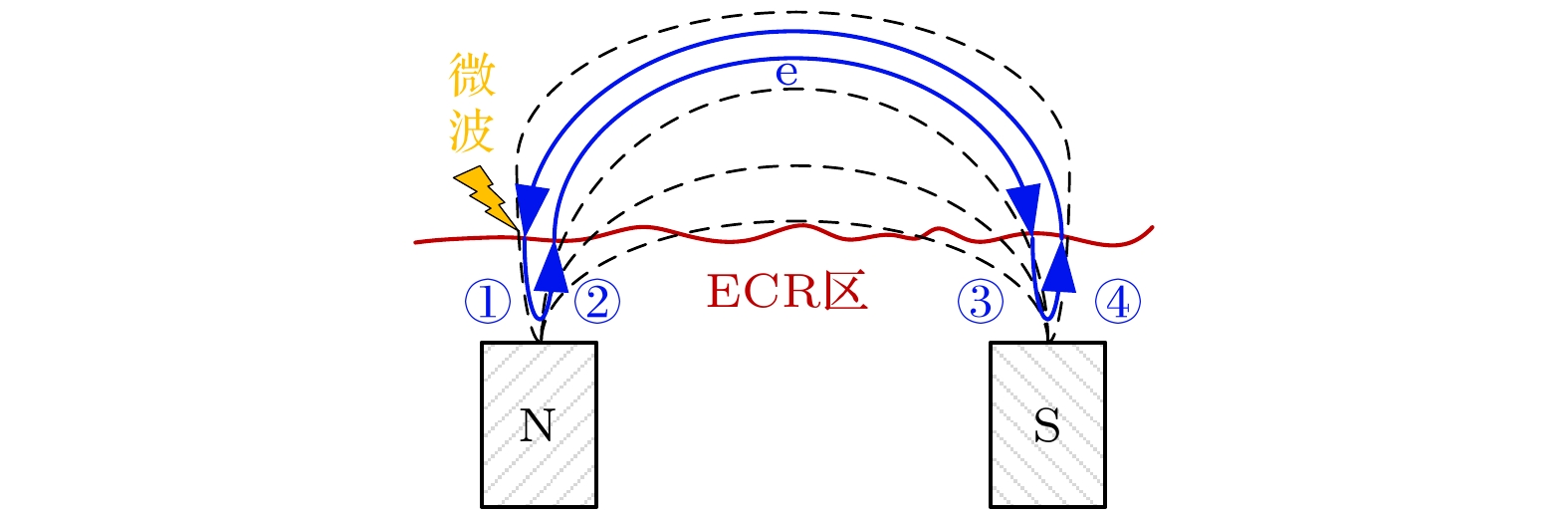
-
在电子回旋共振离子推力器的结构优化中, 放电室长度调节的是栅极与主等离子体区的相对位置, 以此影响栅极上游等离子体密度, 进而改变推力器离子束流大小及聚焦状态, 达到性能优化目的. 然而, 在一体化仿真研究中发现, 施加栅极电压后, Child-Langmuir鞘层前存在高能电子分布, 这与传统的放电室仿真存在明显差异. 本文认为施加栅极电压后, Child-Langmuir鞘层会排斥电子, 使流向栅极的电子返回磁镜区参与加热, 最终在磁镜和Child-Langmuir鞘层之间形成了高能电子分布区域. 这意味着放电室长度对推力器性能的影响不再局限于相对位置的调节, 还能通过调控Child-Langmuir鞘层前的高能电子分布影响等离子体生成. 因此, 本文采用一体化仿真方法, 系统研究了放电室长度对推力器放电和引出性能的影响机理, 并讨论了Child-Langmuir鞘层前高能电子分布对电离体系的影响. 本文研究将为电子回旋共振离子推力器的结构优化设计提供新思路.Discharge chamber length is one of the factors in optimizing the electron cyclotron resonance ion thruster performance. It adjusts the distance between bulk plasma and grid system to change the plasma density upstream of the screen grid, which will affect the ion beam current and focusing state to achieve optimization purpose. However, new evidence shows the discharge chamber length plays an important role in ionization during ion beam extraction, which means that the effect of discharge chamber length on the performance of electron cyclotron resonance ion thruster should be reexamined. After applying grid voltages, another high electron temperature region located upstream of the screen grid is observed in the integrated simulation using particle-in-cell with Monte Carlo collision method, but it is not observed in the traditional discharge chamber simulation. It is believed in the paper that the high electron temperature region exists objectively, because the Child-Langmuir sheath will repel electrons moving towards screen grid back to magnetic mirrors again. Those electrons will gain energy from microwave, and finally form a high electron temperature region along the Child-Langmuir sheath. This phenomenon implies that discharge chamber length can adjust the high electron temperature distribution upstream of screen grid to affect the plasma generation. Therefore, in this work, the effect of discharge chamber length on discharge and ion beam performance is systematically studied by adopting the integrated simulation. In this paper, three ion thrusters with different discharge chamber lengths are simulated. Under the conditions of same magnetic field and operation parameters, the comparisons of electron energy gain, plasma parameter distributions and ion beam current among the three ion thrusters are conducted. The results show that shorter discharge chamber length has higher electron energy gain, plasma density and voltage, but smaller ion beam current. This abnormal phenomenon can also be seen experimentally. By analyzing the ionization rate inside the chamber, it can be seen that high-temperature electrons upstream of the screen grid have a significant contribution to ionization. And thus, a little bit longer discharge chamber length with lower plasma density inside the chamber has bigger ion beam current for having higher plasma density upstream of the screen grid. According to this phenomenon, an electron heating mode is proposed: electrons gain energy by reciprocating through the electron cyclotron resonance layer between the Child-Langmuir sheath and magnetic mirrors. This heating mode can be used as a supplement to the electronic constraints outside the magnetic mirrors to improve the energy utilization efficiency of the thruster, which can provide a new insight into the electron cyclotron resonance ion thruster design in the future.
-
Keywords:
- electron cyclotron resonance /
- ion thruster /
- discharge chamber length
[1] Levchenko I, Keidar M, Cantrell J, et al. 2018 Nature 562 7726
[2] Serjeant S, Elvis M and Tinetti G 2020 Nat. Astron. 4
[3] O’Reilly D, Herdrich G, and Kavanagh DF 2021 Aerospace 8 22
Google Scholar
[4] 于达仁, 乔磊, 蒋文嘉, 刘辉 2020 推进技术 41 1
Yu D R, Qiao L, Jiang W J, Liu H 2020 J. Propuls. Tech. 41 1
[5] 杨涓, 牟浩, 耿海, 吴先明 2023 推进技术 44 78
Yang J, Mou H, Geng H, Wu X M 2023 J. Propuls. Tech. 44 78
[6] Watanabe S, Tsuda Y, Yoshikawa M, Tanaka S, Saiki T, Nakazawa S 2017 Space Sci. Rev. 208 3
Google Scholar
[7] 韩罗峰, 朱康武, 黄文斌, 于学文, 张辰乙, 鲁超, 刘通, 李航, 黄静 2022 真空与低温 28 98
Google Scholar
Han L F, Zhu K W, Huang W B, Yu X W, Zhang C Y, Lu C, Liu T, Li H, Huang J 2022 Vacuum Cry. 28 98
Google Scholar
[8] Tani Y, Tsukizaki R, Koda D, Nishiyama K, Kuninaka H 2019 Acta Astronaut. 157
[9] 夏旭, 杨涓, 付瑜亮, 吴先明, 耿海, 胡展 2021 70 075204
Google Scholar
Xia X, Yang J, Fu Y L, Wu X M, Geng H, Hu Z 2021 Acta Phys. Sin. 70 075204
Google Scholar
[10] Xia X, Yang J, Jin Y Z, Hang G R, Fu Y L, Hu Z 2020 Vacuum 179 109517
Google Scholar
[11] 夏旭, 杨涓, 金逸舟, 杭观荣, 付瑜亮, 胡展 2019 68 235202
Google Scholar
Xia X, Yang J, Jin Y Z, Hang G R, Fu Y L, Hu Z 2019 Acta Phys. Sin. 68 235202
Google Scholar
[12] Motoki T, Takasaki D, Koizumi H, Ataka Y, Komurasaki K, Takao Y 2022 Acta Astronaut. 196
[13] Fu S H, Ding Z F 2022 IEEE Tran. Pla. Sci. 50 6
[14] 汤明杰, 杨涓, 金逸舟, 罗立涛, 冯冰冰 2015 64 215202
Google Scholar
Tang M J, Yang J, Jin Y Z, Luo L T, Feng B B 2015 Acta Phys. Sin. 64 215202
Google Scholar
[15] 夏旭 2022 博士学位论文(西安: 西北工业大学)
Xia X 2022 Ph. D. Dissertation (Xian: Northwestern Polytechnical University) (in Chinese)
[16] 付瑜亮 2022 博士学位论文(西安: 西北工业大学)
Fu Y L 2022 Ph. D. Dissertation (Xian: Northwestern Polytechnical University) (in Chinese)
[17] 迈克尔 A. 力伯曼, 阿伦 J. 里登伯格 著 (蒲以康 译) 2007 等离子体放电原理与材料处理 (北京: 科学出版社 ) 第379—383页
Lieberman M A, Lichtenberg A J (translated by Pu Y K) 2007 Principles of Plasma Discharges and Materials Processing (Beijing: Science Press) pp379–383 (in Chinese)
[18] Fu Y L, Yang J, Mou H, Tan R W, Xia X, Gao Z Y 2022 Comput. Phys. Commun. 278 8395
[19] 付瑜亮, 杨涓, 王彬, 胡展, 夏旭, 牟浩 2022 71 085203
Google Scholar
Fu Y L, Yang J, Wang B, Hu Z, Xia X, Mou H 2022 Acta Phys. Sin. 71 085203
Google Scholar
[20] Yamashita Y, Tsukizaki R, and Nishiyama K 2022 Vacuum 200 110962
Google Scholar
[21] Yamashita Y, Tsukizaki R and Nishiyama K 2021 Plasma Sources Sci. Technol. 30 5023
-
图 4 不同L的电子获能对比 (a) L = 7.6 mm; (b) L = 8.6 mm;
$ \left({\rm{c}}\right)L=9.6{\rm{ }}{\rm{m}}{\rm{m}} $ Fig. 4. Comparison of electronic energy gain for different L: (a) L = 7.6 mm; (b) L = 8.6 mm;
$ \left({\rm{c}}\right)L=9.6{\rm{ }}{\rm{m}}{\rm{m}}$ 
图 5 放电阶段的离子分布 (a) L = 7.6 mm,
${\varphi }_{{\rm{s}}{\rm{c}}} = 0~{\rm{V}};$ (b) L = 8.6 mm,$ {\varphi }_{{\rm{s}}{\rm{c}}}=0\;{\rm{V}};\;\left({\rm{c}}\right)L=9.6{\rm{ }}{\rm{m}}{\rm{m}},\; {\varphi }_{{\rm{s}}{\rm{c}}}=0\;{\rm{V}} $ Fig. 5. Ion distributions in discharge stage: (a) L = 7.6 mm,
$ {\varphi }_{{\rm{s}}{\rm{c}}} = 0\;{\rm{V}}; $ (b) L = 8.6 mm,${\varphi }_{{\rm{sc}}} = 0\;{\rm{V}};\;({\rm{c}})\; L = 9.6\;{\rm{mm}},\; {\varphi }_{{\rm{sc}}} = 0\;{\rm{V}}$ 
图 6 放电阶段的电势分布 (a) L = 7.6 mm,
${\varphi }_{{\rm{s}}{\rm{c}}}=0\;{\rm{V}};$ (b) L = 8.6 mm,${\varphi }_{{\rm{s}}{\rm{c}}}=0\;{\rm{V}};\;({\rm{c}})~ L = 9.6{\rm{ }}{\rm{m}}{\rm{m}}, \;{\varphi }_{{\rm{s}}{\rm{c}}}=0\;{\rm{V}}$ Fig. 6. Potential distributions in discharge stage: (a) L = 7.6 mm,
${\varphi }_{{\rm{s}}{\rm{c}}}=0~{\rm{V}};$ (b) L = 8.6 mm,${\varphi }_{{\rm{s}}{\rm{c}}} = 0\;{\rm{V}};\;\left({\rm{c}}\right)L=9.6{\rm{ }}{\rm{m}}{\rm{m}}, \;{\varphi }_{{\rm{s}}{\rm{c}}} = 0\;{\rm{V}}$ 
图 7 等离子体演化过程中天线的累积电荷量
Fig. 7. Charges accumulating on antenna during plasma evolution.
图 8 引出阶段的离子分布 (a) L = 7.6 mm,
$ {\varphi }_{{\rm{s}}{\rm{c}}}=300\;{\rm{V}}; $ (b) L = 8.6 mm,${\varphi }_{{\rm{s}}{\rm{c}}}=300\;{\rm{V}};$ $\left({\rm{c}}\right)L=9.6{\rm{ }}{\rm{m}}{\rm{m}}, $ ${\varphi }_{{\rm{s}}{\rm{c}}}=$ 300 VFig. 8. Ion distributions in extraction stage: (a) L = 7.6 mm,
$ {\varphi }_{{\rm{s}}{\rm{c}}}=300\;{\rm{V}}; $ (b) L = 8.6 mm,${\varphi }_{{\rm{s}}{\rm{c}}}=300\;{\rm{V}};$ $({\rm{c}})~L=9.6~{\rm{mm}},$ ${\varphi }_{{\rm{s}}{\rm{c}}}=300\;{\rm{V}}$ .
图 10 电子温度分布 (a) L = 7.6 mm,
${\varphi }_{{\rm{s}}{\rm{c}}}=0\;{\rm{V}};$ (b) L = 8.6 mm,${\varphi }_{{\rm{s}}{\rm{c}}}=0\;{\rm{V}};\;\left({\rm{c}}\right)L=9.6\;{\rm{m}}{\rm{m}},$ ${\varphi }_{{\rm{s}}{\rm{c}}}=0\;{\rm{V}};\;\left({\rm{d}}\right)L=7.6\;{\rm{m}}{\rm{m}},$ ${\varphi }_{{\rm{s}}{\rm{c}}}=300\;{\rm{V}};\; $ $ \left({\rm{e}}\right)L= 8.6\;{\rm{m}}{\rm{m}},$ ${\varphi }_{{\rm{s}}{\rm{c}}}=300\;{\rm{V}};\;\left({\rm{f}}\right)L=9.6\;{\rm{m}}{\rm{m}}, {\varphi }_{{\rm{s}}{\rm{c}}}=300{\rm{V}}$ Fig. 10. Electron temperature distributions: (a) L = 7.6 mm,
${\varphi }_{{\rm{s}}{\rm{c}}}=0\;{\rm{V}};$ (b) L = 8.6 mm,${\varphi }_{{\rm{s}}{\rm{c}}}=0\;{\rm{V}};\;\left({\rm{c}}\right)L=9.6\;{\rm{m}}{\rm{m}},$ ${\varphi }_{{\rm{s}}{\rm{c}}}=0\;{\rm{V}}; $ $ \;\left({\rm{d}}\right)L=7.6\;{\rm{m}}{\rm{m}},$ ${\varphi }_{{\rm{s}}{\rm{c}}}=300\;{\rm{V}};\;\left({\rm{e}}\right)L=8.6\;{\rm{m}}{\rm{m}},$ ${\varphi }_{{\rm{s}}{\rm{c}}}=300\;{\rm{V}};\;\left({\rm{f}}\right)L=9.6\;{\rm{m}}{\rm{m}},$ ${\varphi }_{{\rm{s}}{\rm{c}}}=300\;{\rm{V}}$ .
图 11
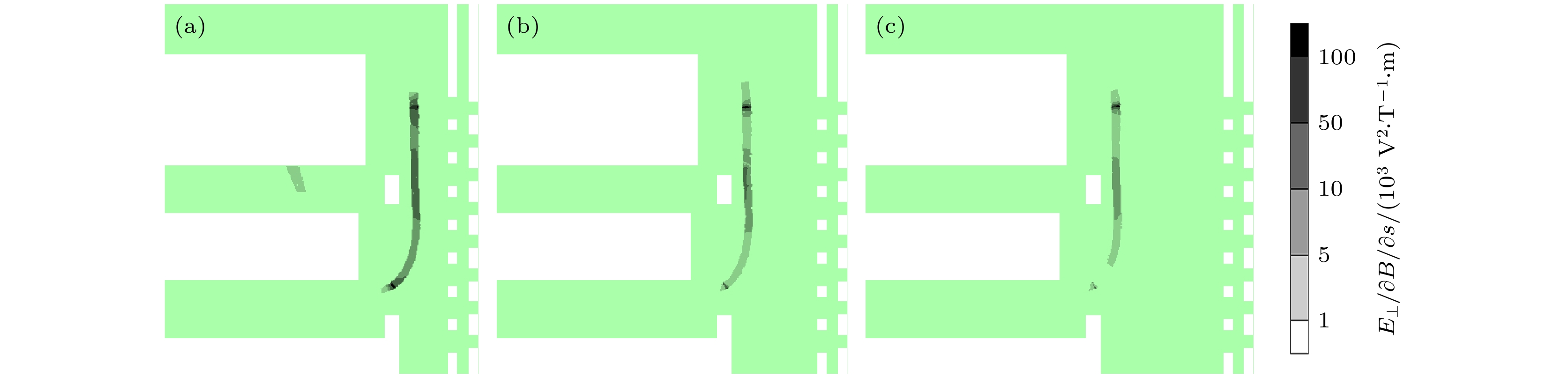
$ {\varphi }_{{\rm{s}}{\rm{c}}} $ = 300 V和$ {\varphi }_{{\rm{s}}{\rm{c}}} $ = 0 V的电离率分布对比 (a) L = 7.6 mm; (b) L = 8.6 mm;$ \left({\rm{c}}\right)L=9.6{\rm{ }}{\rm{m}}{\rm{m}} $ Fig. 11. Comparison of ionization rate distributions between
${\varphi }_{{\rm{sc}}}$ = 300 V with${\varphi }_{{\rm{sc}}}$ = 0 V (a) L = 7.6 mm; (b) L = 8.6 mm;$ \left({\rm{c}}\right)L=9.6{\rm{ }}{\rm{m}}{\rm{m}} $ -
[1] Levchenko I, Keidar M, Cantrell J, et al. 2018 Nature 562 7726
[2] Serjeant S, Elvis M and Tinetti G 2020 Nat. Astron. 4
[3] O’Reilly D, Herdrich G, and Kavanagh DF 2021 Aerospace 8 22
Google Scholar
[4] 于达仁, 乔磊, 蒋文嘉, 刘辉 2020 推进技术 41 1
Yu D R, Qiao L, Jiang W J, Liu H 2020 J. Propuls. Tech. 41 1
[5] 杨涓, 牟浩, 耿海, 吴先明 2023 推进技术 44 78
Yang J, Mou H, Geng H, Wu X M 2023 J. Propuls. Tech. 44 78
[6] Watanabe S, Tsuda Y, Yoshikawa M, Tanaka S, Saiki T, Nakazawa S 2017 Space Sci. Rev. 208 3
Google Scholar
[7] 韩罗峰, 朱康武, 黄文斌, 于学文, 张辰乙, 鲁超, 刘通, 李航, 黄静 2022 真空与低温 28 98
Google Scholar
Han L F, Zhu K W, Huang W B, Yu X W, Zhang C Y, Lu C, Liu T, Li H, Huang J 2022 Vacuum Cry. 28 98
Google Scholar
[8] Tani Y, Tsukizaki R, Koda D, Nishiyama K, Kuninaka H 2019 Acta Astronaut. 157
[9] 夏旭, 杨涓, 付瑜亮, 吴先明, 耿海, 胡展 2021 70 075204
Google Scholar
Xia X, Yang J, Fu Y L, Wu X M, Geng H, Hu Z 2021 Acta Phys. Sin. 70 075204
Google Scholar
[10] Xia X, Yang J, Jin Y Z, Hang G R, Fu Y L, Hu Z 2020 Vacuum 179 109517
Google Scholar
[11] 夏旭, 杨涓, 金逸舟, 杭观荣, 付瑜亮, 胡展 2019 68 235202
Google Scholar
Xia X, Yang J, Jin Y Z, Hang G R, Fu Y L, Hu Z 2019 Acta Phys. Sin. 68 235202
Google Scholar
[12] Motoki T, Takasaki D, Koizumi H, Ataka Y, Komurasaki K, Takao Y 2022 Acta Astronaut. 196
[13] Fu S H, Ding Z F 2022 IEEE Tran. Pla. Sci. 50 6
[14] 汤明杰, 杨涓, 金逸舟, 罗立涛, 冯冰冰 2015 64 215202
Google Scholar
Tang M J, Yang J, Jin Y Z, Luo L T, Feng B B 2015 Acta Phys. Sin. 64 215202
Google Scholar
[15] 夏旭 2022 博士学位论文(西安: 西北工业大学)
Xia X 2022 Ph. D. Dissertation (Xian: Northwestern Polytechnical University) (in Chinese)
[16] 付瑜亮 2022 博士学位论文(西安: 西北工业大学)
Fu Y L 2022 Ph. D. Dissertation (Xian: Northwestern Polytechnical University) (in Chinese)
[17] 迈克尔 A. 力伯曼, 阿伦 J. 里登伯格 著 (蒲以康 译) 2007 等离子体放电原理与材料处理 (北京: 科学出版社 ) 第379—383页
Lieberman M A, Lichtenberg A J (translated by Pu Y K) 2007 Principles of Plasma Discharges and Materials Processing (Beijing: Science Press) pp379–383 (in Chinese)
[18] Fu Y L, Yang J, Mou H, Tan R W, Xia X, Gao Z Y 2022 Comput. Phys. Commun. 278 8395
[19] 付瑜亮, 杨涓, 王彬, 胡展, 夏旭, 牟浩 2022 71 085203
Google Scholar
Fu Y L, Yang J, Wang B, Hu Z, Xia X, Mou H 2022 Acta Phys. Sin. 71 085203
Google Scholar
[20] Yamashita Y, Tsukizaki R, and Nishiyama K 2022 Vacuum 200 110962
Google Scholar
[21] Yamashita Y, Tsukizaki R and Nishiyama K 2021 Plasma Sources Sci. Technol. 30 5023
下载:
计量
- 文章访问数: 2410
- PDF下载量: 58
- 被引次数: 0
