-
准一维混合自旋(1/2, 5/2) Ising-XXZ模型可以用来研究一些材料(如异质三金属化合物Fe-Mn-Cu)的磁性质, 该研究也有助于这类材料在量子信息等领域的应用. 本文利用转移矩阵法计算了该模型的量子相干和互信息, 讨论了Ising作用、温度和磁场对其的影响. 结果表明, 在极低温度下随Ising作用的增强量子相干逐渐减小, 而互信息在各向同性系统中存在一个极小值, 在各向异性系统
$\left( {\varDelta = 4} \right)$ 中存在多个极小值. 进一步研究发现, 量子相干和互信息在量子临界点存在突变, 其一阶导数在该点存在奇异行为. 还研究了有限温度下的量子相干和互信息, 当磁场较弱时, 两者随温度的升高单调减小; 当磁场较强时, 热涨落与磁场的竞争使得两者随温度的升高先增大后减小. 相比于量子互信息, 量子相干存在于更大的磁场和温度范围内, 有利于在实验中对其进行调控.-
关键词:
- 量子相干 /
- 量子互信息 /
- 量子相变 /
- Ising-XXZ模型 /
- 转移矩阵法
The mixed spin-(1/2, 5/2) Ising-XXZ model on quasi-one-dimensional lattices can be used to study the properties of some materials (such as heterotrimetallic Fe-Mn-Cu coordination polymer), and the study on this model is beneficial to the practical applications of such materials in the field of quantum information. The quantum coherence and mutual information are calculated by the transfer matrix method, and the effects of Ising interaction, temperature and magnetic field on them are discussed. The results show that the quantum coherence decreases gradually with the increase of Ising interaction at extremely low temperatures, while there is one minimum value of mutual information in an isotropic system and there appear four minimum values in an anisotropic$\left( {\varDelta = 4} \right)$ system. Furthermore, quantum coherence and mutual information jump abruptly at quantum phase transition points where the first derivatives of them exhibit singular behaviors. The quantum coherence and mutual information at finite temperatures are also studied. As the temperature increases, they decrease monotonically in a weak magnetic field, but they first increase and then decrease in a higher magnetic field, which is caused by the competition between thermal fluctuation and magnetic field. Compared with quantum mutual information, quantum coherence exists over a wider range of magnetic field and temperature, which can be easily manipulated experimentally.-
Keywords:
- quantum coherence /
- quantum mutual information /
- quantum phase transition /
- Ising-XXZ model /
- transfer matrix method
[1] Amico L, Fazio R, Osterloh A, Vedral V 2008 Rev. Mod. Phys. 80 517
Google Scholar
[2] Nielsen M A, Chuang I L 2000 Quantum Computation and Quantum Information (Cambridge: Cambridge University Press) pp1–665
[3] Cui X D, Du M M, Tong D M 2020 Phys. Rev. A 102 032419
Google Scholar
[4] Baumgratz T, Cramer M, Plenio M B 2014 Phys. Rev. Lett. 113 140401
Google Scholar
[5] Hu M L, Hu X Y, Wang J C, Peng Y, Zhang Y R, Fan H 2018 Phys. Rep. 762 1
[6] Lloyd S 2011 J. Phys. Conf. Ser. 302 012037
Google Scholar
[7] Lambert N, Chen Y N, Cheng Y C, Li C M, Chen G Y, Nori F 2013 Nat. Phys. 9 10
Google Scholar
[8] Lostaglio M, Jennings D, Rudolph T 2015 Nat. Commun. 6 6383
Google Scholar
[9] Aberg J 2014 Phys. Rev. Lett. 113 150402
Google Scholar
[10] Shannon C E 1948 Bell Syst. Tech. J. 27 379
Google Scholar
[11] Henderson L, Vedral V 2001 J. Phys. A: Math. Gen. 34 6899
Google Scholar
[12] Ollivier H, Zurek W H 2001 Phys. Rev. Lett. 88 017901
Google Scholar
[13] Osterloh A, Amico L, Falci G, Fazio R 2002 Nature 416 608
Google Scholar
[14] Ma F W, Liu S X, Kong X M 2011 Phys. Rev. A 84 042302
Google Scholar
[15] Gu S J, Lin H Q, Li Y Q 2003 Phys. Rev. A 68 042330
Google Scholar
[16] Osborne T J, Nielsen M A 2002 Phys. Rev. A 66 032110
Google Scholar
[17] Glaser U, Büttner H, Fehske H 2003 Phys. Rev. A 68 032318
Google Scholar
[18] Karpat G, Çakmak B, Fanchini F F 2014 Phys. Rev. B 90 104431
Google Scholar
[19] Sun W Y, Wang D, Ye L 2017 Physica B 524 27
Google Scholar
[20] Lei S G, Tong P Q 2016 Quantum Inf. Process. 15 1811
Google Scholar
[21] Malvezzi A L, Karpat G, Cakmak B, Fanchini F F, Debarba T, Vianna R O 2016 Phys. Rev. B 93 184428
Google Scholar
[22] Hu M L, Gao Y Y, Fan H 2020 Phys. Rev. A 101 032305
Google Scholar
[23] Valdez M A, Jaschke D, Vargas D L, Carr L D 2017 Phys. Rev. Lett. 119 225301
Google Scholar
[24] 伊天成, 丁悦然, 任杰, 王艺敏, 尤文龙 2018 67 140303
Google Scholar
Yi T C, Ding Y R, Ren J, Wang Y M, You W L 2018 Acta Phys. Sin. 67 140303
Google Scholar
[25] Qin M, Ren Z Z, Zhang X 2018 Phys. Rev. A 98 012303
Google Scholar
[26] Thakur P, Durganandini P 2020 Phys. Rev. B 102 064409
Google Scholar
[27] Mao R, Dai Y W, Cho S Y, Zhou H Q 2021 Phys. Rev. B 103 014446
Google Scholar
[28] Li C X, Yang S, Xu J B, Lin H Q 2023 Phys. Rev. B 107 085130
Google Scholar
[29] Dai Y W, Chen X H, Cho S Y, Zhou H Q 2021 Phys. Rev. E 104 044137
Google Scholar
[30] Dong J J, Huang D, Yang Y f 2021 Phys. Rev. B 104 L081115
Google Scholar
[31] Li Y C, Lin H Q 2016 Sci. Rep. 6 26365
Google Scholar
[32] Sha Y T, Wang Y, Sun Z H, Hou X W 2018 Ann. Phys. 392 229
Google Scholar
[33] Yin S Y, Song J, Liu S T, Song G L 2021 Phys. Rev. A 389 127089
[34] Chen J J, Cui J, Zhang Y R, Fan H 2016 Phys. Rev. A 94 022112
Google Scholar
[35] Li Y C, Zhang J, Lin H Q 2020 Phys. Rev. B 101 115142
Google Scholar
[36] Iaconis J, Inglis S, Kallin A B, Melko R G 2013 Phys. Rev. B 87 195134
Google Scholar
[37] Wilms J, Vidal J, Verstraete F, Dusuel S 2012 J. Stat. Mech. Theory Exp. 2012 P01023
Google Scholar
[38] Walsh C, Semon P, Poulin D, Sordi G, Tremblay A S 2019 Phys. Rev. Lett. 122 067203
Google Scholar
[39] Wilms J, Troyer M, Verstraete F 2011 J. Stat. Mech. Theory Exp. 2011 P10011
Google Scholar
[40] Souza F, Lyra M L, Strečka J, Pereira M S S 2019 J. Magn. Magn. Mater. 471 423
Google Scholar
[41] Wang H, Zhang L F, Ni Z H, Zhong W F, Tian L J, Jiang J 2010 Cryst. Growth Des. 10 4231
Google Scholar
[42] Zad H A, Rojas M 2021 Physica E 126 114455
[43] Zheng Y D, Mao Z, Zhou B 2019 Chin. Phys. B 28 120307
[44] Souza F, Veríssimo L M, Strečka J, Lyra M L, Pereira M S S 2020 Phys. Rev. B 102 064414
Google Scholar
[45] Baxter R J 2016 Exactly Solved Models in Statistical Mechanics (San Diego: Academic Press ) pp1—482
[46] Rojas O, Rojas M, Ananikian N S, de Souza S M 2012 Phys. Rev. A 86 042330
Google Scholar
[47] Gao K, Xu Y L, Kong X M, Liu Z Q 2015 Physica A 429 10
Google Scholar
[48] Carvalho I M, Rojas O, de Souza S M, Rojas M 2019 Quantum Inf. Process. 18 134
Google Scholar
[49] Torrico J, Rojas M, de Souza S M, Rojas O, Ananikian N S 2014 Europhys. Lett. 108 50007
Google Scholar
-
图 1 异质三金属化合物Fe-Mn-Cu的磁性结构[40]. 虚线表示Fe3+和Mn2+之间的Ising作用J1, 实线表示Mn2+和Cu2+之间的Heisenberg作用J2. 实线圆圈包围的自旋是第
$ i $ 个自旋块Fig. 1. Magnetic structure of heterotrimetallic Fe-Mn-Cu coordination polymer[40]. The dashed lines denote Ising couplings J1 between Fe3+ and Mn2+, while the solid lines denote Heisenberg couplings J2 between Mn2+ and Cu2+. The i-th spin block consists the spins surrounded by the solid-line circle.
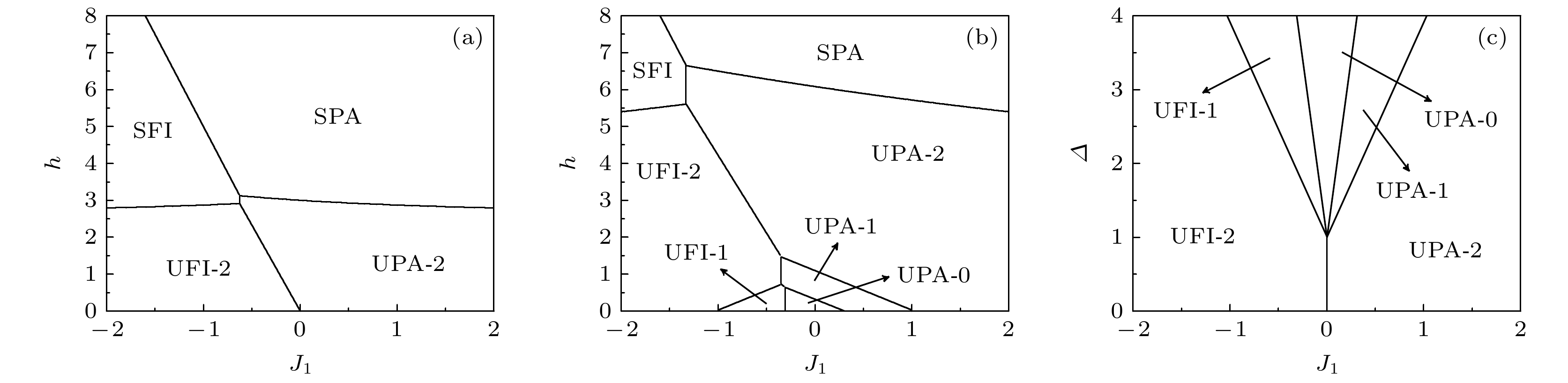
图 2 准一维混合自旋(1/2, 5/2) Ising-XXZ系统的基态相图[44] (a) Δ = 1时, 系统存在4个相UPA-2, SPA, UFI-2和SFI; (b) Δ = 4时, 产生了3个新的相UPA-1, UPA-0和UFI-1; (c) h = 0时, 系统存在5个相UPA-2, UPA-1, UPA-0, UFI-2和 UFI-1
Fig. 2. Ground-state phase diagrams of quasi-one-dimensional mixed spin-(1/2, 5/2) Ising-XXZ system[44]: (a) There are four phases UPA-2, SPA, UFI-2 and SFI for Δ = 1; (b) three new phases UPA-1, UPA-0 and UFI-1 emerge for Δ = 4; (c) there are five phases UPA-2, UPA-1, UPA-0, UFI-2 and UFI-1 for h = 0.
图 3 Δ = 1, h = 0时, 量子相干
$ {C_{{l_1}}} $ 和互信息$ \mathcal{I} $ 及其一阶导数在一定温度下随$ {J_1} $ 的变化 (a), (b)极低温情况下,$ {C_{{l_1}}} $ 在$ {J_1} = 0 $ 时存在极大值,$ \mathcal{I} $ 存在极小值; (c), (d) T = 0.01时,$ {C_{{l_1}}} $ 和$ \mathcal{I} $ 的一阶导数存在奇异行为, 随着温度的升高奇异行为消失Fig. 3. Quantum coherence
$ {C_{{l_1}}} $ , mutual information$ \mathcal{I} $ and their first derivatives as functions of$ {J_1} $ for various temperatures at Δ = 1 and h = 0: (a), (b) At extremely low temperatures, there are a maximum at$ {J_1} = 0 $ for$ {C_{{l_1}}} $ and a minimum for$ \mathcal{I} $ ; (c), (d) when T = 0.01, the first derivatives of$ {C_{{l_1}}} $ and$ \mathcal{I} $ exhibit singular behaviors which disappear as the temperature increases.
图 4 Δ = 1,
$ {J_1} = - 0.5 $ 时, 量子相干$ {C_{{l_1}}} $ 和互信息$ \mathcal{I} $ 及其一阶导数在一定温度下随磁场的变化 (a), (b) T = 0.01时,$ {C_{{l_1}}} $ 和$ \mathcal{I} $ 在h = 2.4和3.1时存在不连续跃变; (c), (d)$ {C_{{l_1}}} $ 和$ \mathcal{I} $ 的一阶导数在量子相变点存在奇异行为Fig. 4. Quantum coherence
$ {C_{{l_1}}} $ , mutual information$ \mathcal{I} $ and their first derivatives with respect to magnetic field for various temperatures at Δ = 1 and$ {J_1} = - 0.5 $ : (a), (b) Discontinuous jumps of$ {C_{{l_1}}} $ and$ \mathcal{I} $ occur at h = 2.4 and 3.1 when T = 0.01; (c), (d) first derivatives of$ {C_{{l_1}}} $ and$ \mathcal{I} $ exhibit singular behaviors at the quantum phase transition points.
图 5 Δ = 1,
$ {J_1} = - 0.5 $ 时, 量子相干$ {C_{{l_1}}} $ 和互信息$ \mathcal{I} $ 随温度和磁场的变化 (a), (b)当h < 3.1时,$ {C_{{l_1}}} $ 和$ \mathcal{I} $ 随T的增大逐渐减小, 而当h > 3.1时,$ {C_{{l_1}}} $ 和$ \mathcal{I} $ 先增大后减小; (c), (d) 当T > 5时,$ {C_{{l_1}}} $ 仍存在有效值, 而$ \mathcal{I} $ 几乎为0Fig. 5. Variations of quantum coherence
$ {C_{{l_1}}} $ and mutual information$ \mathcal{I} $ with temperature and magnetic field at Δ = 1 and$ {J_1} = - 0.5 $ : (a), (b) For h < 3.1,$ {C_{{l_1}}} $ and$ \mathcal{I} $ gradually decrease with increasing T, while increase firstly and then decrease for h > 3.1; (c), (d)$ {C_{{l_1}}} $ still keeps limited strength while$ \mathcal{I} $ approaches to zero in the region of T > 5.
图 6 Δ = 4,
$ h = 0 $ 时, 量子相干$ {C_{{l_1}}} $ 和互信息$ \mathcal{I} $ 及其一阶导数随Ising作用$ {J_1} $ 的变化 (a)$ {C_{{l_1}}} $ 随$ {J_1} $ 的增大逐渐减小, 当温度趋于0时存在突变行为; (b)$ \mathcal{I} $ 随$ {J_1} $ 的增大存在4个极小值, 随着温度降低,$ \mathcal{I} $ 逐渐增大, 其极小值点逐渐趋于量子相变点; (c), (d)$ {C_{{l_1}}} $ 和$ \mathcal{I} $ 的一阶导数在量子相变点存在奇异行为Fig. 6. Ising interaction dependence of quantum coherence
$ {C_{{l_1}}} $ , mutual information$ \mathcal{I} $ and their first derivatives for several values of temperature at Δ = 4 and h = 0: (a) As$ {J_1} $ increases,$ {C_{{l_1}}} $ gradually decreases, and jumps abruptly when T approaches to 0; (b) there are 4 minima for$ \mathcal{I} $ as$ {J_1} $ increases, as T nears to 0,$ \mathcal{I} $ gradually increases, and its minimum points gradually approach to the phase transition points; (c), (d) the first derivatives of$ {C_{{l_1}}} $ and$ \mathcal{I} $ exhibit singular behaviors at quantum phase transition points.
图 7 Δ = 4,
$ {J_1} = - 0.5 $ 时, 量子相干$ {C_{{l_1}}} $ 和互信息$ \mathcal{I} $ 及其一阶导数随磁场的变化 (a) 当T = 0.01时$ {C_{{l_1}}} $ 随h的增大发生3次突变, 随着温度升高, 突变行为消失(插图给出了$ 0 \leqslant h \leqslant 3 $ 时$ {C_{{l_1}}} $ 随h的变化); (b)当T = 0.01时$ \mathcal{I} $ 随h的增大发生3次突变, 在h = 0.6时达到极小值, 随着温度升高, 突变行为消失, 极小值逐渐减小; (c), (d)$ {C_{{l_1}}} $ 和$ \mathcal{I} $ 的一阶导数在量子相变点存在奇异行为(图(c)插图给出了$ 0 \leqslant h \leqslant 3 $ 时$ {C_{{l_1}}} $ 的一阶导数随h的变化)Fig. 7. Quantum coherence
$ {C_{{l_1}}} $ , mutual information$ \mathcal{I} $ and their first derivatives with respect to magnetic field at Δ = 4 and$ {J_1} = - 0.5 $ : (a) For T = 0.01,$ {C_{{l_1}}} $ and$ \mathcal{I} $ jump abruptly 3 times as h increases while changing smoothly with increasing T (Inset: the variations of$ {C_{{l_1}}} $ with h for 0 ≤ h ≤ 3); (b)$ \mathcal{I} $ jumps abruptly 3 times as h increases, and reaches the minimum at h = 0.6 for T = 0.01, as T increases, the jump behaviors disappear, and the minimum gradually decreases; (c), (d) the first derivatives of$ {C_{{l_1}}} $ and$ \mathcal{I} $ exhibit singular behaviors at quantum phase transition points (Inset in panel (c) shows the behavior of the first derivative of$ {C_{{l_1}}} $ versus h for 0 ≤ h ≤ 3).
图 8 Δ = 4,
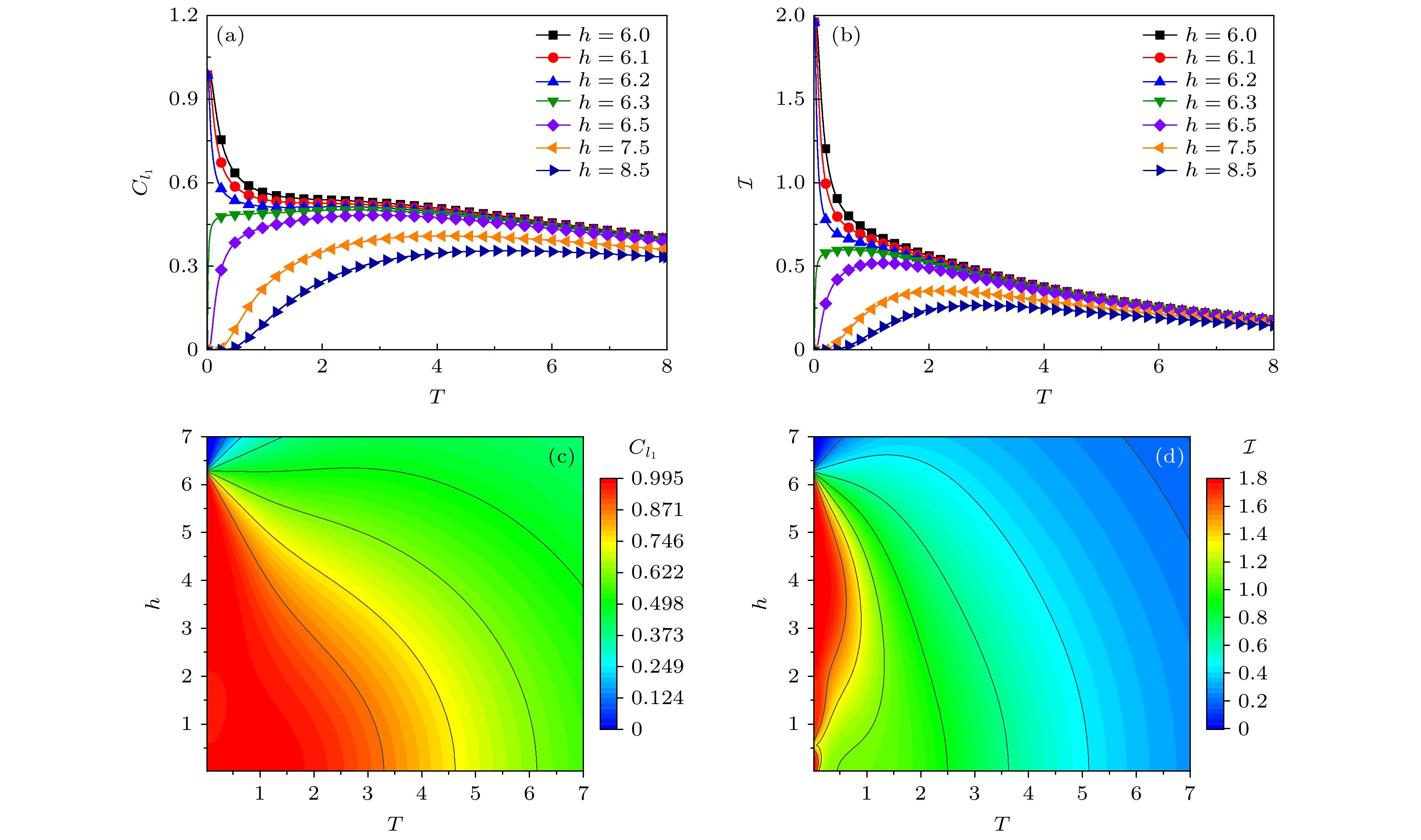
$ {J_1} = - 0.5 $ , 量子相干$ {C_{{l_1}}} $ 和互信息$ \mathcal{I} $ 随温度和磁场的变化 (a), (b)当$ h \geqslant 6.3 $ 时,$ {C_{{l_1}}} $ 和$ \mathcal{I} $ 随温度的升高先增大后减小; (c), (d)当$ T > 3 $ 时,$ {C_{{l_1}}} $ 和$ \mathcal{I} $ 随磁场的增强逐渐减小Fig. 8. Variations of quantum coherence
$ {C_{{l_1}}} $ and mutual information$ \mathcal{I} $ with T and h for Δ = 4 and$ {J_1} = - 0.5 $ : (a), (b)$ {C_{{l_1}}} $ and$ \mathcal{I} $ increase firstly and then decrease with increasing T in the region of$ h \geqslant 6.3 $ ; (c), (d) with the increase of h,$ {C_{{l_1}}} $ and$ \mathcal{I} $ gradually decrease for$ T > 3 $ . -
[1] Amico L, Fazio R, Osterloh A, Vedral V 2008 Rev. Mod. Phys. 80 517
Google Scholar
[2] Nielsen M A, Chuang I L 2000 Quantum Computation and Quantum Information (Cambridge: Cambridge University Press) pp1–665
[3] Cui X D, Du M M, Tong D M 2020 Phys. Rev. A 102 032419
Google Scholar
[4] Baumgratz T, Cramer M, Plenio M B 2014 Phys. Rev. Lett. 113 140401
Google Scholar
[5] Hu M L, Hu X Y, Wang J C, Peng Y, Zhang Y R, Fan H 2018 Phys. Rep. 762 1
[6] Lloyd S 2011 J. Phys. Conf. Ser. 302 012037
Google Scholar
[7] Lambert N, Chen Y N, Cheng Y C, Li C M, Chen G Y, Nori F 2013 Nat. Phys. 9 10
Google Scholar
[8] Lostaglio M, Jennings D, Rudolph T 2015 Nat. Commun. 6 6383
Google Scholar
[9] Aberg J 2014 Phys. Rev. Lett. 113 150402
Google Scholar
[10] Shannon C E 1948 Bell Syst. Tech. J. 27 379
Google Scholar
[11] Henderson L, Vedral V 2001 J. Phys. A: Math. Gen. 34 6899
Google Scholar
[12] Ollivier H, Zurek W H 2001 Phys. Rev. Lett. 88 017901
Google Scholar
[13] Osterloh A, Amico L, Falci G, Fazio R 2002 Nature 416 608
Google Scholar
[14] Ma F W, Liu S X, Kong X M 2011 Phys. Rev. A 84 042302
Google Scholar
[15] Gu S J, Lin H Q, Li Y Q 2003 Phys. Rev. A 68 042330
Google Scholar
[16] Osborne T J, Nielsen M A 2002 Phys. Rev. A 66 032110
Google Scholar
[17] Glaser U, Büttner H, Fehske H 2003 Phys. Rev. A 68 032318
Google Scholar
[18] Karpat G, Çakmak B, Fanchini F F 2014 Phys. Rev. B 90 104431
Google Scholar
[19] Sun W Y, Wang D, Ye L 2017 Physica B 524 27
Google Scholar
[20] Lei S G, Tong P Q 2016 Quantum Inf. Process. 15 1811
Google Scholar
[21] Malvezzi A L, Karpat G, Cakmak B, Fanchini F F, Debarba T, Vianna R O 2016 Phys. Rev. B 93 184428
Google Scholar
[22] Hu M L, Gao Y Y, Fan H 2020 Phys. Rev. A 101 032305
Google Scholar
[23] Valdez M A, Jaschke D, Vargas D L, Carr L D 2017 Phys. Rev. Lett. 119 225301
Google Scholar
[24] 伊天成, 丁悦然, 任杰, 王艺敏, 尤文龙 2018 67 140303
Google Scholar
Yi T C, Ding Y R, Ren J, Wang Y M, You W L 2018 Acta Phys. Sin. 67 140303
Google Scholar
[25] Qin M, Ren Z Z, Zhang X 2018 Phys. Rev. A 98 012303
Google Scholar
[26] Thakur P, Durganandini P 2020 Phys. Rev. B 102 064409
Google Scholar
[27] Mao R, Dai Y W, Cho S Y, Zhou H Q 2021 Phys. Rev. B 103 014446
Google Scholar
[28] Li C X, Yang S, Xu J B, Lin H Q 2023 Phys. Rev. B 107 085130
Google Scholar
[29] Dai Y W, Chen X H, Cho S Y, Zhou H Q 2021 Phys. Rev. E 104 044137
Google Scholar
[30] Dong J J, Huang D, Yang Y f 2021 Phys. Rev. B 104 L081115
Google Scholar
[31] Li Y C, Lin H Q 2016 Sci. Rep. 6 26365
Google Scholar
[32] Sha Y T, Wang Y, Sun Z H, Hou X W 2018 Ann. Phys. 392 229
Google Scholar
[33] Yin S Y, Song J, Liu S T, Song G L 2021 Phys. Rev. A 389 127089
[34] Chen J J, Cui J, Zhang Y R, Fan H 2016 Phys. Rev. A 94 022112
Google Scholar
[35] Li Y C, Zhang J, Lin H Q 2020 Phys. Rev. B 101 115142
Google Scholar
[36] Iaconis J, Inglis S, Kallin A B, Melko R G 2013 Phys. Rev. B 87 195134
Google Scholar
[37] Wilms J, Vidal J, Verstraete F, Dusuel S 2012 J. Stat. Mech. Theory Exp. 2012 P01023
Google Scholar
[38] Walsh C, Semon P, Poulin D, Sordi G, Tremblay A S 2019 Phys. Rev. Lett. 122 067203
Google Scholar
[39] Wilms J, Troyer M, Verstraete F 2011 J. Stat. Mech. Theory Exp. 2011 P10011
Google Scholar
[40] Souza F, Lyra M L, Strečka J, Pereira M S S 2019 J. Magn. Magn. Mater. 471 423
Google Scholar
[41] Wang H, Zhang L F, Ni Z H, Zhong W F, Tian L J, Jiang J 2010 Cryst. Growth Des. 10 4231
Google Scholar
[42] Zad H A, Rojas M 2021 Physica E 126 114455
[43] Zheng Y D, Mao Z, Zhou B 2019 Chin. Phys. B 28 120307
[44] Souza F, Veríssimo L M, Strečka J, Lyra M L, Pereira M S S 2020 Phys. Rev. B 102 064414
Google Scholar
[45] Baxter R J 2016 Exactly Solved Models in Statistical Mechanics (San Diego: Academic Press ) pp1—482
[46] Rojas O, Rojas M, Ananikian N S, de Souza S M 2012 Phys. Rev. A 86 042330
Google Scholar
[47] Gao K, Xu Y L, Kong X M, Liu Z Q 2015 Physica A 429 10
Google Scholar
[48] Carvalho I M, Rojas O, de Souza S M, Rojas M 2019 Quantum Inf. Process. 18 134
Google Scholar
[49] Torrico J, Rojas M, de Souza S M, Rojas O, Ananikian N S 2014 Europhys. Lett. 108 50007
Google Scholar
下载:
计量
- 文章访问数: 3367
- PDF下载量: 108
- 被引次数: 0
