-
拓扑电子材料因为具有非平庸的拓扑态, 所以会展现出许多奇异的物理性质. 本文通过第一性原理计算对应变调控下的烧绿石三元氧化物Tl2Ta2O7 中的拓扑相变进行了研究. 首先分析了原子轨道投影能带, 发现体系费米能级附近O原子的(px + py) 与pz 轨道发生了能带反转, 再构造了紧束缚模型计算得到体系的Z2 拓扑不变量确定了其拓扑非平庸性, 最后研究了表面态等拓扑性质. 研究发现未施加应变的Tl2Ta2O7是一个在费米能级处具有二次能带交叉点的半金属, 而平面内应变会破缺晶体对称性进而使体系发生拓扑相变. 当对体系施加–1%的压缩应变时, 它会转变为狄拉克半金属; 当对体系施加1%的拉伸应变时, 体系相变为拓扑绝缘体. 本研究对于在三维材料中调控拓扑相变有着较重要的指导意义, 并且为低能耗电子器件的设计提供了良好的材料平台.Topological electronic materials exhibit many novel physical properties, such as low dissipation transport and high carrier mobility. These extraordinary properties originate from their non-trivial topological electronic structures in momentum space. In recent years, topological phase transitions based on topological electronic materials have gradually become one of the hot topics in condensed matter physics. Using first-principles calculations, we explore the topological phase transitions driven by in-plane strain in ternary pyrochlore oxide Tl2Ta2O7. Firstly, we analyze the atomic-orbital-resolved band structure and find that the O (px+py) and pz orbitals of the system near the Fermi level have band inversion, indicating the emergence of topological phase transitions in the system. Then the tight-binding models are constructed to calculate the Z2 topological invariants, which can determine the topologically non-trivial feature of the system. Finally, topological properties such as surface states and a three-dimensional Dirac cone are studied. It is found that Tl2Ta2O7 without strain is a semimetal with a quadratic band touching point at Fermi level, while the in-plane strain can drive the topological phase transition via breaking crystalline symmetries. When the system is under the –1% in-plane compression strain and without considering the spin orbit coupling (SOC), the application of strain results in two triply degenerate nodal points formed in the –Z to Γ direction and Γ to Z direction, respectively. When the SOC is included, there are two fourfold degenerate Dirac points on the –Z to Γ path and Γ to Z path, respectively. Thus, the –1% in-plane compression strain makes the system transit from the quadratic contact point semimetal to a Dirac semimetal. When 1% in-plane expansion strain is applied and the SOC is neglected, there exists one band intersection along Y→Γ. When the SOC is taken into consideration, the gap is opened. Therefore, the 1% in-plane expansion strain drives Tl2Ta2O7 into a strong topological insulator. In addition, the system is also expected to have strong correlation effect and superconductivity due to the possible flat band. This work can guide the study of topological phase transitions in three-dimensional materials and provide a good material platform for the design of low-dissipation electronic devices.
-
Keywords:
- topological insulator /
- topological phase transition /
- Dirac semimetal
[1] Bernevig B A, Hughes T L, Zhang S C 2006 Science 314 1757
Google Scholar
[2] 王航天, 赵海慧, 温良恭, 吴晓君, 聂天晓, 赵巍胜 2020 69 200704
Google Scholar
Wang H T, Zhao H H, Wen L G, Wu X J, Nie T X, Zhao W S 2020 Acta Phys. Sin. 69 200704
Google Scholar
[3] Tian W, Yu W, Shi J, Wang Y 2017 Materials 10 814
Google Scholar
[4] Lü H, Wang Z, Cheng Q, Zhang W, Yu R 2021 Phys. Rev. B 103 L241115
Google Scholar
[5] Hu J, Xu S Y, Ni N, Mao Z 2019 Annu. Rev. Mater. Res. 49 207
Google Scholar
[6] Zhang W, Wu Q, Yazyev O V, Weng H, Guo Z, Cheng W D, Chai G L 2018 Phys. Rev. B 98 115411
Google Scholar
[7] Chen Z, Hu Y, Zhu Z, Zhang W 2020 New J. Phys. 22 093055
Google Scholar
[8] Yuan D, Hu Y, Yang Y, Zhang W 2021 Chin. Phys. Lett. 38 117301
Google Scholar
[9] Ferreira P P, Manesco A L R, Dorini T T, et al. 2021 Phys. Rev. B 103 125134
Google Scholar
[10] Cao W, Tang P, Xu Y, Wu J, Gu B L, Duan W 2017 Phys. Rev. B 96 115203
Google Scholar
[11] Jitta R R, Gundeboina R, Veldurthi N K, Guje R, Muga V 2015 J. Chem. Technol. Biot. 90 1937
Google Scholar
[12] Kim M, Park J, Kang M, Kim J Y, Lee S W 2020 ACS Cent. Sci. 6 880
Google Scholar
[13] Yang B J, Kim Y B 2010 Phys. Rev. B 82 085111
Google Scholar
[14] Wan X, Turner A M, Vishwanath A, Savrasov S Y 2011 Phys. Rev. B 83 205101
Google Scholar
[15] Sakata M, Kagayama T, Shimizu K, Matsuhira K, Takagi S, Wakeshima M, Hinatsu Y 2011 Phys. Rev. B 83 041102(R
Google Scholar
[16] Hase I, Yanagisawa T 2020 Symmetry 12 1076
Google Scholar
[17] Das M, Bhowal S, Sannigrahi J, et al. 2022 Phys. Rev. B 105 134421
Google Scholar
[18] Hu Y, Yue C, Yuan D, et al. 2022 Sci. China Phys. Mech. Astron. 65 297211
Google Scholar
[19] Zhang W, Luo K, Chen Z, Zhu Z, Yu R, Fang C, Weng H 2019 npj Comput. Mater. 5 105
Google Scholar
[20] Hase I, Yanagisawa T, Kawashima K 2019 Nanomaterials 9 876
Google Scholar
[21] Kresse G, Furthmüller J 1996 Comput. Mater. Sci. 6 15
Google Scholar
[22] Kresse G, Furthmüller J 1996 Phys. Rev. B 54 11169
Google Scholar
[23] Blöchl P E 1994 Phys. Rev. B 50 17953
Google Scholar
[24] Kresse G, Joubert D 1999 Phys. Rev. B 59 1758
Google Scholar
[25] Perdew J P, Burke K, Ernzerhof M 1996 Phys. Rev. Lett. 77 3865
Google Scholar
[26] Marzari N, Vanderbilt D 1997 Phys. Rev. B 56 12847
Google Scholar
[27] Souza I, Marzari N, Vanderbilt D 2001 Phys. Rev. B 65 035109
Google Scholar
[28] Wu Q, Zhang S, Song H F, Troyer M, Soluyanov A A 2018 Comput. Phys. Commun. 224 405
Google Scholar
[29] 杜永平, 刘慧美, 万贤纲 2015 64 187201
Google Scholar
Du Y P, Liu H M, Wan X G 2015 Acta Phys. Sin. 64 187201
Google Scholar
[30] Sheng X L, Wang Z, Yu R, Weng H, Fang Z, Dai X 2014 Phys. Rev. B 90 245308
Google Scholar
[31] Wang Z, Weng H, Wu Q, et al. 2013 Phys. Rev. B 88 125427
Google Scholar
[32] Cvetkovic V, Vafek O 2013 Phys. Rev. B 88 134510
Google Scholar
[33] Yakovkin I N, Petrova N V 2021 Phys. Lett. A 403 127398
Google Scholar
[34] Liu M Y, Gong L, He Y, Cao C 2021 Phys. Rev. B 103 075421
Google Scholar
[35] Yalameha S, Nourbakhsh Z, Vaez A 2018 J. Magn. Magn. Mater. 468 279
Google Scholar
[36] Zhang Q, Cheng Y, Schwingenschlögl U 2013 Phys. Rev. B 88 155317
Google Scholar
[37] Yu R, Qi X, Bernevig A, Fang Z, Dai X 2011 Phys. Rev. B 84 075119
Google Scholar
[38] Grusdt F, Abanin D, Demler E 2014 Phys. Rev. A 89 043621
Google Scholar
[39] Qi X L, Zhang S C 2010 Phys. Today 63 33
Google Scholar
-
图 1 (a) Tl2Ta2O7的原胞; (b) 体结构第一布里渊区及(100)表面布里渊区示意图
Fig. 1. (a) Primitive unit cell of Tl2Ta2O7; (b) schematic of the bulk first Brillouin zone (BZ) and projected surface BZ for (100) surface
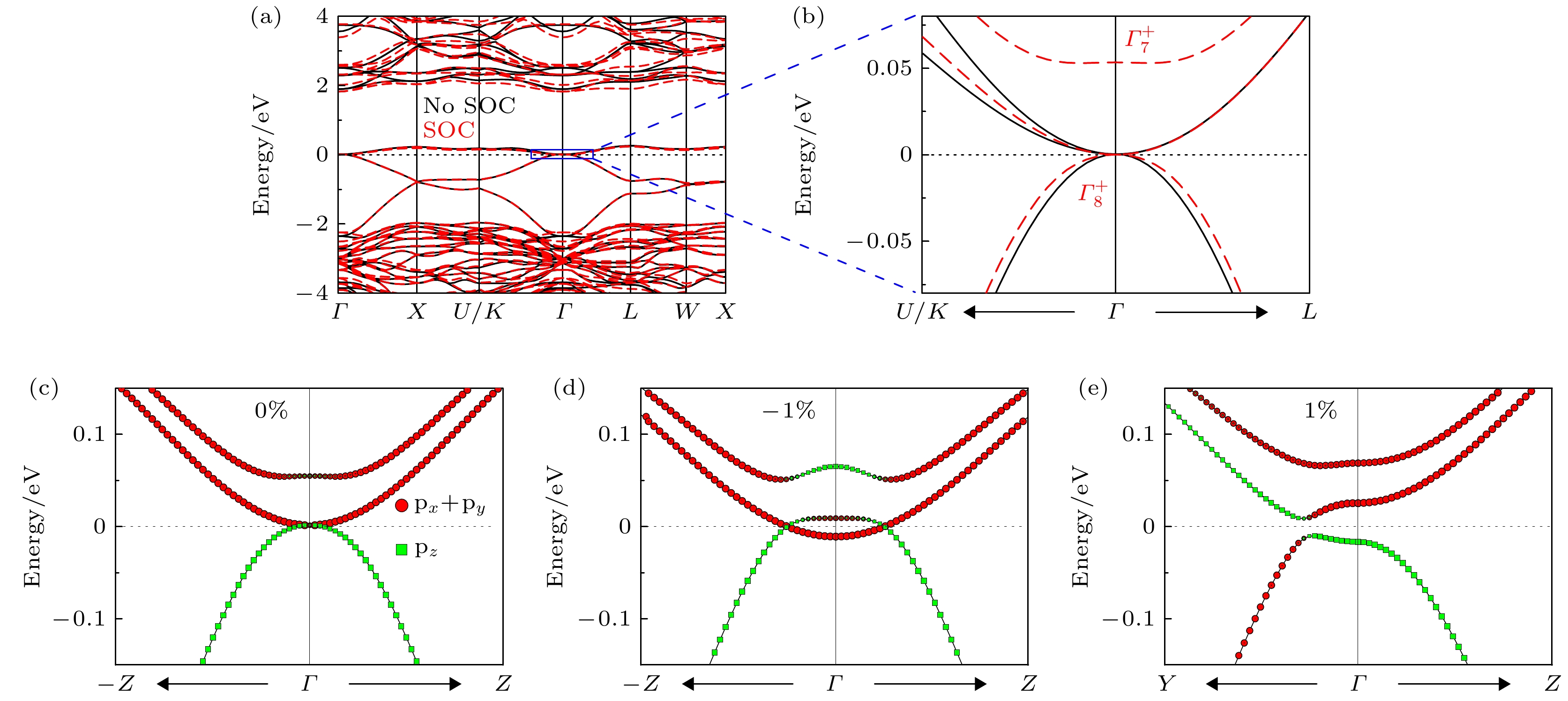
图 2 Tl2Ta2O7的能带结构 (a) 未施加应变时, 不考虑SOC(黑色实线)和考虑SOC(红色虚线)时的能带结构; (b) Γ点费米能级附近能带的放大图. SOC相互作用下体系的轨道分辨能带图 (c) 未施加应变; (d) 应变为–1% (平面内压缩); (e) 应变为1% (平面内拉伸)
Fig. 2. Band structures of Tl2Ta2O7 system: (a) Non-SOC (black solid lines) and SOC (red dashed lines) band structures without strain; (b) the zoom-in band structure around Γ point near the Fermi level. SOC orbital-resolved band structure: (c) Without strain; (d) with strain of –1% (in-plane compression); (e) with strain of 1% (in-plane expansion).
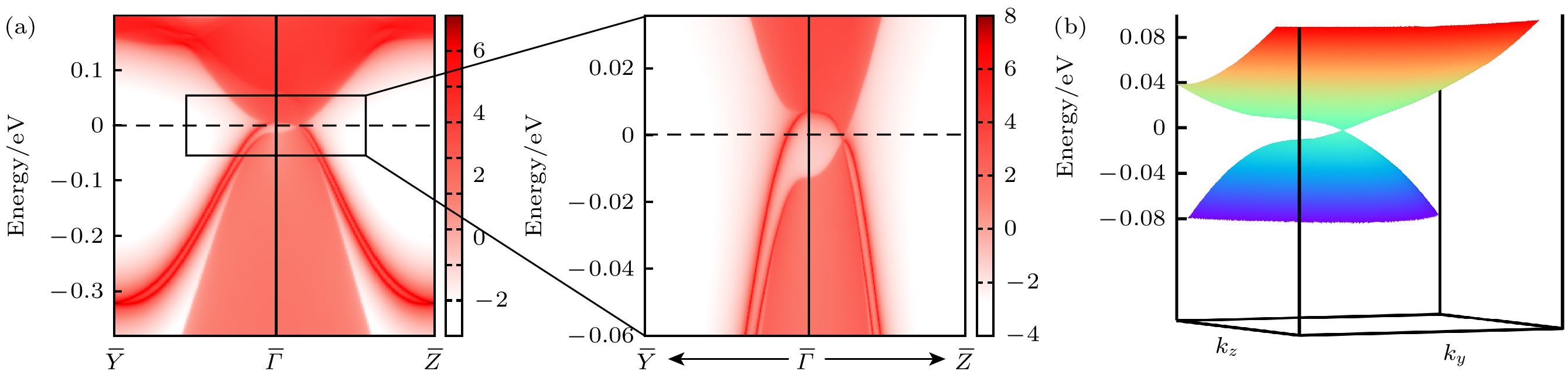
图 3 考虑SOC时, 施加–1% (平面内压缩)应变的Tl2Ta2O7 (100) 表面的拓扑性质 (a) 狄拉克点和拓扑表面态(右图为费米能级附近的放大图); (b) 狄拉克锥三维立体图
Fig. 3. Topological properties of Tl2Ta2O7 with SOC on (100) surface under –1% (in-plane compression) strain: (a) Dirac point and topological surface states (The right panel shows the enlarged image near the Fermi level); (b) three-dimensional display of the Dirac cone.
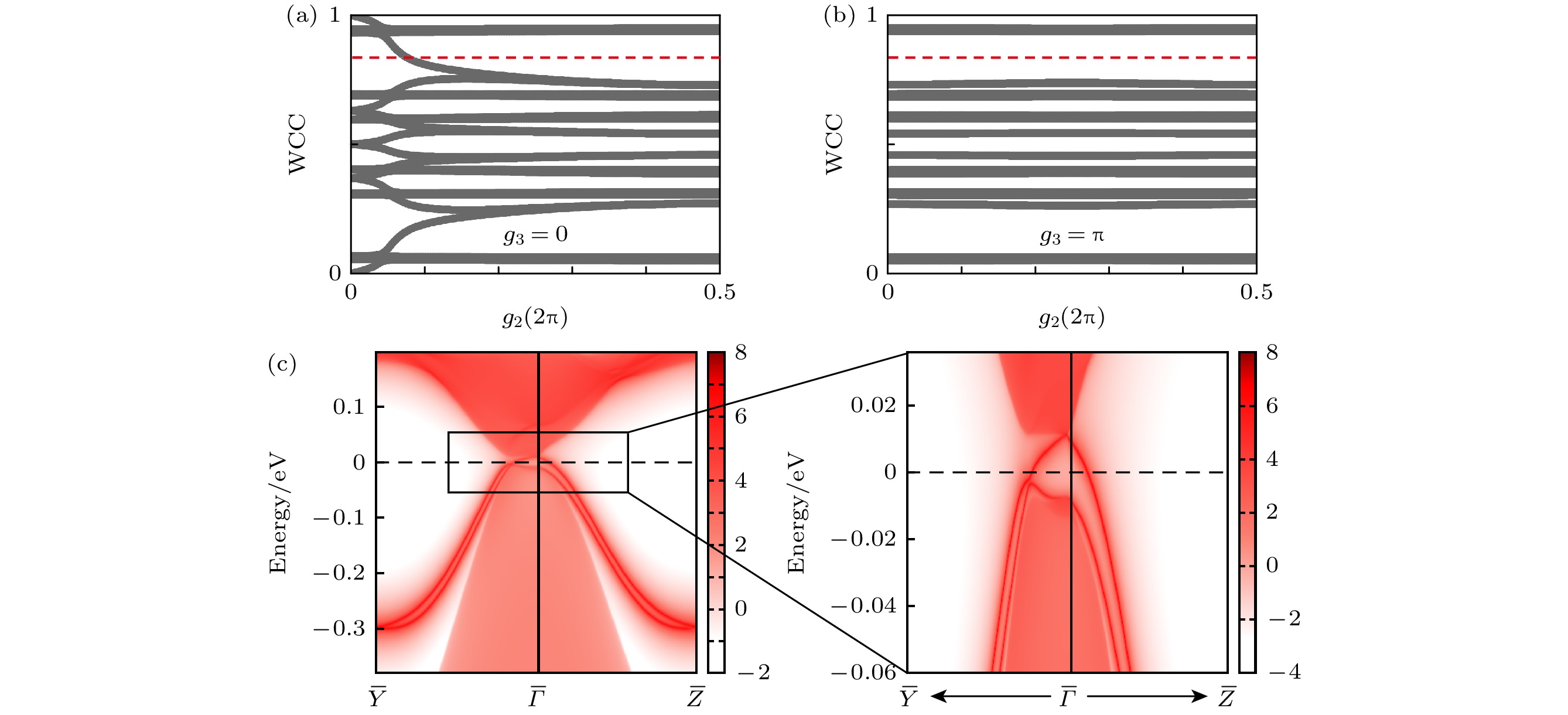
图 4 考虑SOC时, 施加1% (平面内拉伸)应变的Tl2Ta2O7 (100)表面的拓扑性质 (a) g3 = 0平面和(b) g3 = π平面Wannier电荷中心(WCC)的演化, g1, 2, 3是原胞的倒格子基矢. Wannier中心(黑线)与参考线(红线)在g3 = 0平面上相交1次, 而在g3 = π平面上相交0次. (c) 拓扑表面态及其费米能级附近的放大图
Fig. 4. Topological properties of Tl2Ta2O7 with SOC on (100) surface under 1% (in-plane expansion) strain. Evolution of the Wannier charge centers (WCC) in (a) g3 = 0 plane and (b) g3 = π plane, where g1, 2, 3 are reciprocal vectors of the primitive lattice. The WCC (black lines) cross the reference line (red line) once in the g3 = 0 plane and zero times in the g3 = π plane. (c) Topological surface states. The right panel shows the enlarged image near the Fermi level.
-
[1] Bernevig B A, Hughes T L, Zhang S C 2006 Science 314 1757
Google Scholar
[2] 王航天, 赵海慧, 温良恭, 吴晓君, 聂天晓, 赵巍胜 2020 69 200704
Google Scholar
Wang H T, Zhao H H, Wen L G, Wu X J, Nie T X, Zhao W S 2020 Acta Phys. Sin. 69 200704
Google Scholar
[3] Tian W, Yu W, Shi J, Wang Y 2017 Materials 10 814
Google Scholar
[4] Lü H, Wang Z, Cheng Q, Zhang W, Yu R 2021 Phys. Rev. B 103 L241115
Google Scholar
[5] Hu J, Xu S Y, Ni N, Mao Z 2019 Annu. Rev. Mater. Res. 49 207
Google Scholar
[6] Zhang W, Wu Q, Yazyev O V, Weng H, Guo Z, Cheng W D, Chai G L 2018 Phys. Rev. B 98 115411
Google Scholar
[7] Chen Z, Hu Y, Zhu Z, Zhang W 2020 New J. Phys. 22 093055
Google Scholar
[8] Yuan D, Hu Y, Yang Y, Zhang W 2021 Chin. Phys. Lett. 38 117301
Google Scholar
[9] Ferreira P P, Manesco A L R, Dorini T T, et al. 2021 Phys. Rev. B 103 125134
Google Scholar
[10] Cao W, Tang P, Xu Y, Wu J, Gu B L, Duan W 2017 Phys. Rev. B 96 115203
Google Scholar
[11] Jitta R R, Gundeboina R, Veldurthi N K, Guje R, Muga V 2015 J. Chem. Technol. Biot. 90 1937
Google Scholar
[12] Kim M, Park J, Kang M, Kim J Y, Lee S W 2020 ACS Cent. Sci. 6 880
Google Scholar
[13] Yang B J, Kim Y B 2010 Phys. Rev. B 82 085111
Google Scholar
[14] Wan X, Turner A M, Vishwanath A, Savrasov S Y 2011 Phys. Rev. B 83 205101
Google Scholar
[15] Sakata M, Kagayama T, Shimizu K, Matsuhira K, Takagi S, Wakeshima M, Hinatsu Y 2011 Phys. Rev. B 83 041102(R
Google Scholar
[16] Hase I, Yanagisawa T 2020 Symmetry 12 1076
Google Scholar
[17] Das M, Bhowal S, Sannigrahi J, et al. 2022 Phys. Rev. B 105 134421
Google Scholar
[18] Hu Y, Yue C, Yuan D, et al. 2022 Sci. China Phys. Mech. Astron. 65 297211
Google Scholar
[19] Zhang W, Luo K, Chen Z, Zhu Z, Yu R, Fang C, Weng H 2019 npj Comput. Mater. 5 105
Google Scholar
[20] Hase I, Yanagisawa T, Kawashima K 2019 Nanomaterials 9 876
Google Scholar
[21] Kresse G, Furthmüller J 1996 Comput. Mater. Sci. 6 15
Google Scholar
[22] Kresse G, Furthmüller J 1996 Phys. Rev. B 54 11169
Google Scholar
[23] Blöchl P E 1994 Phys. Rev. B 50 17953
Google Scholar
[24] Kresse G, Joubert D 1999 Phys. Rev. B 59 1758
Google Scholar
[25] Perdew J P, Burke K, Ernzerhof M 1996 Phys. Rev. Lett. 77 3865
Google Scholar
[26] Marzari N, Vanderbilt D 1997 Phys. Rev. B 56 12847
Google Scholar
[27] Souza I, Marzari N, Vanderbilt D 2001 Phys. Rev. B 65 035109
Google Scholar
[28] Wu Q, Zhang S, Song H F, Troyer M, Soluyanov A A 2018 Comput. Phys. Commun. 224 405
Google Scholar
[29] 杜永平, 刘慧美, 万贤纲 2015 64 187201
Google Scholar
Du Y P, Liu H M, Wan X G 2015 Acta Phys. Sin. 64 187201
Google Scholar
[30] Sheng X L, Wang Z, Yu R, Weng H, Fang Z, Dai X 2014 Phys. Rev. B 90 245308
Google Scholar
[31] Wang Z, Weng H, Wu Q, et al. 2013 Phys. Rev. B 88 125427
Google Scholar
[32] Cvetkovic V, Vafek O 2013 Phys. Rev. B 88 134510
Google Scholar
[33] Yakovkin I N, Petrova N V 2021 Phys. Lett. A 403 127398
Google Scholar
[34] Liu M Y, Gong L, He Y, Cao C 2021 Phys. Rev. B 103 075421
Google Scholar
[35] Yalameha S, Nourbakhsh Z, Vaez A 2018 J. Magn. Magn. Mater. 468 279
Google Scholar
[36] Zhang Q, Cheng Y, Schwingenschlögl U 2013 Phys. Rev. B 88 155317
Google Scholar
[37] Yu R, Qi X, Bernevig A, Fang Z, Dai X 2011 Phys. Rev. B 84 075119
Google Scholar
[38] Grusdt F, Abanin D, Demler E 2014 Phys. Rev. A 89 043621
Google Scholar
[39] Qi X L, Zhang S C 2010 Phys. Today 63 33
Google Scholar
下载:
计量
- 文章访问数: 3460
- PDF下载量: 87
- 被引次数: 0
