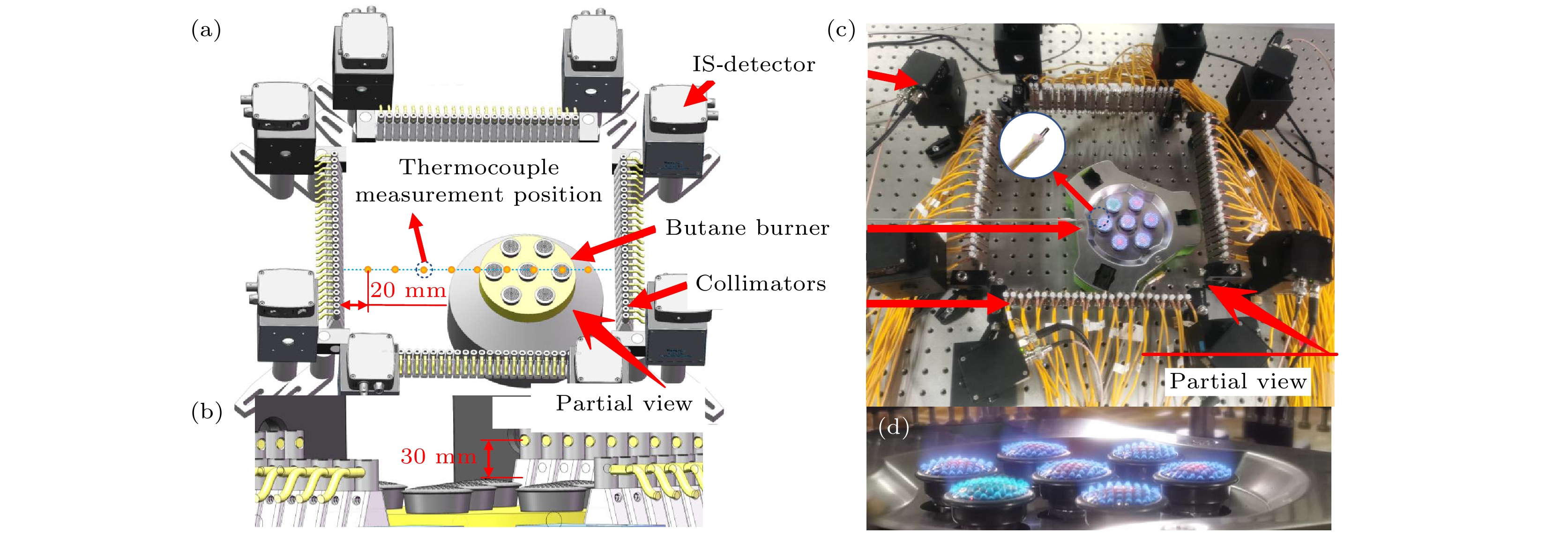
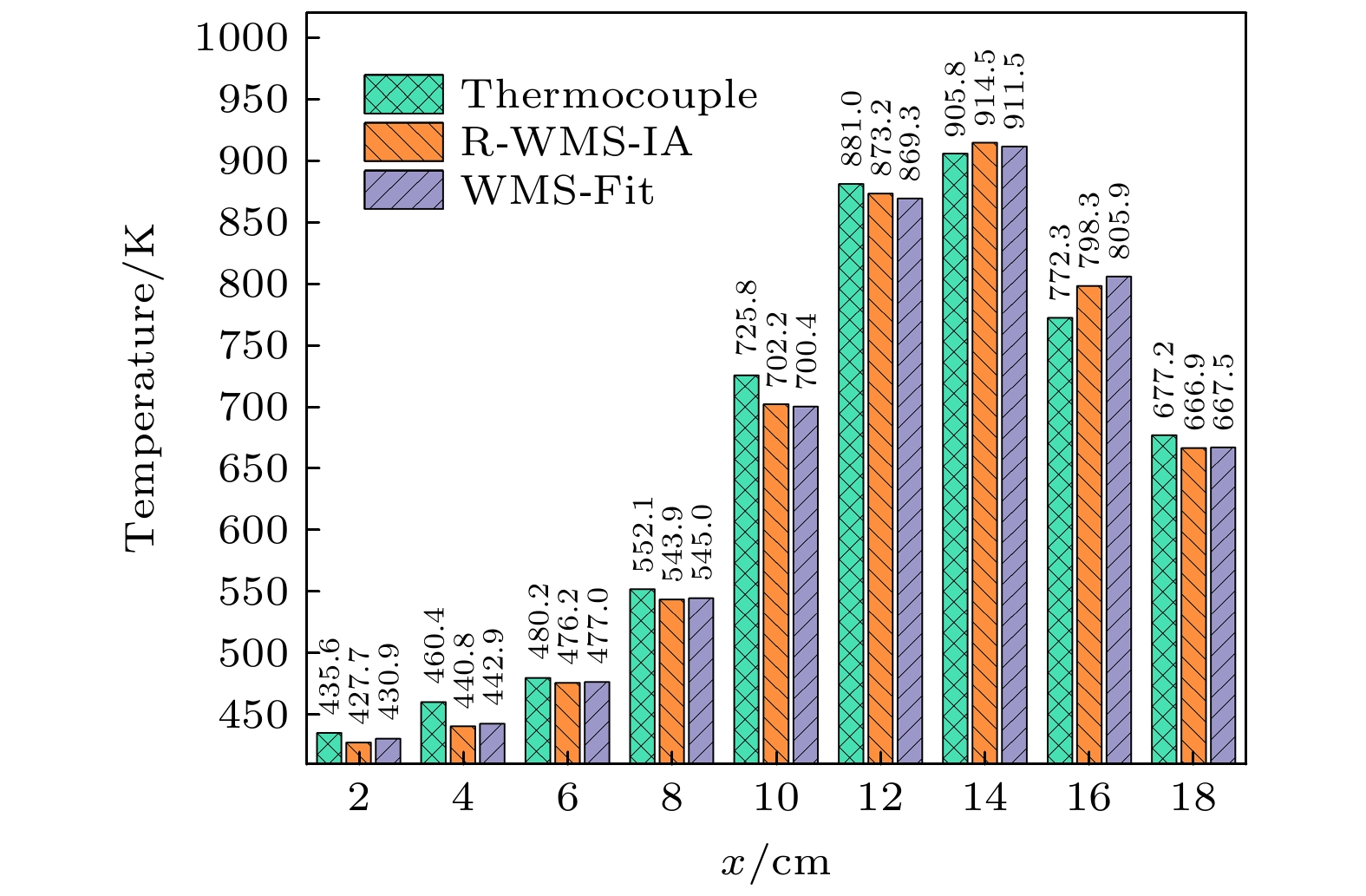
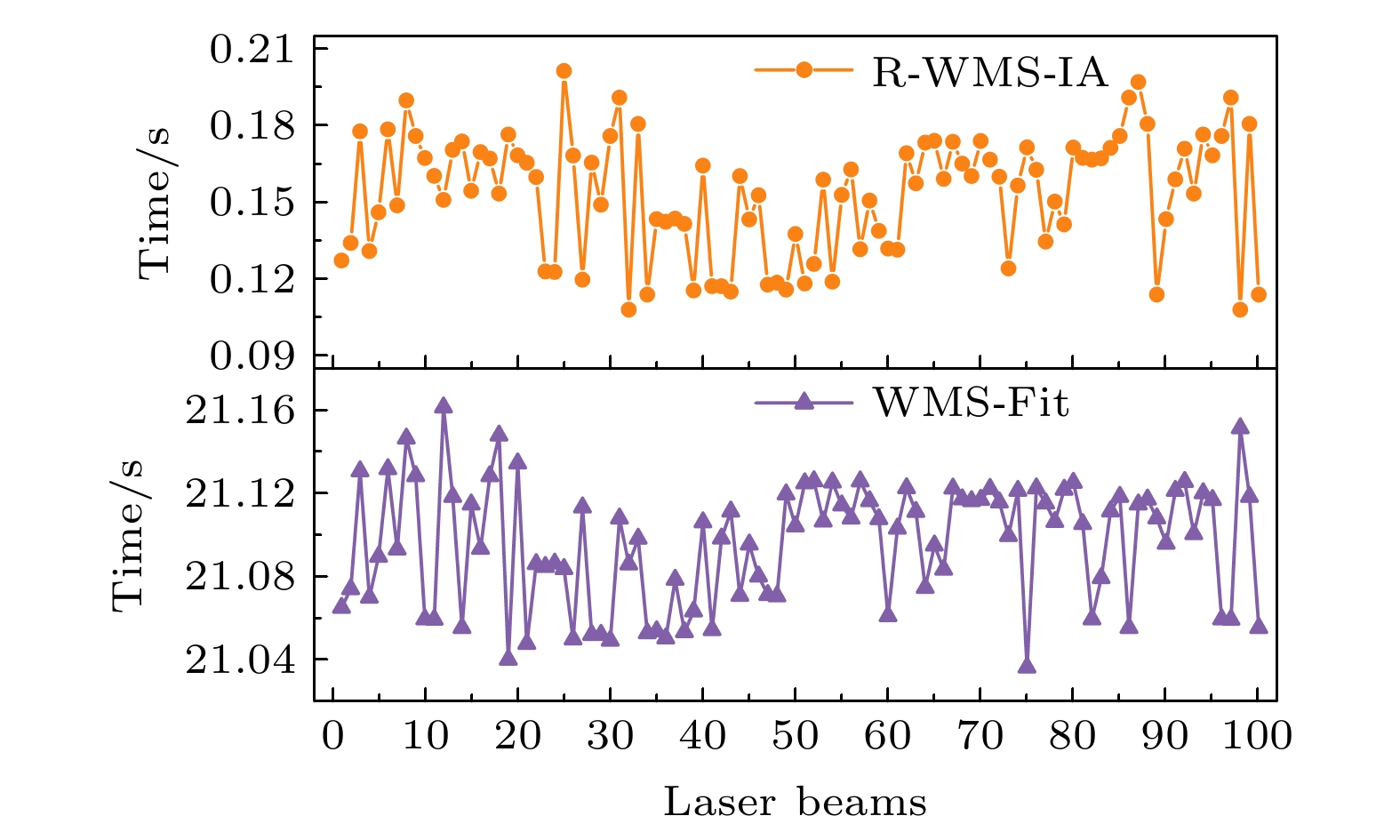
-
传统的免标定波长调制光谱方法一般需要结合光谱数据库和激光调制参数进行复杂的吸收光谱模拟, 对先验光谱参数的准确度和硬件参数提出了很高的要求, 同时不合适的初值会增加计算时间, 甚至会导致陷入局部最优解. 为提高计算效率, 本文引入一种快速免标定波长调制光谱技术获取积分吸光度. 该方法对光谱数据库的依赖性低, 计算效率高, 同时解决了传统方法在高温高压下由于吸收谱线展宽变大而导致的谐波信号不完整问题. 进一步将该方法应用于非均匀复杂燃烧场层析成像, 并结合所提成像系统实现了快速在线重建温度、浓度分布. 通过数值模拟和丁烷喷灯燃烧火焰的实验验证该方法获得积分吸光度的准确性和计算效率. 结果表明, 与传统的波长调制方法相比重建分布基本一致, 最大测量相对偏差仅为0.94%, 与热电偶测量值相比最大相对偏差为3.5%, 验证了该方法的准确性. 在重建精度相当的前提下, 分析两种方法获得积分吸光度的计算效率. 所引方法和传统方法平均每路计算时间分别为0.15 s和21.10 s. 所引方法的计算效率比传统方法至少提高了2个数量级, 为实现在线重建燃烧场的温度、浓度分布提供了快速可靠的研究方法和技术手段.Conventional calibration-free wavelength modulation spectroscopy generally requires complex absorption spectrum simulations in combination with spectral databases and laser modulation parameters, placing high demands on the accuracy of a priori spectral parameters and hardware parameters. Meanwhile, inappropriate initial values can increase the computation time and even lead to local optimal solutions. In order to improve the computational efficiency, a rapid calibration-free wavelength modulation spectroscopy to obtain the integrated absorbance is presented in this work. First, this method is computationally efficient, requiring only algebraic calculations by using the 2nd, 4th, and 6th harmonic center peak height parameters to obtain the integrated absorbance, eliminating the need for computationally intensive harmonic fitting calculations. Secondly, this method has low dependence on the spectral database, requiring only line intensity and low-state energy level spectral parameters. Finally, this method is highly adaptable and does not require scanning the complete absorption spectral line shape, which solves the problem of incomplete harmonic signals caused by the conventional method at high temperature and high pressure due to the broadening of the absorption spectral line. This method has previously been used only for line-of-sight measurements at low-frequency experimental signals in stable environments, and for calculating the integrated absorbance at average temperature, concentration and pressure states. In this work, the method is applied to non-uniform complex combustion field tomography and combined with the proposed tomographic system to achieve online reconstructing temperature and concentration distributions. The accuracy and computational efficiency of the method in obtaining the integrated absorbance are verified by numerical simulations and experiments on the butane burner flame. The results show that the presented method is consistent with the reconstructed distribution compared with the conventional wavelength modulation method, with a maximum relative deviation of only 0.94% from the measurement and 3.5% from the thermocouple measurement, verifying the accuracy of the method. The computational efficiencies of the two methods for obtaining the integrated absorbance are analyzed. The average calculation time per path is 0.15 s for the present method and 21.10 s for the conventional method. The calculation efficiency of the present method is at least two orders of magnitude higher than that of the conventional method, which provides a fast and reliable research method and technical means to realize the industrial-grade online reconstruction of temperature and concentration distribution of combustion fields.
-
Keywords:
- online tomography /
- laser absorption spectroscopy /
- wavelength modulation spectroscopy /
- complex combustion field
[1] 黄安, 许振宇, 夏晖晖, 姚路, 阮俊, 胡佳屹, 臧益鹏, 阚瑞峰 2021 光谱学与光谱分析 41 1144
Google Scholar
Hang A, Xu Z Y, Xia H H, Yao L, Ruan J, Hu J Y, Zang Y P, Kan R F 2021 Spectrosc. Spect. Anal. 41 1144
Google Scholar
[2] Wang Y, Zhou B, Liu C 2021 IEEE Photonics Technol. Lett. 33 1487
Google Scholar
[3] Liu C, Xu L, Li F, Cao Z, Tsekenis S A, McCann H 2015 Appl. Phys. B 120 407
Google Scholar
[4] 宋俊玲, 洪延姬, 王广宇, 潘虎 2012 61 124
Google Scholar
Song J L, Hong Y J, Wang G Y, Pan H 2012 Acta Phys. Sin. 61 124
Google Scholar
[5] Liu C, Xu L 2019 Appl. Spectrosc. Rev. 54 1
Google Scholar
[6] 臧益鹏, 许振宇, 黄安, 艾苏曼, 夏晖晖, 阚瑞峰 2021 70 134205
Google Scholar
Zang Y P, Xu Z Y, Hang A, Ai S M, Xia H H, Kan R F 2021 Acta Phys. Sin. 70 134205
Google Scholar
[7] Liu C, Cao Z, Li F, Lin Y, Xu L 2017 Meas. Sci. Technol. 28 054002
Google Scholar
[8] 屈东胜, 洪延姬, 朱晓辉 2021 光谱学与光谱分析 41 1072
Qu D S, Hong Y J, Zhu X H 2021 Spectrosc. Spect. Anal. 41 1072
[9] Rieker G B, Jeffries J B, Hanson R K 2009 Appl. Opt. 48 5546
Google Scholar
[10] Grauer S J, Emmert J, Sanders S T, Wagner S, Daun K J 2019 Meas. Sci. Technol. 30 105401
Google Scholar
[11] Zhang R, Si J, Enemali G, Bao Y, Liu C 2022 IEEE Sens. J. 22 12728
Google Scholar
[12] Shui C, Wang Y, Cai W, Zhou B 2021 Opt. Express 29 20889
Google Scholar
[13] Song J, Xin M, Rao W, Hong Y, Feng G 2021 Appl. Opt. 60 5056
Google Scholar
[14] Peng D, Jin Y, Zhai C, Yang J 2018 Spectrosc. Lett. 51 7
Google Scholar
[15] Rieker G B, Li H, Liu X, Jeffries J B, Hanson R K, Allen M G, Wehe S D, Mulhall P A, Kindle H S 2007 Meas. Sci. Technol. 18 1195
Google Scholar
[16] Sun K, Chao X, Sur R, Goldenstein C S, Jeffries J B, Hanson R K 2013 Meas. Sci. Technol. 24 125203
Google Scholar
[17] Wang Y, Zhou B, Liu C 2021 Opt. Express 29 26618
Google Scholar
[18] 张书锋, 蓝丽娟, 丁艳军, 贾军伟, 彭志敏 2015 64 053301
Google Scholar
Zhang S F, Lan L J, Ding Y J, Jia J W, Peng Z M 2015 Acta Phys. Sin. 64 053301
Google Scholar
[19] Wang Y, Zhou B, Wang B, Zhao R, Liu Q, Dai M 2022 Mathematics 10 308
Google Scholar
[20] Wang Y, Zhou B, Zhao R, Wang B, Liu Q, Dai M 2022 Mathematics 10 210
Google Scholar
[21] Liu Y, Lin J, Huang G, Guo Y, Duan C 2001 J. Opt. Soc. Am. B 18 666
Google Scholar
[22] Li N, Weng C 2011 Chin. Opt. Lett. 9 061201
Google Scholar
[23] Gordon I E, Rothman L S, Hill C, et al. 2017 J. Quant. Spectrosc. Radiat. Transf. 203 3
Google Scholar
[24] Terzija N, Davidson J L, Garcia-Stewart C A, Wright P, Ozanyan K B, Pegrum S, Litt T J, McCann H 2008 Meas. Sci. Technol. 19 094007
Google Scholar
[25] Grauer S J, Rice K M, Donbar J M, Bisek N J, France J J, Ochs B A, Steinberg A M 2022 AIAA J. 60 1
Google Scholar
[26] Ma L, Lau L Y, Ren W 2017 Appl. Phys. B 123 83
Google Scholar
[27] Huang A, Cao Z, Zhao W, Zhang H, Xu L 2020 IEEE Trans. Instrum. Meas. 69 9087
Google Scholar
-
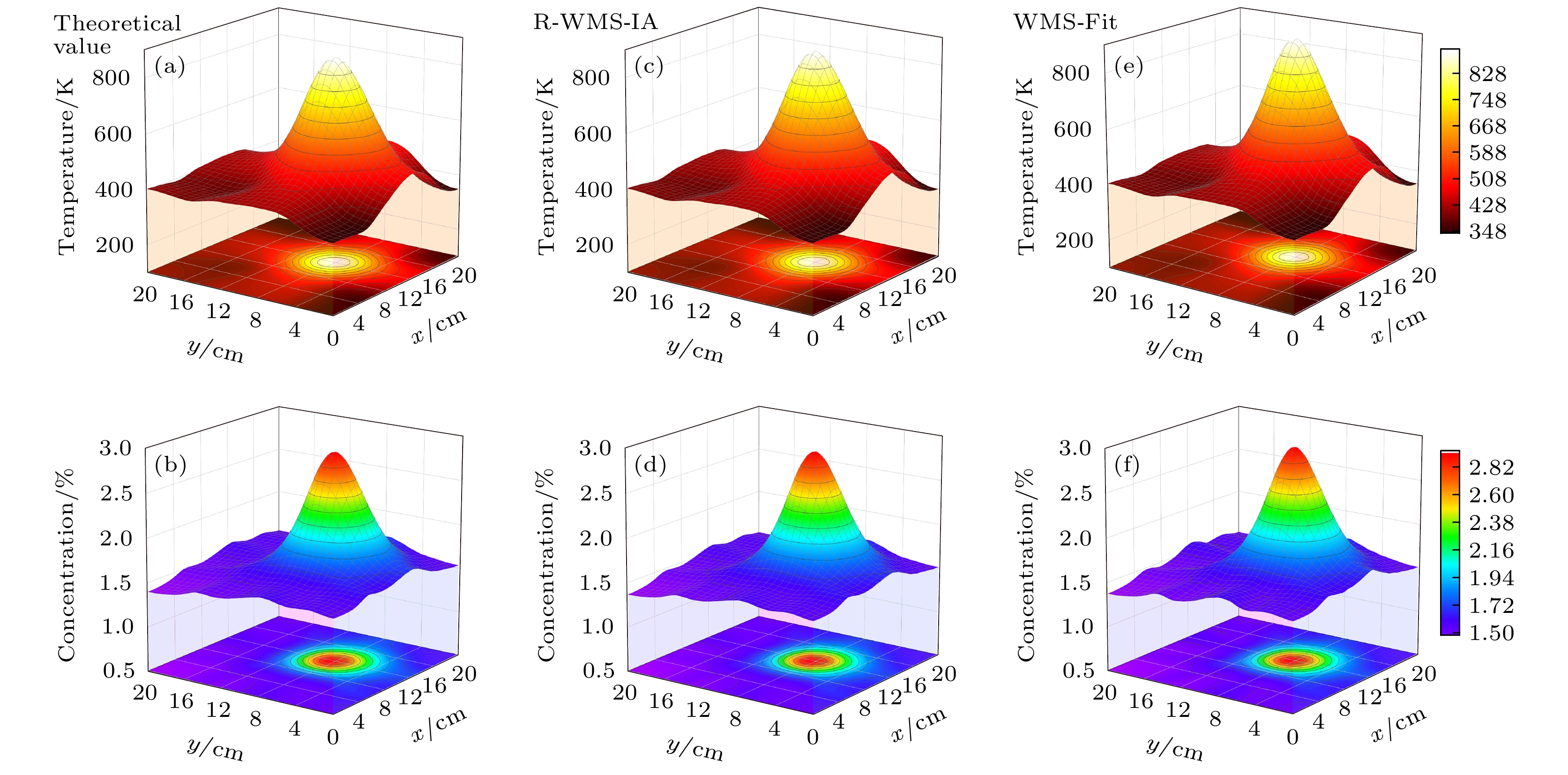
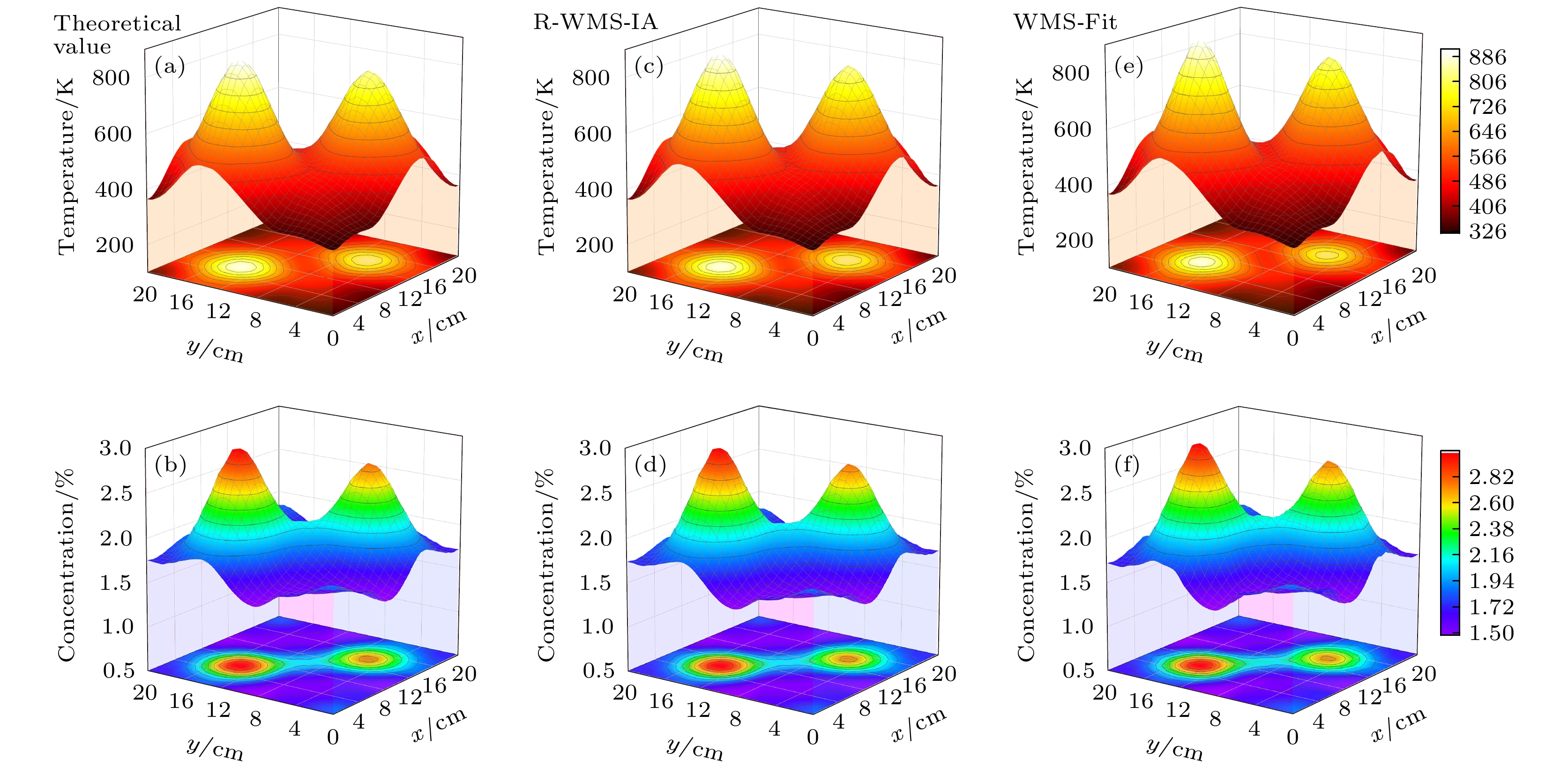
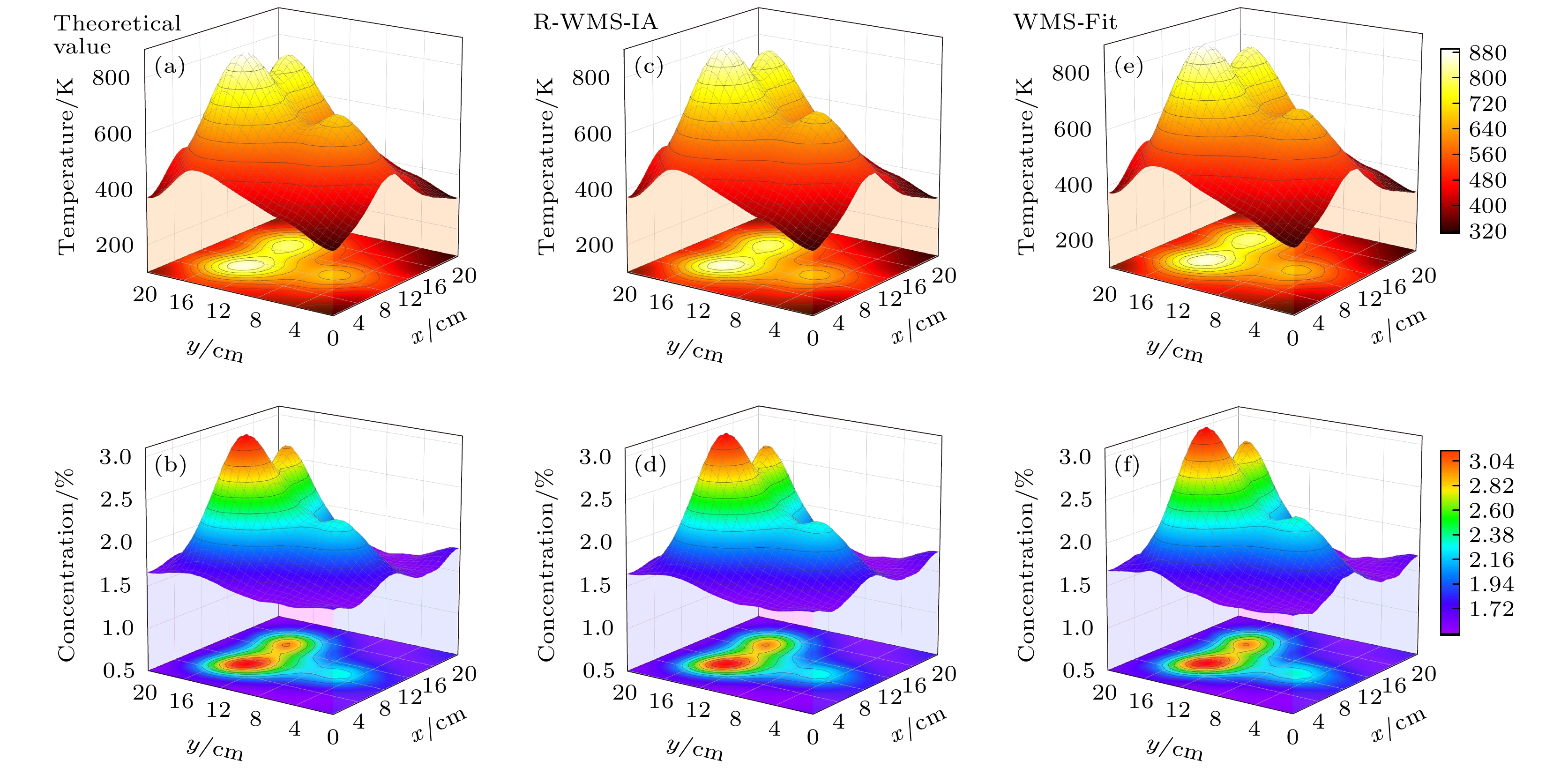
图 2 非均匀温度和H2O浓度分布区域 (a), (b) 分别表示模型1温度和浓度分布; (c), (d) 分别表示模型2温度和浓度分布; (e), (f) 分别表示模型3温度和浓度分布
Fig. 2. Non-uniform temperature and H2O concentration distribution regions: (a), (b) Indicate the temperature, concentration distribution of model 1; (c), (d) indicate the temperature, concentration distribution of model 2; (e), (f) indicate the temperature, concentration distribution of model 3, respectively.
图 3 (a)测量系统中光路布置的位置关系; (b)测量区域中光路布置
Fig. 3. (a) Position relationship of the optical path arrangement in the measurement system; (b) optical paths arrangement in the measurement area.
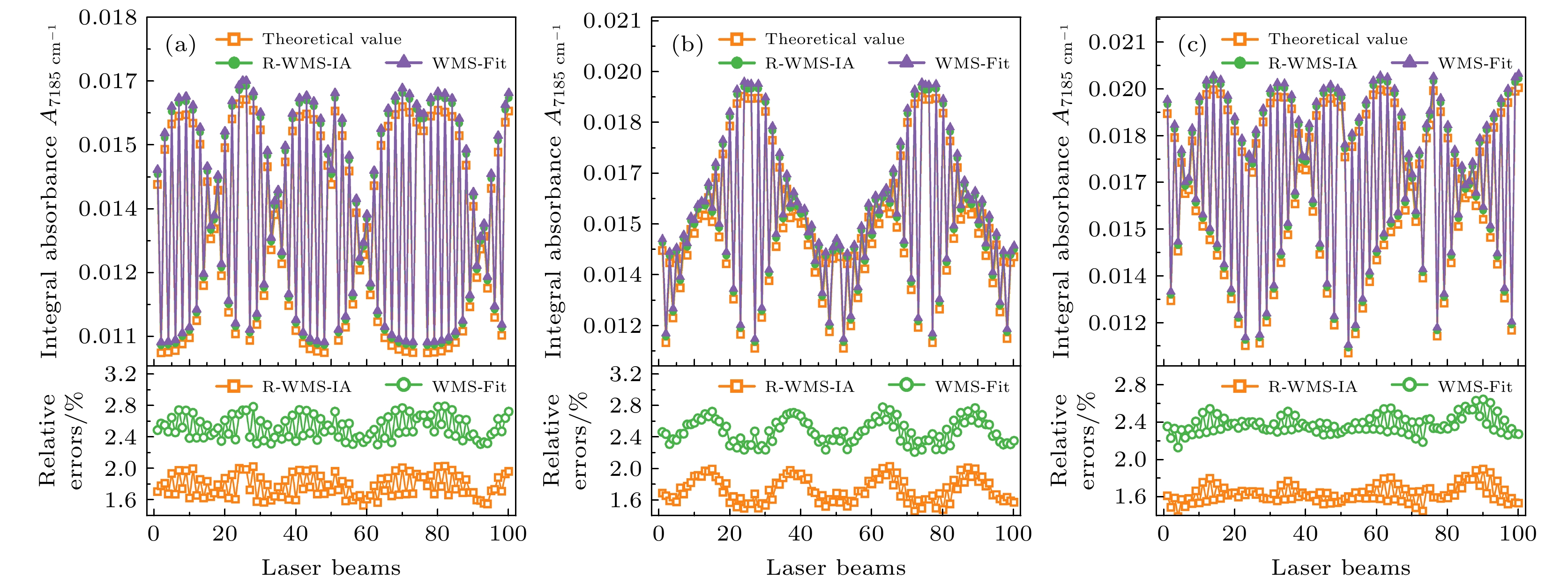
图 4 三种不同分布模型通过R-WMS-IA和WMS-Fit算法获得积分吸光度的计算结果及相对误差 (a) 分布模型1; (b) 分布模型2; (c) 分布模型3
Fig. 4. Calculation results and relative error of integrated absorbance by R-WMS-IA and WMS-Fit algorithms for three different distribution models: (a) Distribution model 1; (b) distribution model 2; (c) distribution model 3.
图 5 分布模型1中不同方法的重建结果 (a) e = 5.34%; (b) e = 5.38%; (c) e = 5.53%; (d) e = 5.42%; (e) e = 5.58%; (f) e = 5.00%
Fig. 5. Reconstruction results of different methods in distribution model 1: (a) e = 5.34%; (b) e = 5.38%; (c) e = 5.53%; (d) e = 5.42%; (e) e = 5.58%; (f) e = 5.00%.
图 6 分布模型2中不同方法的重建结果 (a) e = 5.74%; (b) e = 6.20%; (c) e = 5.91%; (d) e = 5.91%; (e) e = 6.33%; (f) e = 6.36%
Fig. 6. Reconstruction results of different methods in distribution model 2: (a) e = 5.74%; (b) e = 6.20%; (c) e = 5.91%; (d) e = 5.91%; (e) e = 6.33%; (f) e = 6.36%.
图 7 分布模型3中不同方法的重建结果 (a) e = 4.46%; (b) e = 4.70%; (c) e = 4.61%; (d) e = 4.74%; (e) e = 4.87%; (f) e = 4.40%
Fig. 7. Reconstruction results of different methods in distribution model 3: (a) e = 4.46%; (b) e = 4.70%; (c) e = 4.61%; (d) e = 4.74%; (e) e = 4.87%; (f) e = 4.40%.
图 8 仿真分析通过两种方法计算积分吸光度时间
Fig. 8. Simulation analysis calculates the integral absorbance time by two methods.
图 10 丁烷喷灯燃烧器的实验平台 (a)实验平台3D建模; (b)建模局部视图; (c)实验照片; (d)局部火焰视图
Fig. 10. Experimental platform of butane burner: (a) 3D modeling structure of experimental platform; (b) partial view of modeling; (c) experimental photo; (d) partial view of flame.
图 11 第50路透射光强的电压信号和以7185.60 cm–1(绿色)、7444.36 cm–1(紫色)光谱吸收为中心的2、4、6次谐波信号
Fig. 11. Voltage signal of the 50th transmitted light intensity and 2nd, 4th and 6th harmonic signals centered on 7185.60 cm–1 (green) and 7444.36 cm–1 (violet) spectral absorption.
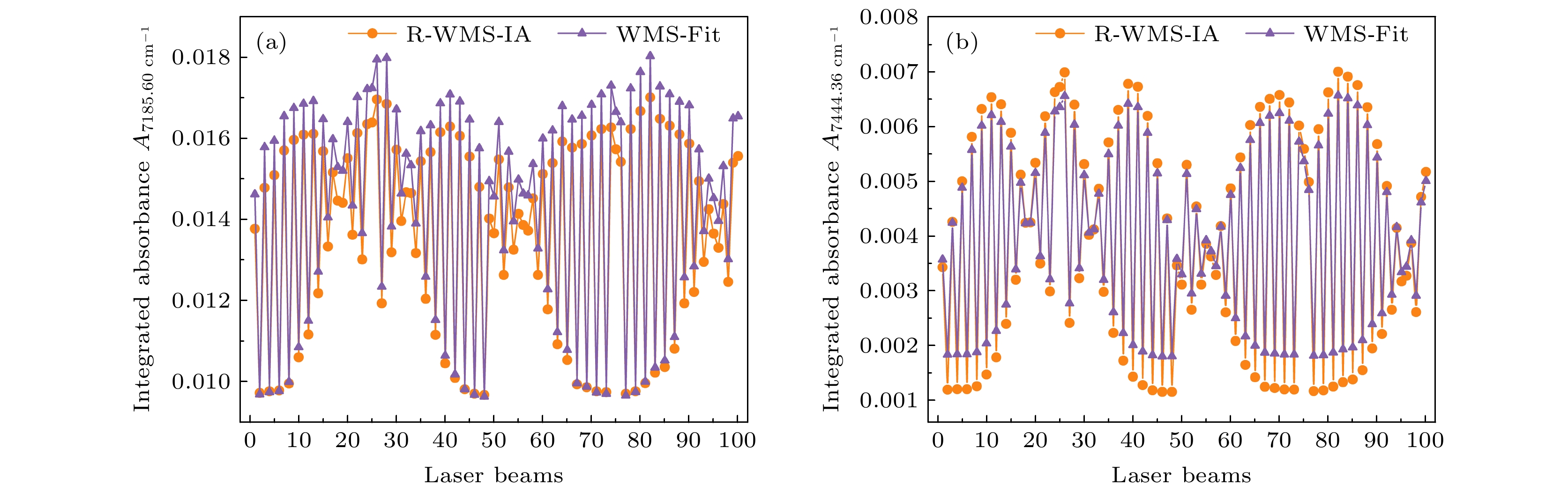
图 12 通过R-WMS-IA和WMS-Fit算法获得积分吸光度的计算结果 (a) 谱线中心为7185.60 cm–1积分吸光度; (b) 谱线中心为7444.36 cm–1积分吸光度
Fig. 12. Results of the integrated absorbance calculations were obtained by R-WMS-IA and WMS-Fit algorithms: (a) Spectral line centered at 7185.60 cm–1 integrated absorbance; (b) spectral line centered at 7444.36 cm–1 integrated absorbance.
图 13 分别通过两种算法获得积分吸光度的温度场重建结果 (a) R-WMS-IA方法; (b) WMS-Fit算法
Fig. 13. The temperature field reconstruction results of the integrated absorbance were obtained by two algorithms, respectively: (a) R-WMS-IA algorithm; (b) WMS-Fit algorithm.
图 14 热电偶与两种算法的温度测量值
Fig. 14. Temperature curves measured by the thermocouple and the two algorithms respectively.
图 15 实验分析通过两种方法计算积分吸光度时间
Fig. 15. Experiment analysis calculates the integral absorbance time by two methods.
表 1 7185.60 cm–1和7444.36 cm–1中心谱线处的参数
Table 1. Parameters of the selected transitions at around 7185.60 cm–1 and 7444.36 cm–1.
Line index wavenumber/cm–1 S(T0)/(cm–2·atm–1) ξself/(cm–1·atm–1) ξair/(cm–1·atm–1) E''/cm–1 nair 1 7185.596 0.00490 0.371 0.0342 1045.0583 0.63 7185.597 0.0147 0.195 0.0413 1045.058 0.65 2 7444.351 0.000541 0.366 0.0199 1774.750 0.44 7444.368 0.000154 0.250 0.0188 1806.670 0.41 7444.371 0.000462 0.194 0.0153 1806.669 0.41 
下载: 导出CSV
表 2 三种不同分布模型的详细参数
Table 2. Detailed parameters of the three different distribution models.
分布模型 η ($ x_{\text{c}}^k $, $ y_{\text{c}}^k $)/cm σ/cm 1 0.40 (12, 8) 4 2 0.40
0.35(6, 14)
(14, 6)4 3 0.40
0.35
0.25(6, 14)
(14, 14)
(9, 6)4
下载: 导出CSV
-
[1] 黄安, 许振宇, 夏晖晖, 姚路, 阮俊, 胡佳屹, 臧益鹏, 阚瑞峰 2021 光谱学与光谱分析 41 1144
Google Scholar
Hang A, Xu Z Y, Xia H H, Yao L, Ruan J, Hu J Y, Zang Y P, Kan R F 2021 Spectrosc. Spect. Anal. 41 1144
Google Scholar
[2] Wang Y, Zhou B, Liu C 2021 IEEE Photonics Technol. Lett. 33 1487
Google Scholar
[3] Liu C, Xu L, Li F, Cao Z, Tsekenis S A, McCann H 2015 Appl. Phys. B 120 407
Google Scholar
[4] 宋俊玲, 洪延姬, 王广宇, 潘虎 2012 61 124
Google Scholar
Song J L, Hong Y J, Wang G Y, Pan H 2012 Acta Phys. Sin. 61 124
Google Scholar
[5] Liu C, Xu L 2019 Appl. Spectrosc. Rev. 54 1
Google Scholar
[6] 臧益鹏, 许振宇, 黄安, 艾苏曼, 夏晖晖, 阚瑞峰 2021 70 134205
Google Scholar
Zang Y P, Xu Z Y, Hang A, Ai S M, Xia H H, Kan R F 2021 Acta Phys. Sin. 70 134205
Google Scholar
[7] Liu C, Cao Z, Li F, Lin Y, Xu L 2017 Meas. Sci. Technol. 28 054002
Google Scholar
[8] 屈东胜, 洪延姬, 朱晓辉 2021 光谱学与光谱分析 41 1072
Qu D S, Hong Y J, Zhu X H 2021 Spectrosc. Spect. Anal. 41 1072
[9] Rieker G B, Jeffries J B, Hanson R K 2009 Appl. Opt. 48 5546
Google Scholar
[10] Grauer S J, Emmert J, Sanders S T, Wagner S, Daun K J 2019 Meas. Sci. Technol. 30 105401
Google Scholar
[11] Zhang R, Si J, Enemali G, Bao Y, Liu C 2022 IEEE Sens. J. 22 12728
Google Scholar
[12] Shui C, Wang Y, Cai W, Zhou B 2021 Opt. Express 29 20889
Google Scholar
[13] Song J, Xin M, Rao W, Hong Y, Feng G 2021 Appl. Opt. 60 5056
Google Scholar
[14] Peng D, Jin Y, Zhai C, Yang J 2018 Spectrosc. Lett. 51 7
Google Scholar
[15] Rieker G B, Li H, Liu X, Jeffries J B, Hanson R K, Allen M G, Wehe S D, Mulhall P A, Kindle H S 2007 Meas. Sci. Technol. 18 1195
Google Scholar
[16] Sun K, Chao X, Sur R, Goldenstein C S, Jeffries J B, Hanson R K 2013 Meas. Sci. Technol. 24 125203
Google Scholar
[17] Wang Y, Zhou B, Liu C 2021 Opt. Express 29 26618
Google Scholar
[18] 张书锋, 蓝丽娟, 丁艳军, 贾军伟, 彭志敏 2015 64 053301
Google Scholar
Zhang S F, Lan L J, Ding Y J, Jia J W, Peng Z M 2015 Acta Phys. Sin. 64 053301
Google Scholar
[19] Wang Y, Zhou B, Wang B, Zhao R, Liu Q, Dai M 2022 Mathematics 10 308
Google Scholar
[20] Wang Y, Zhou B, Zhao R, Wang B, Liu Q, Dai M 2022 Mathematics 10 210
Google Scholar
[21] Liu Y, Lin J, Huang G, Guo Y, Duan C 2001 J. Opt. Soc. Am. B 18 666
Google Scholar
[22] Li N, Weng C 2011 Chin. Opt. Lett. 9 061201
Google Scholar
[23] Gordon I E, Rothman L S, Hill C, et al. 2017 J. Quant. Spectrosc. Radiat. Transf. 203 3
Google Scholar
[24] Terzija N, Davidson J L, Garcia-Stewart C A, Wright P, Ozanyan K B, Pegrum S, Litt T J, McCann H 2008 Meas. Sci. Technol. 19 094007
Google Scholar
[25] Grauer S J, Rice K M, Donbar J M, Bisek N J, France J J, Ochs B A, Steinberg A M 2022 AIAA J. 60 1
Google Scholar
[26] Ma L, Lau L Y, Ren W 2017 Appl. Phys. B 123 83
Google Scholar
[27] Huang A, Cao Z, Zhao W, Zhang H, Xu L 2020 IEEE Trans. Instrum. Meas. 69 9087
Google Scholar
下载:
计量
- 文章访问数: 4147
- PDF下载量: 98
- 被引次数: 0
