准二维空间中的隐秘涡旋量子液滴

陈逸熙; 蔡晓妍; 刘彬; 江迅达; 黎永耀

doi:10.7498/aps.71.20220709

摘要

本文探究了弱囚禁条件下玻色-玻色混合凝聚体中的准二维隐秘涡旋量子液滴及其动力学特性. 前期研究表明, 在完全自由的三维空间中, 隐秘涡旋量子液滴难以稳定; 在二维系统中, 当囚禁尺度是窄囚禁条件时, 系统仅支持拓扑荷${S_{1,2}} = \pm 1$的隐秘涡旋量子液滴; 因此当横向囚禁尺度较弱时, 准二维空间中李-黄-杨修正项仍然采用三维空间中的表达式来描述, 此时隐秘涡旋量子液滴能否保持稳定是一个重要的科学问题. 本文采用虚时间方法获得了拓扑荷${S_{1,2}}$达到$ \pm 4$的隐秘涡旋量子液滴; 进一步论证了隐秘涡旋量子液滴的有效面积${A_{{\text{eff}}}}$和化学势$\mu $与总粒子数$N$之间的依赖关系; 并采用线性稳定性分析结合实时传输方法获得了总粒子数临界值${N_{{\text{th}}}}$分别与拓扑荷${S_1}$和非线性系数${\text{δ}}g$之间的依赖关系. 在动力学部分, 本文研究了由两个不同拓扑荷的隐秘涡旋量子液滴构造的复合涡旋模式, 即嵌套涡旋量子液滴. 结合量子液滴的密度分布具有“平顶型”的特点, 采用Thomas-Fermi近似对数值结果进行了有效验证.

关键词:

Abstract

In this work, we study the quasi-two-dimensional hidden vortices of quantum droplets (QDs) trapped by a thicker transverse confinement and investigate their dynamical properties. Previous studies demonstrated that the hidden vortices of QDs in a three-dimensional free space are unstable and stable two-dimensional hidden vortices of QDs only with ${S_{1,2}} = \pm 1$ can be supported by a thin transverse confinement. Under the conditions of thicker transverse confinement, the Lee-Huang-Yang correction term in quasi-two-dimensional space is still described in the form of the three-dimensional space. Hence, under this condition, the stability and characteristics of the hidden vortices of QDs are worth studying. By using the imaginary time method, the hidden vortices of QDs with topological charge ${S_{1,2}}$ up to $ \pm 4$ are obtained for the first time. Furthermore, the dependence of the effective area${A_{{\text{eff}}}}$and the chemical potential$\mu $on the total norm$N$of the hidden vortices of QDs are demonstrated. Besides, by using the linear stability analysis combined with the direct simulations, we obtain the dependence of the threshold norm${N_{{\text{th}}}}$ on the topological charge ${S_1}$ and the nonlinear coefficient ${\text{δ}}g$. Finally, we study the composite vortex pattern constructed by two hidden vortices of QDs, namely nested vortex QDs. Based on the fact that the hidden vortices of QDs generally have flat-top density profiles, the Thomas-Fermi approximation can be used to verify the numerical results effectively. The results of this paper can be extended in some directions, and provide a theoretical basis for the experimental realization of the hidden vortices of QDs.

Keywords:

作者及机构信息

1.
佛山科学技术学院物理与光电工程学院, 佛山　528225

2.
佛山科学技术学院, 物理与光电工程学院粤港澳智能微纳光电技术联合实验室, 佛山　528225

通信作者: 刘彬, binliu@fosu.edu.cn

基金项目: 国家自然科学基金(批准号: 11905032, 11874112)、广东省自然科学基金面上项目(批准号: 2021A1515010214)、广东省普通高校重点科研项目(批准号: 2019KZDXM001)、广东省基础与应用基础研究基金(批准号: 2021A1515111015)和粤港澳智能微纳光电技术联合实验室(批准号: 2020B1212030010)资助的课题.

Authors and contacts

1.
School of Physics and Optoelectronic Engineering, Foshan University, Foshan 528225, China

2.
Guangdong-Hong Kong-Macao Joint Laboratory for Intelligent Micro-Nano Optoelectronic Technology, School of Physics and Optoelectronic Engineering, Foshan University, Foshan 528225, China

Corresponding author: Liu Bin, binliu@fosu.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 11905032, 11874112), the Natural Science Foundation of Guangdong Province, China (Grant No. 2021A1515010214), the Key Research Projects of General Colleges in Guangdong Province, China (Grant No. 2019KZDXM001), the Guang Dong Basic and Applied Basic Research Foundation, China (Grant No. 2021A1515111015), and Research Fund of Guangdong-Hong Kong-Macao Joint Laboratory for Intelligent Micro-Nano Optoelectronic Technology, China (Grant No. 2020B1212030010).

文章全文 : translate this paragraph

参考文献

[1]	Xu Y, Zhang Y P, Wu B 2013 Phys. Rev. A 87 013614 Google Scholar
[2]	Han W, Zhang X F, Wang D S, Jiang H F, Zhang W, Zhang S G 2018 Phys. Rev. Lett. 121 030404 Google Scholar
[3]	Zhang Y C, Zhou Z W, Malomed B A, Pu H 2015 Phys. Rev. Lett. 115 253902 Google Scholar
[4]	何章明, 张志强, 朱善华, 柳闻鹃 2014 63 190502 Google Scholar He Z M, Zhang Z Q, Zhu S H, Liu W J 2014 Acta Phys. Sin. 63 190502 Google Scholar
[5]	郭慧, 王雅君, 王林雪, 张晓斐 2020 69 010302 Google Scholar Guo H, Wang Y J, Wang L X, Zhang X F 2020 Acta Phys. Sin. 69 010302 Google Scholar
[6]	文林, 梁毅, 周晶, 余鹏, 夏雷, 牛连斌, 张晓斐 2019 68 080301 Google Scholar Wen L, Liang Y, Zhou J, Yu P, Xia L, Niu L B, Zhang X F 2019 Acta Phys. Sin. 68 080301 Google Scholar
[7]	Zhang R F, Zhang X F, Li L 2019 Phys. Lett. A 383 231 Google Scholar
[8]	Zhang R F, Zhang Y P, Li L 2019 Phys. Lett. A 383 3175 Google Scholar
[9]	贾瑞煜, 方乒乒, 高超, 林机 2021 70 180303 Google Scholar Jia R Y, Fang P P, Gao C, Lin J 2021 Acta Phys. Sin. 70 180303 Google Scholar
[10]	陈海军, 李向富 2013 62 070302 Google Scholar Chen H J, Li X F 2013 Acta Phys. Sin. 62 070302 Google Scholar
[11]	Li Y E, Xue J K 2016 Chin. Phys. Lett. 33 100502 Google Scholar
[12]	周嘉仪, 李画眉 2014 浙江师范大学学报 38 303 Zhou J Y, Li H M 2014 J. Zhejiang Normal Univ. 38 303
[13]	唐娜, 杨雪滢, 宋琳, 张娟, 李晓霖, 周志坤, 石玉仁 2020 69 010301 Google Scholar Tang N, Yang X Y, Song L, Zhang J, Li X L, Zhou Z K, Shi Y R 2020 Acta Phys. Sin. 69 010301 Google Scholar
[14]	Wen L, Sun Q, Wang H Q, Ji A C, Liu W M 2012 Phys. Rev. A 86 043602 Google Scholar
[15]	Wang L X, Dai C Q, Wen L, Liu T, Jiang H F, Saito H, Zhang Sh G, Zhang X F 2018 Phys. Rev. A 97 063607 Google Scholar
[16]	Peng P, Li G Q, Zhao L C, Yang W L, Yang Z Y 2019 Phys. Lett. A 383 2883 Google Scholar
[17]	Segev M, Valley G C, Crosignani B, DiPorto P, Yariv A 1994 Phys. Rev. Lett. 73 3211 Google Scholar
[18]	Huang J S, Jiang X D, Chen H Y, Fan Z W, Pang W, Li Y Y 2015 Front. Phys. 10 100507
[19]	Maucher F, Henkel N, Saffman M, Królikowski W, Skupin S, Pohl T 2011 Phys. Rev. Lett. 106 170401 Google Scholar
[20]	Wang Y Y, Chen L, Dai C Q, Zheng J, Fan Y 2017 Nonlinear Dyn. 90 1269 Google Scholar
[21]	Dai C Q, Chen R P, Wang Y Y, Fan Y 2017 Nonlinear Dyn. 87 1675 Google Scholar
[22]	Chen Y X, Zheng L H, Xu F Q 2018 Nonlinear Dyn. 93 2379 Google Scholar
[23]	Li J T, Zhu Y, Han J Z, Qin W, Dai C Q, Wang S H 2018 Nonlinear Dyn. 91 757 Google Scholar
[24]	Chen X W, Deng Z G, Xu X X, Li S L, Fan Z W, Chen Z P, Liu B, Li Y Y 2020 Nonlinear Dyn. 101 569 Google Scholar
[25]	Ye Z J, Chen Y X, Zheng Y Y, Chen X W, Liu B 2020 Chaos Solitons Fractals 130 109418 Google Scholar
[26]	Liu B, Zhong R X, Chen Z P, Qin X Z, Zhong H H, Li Y Y, Malomed B A 2020 New J. Phys. 22 043004 Google Scholar
[27]	Li Y Y, Luo Z H, Liu Y, Chen Z P, Huang C Q, Fu S H, Tan H S, Malomed B A 2017 New J. Phys. 19 113043 Google Scholar
[28]	Hu X H, Zhang X F, Zhao D, Luo H G, Liu W M 2009 Phys. Rev. A 79 023619 Google Scholar
[29]	李吉, 刘斌, 白晶, 王寰宇, 何天琛 2020 69 140301 Google Scholar Li J, Liu B, Bai J, Wang H Y, He T C 2020 Acta Phys. Sin. 69 140301 Google Scholar
[30]	陈海军, 任元, 王华 2022 71 056701 Google Scholar Chen H J, Ren Y, Wang H 2022 Acta Phys. Sin. 71 056701 Google Scholar
[31]	Schmitt M, Wenzel M, Böttcher F, Ferrier-Barbut I, Pfau T 2016 Nature 539 259 Google Scholar
[32]	Cabrera C R, Tanzi L, Sanz J, Naylor B, Thomas P, Cheiney P, Tarruell L 2018 Science 359 301 Google Scholar
[33]	Lee T D, Huang K, Yang C N 1957 Phys. Rev. 106 1135 Google Scholar
[34]	Tylutki M, Astrakharchik G E, Malomed B A, Petrov D S 2020 Phys. Rev. A 101 051601 Google Scholar
[35]	Boudjemâa A 2018 Phys. Rev. A 98 033612 Google Scholar
[36]	Hu H, Liu X J 2020 Phys. Rev. A 102 043302 Google Scholar
[37]	Pylak M, Gampel F, Płodzień M, Gajda M 2022 Phys. Rev. Res. 4 013168 Google Scholar
[38]	Parisi L, Giorgini S 2020 Phys. Rev. A 102 023318 Google Scholar
[39]	Bisset R N, Peña Ardila L A, Santos L 2021 Phys. Rev. Lett. 126 025301 Google Scholar
[40]	Zin P, Pylak M, Gajda M 2021 Phys. Rev. A 103 013312 Google Scholar
[41]	Ferrier-Barbut I, Wenzel M, Böttcher F, Langen T, Isoard M, Stringari S, Pfau T 2018 Phys. Rev. Lett. 120 160402 Google Scholar
[42]	Kartashov Y V, Malomed B A, Torner L 2020 Phys. Rev. Res. 2 033522 Google Scholar
[43]	Otajonov S R, Tsoy E N, Abdullaev F K 2020 Phys. Rev. E 102 062217
[44]	Luo Z H, Pang W, Liu B, Li Y Y, Malomed B A 2021 Front. Phys. 16 32201 Google Scholar
[45]	Liu B, Zhang H F, Zhong R X, Zhang X L, Qin X Z, Huang C Q, Li Y Y, Malomed B A 2019 Phys. Rev. A 99 053602 Google Scholar
[46]	Zhao F Y, Yan Z T, Cai X Y, Li C L, Chen G L, He H X, Liu B, Li Y Y 2021 Chaos Solitons Fractals 152 111313 Google Scholar
[47]	Huang H, Wang H C, Chen M, Lim C S, Wong K C 2022 Chaos Solitons Fractals 158 112079 Google Scholar
[48]	Guo M Y, Pfau T 2021 Front. Phys. 16 32202 Google Scholar
[49]	Malomed B A 2021 Front. Phys. 16 22504 Google Scholar
[50]	Cui X L 2018 Phys. Rev. A 98 023630 Google Scholar
[51]	Wang Y Q, Guo L F, Yi S, Shi T 2020 Phys. Rev. Res. 2 043074 Google Scholar
[52]	Guo Z C, Jia F, Li L T, Ma Y F, Hutson J M, Cui X L, Wang D J 2021 Phys. Rev. Res. 3 033247
[53]	Dong L W, Kartashov Y V 2021 Phys. Rev. Lett. 126 244101 Google Scholar
[54]	Zhang X L, Xu X X, Zheng Y Y, Chen Zh P, Liu B, Huang Ch Q, Malomed B A, Li Y Y 2019 Phys. Rev. Lett. 123 133901 Google Scholar
[55]	Zhou Z, Yu X, Zou Y, Zhong H H 2019 Commun. Nonlinear. Sci. Numer. Simulat. 78 104881
[56]	Dong L W, Qi W, Peng P, Wang L X, Zhou H, Huang Ch. M 2020 Nonlinear Dyn. 102 303 Google Scholar
[57]	Zheng Y Y, Chen S T, Huang Z P, Dai Sh X, Liu B, Li Y Y, Wang S R 2021 Front. Phys. 16 22501 Google Scholar
[58]	Li Y Y, Chen Z P, Luo Z H, Huang C Q, Tan H S, Pang W, Malomed B A 2018 Phys. Rev. A 98 063602 Google Scholar
[59]	Kartashov Y V, Malomed B A, Tarruell L, Torner L 2018 Phys. Rev. A 98 013612 Google Scholar
[60]	Lin Z D, Xu X X, Chen Z K, Yan Z T, Mai Z J, Liu B 2021 Commun. Nonlinear Sci. Numer. Simulat. 93 105536 Google Scholar
[61]	Semeghini G, Ferioli G, Masi L, Mazzinghi C, Wolswijk L, Minardi F, Modugno M, Modugno G, Inguscio M, Fattori M 2018 Phys. Rev. Lett. 120 235301 Google Scholar
[62]	Mihalache D, Mazilu D, Malomed B A, Lederer F 2006 Phys. Rev. A 73 043615 Google Scholar
[63]	Dror N, Malomed B A 2011 Physica D 240 526 Google Scholar
[64]	Yang J K, Lakoba T I 2008 Stud. Appl. Math. 120 265 Google Scholar

施引文献

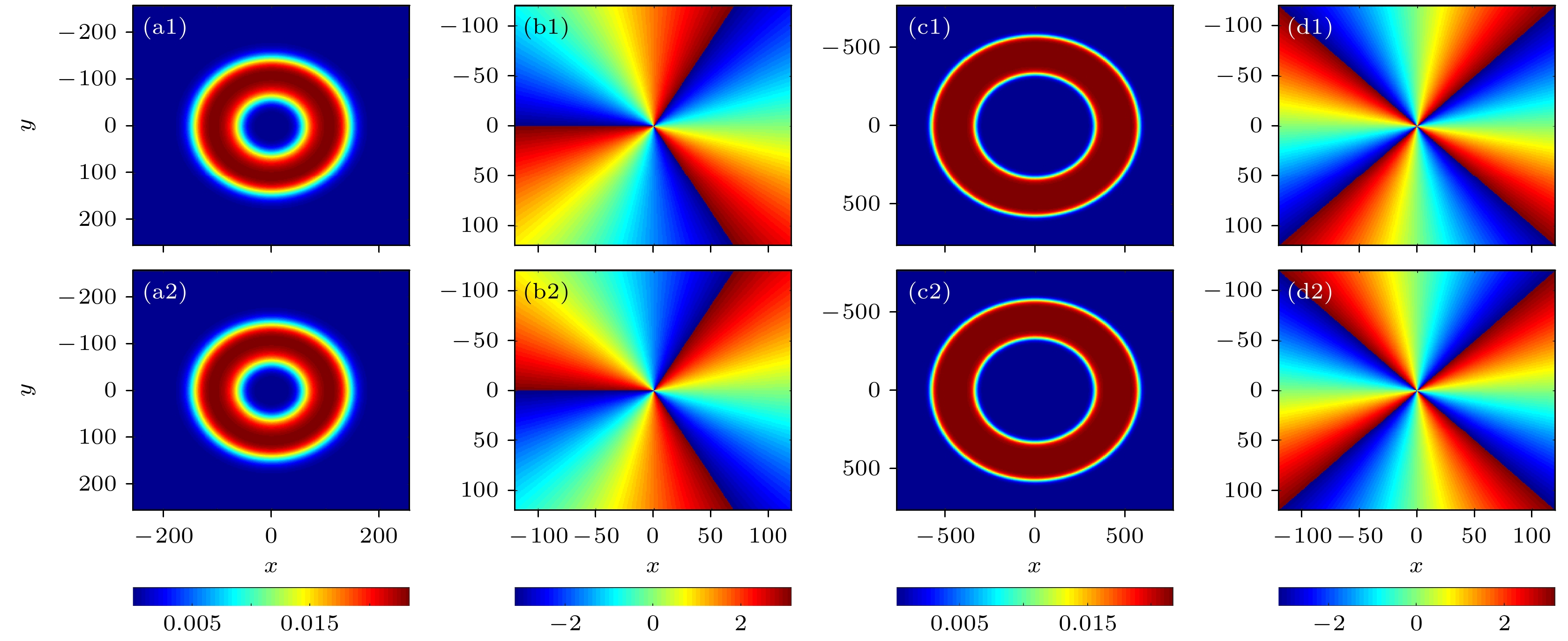
图 1 隐秘涡旋量子液滴的典型例子 (a1), (a2)总粒子数$N = 2500$时, 拓扑荷为${S_{1, 2}}{\text{ = }} \pm {\text{3}}$的两分量密度分布图; (b1), (b2) 分别对应其相位分布; (c1), (c2)总粒子数$N = 30000$时, 拓扑荷为${S_{1, 2}}{\text{ = }} \pm {\text{4}}$的两分量的密度分布图; (d1), (d2) 分别对应其相位分布. 系统参数为$g = 10$和$\text{δ} g = 0.5$

Fig. 1. Typical examples of stable hidden vortices of QDs: (a1), (a2) Density patterns of the two components with $(N, {S_{1, 2}}) = (2500, \pm {\text{3}})$; (b1), (b2) the corresponding phase diagrams of the hidden vortices of QDs are in panels (a1) and (a2), respectively; (c1), (c2) density patterns of the two components with $(N, {S_{1, 2}}) = (30000, \pm 4)$; (d1), (d2) the corresponding phase diagrams of the hidden vortices of QDs are in panels (c1) and (c2), respectively. The other parameters are $g = 10$ and $\text{δ} g = 0.5$.

下载: 全尺寸图片幻灯片

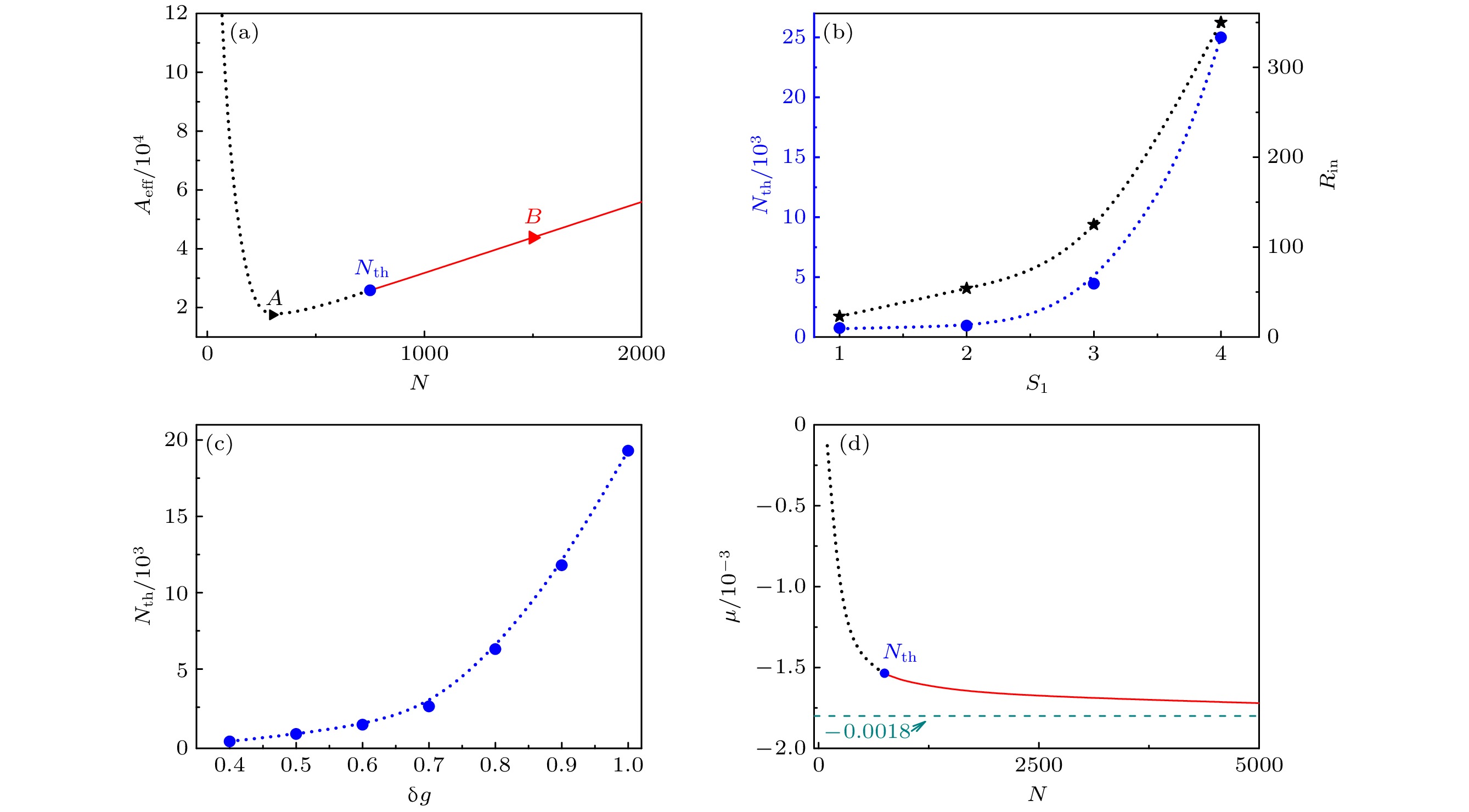
图 2 (a) 隐秘涡旋量子液滴的有效面积${A_{{\text{eff}}}}$与总粒子数$N$之间的依赖关系, ${N_{{\text{th}}}}$表示稳定与不稳定区域的临界值, 红色实线表示稳定区域, 黑色点虚线表示不稳定区域; (b) 蓝色点表示固定${\text{δ}}g = 0.5$时, 临界值${N_{{\text{th}}}}$与拓扑荷$ S_1$之间的依赖关系; 黑色“★”表示临界值下的隐秘涡旋量子液滴的内半径大小${R_{{\text{in}}}}$; (c) 固定${S_{1, 2}} = \pm 1$时, 不同$\delta g$值下的临界值${N_{{\text{th}}}}$; (d) 固定${S_{1, 2}} = \pm 1$时, 化学势$\mu $与总粒子数$N$之间的依赖关系

Fig. 2. (a) The dependence of the effective area${A_{{\text{eff}}}}$on the norm$N$of the hidden vortices of QDs, ${N_{{\text{th}}}}$is the threshold norm, red solid curve shows the stable region and the black dash curve shows the unstable region; (b) the blue dot represents the dependence of the threshold${N_{{\text{th}}}}$on the topological charge$ S_1$when${\text{δ}}g = 0.5$, the black star represents the inner radius of the hidden vortices of QDs at the threshold norm; (c) the threshold norm${N_{{\text{th}}}}$for hidden vortices of QDs with ${S_{1, 2}} = \pm 1$ as a function of ${\text{δ}}g$; (d) the dependence of the chemical potential$\mu $on the total norm$N$with ${S_{1, 2}} = \pm 1$.

下载: 全尺寸图片幻灯片

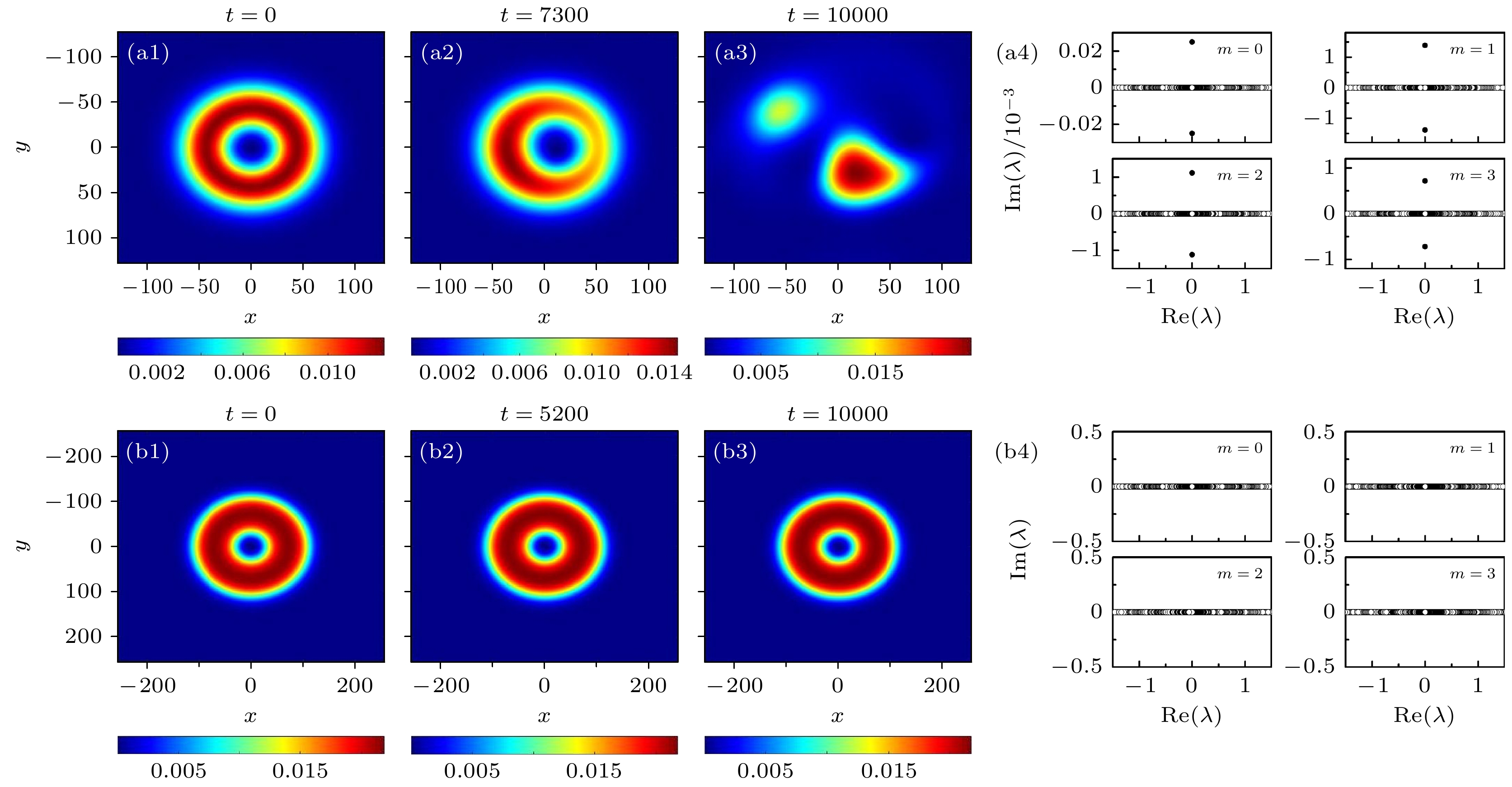
图 3 (a1)—(a3)$ N = 300 $时, ${\varPhi _1}$分量传输到$t = 0$, $t = 7300$和$t = 10000$时密度分布情况, 显然此时传输不稳定; (a4)涡旋拓扑荷数的扰动值$m = 0, 1, 2, 3$时不稳定增长率$\lambda $的实部和虚部的关系图; (b1)—(b3)$ N = 1500 $时, ${\varPhi _1}$分量传输到$t = 0$, $t = 5200$和$t = 10000$时密度分布情况, 显然此时传输稳定; (b4)涡旋拓扑荷数的扰动值$m = 0, 1, 2, 3$时不稳定增长率$\lambda $的实部和虚部的关系图

Fig. 3. (a1)–(a3) The density pattern of the ${\varPhi _1}$ component with $N = 300$ and $t = 0, 7300, 10000$, which is obviously unstable; (a4) perturbation eigenvalues for the corresponding hidden vortices of QDs with $N = 300$ and ${S_{1, 2}} = \pm 1$ for different azimuthal index $m = 0, 1, 2, 3$; (b1)–(b3) the density pattern of the ${\varPhi _1}$ component with $ N = 1500 $ and $t = 0, 5200, 10000$; (b4) perturbation eigenvalues for the corresponding hidden vortices of QDs with $N = 1500$ and ${S_{1, 2}} = \pm 1$ for different azimuthal index $m = 0, 1, 2, 3$.

下载: 全尺寸图片幻灯片

图 4 嵌套涡旋的典型例子 (a1)—(a3)$N = {\text{800}}, {S_{1, {\text{2}}}} = \pm 1$和$N = {\text{30000}}, {S_{1, 2}} = \pm 4$嵌套形成的嵌套涡旋量子液滴; (a4) 液滴传输到$t = 10000$时的相位分布; (b1)—(b3) $N = {\text{800}}, {S_{1, {\text{2}}}} = \pm 1$和$N = {\text{26000}}, {S_{1, 2}} = \pm 4$嵌套形成的嵌套涡旋量子液滴; (b4) 液滴传输到$t = 4500$时的相位分布; (c1)—(c3)$N = {\text{800}}, {S_{1, {\text{2}}}} = \pm 1$和$N = {\text{30000}}, {S_{1, 2}} = \mp 4$嵌套形成的嵌套涡旋量子液滴; (c4) 液滴传输到$t = 10000$时的相位分布; (d1)—(d3) $N = {\text{800}}, {S_{1, {\text{2}}}} = \pm 1$和$N = {\text{26000}}, {S_{1, 2}} = \mp 4$嵌套形成的嵌套涡旋量子液滴; (d4) 液滴传输到$t = 6400$时的相位分布

Fig. 4. Typical examples of the nested vortex QDs: (a1)—(a3) The hidden vortices of QDs with$(N, {S_{1, 2}}) = (800, \pm 1)$nests inside $(N, {S_{1, 2}}) = (30000, \pm 4)$, which has a larger inner radius; (a4)output pattern of the phase structure for the nested hidden vortices of QDs at $t = 10000$; (b1)–(b3) the hidden vortices of QDs with$(N, {S_{1, 2}}) = (800, \pm 1)$nests inside $(N, {S_{1, 2}}) = (26000, \pm 4)$; (b4) output pattern of the phase structure for the nested hidden vortices of QDs at $t = 4500$; (c1)–(c3) the hidden vortices of QDs with$(N, {S_{1, 2}}) = (800, \pm 1)$nests inside $(N, {S_{1, 2}}) = (30000, \mp 4)$; (c4) output pattern of the phase structure for the nested hidden vortices of QDs at $t = 10000$; (d1)–(d3) the hidden vortices of QDs with$(N, {S_{1, 2}}) = (800, \pm 1)$nests inside $(N, {S_{1, 2}}) = (26000, \mp 4)$; (d4) output pattern of the phase structure for the nested hidden vortices of QDs at $t = 6400$.

下载: 全尺寸图片幻灯片

map

[1]	Xu Y, Zhang Y P, Wu B 2013 Phys. Rev. A 87 013614 Google Scholar
[2]	Han W, Zhang X F, Wang D S, Jiang H F, Zhang W, Zhang S G 2018 Phys. Rev. Lett. 121 030404 Google Scholar
[3]	Zhang Y C, Zhou Z W, Malomed B A, Pu H 2015 Phys. Rev. Lett. 115 253902 Google Scholar
[4]	何章明, 张志强, 朱善华, 柳闻鹃 2014 63 190502 Google Scholar He Z M, Zhang Z Q, Zhu S H, Liu W J 2014 Acta Phys. Sin. 63 190502 Google Scholar
[5]	郭慧, 王雅君, 王林雪, 张晓斐 2020 69 010302 Google Scholar Guo H, Wang Y J, Wang L X, Zhang X F 2020 Acta Phys. Sin. 69 010302 Google Scholar
[6]	文林, 梁毅, 周晶, 余鹏, 夏雷, 牛连斌, 张晓斐 2019 68 080301 Google Scholar Wen L, Liang Y, Zhou J, Yu P, Xia L, Niu L B, Zhang X F 2019 Acta Phys. Sin. 68 080301 Google Scholar
[7]	Zhang R F, Zhang X F, Li L 2019 Phys. Lett. A 383 231 Google Scholar
[8]	Zhang R F, Zhang Y P, Li L 2019 Phys. Lett. A 383 3175 Google Scholar
[9]	贾瑞煜, 方乒乒, 高超, 林机 2021 70 180303 Google Scholar Jia R Y, Fang P P, Gao C, Lin J 2021 Acta Phys. Sin. 70 180303 Google Scholar
[10]	陈海军, 李向富 2013 62 070302 Google Scholar Chen H J, Li X F 2013 Acta Phys. Sin. 62 070302 Google Scholar
[11]	Li Y E, Xue J K 2016 Chin. Phys. Lett. 33 100502 Google Scholar
[12]	周嘉仪, 李画眉 2014 浙江师范大学学报 38 303 Zhou J Y, Li H M 2014 J. Zhejiang Normal Univ. 38 303
[13]	唐娜, 杨雪滢, 宋琳, 张娟, 李晓霖, 周志坤, 石玉仁 2020 69 010301 Google Scholar Tang N, Yang X Y, Song L, Zhang J, Li X L, Zhou Z K, Shi Y R 2020 Acta Phys. Sin. 69 010301 Google Scholar
[14]	Wen L, Sun Q, Wang H Q, Ji A C, Liu W M 2012 Phys. Rev. A 86 043602 Google Scholar
[15]	Wang L X, Dai C Q, Wen L, Liu T, Jiang H F, Saito H, Zhang Sh G, Zhang X F 2018 Phys. Rev. A 97 063607 Google Scholar
[16]	Peng P, Li G Q, Zhao L C, Yang W L, Yang Z Y 2019 Phys. Lett. A 383 2883 Google Scholar
[17]	Segev M, Valley G C, Crosignani B, DiPorto P, Yariv A 1994 Phys. Rev. Lett. 73 3211 Google Scholar
[18]	Huang J S, Jiang X D, Chen H Y, Fan Z W, Pang W, Li Y Y 2015 Front. Phys. 10 100507
[19]	Maucher F, Henkel N, Saffman M, Królikowski W, Skupin S, Pohl T 2011 Phys. Rev. Lett. 106 170401 Google Scholar
[20]	Wang Y Y, Chen L, Dai C Q, Zheng J, Fan Y 2017 Nonlinear Dyn. 90 1269 Google Scholar
[21]	Dai C Q, Chen R P, Wang Y Y, Fan Y 2017 Nonlinear Dyn. 87 1675 Google Scholar
[22]	Chen Y X, Zheng L H, Xu F Q 2018 Nonlinear Dyn. 93 2379 Google Scholar
[23]	Li J T, Zhu Y, Han J Z, Qin W, Dai C Q, Wang S H 2018 Nonlinear Dyn. 91 757 Google Scholar
[24]	Chen X W, Deng Z G, Xu X X, Li S L, Fan Z W, Chen Z P, Liu B, Li Y Y 2020 Nonlinear Dyn. 101 569 Google Scholar
[25]	Ye Z J, Chen Y X, Zheng Y Y, Chen X W, Liu B 2020 Chaos Solitons Fractals 130 109418 Google Scholar
[26]	Liu B, Zhong R X, Chen Z P, Qin X Z, Zhong H H, Li Y Y, Malomed B A 2020 New J. Phys. 22 043004 Google Scholar
[27]	Li Y Y, Luo Z H, Liu Y, Chen Z P, Huang C Q, Fu S H, Tan H S, Malomed B A 2017 New J. Phys. 19 113043 Google Scholar
[28]	Hu X H, Zhang X F, Zhao D, Luo H G, Liu W M 2009 Phys. Rev. A 79 023619 Google Scholar
[29]	李吉, 刘斌, 白晶, 王寰宇, 何天琛 2020 69 140301 Google Scholar Li J, Liu B, Bai J, Wang H Y, He T C 2020 Acta Phys. Sin. 69 140301 Google Scholar
[30]	陈海军, 任元, 王华 2022 71 056701 Google Scholar Chen H J, Ren Y, Wang H 2022 Acta Phys. Sin. 71 056701 Google Scholar
[31]	Schmitt M, Wenzel M, Böttcher F, Ferrier-Barbut I, Pfau T 2016 Nature 539 259 Google Scholar
[32]	Cabrera C R, Tanzi L, Sanz J, Naylor B, Thomas P, Cheiney P, Tarruell L 2018 Science 359 301 Google Scholar
[33]	Lee T D, Huang K, Yang C N 1957 Phys. Rev. 106 1135 Google Scholar
[34]	Tylutki M, Astrakharchik G E, Malomed B A, Petrov D S 2020 Phys. Rev. A 101 051601 Google Scholar
[35]	Boudjemâa A 2018 Phys. Rev. A 98 033612 Google Scholar
[36]	Hu H, Liu X J 2020 Phys. Rev. A 102 043302 Google Scholar
[37]	Pylak M, Gampel F, Płodzień M, Gajda M 2022 Phys. Rev. Res. 4 013168 Google Scholar
[38]	Parisi L, Giorgini S 2020 Phys. Rev. A 102 023318 Google Scholar
[39]	Bisset R N, Peña Ardila L A, Santos L 2021 Phys. Rev. Lett. 126 025301 Google Scholar
[40]	Zin P, Pylak M, Gajda M 2021 Phys. Rev. A 103 013312 Google Scholar
[41]	Ferrier-Barbut I, Wenzel M, Böttcher F, Langen T, Isoard M, Stringari S, Pfau T 2018 Phys. Rev. Lett. 120 160402 Google Scholar
[42]	Kartashov Y V, Malomed B A, Torner L 2020 Phys. Rev. Res. 2 033522 Google Scholar
[43]	Otajonov S R, Tsoy E N, Abdullaev F K 2020 Phys. Rev. E 102 062217
[44]	Luo Z H, Pang W, Liu B, Li Y Y, Malomed B A 2021 Front. Phys. 16 32201 Google Scholar
[45]	Liu B, Zhang H F, Zhong R X, Zhang X L, Qin X Z, Huang C Q, Li Y Y, Malomed B A 2019 Phys. Rev. A 99 053602 Google Scholar
[46]	Zhao F Y, Yan Z T, Cai X Y, Li C L, Chen G L, He H X, Liu B, Li Y Y 2021 Chaos Solitons Fractals 152 111313 Google Scholar
[47]	Huang H, Wang H C, Chen M, Lim C S, Wong K C 2022 Chaos Solitons Fractals 158 112079 Google Scholar
[48]	Guo M Y, Pfau T 2021 Front. Phys. 16 32202 Google Scholar
[49]	Malomed B A 2021 Front. Phys. 16 22504 Google Scholar
[50]	Cui X L 2018 Phys. Rev. A 98 023630 Google Scholar
[51]	Wang Y Q, Guo L F, Yi S, Shi T 2020 Phys. Rev. Res. 2 043074 Google Scholar
[52]	Guo Z C, Jia F, Li L T, Ma Y F, Hutson J M, Cui X L, Wang D J 2021 Phys. Rev. Res. 3 033247
[53]	Dong L W, Kartashov Y V 2021 Phys. Rev. Lett. 126 244101 Google Scholar
[54]	Zhang X L, Xu X X, Zheng Y Y, Chen Zh P, Liu B, Huang Ch Q, Malomed B A, Li Y Y 2019 Phys. Rev. Lett. 123 133901 Google Scholar
[55]	Zhou Z, Yu X, Zou Y, Zhong H H 2019 Commun. Nonlinear. Sci. Numer. Simulat. 78 104881
[56]	Dong L W, Qi W, Peng P, Wang L X, Zhou H, Huang Ch. M 2020 Nonlinear Dyn. 102 303 Google Scholar
[57]	Zheng Y Y, Chen S T, Huang Z P, Dai Sh X, Liu B, Li Y Y, Wang S R 2021 Front. Phys. 16 22501 Google Scholar
[58]	Li Y Y, Chen Z P, Luo Z H, Huang C Q, Tan H S, Pang W, Malomed B A 2018 Phys. Rev. A 98 063602 Google Scholar
[59]	Kartashov Y V, Malomed B A, Tarruell L, Torner L 2018 Phys. Rev. A 98 013612 Google Scholar
[60]	Lin Z D, Xu X X, Chen Z K, Yan Z T, Mai Z J, Liu B 2021 Commun. Nonlinear Sci. Numer. Simulat. 93 105536 Google Scholar
[61]	Semeghini G, Ferioli G, Masi L, Mazzinghi C, Wolswijk L, Minardi F, Modugno M, Modugno G, Inguscio M, Fattori M 2018 Phys. Rev. Lett. 120 235301 Google Scholar
[62]	Mihalache D, Mazilu D, Malomed B A, Lederer F 2006 Phys. Rev. A 73 043615 Google Scholar
[63]	Dror N, Malomed B A 2011 Physica D 240 526 Google Scholar
[64]	Yang J K, Lakoba T I 2008 Stud. Appl. Math. 120 265 Google Scholar

[1]	李新月, 祁娟娟, 赵敦, 刘伍明. 自旋-轨道耦合二分量玻色-爱因斯坦凝聚系统的孤子解. , 2023, 72(10): 106701. doi: 10.7498/aps.72.20222319
[2]	邢健崇, 张文静, 杨涛. 玻色-爱因斯坦凝聚中的非正则涡旋态及其动力学. , 2023, 72(10): 100306. doi: 10.7498/aps.72.20222289
[3]	邱旭, 王林雪, 陈光平, 胡爱元, 文林. 自旋张量-动量耦合玻色-爱因斯坦凝聚的动力学性质. , 2023, 72(18): 180304. doi: 10.7498/aps.72.20231076
[4]	郭慧, 王雅君, 王林雪, 张晓斐. 玻色-爱因斯坦凝聚中的环状暗孤子动力学. , 2020, 69(1): 010302. doi: 10.7498/aps.69.20191424
[5]	文林, 梁毅, 周晶, 余鹏, 夏雷, 牛连斌, 张晓斐. 线性塞曼劈裂对自旋-轨道耦合玻色-爱因斯坦凝聚体中亮孤子动力学的影响. , 2019, 68(8): 080301. doi: 10.7498/aps.68.20182013
[6]	贺丽, 余增强. 自旋-轨道耦合作用下玻色-爱因斯坦凝聚在量子相变附近的朗道临界速度. , 2017, 66(22): 220301. doi: 10.7498/aps.66.220301
[7]	李志, 王建忠. 自旋-轨道耦合玻色-爱因斯坦凝聚势垒散射特性的研究. , 2013, 62(10): 100306. doi: 10.7498/aps.62.100306
[8]	赵文垒, 豆福全, 王建忠. 玻色-爱因斯坦凝聚体中非线性相互作用对量子共振棘流的影响. , 2012, 61(22): 220503. doi: 10.7498/aps.61.220503
[9]	王建忠, 曹辉, 豆福全. 玻色-爱因斯坦凝聚体Rosen-Zener跃迁中的多体量子涨落效应. , 2012, 61(22): 220305. doi: 10.7498/aps.61.220305
[10]	宋立军, 严冬, 刘烨. 玻色-爱因斯坦凝聚系统的量子Fisher信息与混沌. , 2011, 60(12): 120302. doi: 10.7498/aps.60.120302
[11]	曲春雷, 赵清. 周期驱动玻色-爱因斯坦凝聚系统的棘齿效应. , 2009, 58(7): 4390-4395. doi: 10.7498/aps.58.4390
[12]	严冬, 宋立军, 陈殿伟. 两分量玻色-爱因斯坦凝聚系统的自旋压缩. , 2009, 58(6): 3679-3684. doi: 10.7498/aps.58.3679
[13]	宗丰德, 杨阳, 张解放. 外势场作用下的玻色-爱因斯坦凝聚啁啾孤子的演化与操控. , 2009, 58(6): 3670-3678. doi: 10.7498/aps.58.3670
[14]	王海雷, 杨世平. 三势阱中玻色-爱因斯坦凝聚的开关特性. , 2008, 57(8): 4700-4705. doi: 10.7498/aps.57.4700
[15]	王志霞, 张喜和, 沈柯. 玻色-爱因斯坦凝聚中的混沌反控制. , 2008, 57(12): 7586-7590. doi: 10.7498/aps.57.7586
[16]	房永翠, 杨志安, 杨丽云. 对称双势阱玻色-爱因斯坦凝聚系统在周期驱动下的动力学相变及其量子纠缠熵表示. , 2008, 57(2): 661-666. doi: 10.7498/aps.57.661
[17]	宗丰德, 张解放. 装载于外势场中的玻色-爱因斯坦凝聚N-孤子间的相互作用. , 2008, 57(5): 2658-2668. doi: 10.7498/aps.57.2658
[18]	刘泽专, 杨志安. 噪声对双势阱玻色-爱因斯坦凝聚体系自俘获现象的影响. , 2007, 56(3): 1245-1252. doi: 10.7498/aps.56.1245
[19]	王冠芳, 傅立斌, 赵鸿, 刘杰. 双势阱玻色-爱因斯坦凝聚体系的自俘获现象及其周期调制效应. , 2005, 54(11): 5003-5013. doi: 10.7498/aps.54.5003
[20]	徐　岩, 贾多杰, 李希国, 左　维, 李发伸. 大N近似下玻色-爱因斯坦凝聚体中单个涡旋态的解. , 2004, 53(9): 2831-2834. doi: 10.7498/aps.53.2831

计量

文章访问数: 4764
PDF下载量: 143
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

准二维空间中的隐秘涡旋量子液滴