-
研究了半开放系统中粒子向开放空间的隧穿问题. 考虑由无限高的墙和多个
$\delta$ 函数势垒组成的半Dirac梳模型, 首先求解该模型的精确解析解, 其能量本征函数可以用递推关系以封闭解析的形式给出. 对单个势垒、多个势垒、无序势垒等不同情况, 利用傅里叶积分计算了任意时刻单粒子波函数的明确表示, 导出了由初态保真度定义的粒子生存几率闭合形式的表达式, 重点研究了粒子生存几率对势垒高度、无序强度等系统参数的依赖, 以及利用相关参数对衰减规律的操控及抑制. 发现多个势垒将大幅度提高粒子的生存几率, 无序的加入会极大地抑制其随时间的振荡.We investigated the tunneling properties of a particle in a semi-open system. Starting initially from the eigenstate of the particle in the one-dimensional infinite well, we quench the infinitely high barrier on the right into a series of $\delta$ barriers to observe the survival probability which is defined as the fidelity to the initial state. This constitutes a semi-Dirac comb model consisting of an infinitely high wall and multiple equally spaced$\delta$ -potential barriers. We first solve the exact analytical solution of this model and obtain the closed analytic form of the eigen function expressed by a recursive relation. For a single barrier, multiple potential barriers, the disordered potential barriers, the closed-form expression of the survival probability i.e., the initial state fidelity, is given for any evolution time and it is used to reveal the mechanism of the particle escape process. The dependence of survival probability on the strength of barrier, number of barriers, and disorder strength is calculated numerically based on fast Fourier transform method. The relevant parameters are used to control and suppress the particle escape problem. We found that for a single$\delta$ -potential barrier, the survival probability of the particle follows different trends in different decay time ranges. The particle in the ground state or excited states decays exponentially in a short time. After some time, the decay of the excited state will proceed with the same decay constant as that of the ground state. Finally, the survival probability follows a long-time inverse power law. The curve changes abruptly at different decay time intervals and is accompanied by significant oscillations. These oscillations in the transition region are caused by the interference of the exponential rate and the inverse power-law term, while the long-time non-exponential decay is due to the fact that the system energy spectrum has a lower bound. Increasing the barrier strength will greatly increase the probability of particles remaining in the well.For multiple potential barriers, the reflection and transmission of particles between the potential barriers interfere with each other. When the strength of the potential barrier is small, the particle still decays exponentially. For a larger potential barrier strength, the probability of particle reflection increases, and the particles that tunnel out may be bounced back. The survival probability oscillates sharply, reaching higher fidelity at certain moments. The oscillatory maximum of the survival probability decreases linearly with the number of barriers, while the moment corresponding to the oscillatory maximum shows a parabolic increase with the number of barriers. The introduction of a series disordered barriers can significantly improve the survival probability and greatly suppress its oscillations over time. Our calculation is expected to find applications in quantum control of particle escape problem in the disordered system. -
Keywords:
- semi-open system /
- quantum tunneling /
- survival probability /
- disorder system
[1] Gamow G 1928 Z. Phys. 51 204
Google Scholar
[2] Gurney R W, Condon E U 1928 Nature 122 439
Google Scholar
[3] Talou P, Strottman D, Carjan N 1999 Phys. Rev. C 60 054318
Google Scholar
[4] Talou P, Carjan N, Negrevergne C, Strottman D 2000 Phys. Rev. C 62 014609
Google Scholar
[5] Balantekin A B, Takigawa N 1998 Rev. Mod. Phys. 70 77
Google Scholar
[6] Bhandari B S 1991 Phys. Rev. Lett. 66 1034
Google Scholar
[7] Vatasescu M, Dulieu O, Amiot C, Comparat D, Drag C, Kokoouline V, Masnou-Seeuws F, Pillet P 2000 Phys. Rev. A 61 044701
Google Scholar
[8] Keller J, Weiner J 1984 Phys. Rev. A 29 2943
Google Scholar
[9] Ricco B, Azbel M Y 1984 Phys. Rev. B 29 1970
Google Scholar
[10] Razavy M 2003 Quantum Theory of Tunneling (Singapore: World Scientific)
[11] Ueda M, Leggett A J 1998 Phys. Rev. Lett. 80 1576
Google Scholar
[12] Salasnich L, Parola A, Reatto L 2001 Phys. Rev. A 64 023601
Google Scholar
[13] Carr L D, Holland M J, Malomed B A 2005 J. Phys. B 38 3217
Google Scholar
[14] Schlagheck P, Paul T 2006 Phys. Rev. A 73 023619
Google Scholar
[15] Huhtamaki J A M, Mottonen M, Ankerhold J, Virtanen S M M 2007 Phys. Rev. A 76 033605
Google Scholar
[16] Zhao X, Alcala D A, McLain M A, Maeda K, Potnis S, Ramos R, Steinberg A M, Carr L D 2017 Phys. Rev. A 96 063601
Google Scholar
[17] Meyrath T P, Schreck F, Hanssen J L, Chuu C S, Raizen M G 2005 Phys. Rev. A 71 041604
Google Scholar
[18] Henderson K, Ryu C, MacCormick C, Boshier M G 2009 New J. Phys. 11 043030
Google Scholar
[19] Van Es J J P, Wicke P, Van Amerongen A H, Rétif C, Whitlock S, Van Druten N J 2010 J. Phys. B 43 155002
Google Scholar
[20] Gorlitz A, Vogels J M, Leanhardt A E, Raman C, Gustavson T L, Abo-Shaeer J R, Chikkatur A P, Gupta S, Inouye S, Rosenband T, Ketterle W 2001 Phys. Rev. Lett. 87 130402
Google Scholar
[21] Greiner M, Bloch I, Mandel O, Hansch T W, Esslinger T 2001 Phys. Rev. Lett. 87 160405
Google Scholar
[22] Schreck F, Khaykovich L, Corwin K L, Ferrari G, Bourdel T, Cubizolles J, Salomon C 2001 Phys. Rev. Lett. 87 080403
Google Scholar
[23] Stoferle T, Moritz H, Schori C, Kohl M, Esslinger T 2004 Phys. Rev. Lett. 92 130403
Google Scholar
[24] Serwane F, Zurn G, Lompe T, Ottenstein T B, Wenz A N, Jochim S 2011 Science 332 336
Google Scholar
[25] Pethick C J, Smith H 2008 Bose-Einstein Condensation in Dilute Gases (Cambridge: Cambridge University Press)
[26] Chin C, Grimm R, Julienne P, Tiesinga E 2010 Rev. Mod. Phys. 82 1225
Google Scholar
[27] Zurn G, Serwane F, Lompe T, Wenz A N, Ries M G, Bohn J E, Jochim S 2012 Phys. Rev. Lett. 108 075303
Google Scholar
[28] Zurn G, Wenz A N, Murmann S, Bergschneider A, Lompe T, Jochim S 2013 Phys. Rev. Lett. 111 175302
Google Scholar
[29] Winter R G 1961 Phys. Rev. 123 1503
Google Scholar
[30] Garcia-Calderón G, Mateos J L, Moshinsky M 1995 Phys. Rev. Lett. 74 337
Google Scholar
[31] Wilkinson S R, Bharucha C F, Fischer M C, Madison K W, Morrow P R, Niu Q, Sundaram B, Raizen M G 1997 Nature 387 575
Google Scholar
[32] Kelkar N G, Nowakowski M, Khemchandani K P 2004 Phys. Rev. C 70 024601
Google Scholar
[33] Crespi A, Pepe F V, Facchi P, Sciarrino F, Mataloni P, Nakazato H, Pascazio S, Osellame R 2019 Phys. Rev. Lett. 122 130401
Google Scholar
[34] Garcia-Calderón G, Mendoza-Luna L G 2011 Phys. Rev. A 84 032106
Google Scholar
[35] Dobrzyniecki J, Sowiński T 2018 Phys. Rev. A 98 013634
Google Scholar
[36] Dobrzyniecki J, Sowiński T 2019 Phys. Rev. A 99 063608
Google Scholar
[37] Lode A U J, Streltsov A I, Alon O E, Meye H D, Cederbaum L S 2009 J. Phys. B 42 044018
Google Scholar
[38] Lode A U J, Streltsov A I, Sakmann K, Alon O E, Cederbaum L S 2012 Proc. Natl. Acad. Sci. U.S.A. 109 13521
Google Scholar
[39] Gharashi S E, Blume D 2015 Phys. Rev. A 92 033629
Google Scholar
[40] Kim S, Brand J 2011 J. Phys. B 44 195301
Google Scholar
[41] Ishmukhamedov I S, Ishmukhamedov A S 2019 Physica E 109 24
Google Scholar
[42] Hunn S, Zimmermann K, Hiller M, Buchleitner A 2013 Phys. Rev. A 87 043626
Google Scholar
[43] Taniguchi T, Sawada S I 2011 Phys. Rev. E 83 026208
Google Scholar
[44] Maruyama T, Oishi T, Hagino K, Sagawa H 2012 Phys. Rev. C 86 044301
[45] Kościk P 2020 Phys. Rev. A 102 033308
Google Scholar
[46] Peshkin M, Volya A, Zelevinsky V 2014 Europhys. Lett. 107 40001
Google Scholar
[47] Qin P, Yin C, Chen S 2014 Phys. Rev. B 90 054303
[48] Zhang Z, Tong P, Gong J, Li B 2012 Phys. Rev. Lett. 108 070603
Google Scholar
-
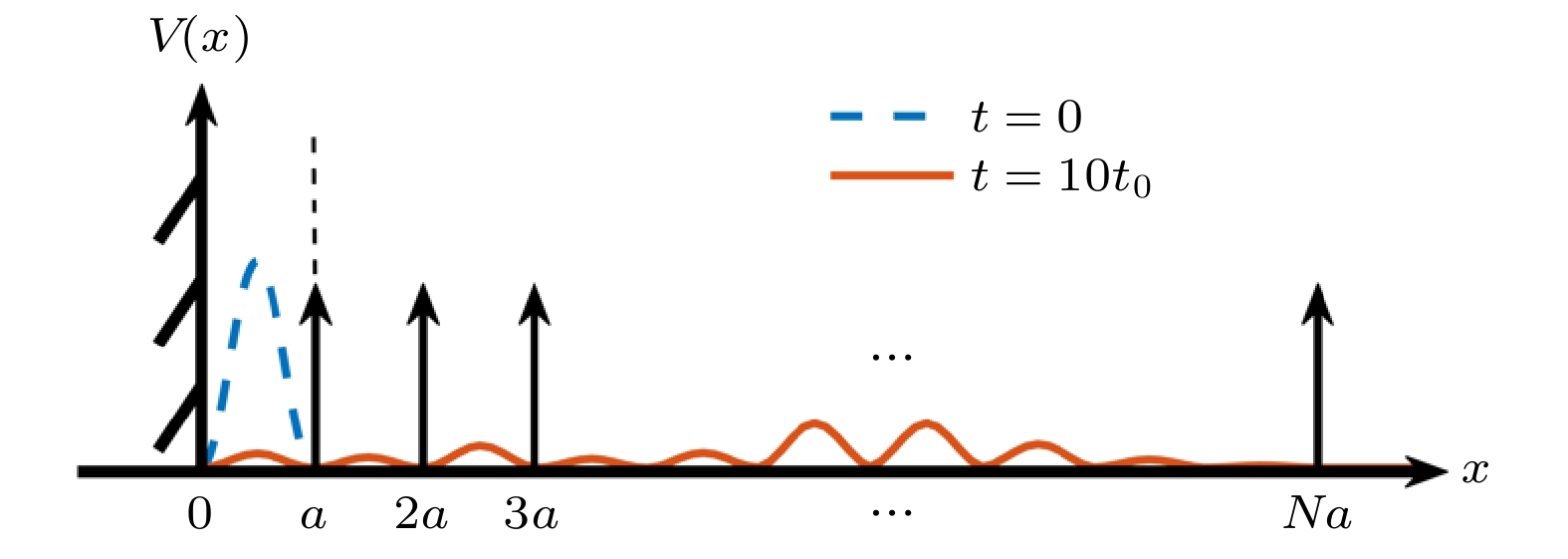
图 1 半开放系统中的粒子逃逸问题. 初始位于宽度为a的无限深势阱中的粒子, 右势垒突然撤掉后向开放空间隧穿, 右方N个势垒与原来的系统组成了半Dirac梳. 图中显示了初始时刻及
$t=10 t_0$ 时在各阱的几率密度分布. 这里取$N=10$ Fig. 1. Particle escape problems from a semi-open system. A particle initially in the eigenstate of an infinite potential well of width a is released at
$t=0$ and tunnels into open space when the right barrier is suddenly switched off, and the N$\delta$ -barrier on the right form a semi-Dirac comb with the original well. The probability density is shown for$t=0$ and$10 t_0$ .
图 2 (a)单个强度为
$h=10 h_0$ 的势垒, 能量最低的5个初始态的生存几率(n表示初态时粒子所处的能级); (b)短时情况, 黑色虚线处粒子的衰减率随着能级增高而增大; (c)中间时刻, 黑色虚线部分粒子的衰减率不随能级而变化; (d)长时情况, 粒子生存几率最后趋于的平衡值($\ln S_{\infty}$ ), 随着能级增高而减小Fig. 2. (a) The survival probability of the five initial states with the lowest energy for single barrier
$(N=1)$ with strength$h=10 h_0$ ; (b) the decay rate of the particle (black dotted lines) in short-term becomes faster for higher energy level; (c) in the medium-term, the decay rate of the particle (black dotted lines) does not change with n; (d) the equilibrium value ($\ln S_{\infty}$ ) of the survival probability after the long-term decay decreases as the energy level increases
图 3 (a)单个强度为
$h=(10, 20, 30, 40)h_0$ 的势垒初始基态的生存几率($n=1$ ); (b)短时情况, 黑色虚线处粒子的衰减率随着势垒强度的增大而减小; (c)中间时刻, 粒子保持短时内的衰减率; (d)长时情况, 粒子生存几率最后趋于的平衡值随着势垒强度的增大而减小Fig. 3. (a) The survival probability of the initial ground state (
$n=1$ ) when$N=1$ and the barrier strengths$h=(10, 20, $ $ 30, 40)h_0$ ; (b) the decay rate of the particle (black dotted lines) in the short-term decreases with the increase of the barrier strength; (c) particle maintains a short-term decay rate in the medium-term; (d) the equilibrium value of the particle survival probability after the long-term decay decreases with the increase of the barrier strength
图 4 (a)势垒数目
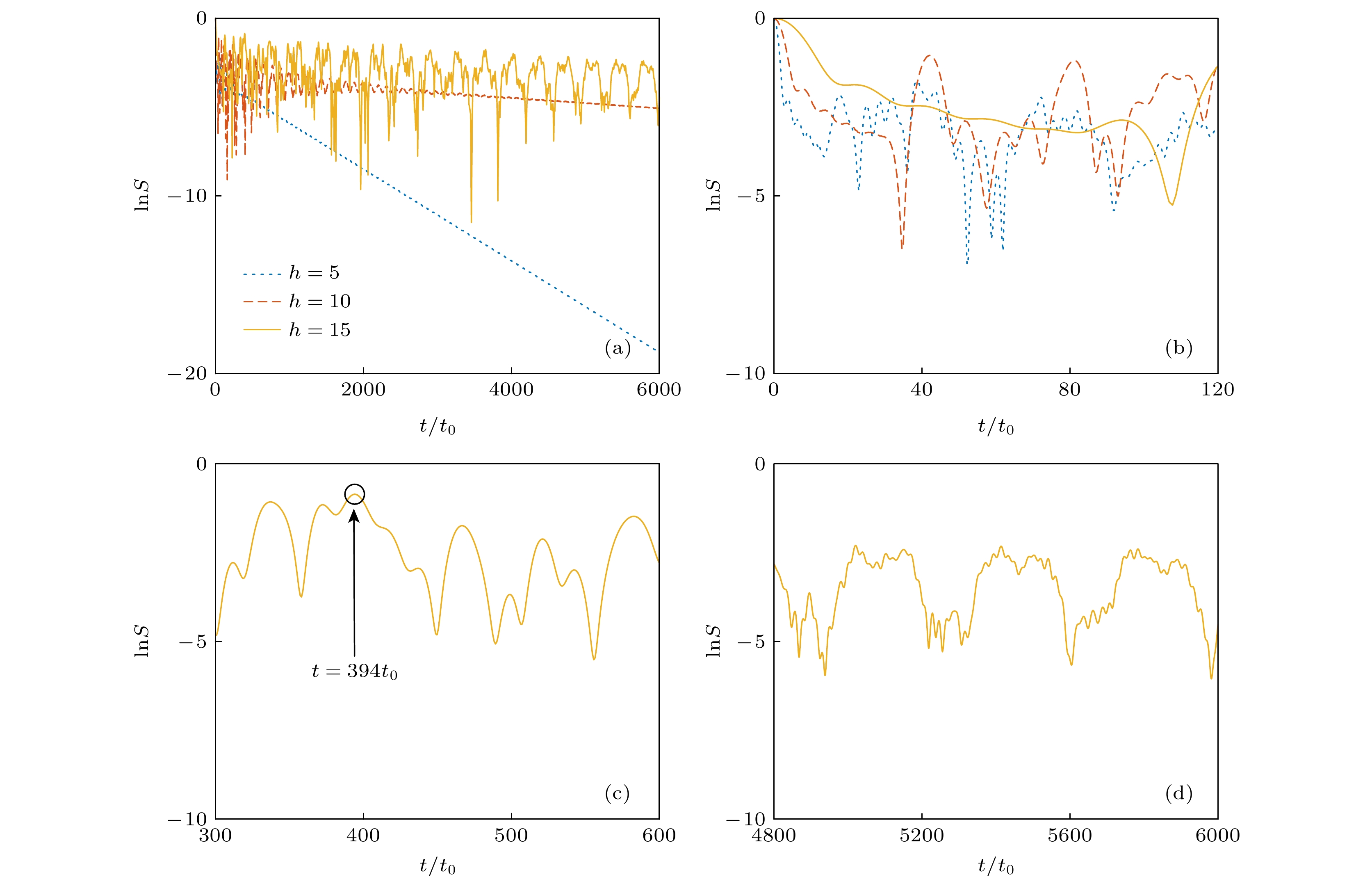
$N=10$ , 势垒强度$h=(5, 15, 50)h_0$ 时能量最低的初始基态的生存几率; (b)-(d)分别为短时、中时、长时的行为. (c)图黑色圆圈处为$h=50 h_0$ 时粒子生存几率的振荡最高点Fig. 4. (a) The survival probability of the initial state with the lowest energy for
$N=10$ identical barriers for three barrier strengths$h=(5, 15, 50)h_0$ ; (b)-(d) are short-term, medium-term, long-term behavior, respectively. The black circle in panel (c) indicates the highest point of oscillation of the survival probability for$h=50 h_0$ 
图 5 势垒强度
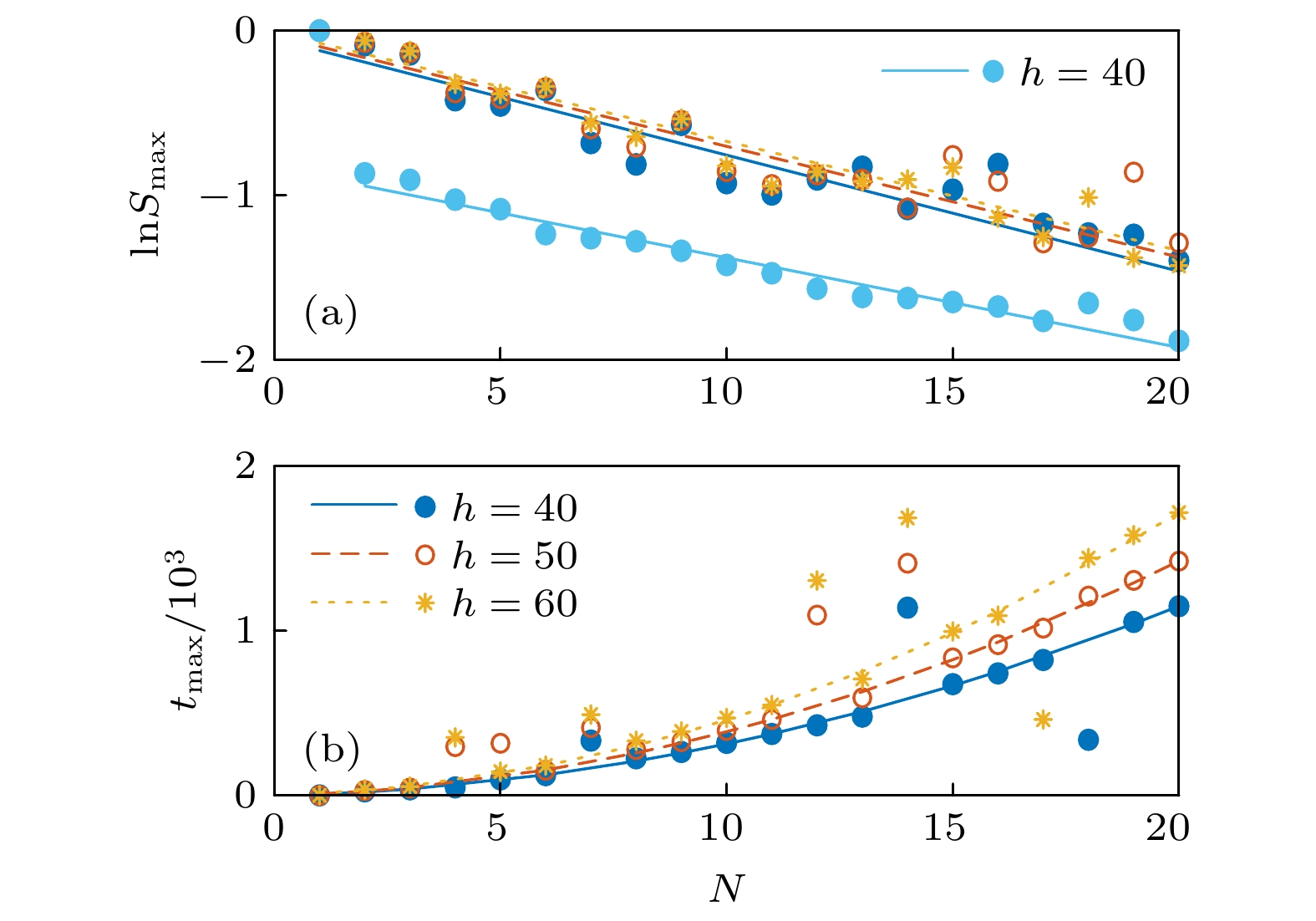
$h=(40, 50, 60)h_0$ 时, 粒子生存几率的振荡最高点(a)及其对应的时间(b)随势垒数目的变化. 浅蓝色圆点对应$h=40$ 时不同势垒数目下粒子生存几率振荡多个最高点(此处取20个)的平均值Fig. 5. Variation of the peak value of the oscillation of survival probability (a) and its corresponding time (b) with the number of barriers for barrier strengths
$h=(40, 50, $ $ 60)h_0$ . The light blue dots in (a) correspond to the average of 20 highest points in the oscillation for different numbers of potential barriers with strength$h=40$ 
图 6
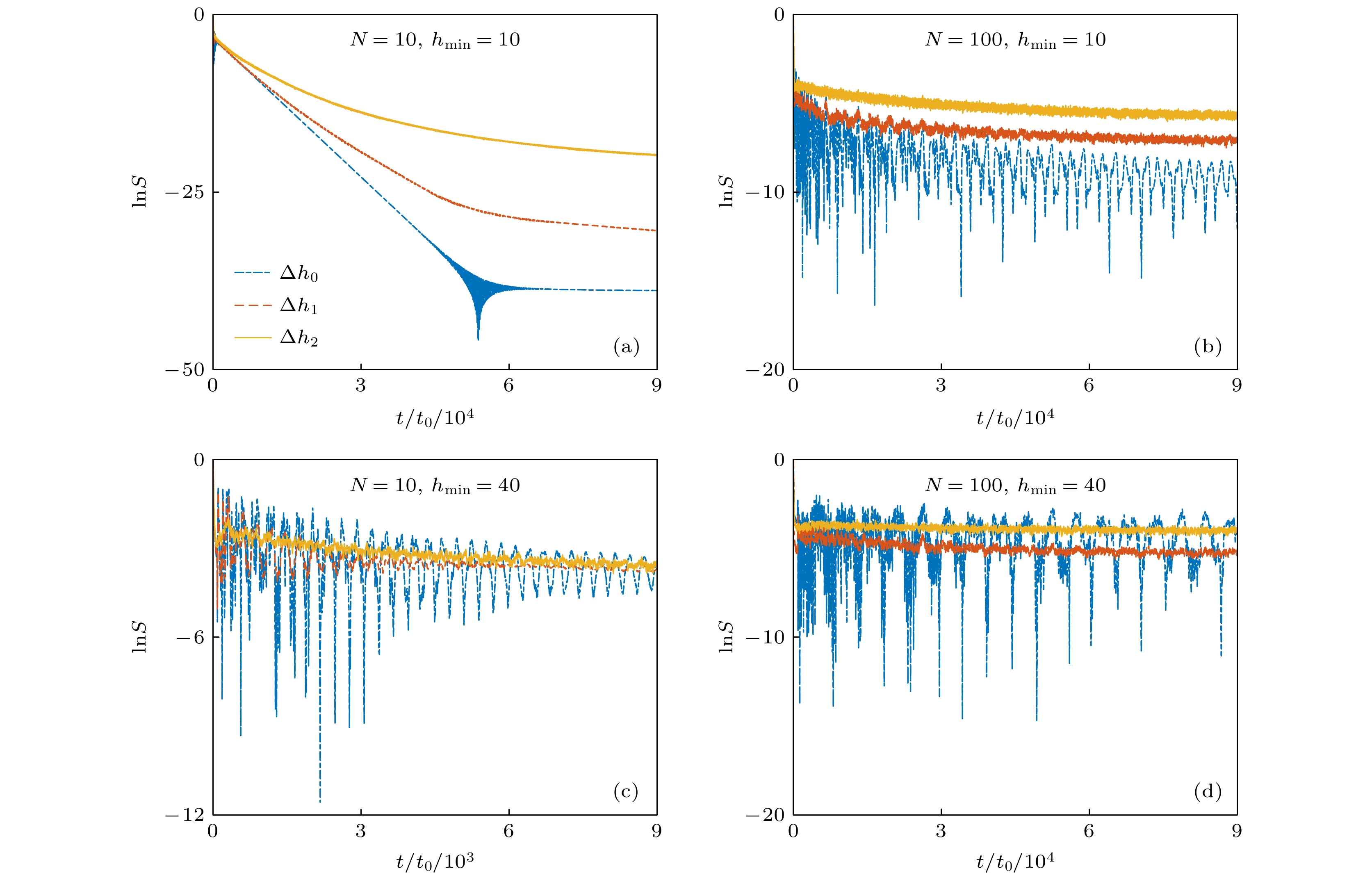
$N=10$ 和$N=100$ 个无序势垒组成的半开放系统中粒子的生存几率在大小两种无序强度下与没有无序($\Delta h_0 = 0$ )情况的对比. 图中所有数据都是100 次无序势垒构型的平均 (a), (b)$h_{{\rm{min}}}=10 h_0$ ,$\Delta h_1 = 3 h_0$ ,$\Delta h_2 = 9 h_0$ ; (c), (d)$h_{{\rm{min}}}=40 h_0$ ,$\Delta h_1 = 5 h_0$ ,$\Delta h_2 = 35 h_0$ Fig. 6. The survival probability of the particle for
$N=10$ and$N=100$ barriers with randomly distributed strengths. Here$\Delta h_0 = 0$ denotes the case of regular barriers without disorder. All data in this figure are averaged over 100 disorder realizations of the barrier configuration. (a)(b)$h_{{\rm{min}}}=10 h_0$ ,$\Delta h_1 = 3 h_0$ ,$\Delta h_2 = 9 h_0$ ; (c), (d)$h_{{\rm{min}}}=40$ ,$\Delta h_1 = 5 h_0$ ,$\Delta h_2 = 35 h_0$ -
[1] Gamow G 1928 Z. Phys. 51 204
Google Scholar
[2] Gurney R W, Condon E U 1928 Nature 122 439
Google Scholar
[3] Talou P, Strottman D, Carjan N 1999 Phys. Rev. C 60 054318
Google Scholar
[4] Talou P, Carjan N, Negrevergne C, Strottman D 2000 Phys. Rev. C 62 014609
Google Scholar
[5] Balantekin A B, Takigawa N 1998 Rev. Mod. Phys. 70 77
Google Scholar
[6] Bhandari B S 1991 Phys. Rev. Lett. 66 1034
Google Scholar
[7] Vatasescu M, Dulieu O, Amiot C, Comparat D, Drag C, Kokoouline V, Masnou-Seeuws F, Pillet P 2000 Phys. Rev. A 61 044701
Google Scholar
[8] Keller J, Weiner J 1984 Phys. Rev. A 29 2943
Google Scholar
[9] Ricco B, Azbel M Y 1984 Phys. Rev. B 29 1970
Google Scholar
[10] Razavy M 2003 Quantum Theory of Tunneling (Singapore: World Scientific)
[11] Ueda M, Leggett A J 1998 Phys. Rev. Lett. 80 1576
Google Scholar
[12] Salasnich L, Parola A, Reatto L 2001 Phys. Rev. A 64 023601
Google Scholar
[13] Carr L D, Holland M J, Malomed B A 2005 J. Phys. B 38 3217
Google Scholar
[14] Schlagheck P, Paul T 2006 Phys. Rev. A 73 023619
Google Scholar
[15] Huhtamaki J A M, Mottonen M, Ankerhold J, Virtanen S M M 2007 Phys. Rev. A 76 033605
Google Scholar
[16] Zhao X, Alcala D A, McLain M A, Maeda K, Potnis S, Ramos R, Steinberg A M, Carr L D 2017 Phys. Rev. A 96 063601
Google Scholar
[17] Meyrath T P, Schreck F, Hanssen J L, Chuu C S, Raizen M G 2005 Phys. Rev. A 71 041604
Google Scholar
[18] Henderson K, Ryu C, MacCormick C, Boshier M G 2009 New J. Phys. 11 043030
Google Scholar
[19] Van Es J J P, Wicke P, Van Amerongen A H, Rétif C, Whitlock S, Van Druten N J 2010 J. Phys. B 43 155002
Google Scholar
[20] Gorlitz A, Vogels J M, Leanhardt A E, Raman C, Gustavson T L, Abo-Shaeer J R, Chikkatur A P, Gupta S, Inouye S, Rosenband T, Ketterle W 2001 Phys. Rev. Lett. 87 130402
Google Scholar
[21] Greiner M, Bloch I, Mandel O, Hansch T W, Esslinger T 2001 Phys. Rev. Lett. 87 160405
Google Scholar
[22] Schreck F, Khaykovich L, Corwin K L, Ferrari G, Bourdel T, Cubizolles J, Salomon C 2001 Phys. Rev. Lett. 87 080403
Google Scholar
[23] Stoferle T, Moritz H, Schori C, Kohl M, Esslinger T 2004 Phys. Rev. Lett. 92 130403
Google Scholar
[24] Serwane F, Zurn G, Lompe T, Ottenstein T B, Wenz A N, Jochim S 2011 Science 332 336
Google Scholar
[25] Pethick C J, Smith H 2008 Bose-Einstein Condensation in Dilute Gases (Cambridge: Cambridge University Press)
[26] Chin C, Grimm R, Julienne P, Tiesinga E 2010 Rev. Mod. Phys. 82 1225
Google Scholar
[27] Zurn G, Serwane F, Lompe T, Wenz A N, Ries M G, Bohn J E, Jochim S 2012 Phys. Rev. Lett. 108 075303
Google Scholar
[28] Zurn G, Wenz A N, Murmann S, Bergschneider A, Lompe T, Jochim S 2013 Phys. Rev. Lett. 111 175302
Google Scholar
[29] Winter R G 1961 Phys. Rev. 123 1503
Google Scholar
[30] Garcia-Calderón G, Mateos J L, Moshinsky M 1995 Phys. Rev. Lett. 74 337
Google Scholar
[31] Wilkinson S R, Bharucha C F, Fischer M C, Madison K W, Morrow P R, Niu Q, Sundaram B, Raizen M G 1997 Nature 387 575
Google Scholar
[32] Kelkar N G, Nowakowski M, Khemchandani K P 2004 Phys. Rev. C 70 024601
Google Scholar
[33] Crespi A, Pepe F V, Facchi P, Sciarrino F, Mataloni P, Nakazato H, Pascazio S, Osellame R 2019 Phys. Rev. Lett. 122 130401
Google Scholar
[34] Garcia-Calderón G, Mendoza-Luna L G 2011 Phys. Rev. A 84 032106
Google Scholar
[35] Dobrzyniecki J, Sowiński T 2018 Phys. Rev. A 98 013634
Google Scholar
[36] Dobrzyniecki J, Sowiński T 2019 Phys. Rev. A 99 063608
Google Scholar
[37] Lode A U J, Streltsov A I, Alon O E, Meye H D, Cederbaum L S 2009 J. Phys. B 42 044018
Google Scholar
[38] Lode A U J, Streltsov A I, Sakmann K, Alon O E, Cederbaum L S 2012 Proc. Natl. Acad. Sci. U.S.A. 109 13521
Google Scholar
[39] Gharashi S E, Blume D 2015 Phys. Rev. A 92 033629
Google Scholar
[40] Kim S, Brand J 2011 J. Phys. B 44 195301
Google Scholar
[41] Ishmukhamedov I S, Ishmukhamedov A S 2019 Physica E 109 24
Google Scholar
[42] Hunn S, Zimmermann K, Hiller M, Buchleitner A 2013 Phys. Rev. A 87 043626
Google Scholar
[43] Taniguchi T, Sawada S I 2011 Phys. Rev. E 83 026208
Google Scholar
[44] Maruyama T, Oishi T, Hagino K, Sagawa H 2012 Phys. Rev. C 86 044301
[45] Kościk P 2020 Phys. Rev. A 102 033308
Google Scholar
[46] Peshkin M, Volya A, Zelevinsky V 2014 Europhys. Lett. 107 40001
Google Scholar
[47] Qin P, Yin C, Chen S 2014 Phys. Rev. B 90 054303
[48] Zhang Z, Tong P, Gong J, Li B 2012 Phys. Rev. Lett. 108 070603
Google Scholar
下载:
计量
- 文章访问数: 3232
- PDF下载量: 79
- 被引次数: 0
