时间演化矩阵乘积算符方法及其在量子开放系统中的应用

陈若凡

doi:10.7498/aps.72.20222267

摘要

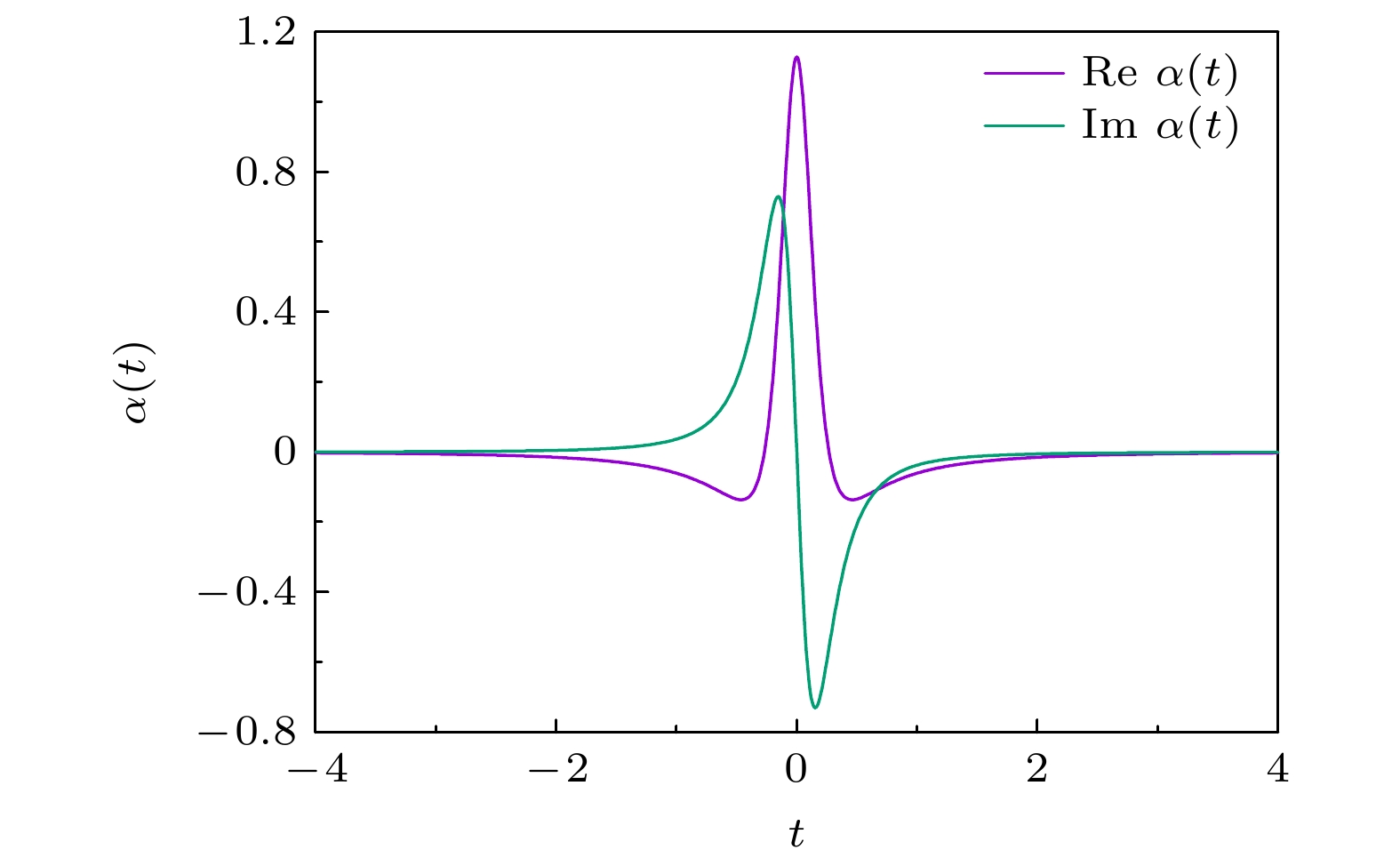
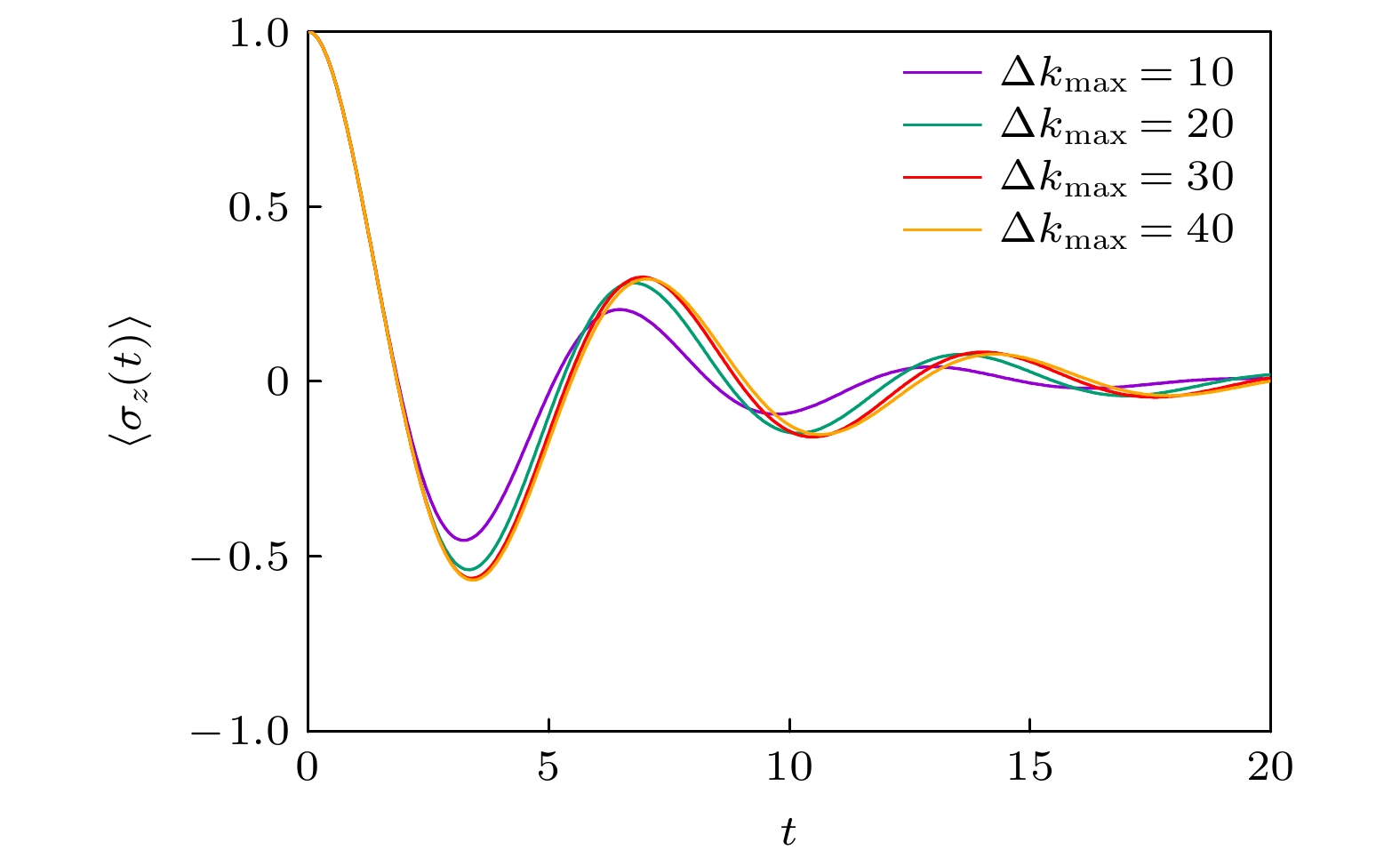
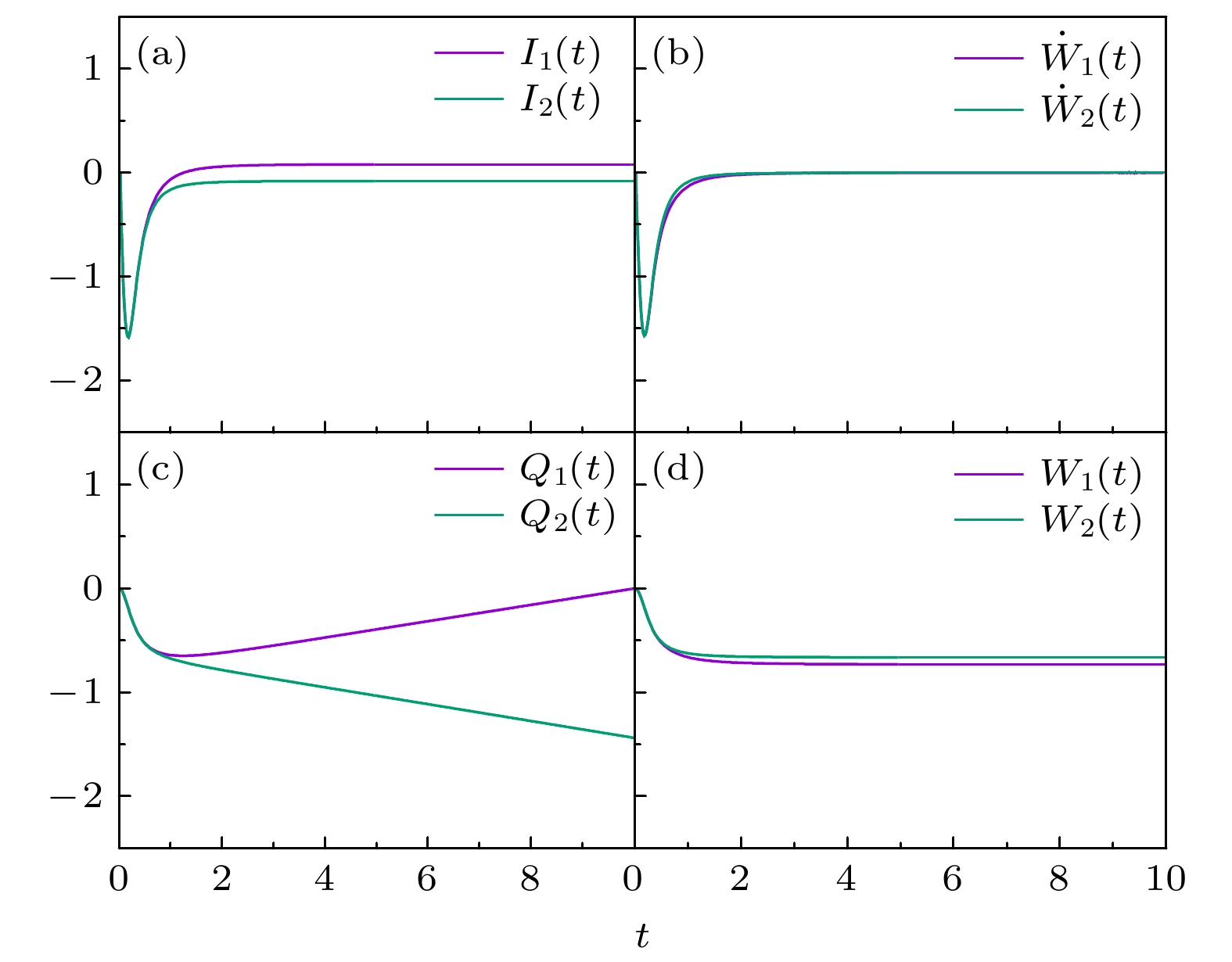
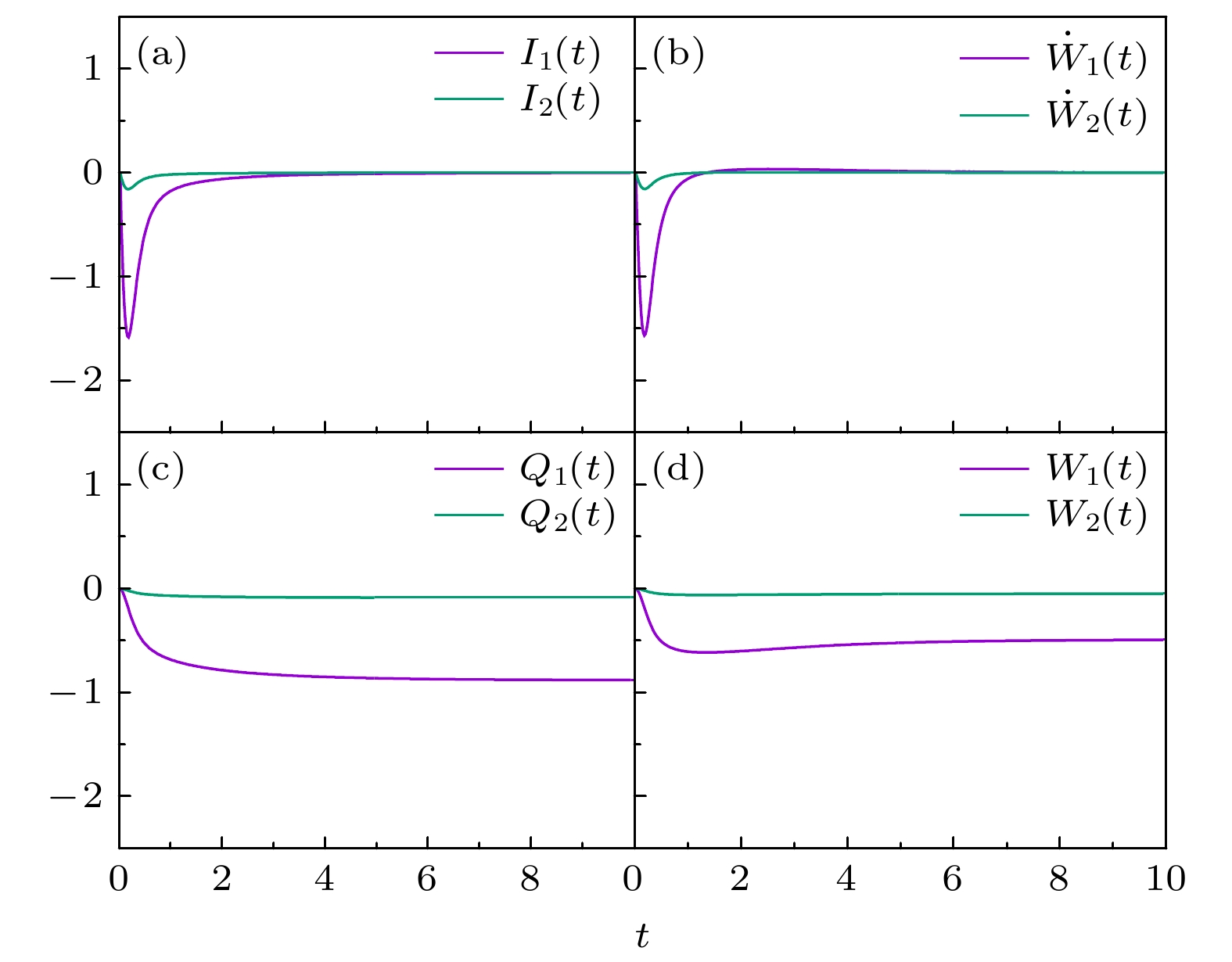
量子开放系统在量子科学的发展中占据着举足轻重的地位, 因此对其数值计算方法进行研究具有重要的意义. 对于量子开放系统, 在20世纪90年代发明的准绝热传播子路径积分方法是为数不多的精确的数值计算方法, 但其计算复杂度随着系统的大小和关联时间长度呈指数增长, 因此在实际计算中它所能计算的物理模型比较受限. 近年来, 张量网络的研究和应用有了长足的进展. 使用张量网络来表达该方法可以使其计算复杂度变成多项式增长, 极大地提高了计算效率. 由此发展出的新方法则被称为时间演化矩阵乘积算符方法, 是一种高效的、数值精确的、并且非马尔可夫的计算方法, 在量子开放系统的研究中有着广泛的应用前景. 本文首先综述了准绝热传播子路径积分方法, 接着介绍了矩阵乘积态的基本思路, 然后利用矩阵乘积态来表述准绝热传播子路径积分方法, 从而对时间演化矩阵乘积算符方法进行介绍; 最后综述了该方法在量子开放系统中的应用, 并以自旋-玻色子系统中的关联函数和热流计算为例对该方法进行了展示.

关键词:

Abstract

Open quantum systems play an important role in developing quantum sciences, and therefore the study of corresponding numerical method is of great significance. For the open quantum systems, the quasi-adiabatic propagator path integral invented in 1990s is one of the few numerically exact methods. However, its computational complexity scales exponentially with system size and correlation length, and therefore its application is limited in practical calculation. In recent years, the study and application of tensor network have made rapid progress. Representing the path integral by tensor network makes the computational complexity increase polynomially, thus greatly improving the computational efficiency. Such a new method is called time-evolving matrix product operator. At the very beginning, the reduced density matrix is represented as a matrix product state. Then the time evolution of the system can be achieved by iteratively applying matrix product operators to the matrix product state. The iterative process is amenable to the standard matrix product states compression algorithm, which keeps the computational cost on a polynomial scale. The time-evolving matrix product operator is an efficient, numerically exact and fully non-Markovian method, which has a broad application prospect in the study of quantum open systems. For instance, it is already used in the study of the thermalization, heat statistic, heat transfer and optimal control of the quantum open systems, and conversely it can be also used to investigate the effect of the system on the environment. In addition, the TEMPO method is naturally related to the process tensor, and can be used to calculate the correlation function of the system efficiently. In this article we review this method and its applications. We give a brief introduction of the path integral formalism of Caldeira-Leggett model. According to the path integral formalism, we demonstrate the usage of quasi-adiabatic propagator path integral method. we give the basic idea of matrix product states, and we show how to recast quasi-adiabatic propagator path integral method into time-evolving matrix product operators method by employing the concept of matrix product states and matrix product operators, and give a review of its applications. In addition, we use the calculation results of physical quantities, correlation functions and heat currents in the spin-boson model to illustrate the applications of the time-evolving matrix product operator method.

Keywords:

quantum open system /
quasi-adiabatic propagator path integral /
time-evolving matrix product operators /
tensor network /
non-Markovian dynamics

作者及机构信息

陈若凡

四川师范大学物理与电子工程学院, 计算科学中心, 成都　610068

通信作者: 陈若凡, physcrf@sicnu.edu.cn

基金项目: 国家自然科学基金(批准号: 12104328)资助的课题

Authors and contacts

Chen Ruo-Fan

Center for Computational Sciences, College of Physics and Electronic Engineering, Sichuan Normal University, Chengdu 610068, China

Corresponding author: Chen Ruo-Fan, physcrf@sicnu.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant No. 12104328)

文章全文 : translate this paragraph

参考文献

[1]	Weiss U 1993 Quantum Dissipative Systems (Singapore: World Scientific)
[2]	Breuer H P 2007 The Theory of Open Quantum Systems (New York: Oxford University Press)
[3]	Caldeira A O, Leggett A J 1981 Phys. Rev. Lett. 46 211 Google Scholar
[4]	Caldeira A O, Leggett A J 1983 Physica A 121 587 Google Scholar
[5]	Caldeira A, Leggett A 1983 Ann. Phys. 149 374 Google Scholar
[6]	Leggett A J, Chakravarty S, Dorsey A T, Fisher M P A, Garg A, Zwerger W 1987 Rev. Mod. Phys. 59 1 Google Scholar
[7]	Makarov D E, Makri N 1994 Chem. Phys. Lett. 221 482 Google Scholar
[8]	Makri N 1995 J. Math. Phys. 36 2430 Google Scholar
[9]	Makarov D E, Makri N 1995 Phys. Rev. B 52 R2257
[10]	Makarov D E, Makri N 1995 Phys. Rev. E 52 5863
[11]	Golosov A A, Friesner R A, Pechukas P 1999 J. Chem. Phys. 110 138 Google Scholar
[12]	Shao J, Makri N 2002 J. Chem. Phys. 116 507 Google Scholar
[13]	Dong K, Makri N 2004 Chem. Phys. 296 273 Google Scholar
[14]	Dong K, Makri N 2004 Phys. Rev. A 70 042101 Google Scholar
[15]	Thorwart M, Eckel J, Mucciolo E R 2005 Phys. Rev. B 72 235320 Google Scholar
[16]	Nalbach P, Thorwart M 2009 Phys. Rev. Lett. 103 220401 Google Scholar
[17]	Arceci L, Barbarino S, Fazio R, Santoro G E 2017 Phys. Rev. B 96 054301 Google Scholar
[18]	Schollwöck U 2011 Ann. Phys. 326 96 Google Scholar
[19]	Orús R 2014 Ann. Phys. 349 117 Google Scholar
[20]	Strathearn A, Kirton P, Kilda D, Keeling J, Lovett B W 2018 Nat. Commun. 9 3322 Google Scholar
[21]	Keldysh L V 1965 Sov. Phys. JETP 20 1018
[22]	Lifshitz E M, Pitaevskii L P 1981 Course of Theoretical Physics Volume 10: Physical Kinetics (Elsevier)
[23]	Kamenev A, Levchenko A 2009 Adv. Phys. 58 197 Google Scholar
[24]	Wang J S, Agarwalla B K, Li H, Thingna J 2014 Front. Phys. 9 673
[25]	Chen R 2023 New J. Phys. 25 033035 Google Scholar
[26]	Feynman R P, Vernon F L 1963 Ann. Phys. 24 118 Google Scholar
[27]	Grabert H, Schramm P, Ingold G L 1988 Phys. Rep. 168 115 Google Scholar
[28]	Negele J W, Orland H 1998 Quantum Many-Particle Systems (Colorado: Westview Press)
[29]	Trotter H F 1959 Proc. Amer. Math. Soc. 10 545 Google Scholar
[30]	Suzuki M 1976 Commun. Math. Phys. 51 183 Google Scholar
[31]	Makarov D E, Makri N 1993 Phys. Rev. A 48 3626 Google Scholar
[32]	Makri N, Makarov D E 1995 J. Chem. Phys. 102 4600 Google Scholar
[33]	Dattani N S, Pollock F A, Wilkins D M 2012 Quantum Phys. Lett. 1 35
[34]	Chen R, Xu X 2023 Eur. Phys. J. Plus 138 194 Google Scholar
[35]	Verstraete F, García-Ripoll J J, Cirac J I 2004 Phys. Rev. Lett. 93 207204 Google Scholar
[36]	Zwolak M, Vidal G 2004 Phys. Rev. Lett. 93 207205 Google Scholar
[37]	Barthel T, Schollwöck U, White S R 2009 Phys. Rev. B 79 245101 Google Scholar
[38]	Schollwöck U 2005 Rev. Mod. Phys. 77 259 Google Scholar
[39]	Levin M, Nave C P 2007 Phys. Rev. Lett. 99 120601 Google Scholar
[40]	Evenbly G, Vidal G 2015 Phys. Rev. Lett. 115 180405 Google Scholar
[41]	Ran S J, Li W, Xi B, Zhang Z, Su G 2012 Phys. Rev. B 86 134429 Google Scholar
[42]	Czarnik P, Cincio L, Dziarmaga J 2012 Phys. Rev. B 86 245101 Google Scholar
[43]	Kshetrimayum A, Weimer H, Orús R 2017 Nat. Commun. 8 1291 Google Scholar
[44]	Fishman M T, White S R 2015 Phys. Rev. B 92 075132 Google Scholar
[45]	Dorda A, Ganahl M, Evertz H G, Linden W, Arrigoni E 2015 Phys. Rev. B 92 125145 Google Scholar
[46]	Ganahl M, Aichhorn M, Evertz H G, Thunström P, Held K, Verstraete F 2015 Phys. Rev. B 92 155132 Google Scholar
[47]	Wolf F A, Go A, McCulloch I P, Millis A J, Schollwöck U 2015 Phys. Rev. X 5 041032
[48]	Linden N O, Zingl M, Hubig C, Parcollet O, Schollwöck U 2020 Phys. Rev. B 101 041101 Google Scholar
[49]	Kohn L, Santoro G E 2021 Phys. Rev. B 104 014303
[50]	Jørgensen M R, Pollock F A 2019 Phys. Rev. Lett. 123 240602 Google Scholar
[51]	Carmichael H J 1999 Statistical Methods in Quantum Optics 1: Master Equations and Fokker-Planck Equations (Berlin: Springer-Verlag)
[52]	Gardiner C W, Zoller P 2004 Quantum Noise: A Handbook of Markovian and Non-Markovian Quantum Stochastic Methods with Applications to Quantum Optics (Berlin: Springer-Verlag)
[53]	Jørgensen M R, Pollock F A 2020 Phys. Rev. A 102 052206 Google Scholar
[54]	Cerrillo J, Cao J 2014 Phys. Rev. Lett. 112 110401 Google Scholar
[55]	Popovic M, Mitchison M T, Strathearn A, Lovett B W, Goold J, Eastham P R 2021 PRX Quantum 2 020338 Google Scholar
[56]	Fux G E, Butler E P, Eastham P R, Lovett B W, Keeling J 2021 Phys. Rev. Lett. 126 200401 Google Scholar
[57]	Fux G E, Kilda D, Lovett B W, Keeling J 2022 10.48550/arXiv.2201.05529 [quant-ph]
[58]	Gribben D, Strathearn A, Fux G E, Kirton P, Lovett B W 2021 Quantum 6 847
[59]	Gribben D, Rouse D M, Iles-Smith J, Strathearn A, Maguire H, Kirton P, Nazir A, Gauger E M, Lovett B W 2022 PRX Quantum 3 010321 Google Scholar
[60]	Ye E, Chan G K L 2021 J. Chem. Phys. 155 044104 Google Scholar
[61]	Chiu Y F, Strathearn A, Keeling J 2022 Phys. Rev. A 106 012204 Google Scholar
[62]	Otterpohl F, Nalbach P, Thorwart M 2022 Phys. Rev. Lett. 129 120406 Google Scholar
[63]	Bose A 2022 Phys. Rev. B 105 024309
[64]	Florens S, Venturelli D, Narayanan R (edited by Chandra A K, Das A, Chakrabarti B K) 2010 Quantum Quenching, Annealing and Computation (Berlin, Heidelberg: Springer) p145
[65]	Hur K L (edited by Carr L D) 2010 Understanding Quantum Phase Transitions (New York: CRC Press) p217
[66]	Löfstedt R, Coppersmith S N 1994 Phys. Rev. Lett. 72 1947 Google Scholar
[67]	Gammaitoni L, Hänggi P, Jung P, Marchesoni F 1998 Rev. Mod. Phys. 70 223 Google Scholar
[68]	Grifoni M, Hänggi P 1998 Phys. Rep. 304 229 Google Scholar
[69]	Meir Y, Wingreen N S 1992 Phys. Rev. Lett. 68 2512 Google Scholar
[70]	Brandes T, Aguado R, Platero G 2004 Phys. Rev. B 69 205326 Google Scholar
[71]	Wang J S 2007 Phys. Rev. Lett. 99 160601 Google Scholar
[72]	Segal D 2013 Phys. Rev. B 87 195436 Google Scholar
[73]	Lü J T, Christensen R B, Wang J S, Hedegård P, Brandbyge M 2015 Phys. Rev. Lett. 114 096801 Google Scholar
[74]	Ludovico M F, Lim J S, Moskalets M, Arrachea L, Sánchez D 2014 Phys. Rev. B 89 161306 Google Scholar
[75]	Ludovico M, Arrachea L, Moskalets M, Sánchez D 2016 Entropy 18 419 Google Scholar
[76]	Ludovico M F, Moskalets M, Sánchez D, Arrachea L 2016 Phys. Rev. B 94 035436 Google Scholar
[77]	Ludovico M F, Arrachea L, Moskalets M, Sánchez D 2018 Phys. Rev. B 97 041416 Google Scholar

施引文献

图 1 Keldysh回路$ \cal{C} $, 上部为正向分支, 下部为反向分支^[25]

Fig. 1. Keldysh Contour $ \cal{C} $, the upper is forward branch and the lower is backward branch ^[25].

下载: 全尺寸图片幻灯片

图 2 递归关系的示意图 (a)递归公式(40); (b)从初始条件 $ F^{s_0} $开始演化N = 4步的递推步骤

Fig. 2. An illustration of the recursive relation: (a) Recursive relation (40); (b) the iterative progress with N = 4 steps evolution from initial condition $ F^{s_0} $.

下载: 全尺寸图片幻灯片

图 3 自相关函数$ \alpha(t) $的一种典型形状^[34]

Fig. 3. A typical shape of autocorrelation function $ \alpha(t) $^[34].

下载: 全尺寸图片幻灯片

图 4 截断之后的递归关系(其中灰色方格代表(42)式中的求和) (a)递归公式(42); (b)从初始条件 $ F^{s_0} $开始演化到 $ N=4 $步时的递推步骤

Fig. 4. An illustration of recursive relation after truncation, here the gray square stands for the summation in Eq. (42): (a) Recursive relation (42); (b) the iterative progress of $ N=4 $ steps evolution with initial condition $ F^{s_0} $.

下载: 全尺寸图片幻灯片

图 5 TEMPO算法中的一些张量网络图示 (a) $ F $张量的矩阵乘积态表示; (b) $ B $张量的矩阵乘积算符表示; (c)递归关系(40)的张量网络表示

Fig. 5. An illustration of some tensor networks in TEMPO: (a) The matrix product states representation of $ F $ tensor; (b) the matrix product operators representation of $ B $ tensor; (c) the tensor network representation of recursive relation (40).

下载: 全尺寸图片幻灯片

图 6 $ N=4 $步演化的张量网络

Fig. 6. Tensor network of $ N=4 $ steps evolution.

下载: 全尺寸图片幻灯片

图 7 $ \left\langle {{{\hat \sigma }_z}(t)} \right\rangle $在不同$ \Delta k_{\rm{max}} $下的演化

Fig. 7. Dynamics of $ \left\langle {{{\hat \sigma }_z}(t)} \right\rangle $ with respect to different $ \Delta k_{\rm{max}} $

下载: 全尺寸图片幻灯片

图 8 $ \left\langle {{{\hat \sigma }_z}(t)} \right\rangle $在不同耦合强度$ \lambda $下的演化

Fig. 8. Dynamics of $ \left\langle {{{\hat \sigma }_z}(t)} \right\rangle $ with respect to different coupling strength $ \lambda $.

下载: 全尺寸图片幻灯片

图 9 在不同$ \Delta k_{\rm{max}} $和$ t_0 $下的关联函数$ C(t_0, t_0+t) $^[34]

Fig. 9. Correlation function $ C(t_0, t_0+t) $ with respect to different $ \Delta k_{\rm{max}} $ and $ t_0 $^[34].

下载: 全尺寸图片幻灯片

图 10 系统和浴在$ T_1 $远大于$ T_2 $时的演化过程^[25]

Fig. 10. Evolution of system and bath when $ T_1 $ is much larger than $ T_2 $^[25].

下载: 全尺寸图片幻灯片

图 11 系统和浴在$ T_1=T_2 $但$ \lambda_1 $远大于$ \lambda_2 $时的演化过程^[25]

Fig. 11. Evolution of system and bath when $ T_1=T_2 $ but $ \lambda_1 $ is larger than $ \lambda_2 $^[25].

下载: 全尺寸图片幻灯片

map

[1]	Weiss U 1993 Quantum Dissipative Systems (Singapore: World Scientific)
[2]	Breuer H P 2007 The Theory of Open Quantum Systems (New York: Oxford University Press)
[3]	Caldeira A O, Leggett A J 1981 Phys. Rev. Lett. 46 211 Google Scholar
[4]	Caldeira A O, Leggett A J 1983 Physica A 121 587 Google Scholar
[5]	Caldeira A, Leggett A 1983 Ann. Phys. 149 374 Google Scholar
[6]	Leggett A J, Chakravarty S, Dorsey A T, Fisher M P A, Garg A, Zwerger W 1987 Rev. Mod. Phys. 59 1 Google Scholar
[7]	Makarov D E, Makri N 1994 Chem. Phys. Lett. 221 482 Google Scholar
[8]	Makri N 1995 J. Math. Phys. 36 2430 Google Scholar
[9]	Makarov D E, Makri N 1995 Phys. Rev. B 52 R2257
[10]	Makarov D E, Makri N 1995 Phys. Rev. E 52 5863
[11]	Golosov A A, Friesner R A, Pechukas P 1999 J. Chem. Phys. 110 138 Google Scholar
[12]	Shao J, Makri N 2002 J. Chem. Phys. 116 507 Google Scholar
[13]	Dong K, Makri N 2004 Chem. Phys. 296 273 Google Scholar
[14]	Dong K, Makri N 2004 Phys. Rev. A 70 042101 Google Scholar
[15]	Thorwart M, Eckel J, Mucciolo E R 2005 Phys. Rev. B 72 235320 Google Scholar
[16]	Nalbach P, Thorwart M 2009 Phys. Rev. Lett. 103 220401 Google Scholar
[17]	Arceci L, Barbarino S, Fazio R, Santoro G E 2017 Phys. Rev. B 96 054301 Google Scholar
[18]	Schollwöck U 2011 Ann. Phys. 326 96 Google Scholar
[19]	Orús R 2014 Ann. Phys. 349 117 Google Scholar
[20]	Strathearn A, Kirton P, Kilda D, Keeling J, Lovett B W 2018 Nat. Commun. 9 3322 Google Scholar
[21]	Keldysh L V 1965 Sov. Phys. JETP 20 1018
[22]	Lifshitz E M, Pitaevskii L P 1981 Course of Theoretical Physics Volume 10: Physical Kinetics (Elsevier)
[23]	Kamenev A, Levchenko A 2009 Adv. Phys. 58 197 Google Scholar
[24]	Wang J S, Agarwalla B K, Li H, Thingna J 2014 Front. Phys. 9 673
[25]	Chen R 2023 New J. Phys. 25 033035 Google Scholar
[26]	Feynman R P, Vernon F L 1963 Ann. Phys. 24 118 Google Scholar
[27]	Grabert H, Schramm P, Ingold G L 1988 Phys. Rep. 168 115 Google Scholar
[28]	Negele J W, Orland H 1998 Quantum Many-Particle Systems (Colorado: Westview Press)
[29]	Trotter H F 1959 Proc. Amer. Math. Soc. 10 545 Google Scholar
[30]	Suzuki M 1976 Commun. Math. Phys. 51 183 Google Scholar
[31]	Makarov D E, Makri N 1993 Phys. Rev. A 48 3626 Google Scholar
[32]	Makri N, Makarov D E 1995 J. Chem. Phys. 102 4600 Google Scholar
[33]	Dattani N S, Pollock F A, Wilkins D M 2012 Quantum Phys. Lett. 1 35
[34]	Chen R, Xu X 2023 Eur. Phys. J. Plus 138 194 Google Scholar
[35]	Verstraete F, García-Ripoll J J, Cirac J I 2004 Phys. Rev. Lett. 93 207204 Google Scholar
[36]	Zwolak M, Vidal G 2004 Phys. Rev. Lett. 93 207205 Google Scholar
[37]	Barthel T, Schollwöck U, White S R 2009 Phys. Rev. B 79 245101 Google Scholar
[38]	Schollwöck U 2005 Rev. Mod. Phys. 77 259 Google Scholar
[39]	Levin M, Nave C P 2007 Phys. Rev. Lett. 99 120601 Google Scholar
[40]	Evenbly G, Vidal G 2015 Phys. Rev. Lett. 115 180405 Google Scholar
[41]	Ran S J, Li W, Xi B, Zhang Z, Su G 2012 Phys. Rev. B 86 134429 Google Scholar
[42]	Czarnik P, Cincio L, Dziarmaga J 2012 Phys. Rev. B 86 245101 Google Scholar
[43]	Kshetrimayum A, Weimer H, Orús R 2017 Nat. Commun. 8 1291 Google Scholar
[44]	Fishman M T, White S R 2015 Phys. Rev. B 92 075132 Google Scholar
[45]	Dorda A, Ganahl M, Evertz H G, Linden W, Arrigoni E 2015 Phys. Rev. B 92 125145 Google Scholar
[46]	Ganahl M, Aichhorn M, Evertz H G, Thunström P, Held K, Verstraete F 2015 Phys. Rev. B 92 155132 Google Scholar
[47]	Wolf F A, Go A, McCulloch I P, Millis A J, Schollwöck U 2015 Phys. Rev. X 5 041032
[48]	Linden N O, Zingl M, Hubig C, Parcollet O, Schollwöck U 2020 Phys. Rev. B 101 041101 Google Scholar
[49]	Kohn L, Santoro G E 2021 Phys. Rev. B 104 014303
[50]	Jørgensen M R, Pollock F A 2019 Phys. Rev. Lett. 123 240602 Google Scholar
[51]	Carmichael H J 1999 Statistical Methods in Quantum Optics 1: Master Equations and Fokker-Planck Equations (Berlin: Springer-Verlag)
[52]	Gardiner C W, Zoller P 2004 Quantum Noise: A Handbook of Markovian and Non-Markovian Quantum Stochastic Methods with Applications to Quantum Optics (Berlin: Springer-Verlag)
[53]	Jørgensen M R, Pollock F A 2020 Phys. Rev. A 102 052206 Google Scholar
[54]	Cerrillo J, Cao J 2014 Phys. Rev. Lett. 112 110401 Google Scholar
[55]	Popovic M, Mitchison M T, Strathearn A, Lovett B W, Goold J, Eastham P R 2021 PRX Quantum 2 020338 Google Scholar
[56]	Fux G E, Butler E P, Eastham P R, Lovett B W, Keeling J 2021 Phys. Rev. Lett. 126 200401 Google Scholar
[57]	Fux G E, Kilda D, Lovett B W, Keeling J 2022 10.48550/arXiv.2201.05529 [quant-ph]
[58]	Gribben D, Strathearn A, Fux G E, Kirton P, Lovett B W 2021 Quantum 6 847
[59]	Gribben D, Rouse D M, Iles-Smith J, Strathearn A, Maguire H, Kirton P, Nazir A, Gauger E M, Lovett B W 2022 PRX Quantum 3 010321 Google Scholar
[60]	Ye E, Chan G K L 2021 J. Chem. Phys. 155 044104 Google Scholar
[61]	Chiu Y F, Strathearn A, Keeling J 2022 Phys. Rev. A 106 012204 Google Scholar
[62]	Otterpohl F, Nalbach P, Thorwart M 2022 Phys. Rev. Lett. 129 120406 Google Scholar
[63]	Bose A 2022 Phys. Rev. B 105 024309
[64]	Florens S, Venturelli D, Narayanan R (edited by Chandra A K, Das A, Chakrabarti B K) 2010 Quantum Quenching, Annealing and Computation (Berlin, Heidelberg: Springer) p145
[65]	Hur K L (edited by Carr L D) 2010 Understanding Quantum Phase Transitions (New York: CRC Press) p217
[66]	Löfstedt R, Coppersmith S N 1994 Phys. Rev. Lett. 72 1947 Google Scholar
[67]	Gammaitoni L, Hänggi P, Jung P, Marchesoni F 1998 Rev. Mod. Phys. 70 223 Google Scholar
[68]	Grifoni M, Hänggi P 1998 Phys. Rep. 304 229 Google Scholar
[69]	Meir Y, Wingreen N S 1992 Phys. Rev. Lett. 68 2512 Google Scholar
[70]	Brandes T, Aguado R, Platero G 2004 Phys. Rev. B 69 205326 Google Scholar
[71]	Wang J S 2007 Phys. Rev. Lett. 99 160601 Google Scholar
[72]	Segal D 2013 Phys. Rev. B 87 195436 Google Scholar
[73]	Lü J T, Christensen R B, Wang J S, Hedegård P, Brandbyge M 2015 Phys. Rev. Lett. 114 096801 Google Scholar
[74]	Ludovico M F, Lim J S, Moskalets M, Arrachea L, Sánchez D 2014 Phys. Rev. B 89 161306 Google Scholar
[75]	Ludovico M, Arrachea L, Moskalets M, Sánchez D 2016 Entropy 18 419 Google Scholar
[76]	Ludovico M F, Moskalets M, Sánchez D, Arrachea L 2016 Phys. Rev. B 94 035436 Google Scholar
[77]	Ludovico M F, Arrachea L, Moskalets M, Sánchez D 2018 Phys. Rev. B 97 041416 Google Scholar

[1]	蒋世民, 贾欣燕, 樊代和. 非马尔科夫环境中Werner态的量子非局域关联检验研究. , 2024, 73(16): 160301. doi: 10.7498/aps.73.20240450
[2]	杜啸颖, 俞振华. 分形格点中伊辛模型的临界行为. , 2023, 72(8): 080503. doi: 10.7498/aps.72.20222432
[3]	宋峰峰, 张广铭. 拓扑激发驱动的热力学相变及其张量网络研究方法. , 2023, 72(23): 230301. doi: 10.7498/aps.72.20231152
[4]	张茂芳, 游慧敏, 尹相国, 张云波. 半开放系统中的粒子逃逸问题. , 2022, 71(16): 167302. doi: 10.7498/aps.71.20220450
[5]	贺志, 蒋登魁, 李艳. 一种与开放系统初态无关的非马尔科夫度量. , 2022, 71(21): 210303. doi: 10.7498/aps.71.20221053
[6]	张金峰, 阿拉帕提·阿不力米提, 杨帆, 艾克拜尔·阿木提江, 唐诗生, 艾合买提·阿不力孜. 不同外加磁场中Kaplan-Shekhtman-Entin-Wohlman-Aharony相互作用对量子失协非马尔科夫演化的影响. , 2021, 70(22): 223401. doi: 10.7498/aps.70.20211277
[7]	陈宇. 耗散响应理论及其在开放系统中的应用. , 2021, 70(23): 230306. doi: 10.7498/aps.70.20211687
[8]	张科, 李兰兰, 任刚, 杜建明, 范洪义. 量子扩散通道中Wigner算符的演化规律. , 2020, 69(9): 090301. doi: 10.7498/aps.69.20200106
[9]	王秀娟, 李生好. 基于U(1)对称的无限矩阵乘积态张量网络算法提取Luttinger液体参数K . , 2019, 68(16): 160201. doi: 10.7498/aps.68.20190379
[10]	李晓克, 冯伟. 非绝热分子动力学的量子路径模拟. , 2017, 66(15): 153101. doi: 10.7498/aps.66.153101
[11]	梁潇, 钱志鸿, 田洪亮, 王雪. 基于马尔可夫决策模型的异构无线网络切换选择算法. , 2016, 65(23): 236402. doi: 10.7498/aps.65.236402
[12]	贺志, 李莉, 姚春梅, 李艳. 利用量子相干性判定开放二能级系统中非马尔可夫性. , 2015, 64(14): 140302. doi: 10.7498/aps.64.140302
[13]	游波, 岑理相. 非马尔科夫耗散系统长时演化下的极限环振荡现象. , 2015, 64(21): 210302. doi: 10.7498/aps.64.210302
[14]	谢文贤, 许鹏飞, 蔡力, 李东平. 随机双指数记忆耗散系统的非马尔可夫扩散. , 2013, 62(8): 080503. doi: 10.7498/aps.62.080503
[15]	周军, 袁好, 宋军. 相位扩散通道中密度算符相关态的时间演化. , 2012, 61(3): 030302. doi: 10.7498/aps.61.030302
[16]	钱江海, 韩定定, 马余刚. 开放式复杂航空网络系统的动力学演化. , 2011, 60(9): 098901. doi: 10.7498/aps.60.098901
[17]	郑力明, 刘颂豪, 王发强. 非马尔可夫环境下原子的几何相位演化. , 2009, 58(4): 2430-2434. doi: 10.7498/aps.58.2430
[18]	樊锡君, 田淑芬, 李健, 刘杰, 白成杰. 开放的无粒子数反转激光系统中原子响应的时间演化和光放大机制. , 2000, 49(9): 1719-1725. doi: 10.7498/aps.49.1719
[19]	刘堂昆, 王继锁, 柳晓军, 詹明生. 非共振相互作用系统中密度算符间距的演化. , 1999, 48(11): 2051-2059. doi: 10.7498/aps.48.2051
[20]	林东海, 吴钦义. 描述自旋体系密度算符演化的新方法. , 1993, 42(6): 893-904. doi: 10.7498/aps.42.893

计量

文章访问数: 3680
PDF下载量: 148
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

时间演化矩阵乘积算符方法及其在量子开放系统中的应用