-
谱域光学相干层析成像(spectral-domain optical coherence tomography, SD-OCT)系统中普遍存在波数域的非线性采样问题. 为实现常规快速傅里叶变换算法下离散界面的精确定位与OCT图像的高质量重建, 需要解决光谱仪中离散采样点绝对波数的精确标定问题. 本文提出了一种基于精确光程差下特征谱线与约束拟合相位的绝对波数标定方法, 在谱域OCT系统的样品臂中, 使用具有精确厚度差异的金属量规, 获得特征谱线对应的绝对相位值, 进一步精确求解出特征谱线对应的相位包裹次数, 克服了常规干涉光谱相位方法中普遍存在的2π 歧义, 结合窗口约束条件下高信噪比区域的拟合相位, 实现光谱仪采样点绝对波数的精确标定. 通过全面比较本文方法与传统插值重采样方法在离散界面定位、轴向分辨率以及图像重建质量等方面的差异, 验证了本方法的显著优势.
-
关键词:
- 谱域光学相干层析成像 /
- 光谱相位 /
- 绝对波数标定 /
- 窗约束拟合
Spectral-domain optical coherence tomography (SD-OCT) systems generally have nonlinear sampling problems in wavenumber domain. In order to realize the precise positioning of the discrete interfaces and the high-quality reconstruction of OCT images under conventional fast Fourier transform, it is necessary to solve the precise calibration problem of the absolute wavenumber of the discrete sampling points in the spectrometer. In this work, an absolute wavenumber calibration method is proposed based on the absolute phase of the characteristic spectral line and the constraint polynomial fitting phase under precise optical path difference. In the sample arm of the SD-OCT system, the metal gauges with precise thickness difference are used to obtain the absolute phase value of the characteristic spectral line, and the phase wrapping times corresponding to the characteristic spectral line are further accurately solved. Thus, this method overcomes the 2π ambiguity of spectral phase in conventional interferometric phase methods. At the same time, combined with the polynomial fitting phase of the high signal-to-noise ratio region under window constraint, the accurate calibration of the absolute wavenumber of each sampling point is realized. Finally, comprehensive comparison between the proposed method and the traditional resampling method in terms of discrete interface positioning, axial resolution and image reconstruction quality verifies the significant advantages of this method.-
Keywords:
- spectral-domain optical coherence tomography /
- spectral phase /
- absolute wavenumber calibration /
- window-constrained polynomial fitting.
[1] Huang D, Swanson E A, Lin C P, Schuman J S, Stinson W G, Chang W, Hee M R, Flotte T, Gregory K, Puliafito C A, Fujimoto J G 1991 Science 254 1178
Google Scholar
[2] Tomlins P H, Wang R K 2005 J. Phys. D: Appl. Phys. 38 2519
Google Scholar
[3] 赵晨, 陈志彦, 丁志华, 李鹏, 沈毅, 倪秧 2014 63 194201
Google Scholar
Zhao C, Chen Z Y, Ding Z H, Li P, Shen Y, Ni Y 2014 Acta Phys. Sin. 63 194201
Google Scholar
[4] Kumar M, Islam M N, Terry F L, Aleksoff C C, Davidson D 2010 Opt. Express 18 22471
Google Scholar
[5] Heise B, Schausberger S E, Häuser S, Plank B, Salaberger D, Leiss-Holzinger E, Stifter D 2012 Opt. Fiber. Technol. 18 403
Google Scholar
[6] Wiesauer K, Pircher M, Götzinger E, Bauer S, Engelke R, Ahrens G, Grützner G, Hitzenberger C K, Stifter D 2005 Opt. Express 13 1015
Google Scholar
[7] Endo T, Yasuno Y, Makita S, Itoh M, Yatagai T 2005 Opt. Express 13 695
Google Scholar
[8] Leitgeb R, Hitzenberger C K, Fercher A F 2003 Opt. Express 11 889
Google Scholar
[9] Choma M A, Sarunic M V, Yang C, Izatt J A 2003 Opt. Express 11 2183
Google Scholar
[10] de Boer J F, Cense B, Park B H, Pierce M C, Tearney G J, Bouma B E 2003 Opt. Lett. 28 2067
Google Scholar
[11] Dorrer C, Belabas N, Likforman J P, Joffre M 2000 J. Opt. Soc. Am. B 17 1795
Google Scholar
[12] Uribe-Patarroyo N, Kassani S H, Villiger M, Bouma B E 2018 Opt. Express 26 9081
Google Scholar
[13] Hu Z, Rollins A M 2007 Opt. Lett. 32 3525
Google Scholar
[14] 吴彤, 孙帅帅, 王绪晖, 王吉明, 赫崇君, 顾晓蓉, 刘友文 2018 67 104208
Google Scholar
Wu T, Sun S S, Wang X H, Wang J M, He C J, Gu X R, Liu Y W 2018 Acta Phys. Sin. 67 104208
Google Scholar
[15] Hyle Park B, Pierce M C, Cense B, Yun S H, Mujat M, Tearney G J, Bouma B E, Boer J F D 2005 Opt. Express 13 3931
Google Scholar
[16] Perret E, Balmer T E, Heuberger M 2010 Appl. Spectrosc. 64 1139
Google Scholar
[17] Eom T J, Ahn Y C, Kim C S, Chen Z P 2011 J. Biomed. Opt. 16 1
Google Scholar
[18] Wang K, Ding Z 2008 Chin. Opt. Lett. 6 902
Google Scholar
[19] Wu X, Ye X, Yu D, Yu J, Huang Y, Tan H, Qin J, An L 2020 OSA Continuum. 3 2156
Google Scholar
[20] Ikeda T, Popescu G, Dasari R R, Feld M S 2005 Opt. Lett. 30 1165
Google Scholar
[21] Meissner M 2012 Acta Phys. Pol. A 121 164
Google Scholar
[22] Yan Y, Ding Z, Shen Y, Chen Z, Zhao C, Ni Y 2013 Opt. Express 21 25734
Google Scholar
[23] Han T, Qiu J, Wang D, Meng J, Liu Z, Ding Z 2020 J. Innov. Opt. Heal. Sci. 14 2140008
Google Scholar
[24] 唐弢, 赵晨, 陈志彦, 李鹏, 丁志华 2015 64 174201
Google Scholar
Tang T, Zhao C, Chen Z Y, Li P, Ding Z H 2015 Acta Phys. Sin. 64 174201
Google Scholar
[25] Wang C, You Y J, Ai S, Zhang W, Liao W, Zhang X, Hsieh J, Zhang N, Tang B, Pan C L, Xue P 2019 J. Innov. Opt. Heal. Sci. 12 1950009
Google Scholar
-
图 1 用于光谱仪标定实验的谱域OCT系统
Fig. 1. Schematic diagram of the spectral domain optical coherence tomography system for spectrometer calibration.
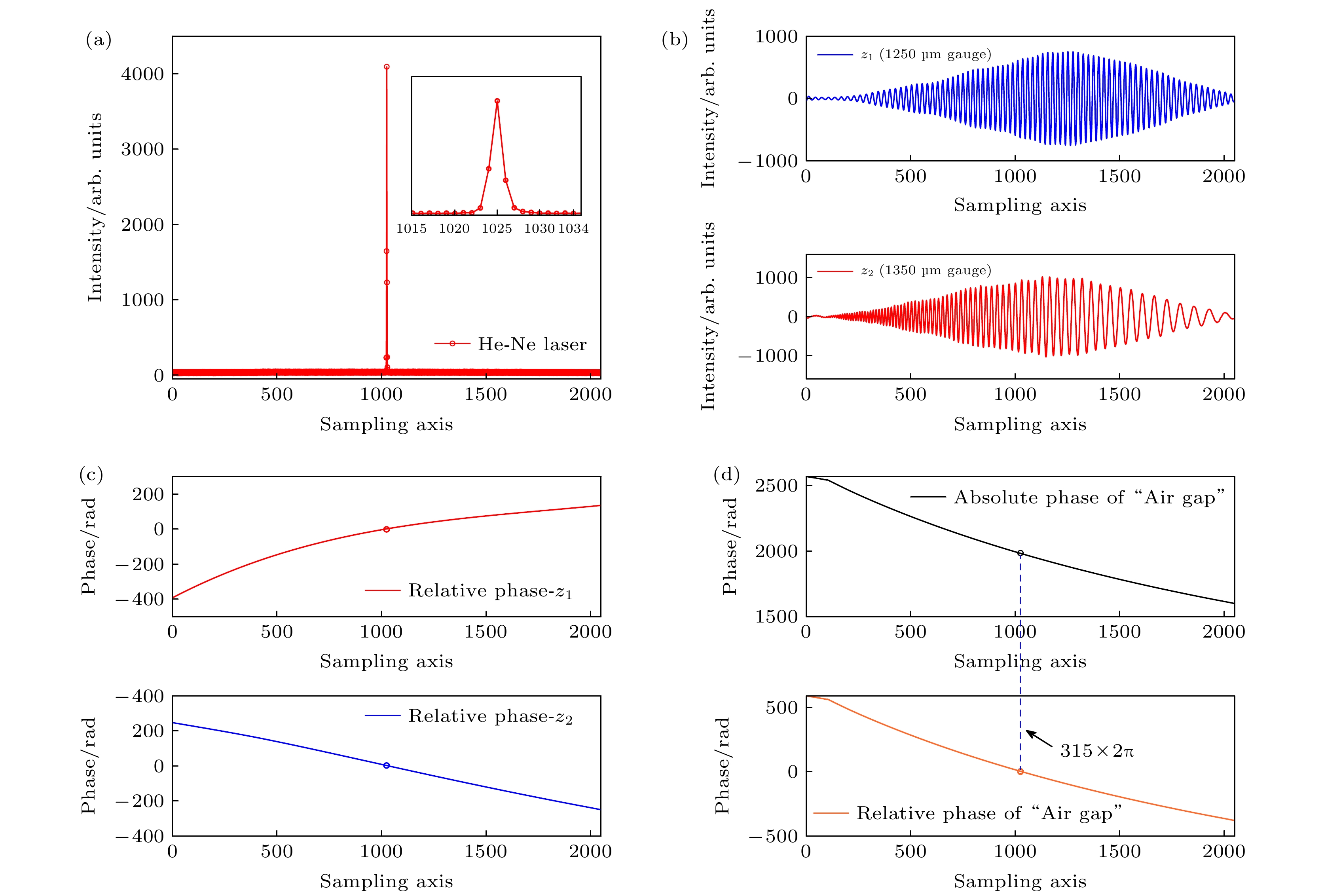
图 2 He-Ne特征谱线恢复“空气隙”绝对相位的过程 (a) He-Ne特征谱线标定光谱; (b)两组金属量规的互相关干涉信号; (c)连续化处理后的相对相位分布; (d)恢复的“空气隙”绝对相位分布
Fig. 2. Process of recovering the absolute phase of “air gap” from He-Ne characteristic spectral line: (a) Spectrum of He-Ne characteristic line calibration; (b) cross correlation interference signals of two groups of metal gauges; (c) relative phase distribution after continuity; (d) recovered absolute phase of “air gap”.
图 3 “空气隙”采样绝对相位的相位差波动及不同区域相位差波动情况
Fig. 3. Phase difference fluctuations of absolute phase of “air gap” and phase difference fluctuations in different sampling regions.
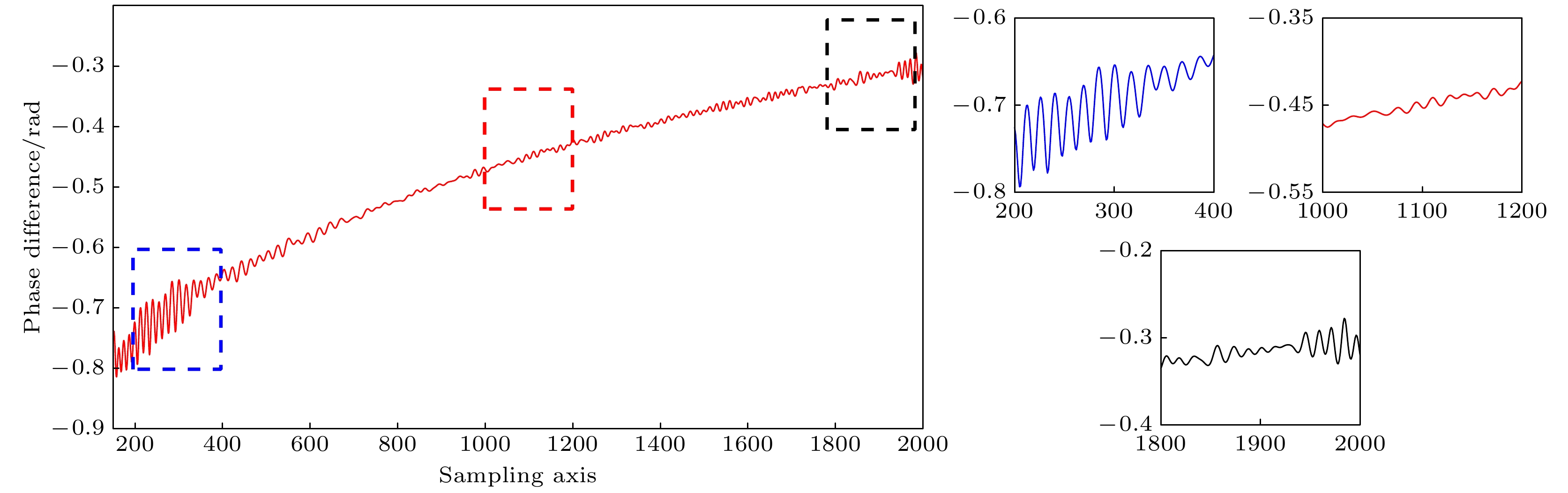
图 4 最佳窗口约束条件下的拟合绝对相位及不同窗约束条件下相位标准偏差分布曲线 (a)最佳拟合绝对相位分布; (b)不同窗约束下相位标准偏差变化曲线
Fig. 4. Absolute phase fitting curve under optimum window constraints and phase standard deviation curve under different window constraints: (a) The optimal fitting of the absolute phase distribution; (b) phase standard deviation curve under different window constraints.
图 6 经KDSI方法和WC-KDSI方法处理得到的量规反射面定位结果
Fig. 6. Positioning results of gauges’ interfaces processed by KDSI method and WC-KDSI method.
图 7 使用(a)直接FFT, (b) KDSI, (c) WC-KDSI算法获得的镜面腐蚀微形貌OCT重建图像; (d)不同扫描位置轴向PSF比较
Fig. 7. Reconstructed OCT images of mirror corrosion by (a) direct FFT, (b) KDSI, (c) WC-KDSI method; (d) comparison of axial PSFs at different scanning points.
图 8 使用(a) KDSI算法及(b) WC-KDSI算法处理获得的橘子果肉OCT重建图像; (c)特定扫描位置的轴向PSF比较
Fig. 8. Reconstructed OCT images of orange pulp by (a) KDSI and (b) WC-KDSI algorithm; (c) comparison of axial PSFs at certain scanning position.
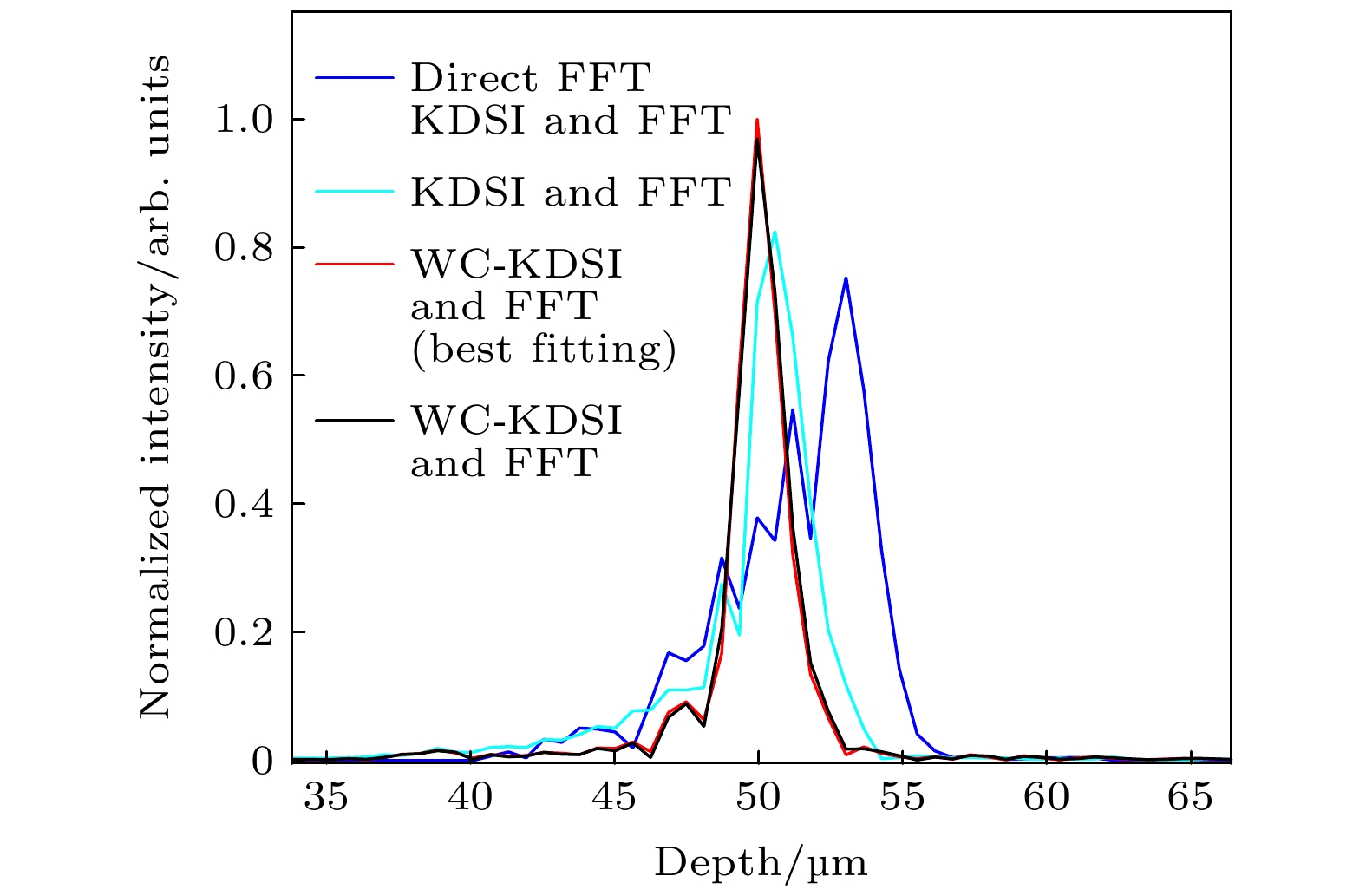
表 1 不同方法获得的轴向分辨率和峰值归一化强度
Table 1. Axial resolution and peak intensity resulted from different methods.
WC-KDSI
(Best fitting)WC-KDSI
(1800 window)KDSI Direct FFT Axial resolution/μm 1.6 1.6 2.1 5.3 Normalized intensity 1.0 0.97 0.81 0.73 
下载: 导出CSV
-
[1] Huang D, Swanson E A, Lin C P, Schuman J S, Stinson W G, Chang W, Hee M R, Flotte T, Gregory K, Puliafito C A, Fujimoto J G 1991 Science 254 1178
Google Scholar
[2] Tomlins P H, Wang R K 2005 J. Phys. D: Appl. Phys. 38 2519
Google Scholar
[3] 赵晨, 陈志彦, 丁志华, 李鹏, 沈毅, 倪秧 2014 63 194201
Google Scholar
Zhao C, Chen Z Y, Ding Z H, Li P, Shen Y, Ni Y 2014 Acta Phys. Sin. 63 194201
Google Scholar
[4] Kumar M, Islam M N, Terry F L, Aleksoff C C, Davidson D 2010 Opt. Express 18 22471
Google Scholar
[5] Heise B, Schausberger S E, Häuser S, Plank B, Salaberger D, Leiss-Holzinger E, Stifter D 2012 Opt. Fiber. Technol. 18 403
Google Scholar
[6] Wiesauer K, Pircher M, Götzinger E, Bauer S, Engelke R, Ahrens G, Grützner G, Hitzenberger C K, Stifter D 2005 Opt. Express 13 1015
Google Scholar
[7] Endo T, Yasuno Y, Makita S, Itoh M, Yatagai T 2005 Opt. Express 13 695
Google Scholar
[8] Leitgeb R, Hitzenberger C K, Fercher A F 2003 Opt. Express 11 889
Google Scholar
[9] Choma M A, Sarunic M V, Yang C, Izatt J A 2003 Opt. Express 11 2183
Google Scholar
[10] de Boer J F, Cense B, Park B H, Pierce M C, Tearney G J, Bouma B E 2003 Opt. Lett. 28 2067
Google Scholar
[11] Dorrer C, Belabas N, Likforman J P, Joffre M 2000 J. Opt. Soc. Am. B 17 1795
Google Scholar
[12] Uribe-Patarroyo N, Kassani S H, Villiger M, Bouma B E 2018 Opt. Express 26 9081
Google Scholar
[13] Hu Z, Rollins A M 2007 Opt. Lett. 32 3525
Google Scholar
[14] 吴彤, 孙帅帅, 王绪晖, 王吉明, 赫崇君, 顾晓蓉, 刘友文 2018 67 104208
Google Scholar
Wu T, Sun S S, Wang X H, Wang J M, He C J, Gu X R, Liu Y W 2018 Acta Phys. Sin. 67 104208
Google Scholar
[15] Hyle Park B, Pierce M C, Cense B, Yun S H, Mujat M, Tearney G J, Bouma B E, Boer J F D 2005 Opt. Express 13 3931
Google Scholar
[16] Perret E, Balmer T E, Heuberger M 2010 Appl. Spectrosc. 64 1139
Google Scholar
[17] Eom T J, Ahn Y C, Kim C S, Chen Z P 2011 J. Biomed. Opt. 16 1
Google Scholar
[18] Wang K, Ding Z 2008 Chin. Opt. Lett. 6 902
Google Scholar
[19] Wu X, Ye X, Yu D, Yu J, Huang Y, Tan H, Qin J, An L 2020 OSA Continuum. 3 2156
Google Scholar
[20] Ikeda T, Popescu G, Dasari R R, Feld M S 2005 Opt. Lett. 30 1165
Google Scholar
[21] Meissner M 2012 Acta Phys. Pol. A 121 164
Google Scholar
[22] Yan Y, Ding Z, Shen Y, Chen Z, Zhao C, Ni Y 2013 Opt. Express 21 25734
Google Scholar
[23] Han T, Qiu J, Wang D, Meng J, Liu Z, Ding Z 2020 J. Innov. Opt. Heal. Sci. 14 2140008
Google Scholar
[24] 唐弢, 赵晨, 陈志彦, 李鹏, 丁志华 2015 64 174201
Google Scholar
Tang T, Zhao C, Chen Z Y, Li P, Ding Z H 2015 Acta Phys. Sin. 64 174201
Google Scholar
[25] Wang C, You Y J, Ai S, Zhang W, Liao W, Zhang X, Hsieh J, Zhang N, Tang B, Pan C L, Xue P 2019 J. Innov. Opt. Heal. Sci. 12 1950009
Google Scholar
下载:
计量
- 文章访问数: 3226
- PDF下载量: 48
- 被引次数: 0
