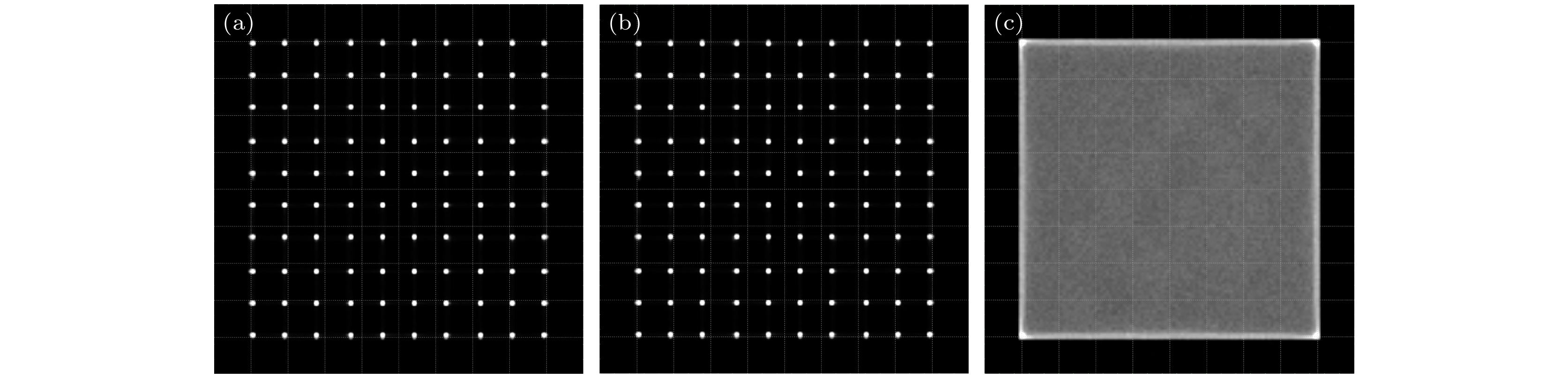
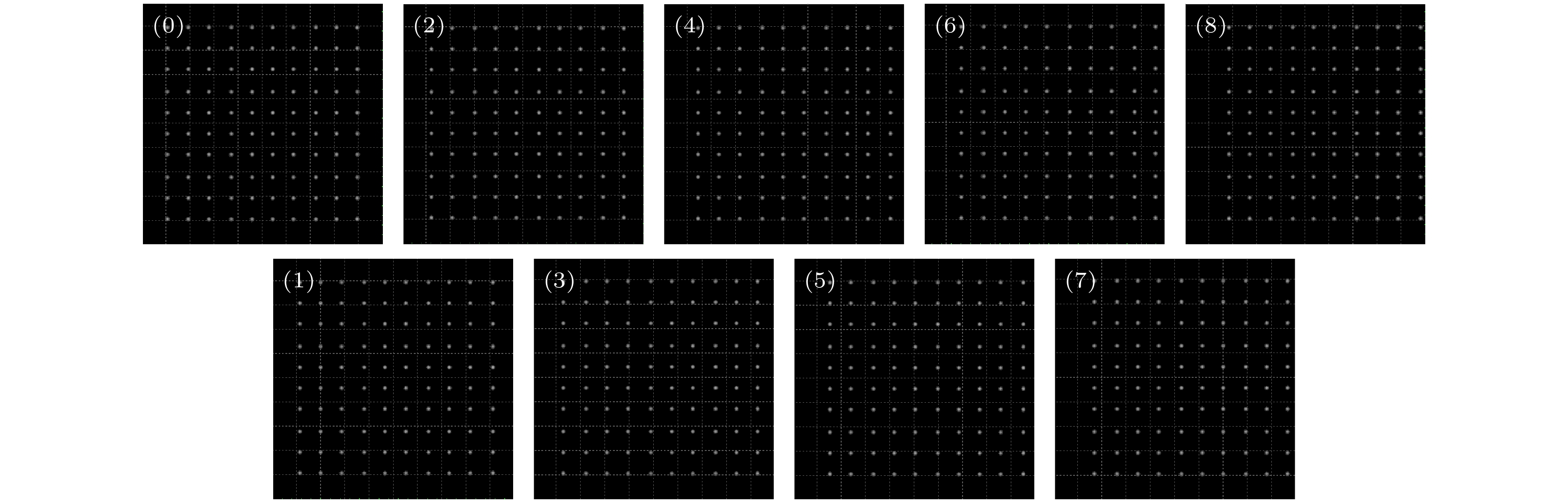
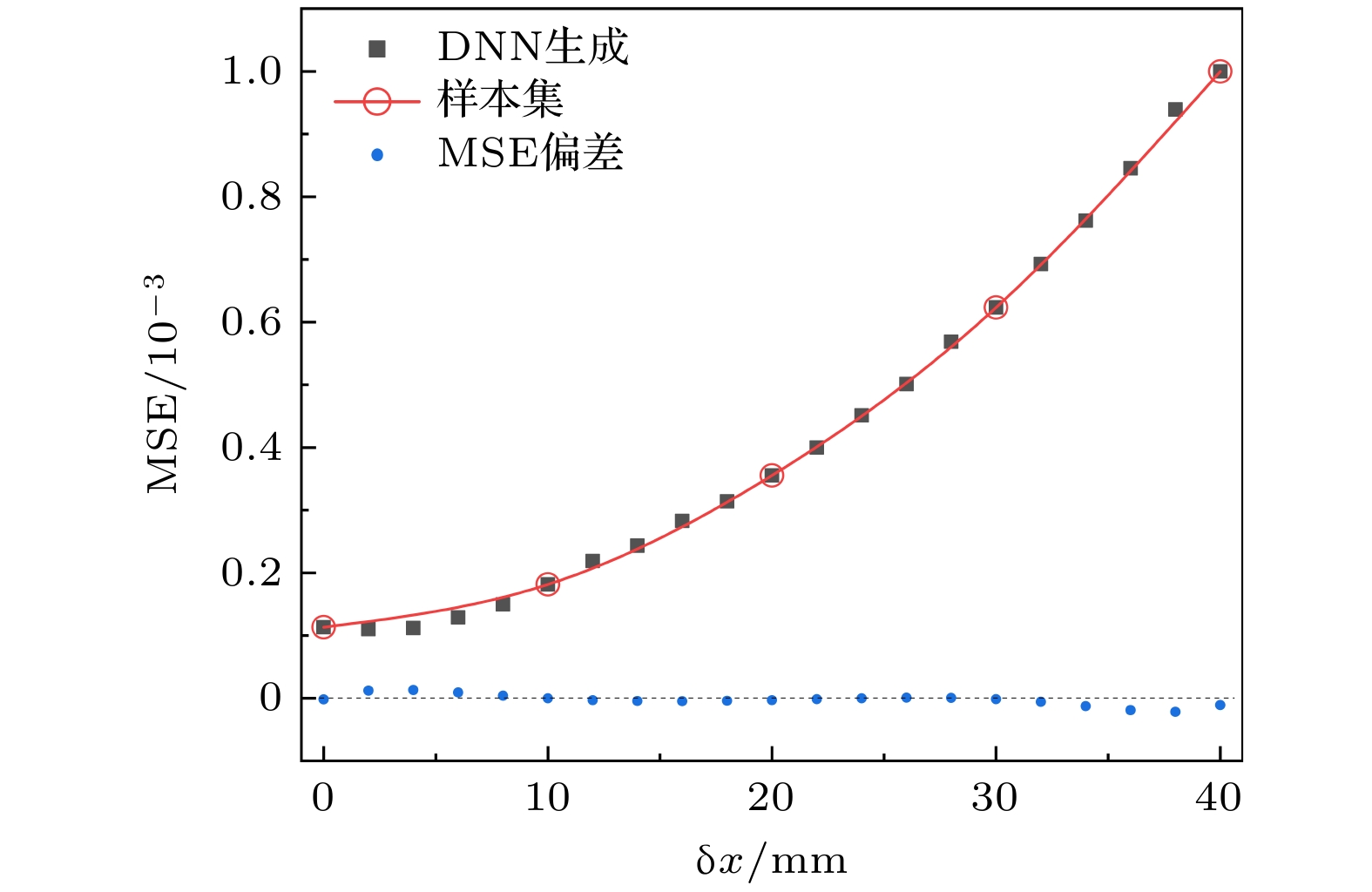
-
以能量控制为目标的非成像光学设计在光电领域有着广泛的应用, 由光源、光学器件和目标面三者组成的非成像光学系统可用一个配光方程来描述. 给定光源和目标光斑, 求解配光方程可得相应的光学表面. 如果光源不变而目标光斑仅在目标面上发生移动, 此时光学表面就得做出相应的变换, 这种变换可由一个配光平移群来刻画. 通过引入具有单调性质的光程常数与能量之间的映射关系, 并利用深度神经网络拟合构建了配光平移变换群. 以均匀方斑为例, 利用程能映射之一的支撑椭流面法生成训练样本数据, 通过对深度神经网络进行多维度调参和训练, 实现配光平移群的学习. 光学仿真结果表明深度神经网络对配光平移群表达具有误差小和速度快的优点, 在一定程度上实现了非成像光学设计的智能化.
Nonimaging optical design aiming at energy control has wide applications in optoelectronics. A nonimaging optical system is composed of a light source, optical components, and a target screen, and can be described by an equation named light taming equation(LTE). Given the light source and prescribed target spot, the required freeform surfaces of the optical component can be obtained by solving the LTE. If the light source profile does not change, the optical surface will make some suitable morphs when the target spot translates on the screen, and these morph operators can well be described by the group theory. The basic LTE is established for a normal nonimaging optical system, which is to design an optical element for redirecting the light from the source so that a prescribed light distribution is generated on a given target. A translation light taming equation(T-LTE) is derived for the case of only spot translating on the target screen, and an optical translation group(OTG) is introduced for describing all of the morph operators of the optical surface caused by light spot translation. There are multiple solutions for the same T-LTE, but the uniqueness of the T-LTE solution is necessary for OTG. Fortunately, the eikonal-energy(KE) mapping method can guarantee the uniqueness of the T-LTE solution, where K is the optical path length. The supporting quadric method(SQM) is one of the KE mapping methods when the nonimaging optical system has only one optical surface to be resolved. The LTE with SQM is deduced, and the OTG can be discussed in K-space. A deep neural network(DNN) is introduced to fit the KE mapping and spot translating operators to obtain the required optical surface. Taking the uniform square spot for example, the SQM generates the sample data of spot translation to train the DNN. The optical simulation results show that the error between the light distribution generated by the DNN and the standard uniform square spot is small, all on the order of 10−3, which indicates that the DNN and KE mapping method have successfully realized the function of the OTG. The results are of guiding significance in implementing the intelligent nonimaging optical design. -
Keywords:
- nonimaging optical design /
- freeform surface /
- deep neural network /
- eikonal-energy mapping
[1] Fang F Z, Zhang N, Zhang X D 2016 Adv. Opt. Techn. 5 303
[2] Wu R M, Feng Z X, Zheng Z R, Liang R G, Benítez P, Miñano J C, Duerret F 2018 Laser Photonics Rev. 12 1700310
Google Scholar
[3] Newman J N 2020 Appl. Ocean Res. 94 101973
Google Scholar
[4] Mendes-Lopes J, Benítez P, Miñano J C, A Santamaría 2016 Opt. Express. 24 5584
Google Scholar
[5] Ries H, Muschaweck J 2002 J. Opt. Soc. Am. A. 19 590
Google Scholar
[6] Chang S Q, Wu R M, An L, Zheng Z R 2016 J. Opt. 18 125602
Google Scholar
[7] Gutiérrez C E, Pallucchini L 2018 J. Opt. Soc. Am. 35 1523
Google Scholar
[8] Ma Y F, Zhang H, Su Z Y, He Y, Xu L, Liu X, Li H F 2015 Appl. Opt. 54 4503
Google Scholar
[9] Oliker V 2017 Opt. Express. 25 A58
Google Scholar
[10] Doskolovich L L, Moiseev M A, Bezus E A, Oliker V 2015 Opt. Express. 23 19605
Google Scholar
[11] Lucas A, Iliadis M, Molina R, Katsaggelos A K 2018 IEEE Signal Proc. Mag. 35 20
[12] Goodfellow I, Bengio Y, Courville A 2016 Deep learning (Cambridge: MIT) pp167–227
[13] 张瑶, 张云波, 陈立 2021 70 168702
Google Scholar
Zhang Y, Zhang Y B, Chen L 2021 Acta Phys. Sin. 70 168702
Google Scholar
[14] 苏博, 陶芬, 李可, 杜国浩, 张玲, 李中亮, 邓彪, 谢红兰, 肖体乔 2021 70 160704
Google Scholar
Su B, Tao F, Li K, Du G H, Zhang L, Li Z L, Deng B, Xie H L, Xiao T Q 2021 Acta Phys. Sin. 70 160704
Google Scholar
[15] 王飞, 王昊, 卞耀明, 司徒国海 2020 光学学报 40 0111002
Google Scholar
Wang F, Wang H, Bian Y M, Situ G H 2020 Acta Opt. Sin. 40 0111002
Google Scholar
[16] 张航, 严金华 2016 非成像光学设计 (北京: 科学出版社) 第7—12页
Zhang H, Yan J H 2016 Nonimaging Optical Design (Beijing: Science Press) pp7–12 (in Chinese)
[17] Jeevanjee N 2011 An Introduction to Tensors and Group Theory for Physicists (New York: Springer Press) p87–96
[18] 马中骐 2003 物理学中的群论 (北京: 科学出版社) 第27—32页
Ma Z Q 2003 Group in Physics (Beijing: Science Press) p27–32 (in Chinese)
[19] Zins P, Dagenais M 2019 Int. J. Parallel Prog. 47 973
Google Scholar
-
图 4 均匀方斑光分布 (a) 目标分布; (b) 仿真分布; (c) 光滑化分布
Fig. 4. Flux distribution of uniform square spot: (a) Original target; (b) simulation; (c) smooth spot.
图 5 DNN生成的平移光斑:
$\Delta = [i{\kern 1 pt} {\kern 1 pt} d, 0]$ , i为子图号Fig. 5. The shift spot generated by DNN:
$\Delta = [i{\kern 1 pt} {\kern 1 pt} d, 0]$ , i is the number of subfigure. -
[1] Fang F Z, Zhang N, Zhang X D 2016 Adv. Opt. Techn. 5 303
[2] Wu R M, Feng Z X, Zheng Z R, Liang R G, Benítez P, Miñano J C, Duerret F 2018 Laser Photonics Rev. 12 1700310
Google Scholar
[3] Newman J N 2020 Appl. Ocean Res. 94 101973
Google Scholar
[4] Mendes-Lopes J, Benítez P, Miñano J C, A Santamaría 2016 Opt. Express. 24 5584
Google Scholar
[5] Ries H, Muschaweck J 2002 J. Opt. Soc. Am. A. 19 590
Google Scholar
[6] Chang S Q, Wu R M, An L, Zheng Z R 2016 J. Opt. 18 125602
Google Scholar
[7] Gutiérrez C E, Pallucchini L 2018 J. Opt. Soc. Am. 35 1523
Google Scholar
[8] Ma Y F, Zhang H, Su Z Y, He Y, Xu L, Liu X, Li H F 2015 Appl. Opt. 54 4503
Google Scholar
[9] Oliker V 2017 Opt. Express. 25 A58
Google Scholar
[10] Doskolovich L L, Moiseev M A, Bezus E A, Oliker V 2015 Opt. Express. 23 19605
Google Scholar
[11] Lucas A, Iliadis M, Molina R, Katsaggelos A K 2018 IEEE Signal Proc. Mag. 35 20
[12] Goodfellow I, Bengio Y, Courville A 2016 Deep learning (Cambridge: MIT) pp167–227
[13] 张瑶, 张云波, 陈立 2021 70 168702
Google Scholar
Zhang Y, Zhang Y B, Chen L 2021 Acta Phys. Sin. 70 168702
Google Scholar
[14] 苏博, 陶芬, 李可, 杜国浩, 张玲, 李中亮, 邓彪, 谢红兰, 肖体乔 2021 70 160704
Google Scholar
Su B, Tao F, Li K, Du G H, Zhang L, Li Z L, Deng B, Xie H L, Xiao T Q 2021 Acta Phys. Sin. 70 160704
Google Scholar
[15] 王飞, 王昊, 卞耀明, 司徒国海 2020 光学学报 40 0111002
Google Scholar
Wang F, Wang H, Bian Y M, Situ G H 2020 Acta Opt. Sin. 40 0111002
Google Scholar
[16] 张航, 严金华 2016 非成像光学设计 (北京: 科学出版社) 第7—12页
Zhang H, Yan J H 2016 Nonimaging Optical Design (Beijing: Science Press) pp7–12 (in Chinese)
[17] Jeevanjee N 2011 An Introduction to Tensors and Group Theory for Physicists (New York: Springer Press) p87–96
[18] 马中骐 2003 物理学中的群论 (北京: 科学出版社) 第27—32页
Ma Z Q 2003 Group in Physics (Beijing: Science Press) p27–32 (in Chinese)
[19] Zins P, Dagenais M 2019 Int. J. Parallel Prog. 47 973
Google Scholar
下载:
计量
- 文章访问数: 3200
- PDF下载量: 35
- 被引次数: 0
