-
采用磁控溅射方法在MgO(001)单晶衬底上制备了交换偏置分别沿着FeGa
$ \left[100\right] $ 和[110]方向的FeGa/IrMn外延交换偏置双层膜, 研究了交换偏置取向对磁化翻转过程与磁化翻转场的影响. 铁磁共振场的角度依赖关系的测量与拟合, 表明样品存在不同取向的四重对称磁晶各向异性、单向交换磁各向异性和单轴磁各向异性的叠加. 矢量磁光克尔效应测量表明交换偏置沿着$ \left[100\right] $ 方向的样品在不同磁场方向下表现矩形、非对称和单边两步磁滞回线; 交换偏置沿着$ \left[110\right] $ 方向的样品在不同磁场方向下表现单边两步和双边两步磁滞回线. 考虑不同交换偏置方向的畴壁形核和位移模型, 能够很好地解释磁化翻转路径随磁场方向的变化规律和拟合磁化翻转场的角度依赖关系, 表明交换偏置方向的改变使得畴壁形核能发生显著变化.Epitaxial FeGa/IrMn bilayers with exchange biases along the FeGa[100] and [110] directions are prepared on MgO(001) single crystal substrates by magnetron sputtering through controlling the orientation of the external field in situ applied during growth. The effect of the exchange bias orientation on the magnetic switching process and the magnetic switching field are studied. The X-ray φ-scan indicates that the FeGa layer is epitaxially grown with a 45° in-plane rotation on the MgO(001) substrate along the FeGa(001)[110] direction and the MgO(001)[100] direction. The measurements of the angular dependence of the ferromagnetic resonance field and the corresponding fitting to the Kittel equation show that the samples have a superposition of fourfold symmetric magnetocrystalline anisotropy$ {K}_{1} $ , unidirectional magnetic exchange bias anisotropy$ {K}_{\mathrm{e}\mathrm{b}} $ , and uniaxial magnetic anisotropy$ {K}_{\mathrm{u}} $ with configuration of$ {K}_{\mathrm{e}\mathrm{b}}//\left[100\right] $ or$ {K}_{\mathrm{e}\mathrm{b}}//\left[110\right] $ . The combined longitudinal and transverse magneto-optical Kerr effect measurements show that sample with$ {K}_{\mathrm{e}\mathrm{b}}//\left[100\right] $ exhibits square loops, asymmetrically shaped loops, and one-sided two-step loops in different external magnetic field directions. In contrast, the sample with$ {K}_{\mathrm{e}\mathrm{b}}//\left[110\right] $ exhibits one-sided two-step and two-sided two-step loops as the magnetic field orientation changes. Because the$ {K}_{1} $ is superimposed by$ {K}_{\mathrm{u}} $ and$ {K}_{\mathrm{e}\mathrm{b}} $ , the in-plane fourfold symmetry of the magnetic anisotropy energy is broken. The local minima are no longer strictly along the in-plane$ \left\langle{100}\right\rangle $ directions, but make a deviation angle which depends on the relative orientation and strength of magnetic anisotropy. A model based on the domain wall nucleation and propagation is proposed with considering the different orientations of$ {K}_{\mathrm{e}\mathrm{b}} $ , which can nicely explain the change of the magnetic switching route with the magnetic field orientation and fit the angular dependence of the magnetic switching fields, indicating a significant change of domain wall nucleation energy as the orientation of$ {K}_{\mathrm{e}\mathrm{b}} $ changes.-
Keywords:
- epitaxial exchange bias /
- magnetic anisotropy /
- magnetization reversal /
- domain wall nucleation and propagation
[1] Nogues J, Schuller I K 1999 J. Magn. Magn. Mater. 192 203
Google Scholar
[2] Blachowicz T, Ehrmann A 2021 Coatings 11 122
Google Scholar
[3] Meiklejohn W H, Bean C P 1956 Phys. Rev. 102 1413
Google Scholar
[4] Wolf S A, Awschalom D D, Buhrman R A, Daughton J M, Von Molnar S, Roukes M L, Chtchelkanova A Y, Treger D M 2001 Science 294 1488
Google Scholar
[5] Wen X, Wu R, Yang W Y, Wang C S, Liu S Q, Han J Z, Yang J B 2020 Chin. Phys. B 29 098503
Google Scholar
[6] Wu S M, Cybart S A, Yu P, Rossell M D, Zhang J X, Ramesh R, Dynes R C 2010 Nat. Mater. 9 756
Google Scholar
[7] Schafer D, Geshev J, Nicolodi S, Pereira L G, Schmidt J E, Grande P L 2008 Appl. Phys. Lett. 93 042501
Google Scholar
[8] Zhang X S, Zhan Q F, Dai G H, Liu Y W, Zuo Z H, Yang H L, Chen B, Li R W 2013 Appl. Phys. Lett. 102 022412
Google Scholar
[9] Jiménez E, Camarero J, Perna P, Mikuszeit N, Terán F J, Sort J, Nogués J, García Martín J M, Hoffmann A, Dieny B, Miranda R 2011 J. Appl. Phys. 109 07D730
Google Scholar
[10] Zhan Q F, Vandezande S, Van Haesendonck C, Temst K 2007 Appl. Phys. Lett. 91 122510
Google Scholar
[11] Bera A K, Kumar D 2020 AIP Conf. Proc. 2265 030315
Google Scholar
[12] Wang S G, Kohn A, Wang C, Petford Long A K, Lee S, Fan R, Goff J P, Singh L J, Barber Z H, Ward R C C 2009 J. Phys. D 42 225001
Google Scholar
[13] Camarero J, Sort J, Hoffmann A, Garcia Martin J M, Dieny B, Miranda R, Nogues J 2005 Phys. Rev. Lett. 95 057204
Google Scholar
[14] Zhan Q F, Zhang W, Krishnan K M 2011 Phys. Rev. B 83 094404
Google Scholar
[15] Zhang Y, Zhan Q F, Zuo Z H, Yang H L, Zhang X S, Dai G H, Liu Y W, Yu Y, Wang J, Wang B M, Li R W 2015 Phys. Rev. B 91 174411
Google Scholar
[16] Chen Y, Washburn J 1996 Phys. Rev. Lett. 77 4046
Google Scholar
[17] Zhan Q F, Krishnan K M 2010 Appl. Phys. Lett. 96 112506
Google Scholar
[18] Mendes J B S, Cunha R O, Alves Santos O, Ribeiro P R T, Machado F L A, Rodríguez Suárez R L, Azevedo A, Rezende S M 2014 Phys. Rev. B 89 140406
Google Scholar
[19] Baltz V, Manchon A, Tsoi M, Moriyama T, Ono T, Tserkovnyak Y 2018 Rev. Mod. Phys. 90 015005
Google Scholar
[20] Sun R, Li Y, Xie Z K, Li Y, Zhao X T, Liu W, Zhang Z D, Zhu T, Cheng Z H, He W 2020 J. Magn. Magn. Mater. 497 165971
Google Scholar
[21] Farle M 1998 Rep. Prog. Phys. 61 755
Google Scholar
[22] Li Y, Li Y, Liu Q, Xie Z K, Vetter E, Yuan Z, He W, Liu H L, Sun D L, Xia K, Yu W, Sun Y B, Zhao J J, Zhang X Q, Cheng Z H 2019 New J. Phys. 21 103040
Google Scholar
[23] Zhan Q F, Stijn V, Kristiaan T, Chris V H 2009 New J. Phys. 11 063003
Google Scholar
[24] Dean J, Bryan M T, Morley N A, Hrkac G, Javed A, Gibbs M R J, Allwood D A 2011 J. Appl. Phys. 110 043902
Google Scholar
[25] Cowburn R P, Gray S J, Ferré J, Bland J A C, Miltat J 1995 J. Appl. Phys. 78 7210
Google Scholar
[26] Postava K, Jaffres H, Schuhl A, Van Dau F N, Goiran M, Fert A R 1997 J. Magn. Magn. Mater. 172 199
Google Scholar
-
图 1 样品FeGa/IrMn/MgO(001)的X射线衍射图 (a) θ-2θ 扫描图; (b) 面内φ扫描图
Fig. 1. X-ray diffraction measurement for the sample of FeGa/IrMn/MgO(001): (a) θ-2θ scan; (b) in-plane φ
-scan. 
图 2 (a) FeGa单层膜和(b)
$ {K}_{\mathrm{e}\mathrm{b}}//\left[100\right] $ 的FeGa/IrMn双层膜在${\varphi }_{H}=$ 0°, 30°, 45°时的代表性铁磁共振微分吸收谱; (c), (d) 相应的共振场$ {H}_{\mathrm{r}} $ 随${\varphi }_{H}$ 的变化关系(空心点为实验值, 实线(a), (b)和虚线(c), (d)为拟合曲线)Fig. 2. Representative ferromagnetic resonance derivative absorption spectra for (a) FeGa single layer and (b) FeGa/IrMn bilayer with
$ {K}_{\mathrm{e}\mathrm{b}}//\left[100\right] $ measured at${\varphi }_{H}=$ 0°, 30°, 45°; (c), (d) the corresponding resonance field$ {H}_{\mathrm{r}} $ as a function of${\varphi }_{H}$ (Open dots are the experimental data, solid (a), (b) and dashed (c), (d) lines are the theoretical fitting results).
图 3 在不同外磁场方向
$ {\varphi }_{H} $ 下,$ {K}_{\mathrm{e}\mathrm{b}}//\left[100\right] $ 和$ {K}_{\mathrm{e}\mathrm{b}}//\left[110\right] $ 的FeGa/IrMn双层膜的典型纵向和横向MOKE磁滞回线(Ms是饱和磁化强度) (a)$ {K}_{\mathrm{e}\mathrm{b}}//\left[100\right], $ $ {\varphi }_{H}=0^\circ; $ (b)$ {K}_{\mathrm{e}\mathrm{b}}//\left[100\right], $ $ {\varphi }_{H}=30^\circ; $ (c)$ {K}_{\mathrm{e}\mathrm{b}}//\left[100\right], $ $ {\varphi }_{H}= $ $ 45^\circ; $ (d)$ {K}_{\mathrm{e}\mathrm{b}}//\left[100\right], $ $ {\varphi }_{H}=90^\circ $ ; (e)$ {K}_{\mathrm{e}\mathrm{b}}//\left[110\right], {\varphi }_{H}=0^\circ; $ (f)$ {K}_{\mathrm{e}\mathrm{b}}//\left[110\right], {\varphi }_{H}=35^\circ; $ (g)$ {K}_{\mathrm{e}\mathrm{b}}//\left[110\right], {\varphi }_{H}=45^\circ; $ (h)$ {K}_{\mathrm{e}\mathrm{b}}//\left[110\right], {\varphi }_{H}=90^\circ\mathrm{. } $ 蓝线和红线分别对应于磁滞回线的磁场下行支和磁场上行支; 磁化翻转过程中FeGa自旋方向用箭头表示; 相应的磁化翻转场也标记在图中Fig. 3. Typical longitudinal and transverse MOKE loops at different external field orientations
$ {\varphi }_{H} $ for the FeGa/IrMn bilayer with$ {K}_{\mathrm{e}\mathrm{b}}//\left[100\right] $ and$ {K}_{\mathrm{e}\mathrm{b}}//\left[110\right], $ Ms is the saturation magnetization: (a)$ {K}_{\mathrm{e}\mathrm{b}}//\left[100\right], $ $ {\varphi }_{H}=0^\circ; $ (b)$ {K}_{\mathrm{e}\mathrm{b}}//\left[100\right], $ $ {\varphi }_{H}=30^\circ; $ (c)${K}_{\mathrm{e}\mathrm{b}}// $ $ \left[100\right],$ $ {\varphi }_{H}= $ $ 45^\circ; $ (d)$ {K}_{\mathrm{e}\mathrm{b}}//\left[100\right], $ $ {\varphi }_{H}=90^\circ $ ; (e)$ {K}_{\mathrm{e}\mathrm{b}}//\left[110\right], {\varphi }_{H}=0^\circ; $ (f)$ {K}_{\mathrm{e}\mathrm{b}}//\left[110\right], {\varphi }_{H}=35^\circ; $ (g)$ {K}_{\mathrm{e}\mathrm{b}}//\left[110\right], {\varphi }_{H}=45^\circ; $ (h)${K}_{\mathrm{e}\mathrm{b}}//\left[110\right], $ $ {\varphi }_{H}=90^\circ.$ The blue and red curves correspond to the magnetic field descending and ascending branches of hyste-resis loops, respectively; the arrows enclosed by a square represent the orientation of FeGa spins in the magnetic switching routes; the corresponding magnetic switching fields are presented as well.
图 4 FeGa/IrMn双层膜的磁化翻转场随外磁场方向
$ {\varphi }_{H} $ 的变化关系 (a)$ {K}_{\mathrm{e}\mathrm{b}}//\left[100\right] $ ; (b)$ {K}_{\mathrm{e}\mathrm{b}}//\left[110\right] $ (实心和空心点对应于实验值, 实线和虚线对应于拟合曲线)Fig. 4. External magnetic field orientation
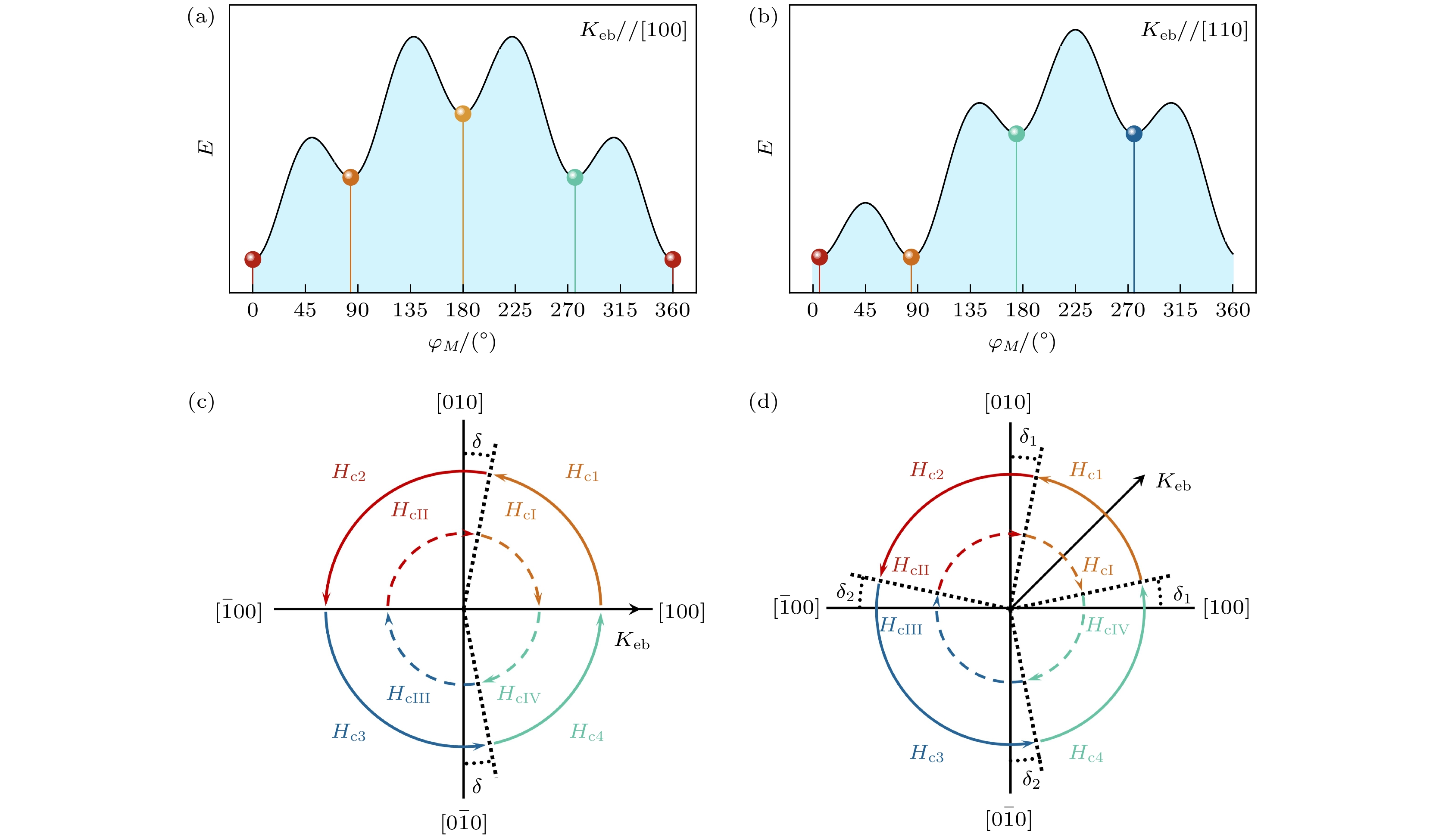
$ {\varphi }_{H} $ dependence of the magnetic switching fields for the FeGa/IrMn bilayers: (a)$ {K}_{\mathrm{e}\mathrm{b}}//\left[100\right] $ ; (b)$ {K}_{\mathrm{e}\mathrm{b}}//\left[110\right] $ (The solid and open dots represent experimental values, and the solid and dashed lines represent fitted curves).
图 5 FeGa/IrMn双层膜的磁各向异性能随磁化强度方向的变化关系 (a)
$ {K}_{\mathrm{e}\mathrm{b}}//\left[100\right] $ ; (b)$ {K}_{\mathrm{e}\mathrm{b}}//\left[110\right] $ ; (c), (d) 相对应的磁各向异性改变示意图Fig. 5. Magnetic anisotropy energy as a function of orientation of magnetization in FeGa/IrMn bilayers: (a)
$ {K}_{\mathrm{e}\mathrm{b}}//\left[100\right] $ ; (b)$ {K}_{\mathrm{e}\mathrm{b}}//\left[110\right] $ ; (c), (d) corresponding schematic diagram of magnetic anisotropy change. -
[1] Nogues J, Schuller I K 1999 J. Magn. Magn. Mater. 192 203
Google Scholar
[2] Blachowicz T, Ehrmann A 2021 Coatings 11 122
Google Scholar
[3] Meiklejohn W H, Bean C P 1956 Phys. Rev. 102 1413
Google Scholar
[4] Wolf S A, Awschalom D D, Buhrman R A, Daughton J M, Von Molnar S, Roukes M L, Chtchelkanova A Y, Treger D M 2001 Science 294 1488
Google Scholar
[5] Wen X, Wu R, Yang W Y, Wang C S, Liu S Q, Han J Z, Yang J B 2020 Chin. Phys. B 29 098503
Google Scholar
[6] Wu S M, Cybart S A, Yu P, Rossell M D, Zhang J X, Ramesh R, Dynes R C 2010 Nat. Mater. 9 756
Google Scholar
[7] Schafer D, Geshev J, Nicolodi S, Pereira L G, Schmidt J E, Grande P L 2008 Appl. Phys. Lett. 93 042501
Google Scholar
[8] Zhang X S, Zhan Q F, Dai G H, Liu Y W, Zuo Z H, Yang H L, Chen B, Li R W 2013 Appl. Phys. Lett. 102 022412
Google Scholar
[9] Jiménez E, Camarero J, Perna P, Mikuszeit N, Terán F J, Sort J, Nogués J, García Martín J M, Hoffmann A, Dieny B, Miranda R 2011 J. Appl. Phys. 109 07D730
Google Scholar
[10] Zhan Q F, Vandezande S, Van Haesendonck C, Temst K 2007 Appl. Phys. Lett. 91 122510
Google Scholar
[11] Bera A K, Kumar D 2020 AIP Conf. Proc. 2265 030315
Google Scholar
[12] Wang S G, Kohn A, Wang C, Petford Long A K, Lee S, Fan R, Goff J P, Singh L J, Barber Z H, Ward R C C 2009 J. Phys. D 42 225001
Google Scholar
[13] Camarero J, Sort J, Hoffmann A, Garcia Martin J M, Dieny B, Miranda R, Nogues J 2005 Phys. Rev. Lett. 95 057204
Google Scholar
[14] Zhan Q F, Zhang W, Krishnan K M 2011 Phys. Rev. B 83 094404
Google Scholar
[15] Zhang Y, Zhan Q F, Zuo Z H, Yang H L, Zhang X S, Dai G H, Liu Y W, Yu Y, Wang J, Wang B M, Li R W 2015 Phys. Rev. B 91 174411
Google Scholar
[16] Chen Y, Washburn J 1996 Phys. Rev. Lett. 77 4046
Google Scholar
[17] Zhan Q F, Krishnan K M 2010 Appl. Phys. Lett. 96 112506
Google Scholar
[18] Mendes J B S, Cunha R O, Alves Santos O, Ribeiro P R T, Machado F L A, Rodríguez Suárez R L, Azevedo A, Rezende S M 2014 Phys. Rev. B 89 140406
Google Scholar
[19] Baltz V, Manchon A, Tsoi M, Moriyama T, Ono T, Tserkovnyak Y 2018 Rev. Mod. Phys. 90 015005
Google Scholar
[20] Sun R, Li Y, Xie Z K, Li Y, Zhao X T, Liu W, Zhang Z D, Zhu T, Cheng Z H, He W 2020 J. Magn. Magn. Mater. 497 165971
Google Scholar
[21] Farle M 1998 Rep. Prog. Phys. 61 755
Google Scholar
[22] Li Y, Li Y, Liu Q, Xie Z K, Vetter E, Yuan Z, He W, Liu H L, Sun D L, Xia K, Yu W, Sun Y B, Zhao J J, Zhang X Q, Cheng Z H 2019 New J. Phys. 21 103040
Google Scholar
[23] Zhan Q F, Stijn V, Kristiaan T, Chris V H 2009 New J. Phys. 11 063003
Google Scholar
[24] Dean J, Bryan M T, Morley N A, Hrkac G, Javed A, Gibbs M R J, Allwood D A 2011 J. Appl. Phys. 110 043902
Google Scholar
[25] Cowburn R P, Gray S J, Ferré J, Bland J A C, Miltat J 1995 J. Appl. Phys. 78 7210
Google Scholar
[26] Postava K, Jaffres H, Schuhl A, Van Dau F N, Goiran M, Fert A R 1997 J. Magn. Magn. Mater. 172 199
Google Scholar
下载:
计量
- 文章访问数: 4589
- PDF下载量: 111
- 被引次数: 0
