-
石墨烯是一种特殊的二维材料, 其独特的能带结构允许人们通过电场来调控其载流子的类型和浓度, 因此, 在构建双极型纳米电子器件方面具有潜在应用前景. 本文基于紧束缚格点模型, 利用非平衡格林函数方法及Landauer-Büttiker公式, 研究了石墨烯p-n结在磁场中的电输运热耗散问题. 在强磁场作用下, 结的两边均处于量子霍尔相, 存在拓扑保护的手性边缘态. 直觉上, 这种拓扑保护的手性边缘态应当是无热耗散的. 但本文研究发现, 当有耗散源时, 尽管手性边缘态受到拓扑保护, 热耗散却依然可以发生. 对于完美的石墨烯, 单极结输运时热耗散发生在体系边缘; 偶极结输运时在体系边缘和结的界面处均可以发生热耗散. 当无序存在时, 无论单极结还是偶极结, 无序均能增强热耗散. 此外, 本文还研究了不同位置处的电子能量分布, 发现热耗散是否发生只取决于电子是否处于非平衡分布. 这些结果表明拓扑只能保护电子的传播方向, 却不能禁止热耗散的发生.Graphene, a special two-dimensional material, has a unique band structure that allows the type and concentration of carriers to be controlled through a gate voltage, and it has potential applications in bipolar nanoelectronic devices. In this paper, based on the tight-binding model of graphene p-n junctions, by using the nonequilibrium Green’s function method and Landauer-Büttiker formula, the thermal dissipation of electric transport in graphene p-n junctions in a magnetic field is investigated. Under a strong magnetic field, both sides of the junction are in the quantum Hall regime, thus the topologically protected chiral edge states appear. Intuitively, the topologically protected chiral edge states are dissipationless. However, the results show that thermal dissipation can occur in the quantum Hall regime in graphene junctions in the presence of dissipation sources, although the topologically protected chiral edge states still exist. In clean graphene junctions, thermal dissipation occurs mainly at the edge for the unipolar transport, but it occurs both at the edge and at the interface of the junctions for the bipolar transport. In the presence of disorder, thermal dissipation is significantly enhanced both in the unipolar junction and in the bipolar junction, and it increases with disorder strength increasing. Besides, the energy distribution of electrons at different positions is also studied, which shows that the thermal dissipation always occurs as long as the energy distribution is in nonequilibrium. This indicates that the topology can protect only the propagation direction of electrons, but it can not suppress the occurrence of thermal dissipation.
-
Keywords:
- graphene p-n junctions /
- thermal dissipation /
- quantum transport /
- topology
[1] von Klitzing K, Dorda G, Pepper M 1980 Phys. Rev. Lett. 45 494
Google Scholar
[2] Tsui D C, Stormer H L, Gossard A C 1982 Phys. Rev. Lett. 48 1559
Google Scholar
[3] Qi X L, Zhang S C 2011 Rev. Mod. Phys. 83 1057
Google Scholar
[4] Hasan M Z, Kane C L 2010 Rev. Mod. Phys. 82 3045
Google Scholar
[5] Halperin 1982 Phys. Rev. B 25 2185
Google Scholar
[6] von Klitzing K 2019 Phys. Rev. Lett. 122 200001
Google Scholar
[7] Tang F, Ren Y, Wang P, Zhong R, Schneeloch J, Yang S A, Yang K, Lee P A, Gu G, Qiao Z, Zhang L 2019 Nature 569 537
Google Scholar
[8] Datta S 1995 Electronic Transport in Mesoscopic System (Cambridge: Cambridge University Press) p293
[9] Büttiker M 1988 Phys. Rev. B 38 9375
Google Scholar
[10] Laughlin 1981 Phys. Rev. B 23 5632
Google Scholar
[11] Laughlin 1983 Phys. Rev. Lett. 50 1395
Google Scholar
[12] Halbertal D, Cuppens J, Shalom M B, Embon L, Shadmi N, Anahory Y, Naren H R, Sarkar J, Uri A, Ronen Y, Myasoedov Y, Levitov L S, Joselevich E, Geim A K, Zeldov E 2016 Nature 539 407
Google Scholar
[13] Halbertal D, Shalom M B, Uri A, Bagani K, Meltzer A Y, Marcus I, Myasoedov Y, Birkbeck J, Levitov L S, Geim A K, Zeldov E 2017 Science 358 1303
Google Scholar
[14] Castro Neto A H, Guinea F, Peres N M R, Novoselov K S, Geim A K 2009 Rev. Mod. Phys. 81 109
Google Scholar
[15] Beenakker C W J 2008 Rev. Mod. Phys. 80 1337
Google Scholar
[16] Novoselov K S, Geim A K, Morozov S V, Jiang D, Zhang Y, Dubonos S V, Grigorieva I V, Firsov A A 2004 Science 306 666
Google Scholar
[17] Novoselov K S, Geim A K, Morozov S V, Jiang D, Katsnelson M I, Grigorieva I V, Dubonos S V, Firsov A A 2005 Nature 438 197
Google Scholar
[18] Zhang Y, Tan Y W, Stormer H L, Kim P 2005 Nature 438 201
Google Scholar
[19] Novoselov K S, Jiang Z, Zhang Y, Morozov S V, Stormer H L, Zeitler U, Maan J C, Boebinger G S, Kim P, Geim A K 2007 Science 315 1379
Google Scholar
[20] Semenoff G W 1984 Phys. Rev. Lett. 53 2449
Google Scholar
[21] Gusynin V P, Sharapov S G 2005 Phys. Rev. Lett. 95 146801
Google Scholar
[22] Marguerite A, Birkbeck J, Aharon-Steinberg A, Halbertal D, Bagani K, Marcus I, Myasoedov Y, Geim K, Perello D J, Zeldov E 2019 Nature 575 628
Google Scholar
[23] Zhang G, Gornyi I V, Mirlin A D 2020 Phys. Rev. Res. 2 013337
Google Scholar
[24] Fang J Y, Yang N X, Yan Q, Guo A M, Sun Q F 2021 Phys. Rev. B 104 115411
Google Scholar
[25] Aharon-Steinberg A, Marguerite A, Perello D J, Bagani K, Holder T, Myasoedov Y, Levitov L S, Geim A K, Zeldov E 2021 Nature 593 528
Google Scholar
[26] Anahory Y, Naren H R, Lachman E O, Buhbut Sinai S, Uri A, Embon L, Yaakobi E, Myasoedov Y, Huber M E, Klajn R, Zeldov E 2020 Nanoscale 12 3174
Google Scholar
[27] Huard B, Sulpizio J A, Stander N, Todd K, Yang B, Goldhaber-Gordon D 2007 Phys. Rev. Lett. 98 236803
Google Scholar
[28] Heersche H B, Jarillo-Herrero P, Oostinga J B, Vandersypen L M K, Morpurgo A F 2007 Nature 446 56
Google Scholar
[29] Williams J R, DiCarlo L, Marcus C M 2007 Science 317 638
Google Scholar
[30] Özyilmaz B, Jarillo-Herrero P, Efetov D, Abanin D A, Levitov L S, Kim P 2007 Phys. Rev. Lett. 99 166804
Google Scholar
[31] Katsnelson M I, Novoselov K S, Geim A K 2006 Nat. Phys. 2 620
Google Scholar
[32] Stander N, Huard B, Goldhaber-Gordon D 2009 Phys. Rev. Lett. 102 026807
Google Scholar
[33] Cheianov V V, Fal’ko V, Altshuler B L 2007 Science 315 1252
Google Scholar
[34] Rycerz A, Tworzydlo Beenakker C W J 2007 Nat. Phys. 3 172
Google Scholar
[35] Abanin D A, Levitov L S 2007 Science 317 641
Google Scholar
[36] Lohmann T, von Klitzing K, Smet J H 2009 Nano Lett. 9 1973
Google Scholar
[37] Wang K, Harzheim A, Taniguchi T, Watanabei K, Lee J U, Kim P 2019 Phys. Rev. Lett. 122 146801
Google Scholar
[38] Meir Y, Wingreen N S 1992 Phys. Rev. Lett. 68 2512
Google Scholar
[39] Jauho A P, Wingreen N S, Meir Y 1994 Phys. Rev. B 50 5528
Google Scholar
[40] Büttiker M 1986 Phys. Rev. Lett. 57 1761
Google Scholar
[41] Sheng D N, Sheng L, Weng Z Y 2006 Phys. Rev. B 73 233406
Google Scholar
[42] Sun Q F, Xie X C 2010 Phys. Rev. Lett. 104 066805
Google Scholar
[43] Long W, Sun Q F, Wang J 2008 Phys. Rev. Lett. 101 166806
Google Scholar
[44] Li J, Shen S Q 2008 Phys. Rev. B 78 205308
Google Scholar
[45] Sancho M P L, Sancho J M L, Rubio J 1985 J. Phys. F: Met. Phys. 15 851
Google Scholar
[46] Tian J, Jiang Y, Childres I, Cao H, Hu J, Chen Y P 2013 Phys. Rev. B 88 125410
Google Scholar
[47] Chen J C, Au Yeung T C, Sun Q F 2010 Phys. Rev. B 81 245417
Google Scholar
-
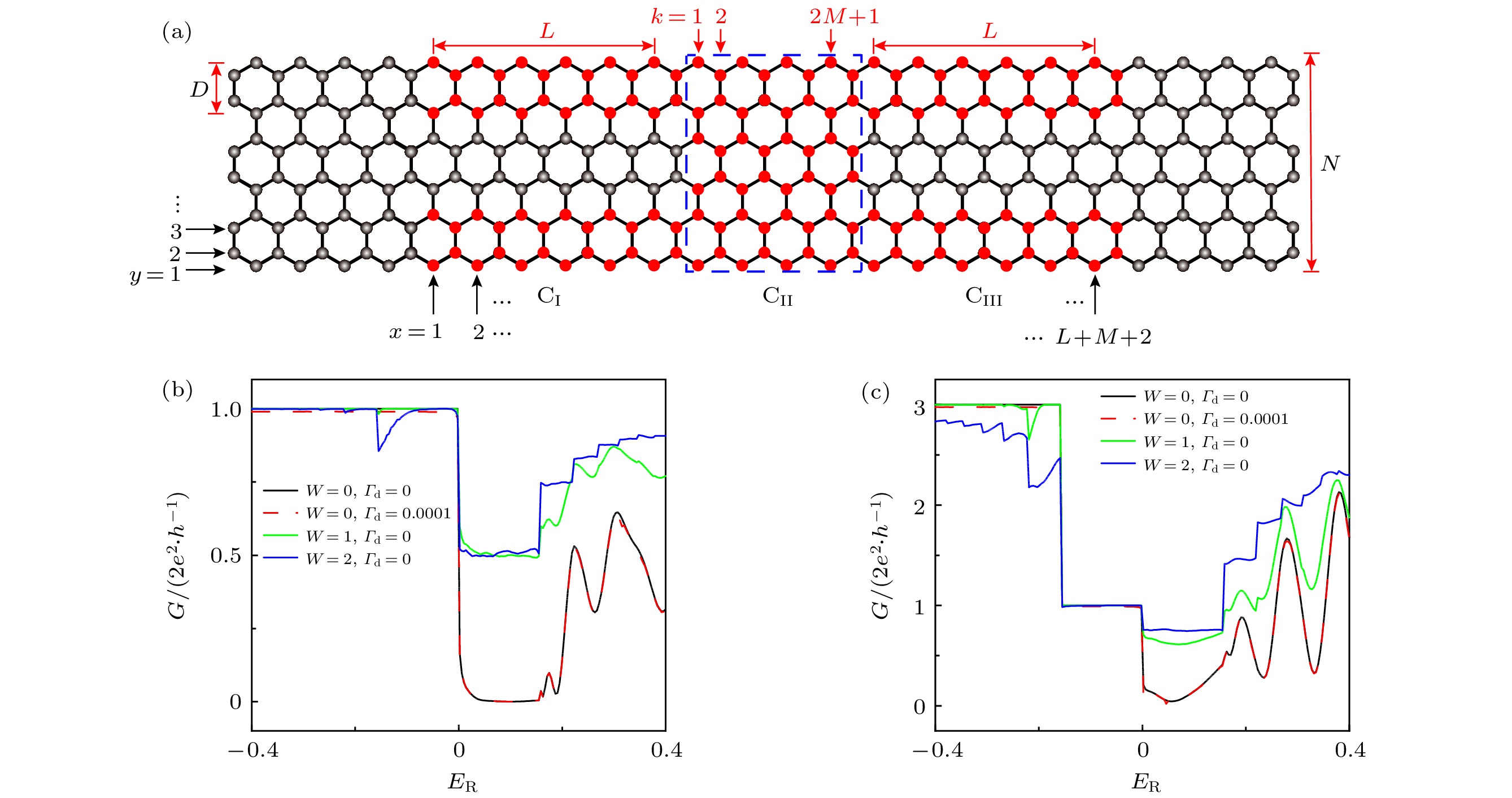
图 1 (a) 锯齿型石墨烯p-n结示意图, 红色格点表示在这些格点上耦合了虚拟导线, 图中
$ D=4, N=12, L=11, M=3 $ ; (b), (c) 不同无序强度W下电导G随着$ {E}_{\mathrm{R}} $ 的变化曲线. 当$ \mathrm{W}\ne 0 $ 时, 无序构型平均取$ 2000 $ 次. 图(b)中$ {E}_{\mathrm{L}}=-0.1 $ ($ {\nu }_{\mathrm{L}}=1 $ ), 图(c)中$ {E}_{\mathrm{L}}=-0.2 $ ($ {\nu }_{\mathrm{L}}=3 $ )Fig. 1. (a) Schematic diagram for a zigzag graphene p-n junction. The red sites indicate that virtual leads are coupled to these sites, and
$ D=4, N=12, L=11, M=3 $ in this diagram. (b), (c) The conductance G vs.$ {E}_{\mathrm{R}} $ for different disorder strengths W. Here the conductance is averaged up to 2000 configurations when$ W\ne 0 $ . The parameters$ {E}_{\mathrm{L}}=-0.1 $ ($ {\nu }_{\mathrm{L}}=1 $ ) in panel (b),$ {E}_{\mathrm{L}}=-0.2 $ ($ {\nu }_{\mathrm{L}}=3 $ ) in panel (c).
图 2 局域热产生
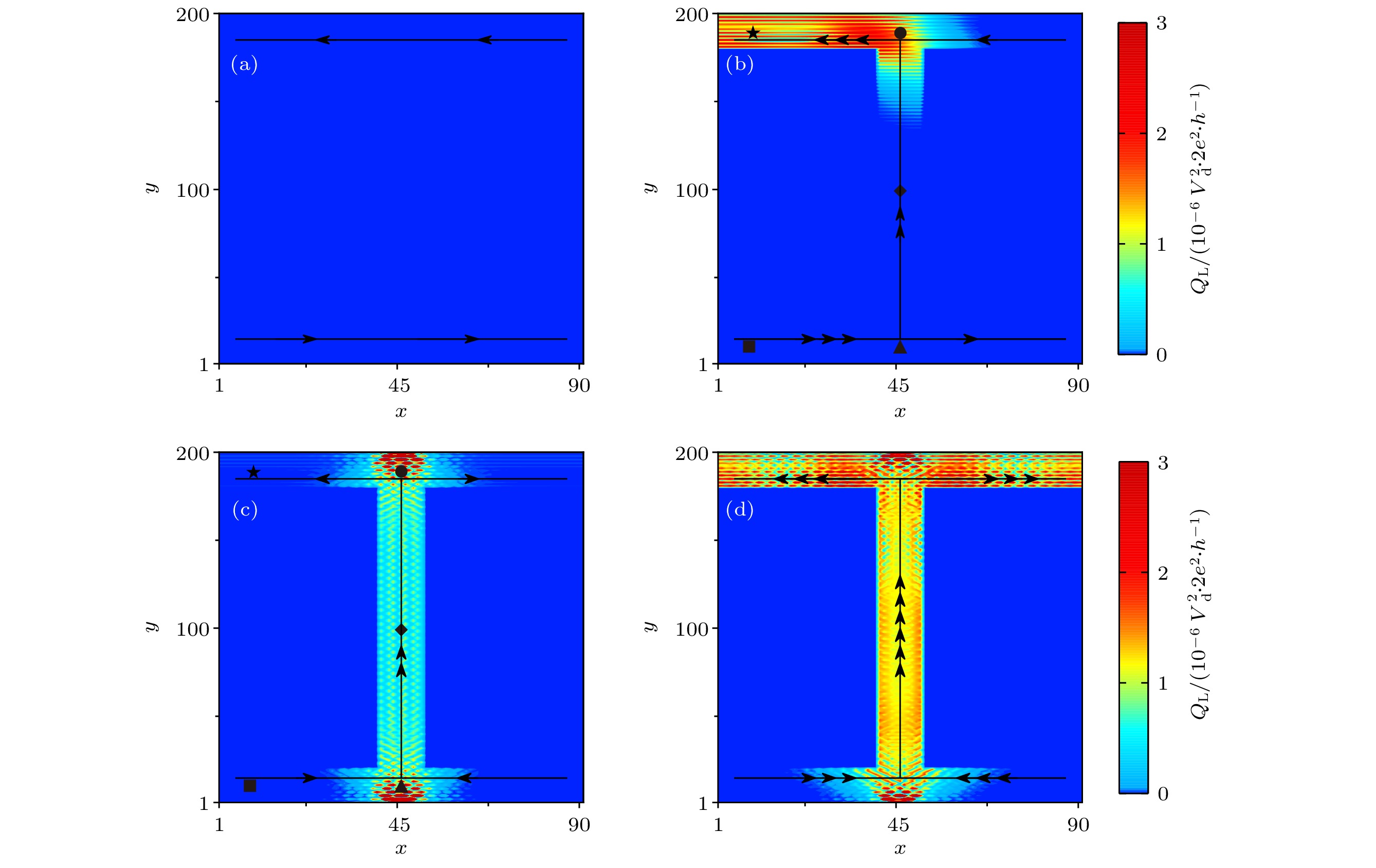
$ {Q}_{\mathrm{L}} $ 随着格点位置坐标$ (x, y) $ 的变化, 带箭头的黑色实线代表手性边缘态的数目和传播方向. 符号■, ▲, ◆, ●, ★标记的位置分别是$ (10, 10) $ ,$ \left(\mathrm{46, 10}\right) $ ,$ \left(\mathrm{46, 100}\right) $ ,$ \left(\mathrm{46, 190}\right) $ ,$ \left(\mathrm{10, 190}\right) $ , 在图4中将给出这些位置上的电子能量分布.$ \left({E}_{\mathrm{L}}, {E}_{\mathrm{R}}\right) $ =$ (-0.1, -0.1) $ (a)$ , (-0.2, -0.1) $ (b),$ (-\mathrm{0.1, 0.1}) $ (c),$ (-\mathrm{0.2, 0.2}) $ (d), 分别对应左右填充因子$ ({\nu }_{\mathrm{L}}, {\nu }_{\mathrm{R}})=\left(\mathrm{1, 1}\right) $ (a),$ \left(\mathrm{3, 1}\right) $ (b),$ (1, -1) $ (c),$ (3, -3) $ (d)Fig. 2. Local heat generation
$ {Q}_{\mathrm{L}} $ vs. lattice position$ (x, y) $ , the black solid lines with arrows represent the number and propagation direction of the chiral edge states. Positions marked by symbols ■, ▲, ◆, ●, ★ are, respectively,$ \left(\mathrm{10, 10}\right) $ ,$ \left(\mathrm{46, 10}\right) $ ,$ \left(\mathrm{46, 100}\right) $ ,$ \left(\mathrm{46, 190}\right) $ ,$ \left(\mathrm{10, 190}\right) $ , and energy distribution of electrons at these positions will be shown in Fig. 4.$ \left({E}_{\mathrm{L}}, {E}_{\mathrm{R}}\right)=(-0.1, -0.1) $ (a),$ (-0.2, -0.1) $ (b),$ (-\mathrm{0.1, 0.1}) $ (c),$ (-\mathrm{0.2, 0.2}) $ (d), which correspond to the left and right filling factors$ ({\nu }_{\mathrm{L}}, {\nu }_{\mathrm{R}})=\left(\mathrm{1, 1}\right) $ (a),$ \left(\mathrm{3, 1}\right) $ (b),$ (1, -1) $ (c), and$ (3, -3) $ (d), respectively.
图 3 局域热产生
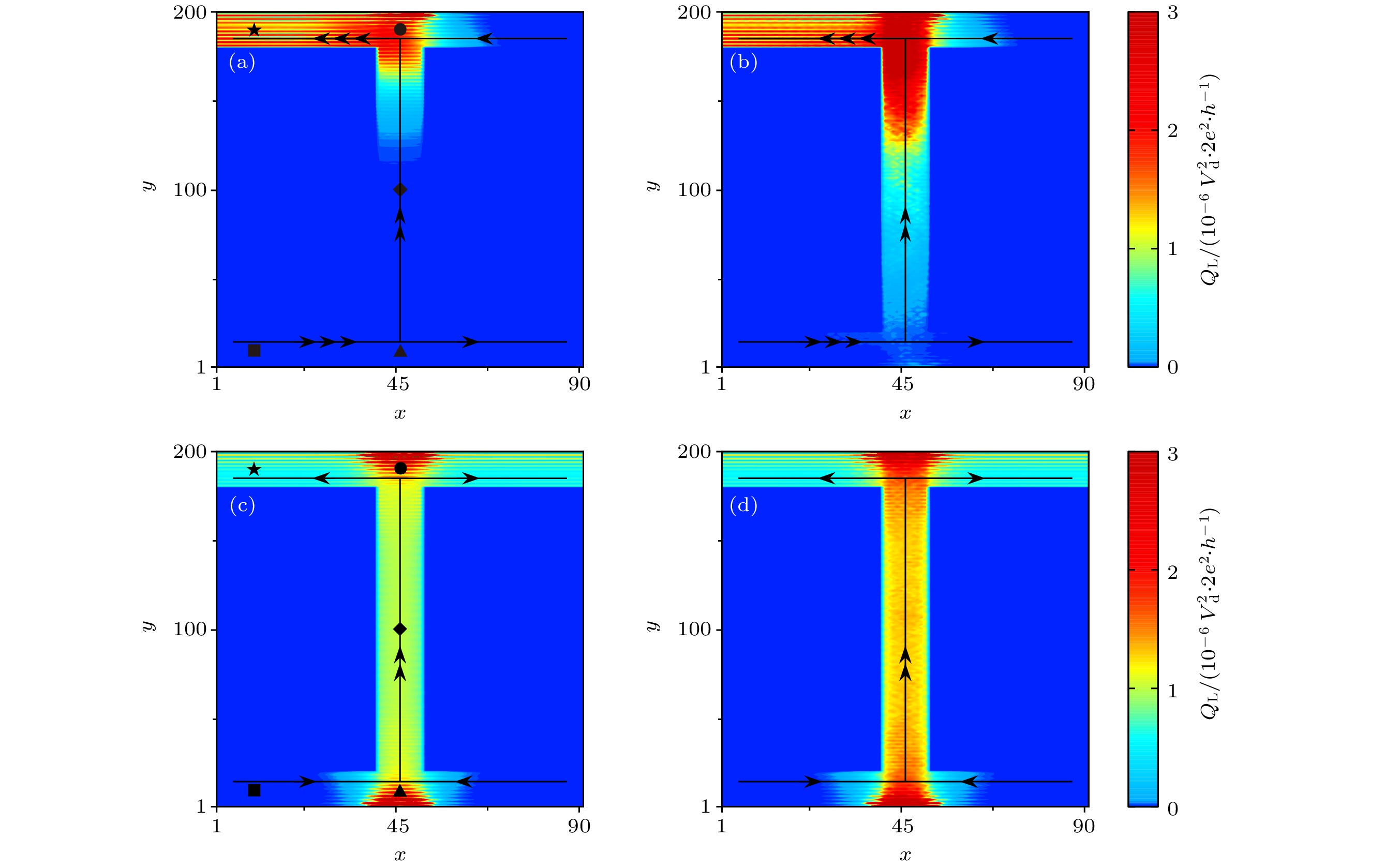
$ {Q}_{\mathrm{L}} $ 随着格点位置坐标$ (x, y) $ 的变化, 带箭头的黑色实线代表手性边缘态的数目和传播方向. 符号■, ▲, ◆, ●, ★标记的位置分别是$ \left(\mathrm{10, 10}\right) $ ,$ \left(\mathrm{46, 10}\right) $ ,$ \left(\mathrm{46, 100}\right) $ ,$ \left(\mathrm{46, 190}\right) $ ,$ \left(\mathrm{10, 190}\right) $ , 在图4中将给出这些位置上的电子能量分布. (a), (b)$ \left({E}_{\mathrm{L}}, {E}_{\mathrm{R}}\right)=(-0.2, -0.1) $ ;$ \left(\mathrm{c}\right), \left(\mathrm{d}\right) $ $ \left({E}_{\mathrm{L}}, {E}_{\mathrm{R}}\right)= (-\mathrm{0.1, 0.1}) $ . (a), (c) 无序强度$ W=1 $ ; (b), (d) 无序强度$ W= $ 2. 无序构型平均取$ 400 $ 次Fig. 3. Local heat generation
$ {Q}_{\mathrm{L}} $ vs. lattice position$ (x, y) $ , the black solid lines with arrows represent the number and propagation direction of the chiral edge states. Positions marked by symbols ■, ▲, ◆, ●, ★ are$ \left(\mathrm{10, 10}\right) $ ,$ \left(\mathrm{46, 10}\right) $ ,$ \left(\mathrm{46, 100}\right) $ ,$ \left(\mathrm{46, 190}\right) $ ,$ \left(\mathrm{10, 190}\right) $ , respectively, and energy distribution of electrons at these positions will be shown in Fig. 4. (a), (b)$\left({E}_{\mathrm{L}}, {E}_{\mathrm{R}}\right)= $ $ (-0.2, -0.1)$ ; (c), (d)$ \left({E}_{\mathrm{L}}, {E}_{\mathrm{R}}\right)=(-\mathrm{0.1, 0.1}) $ . (a), (c) Disorder strength$ W=1 $ ; (b) (d)$ W= $ 2. Here the local heat generation is averaged up to 400 configurations.
图 4 不同位置处的分布函数F随着能量E的变化, 符号■, ▲, ◆, ●, ★对应图2和图3中标记的位置. (a), (b)
$\left({E}_{\mathrm{L}}, {E}_{\mathrm{R}}\right)= $ $ (-0.2, -0.1)$ ; (c), (d)$ (-\mathrm{0.1, 0.1}) $ . (a), (c) 无序强度$ W=0 $ ; (b), (d)$ W=1 $ . 当$ \mathrm{W}\ne 0 $ 时, 无序构型平均取$ 400 $ 次Fig. 4. Distribution function F vs. energy E for different positions, the symbols ■, ▲, ◆, ●, ★ correspond to positions marked in Fig. 2 and Fig. 3. (a), (b)
$ \left({E}_{\mathrm{L}}, {E}_{\mathrm{R}}\right)=(-0.2, -0.1) $ ; (c), (d)$ \left({E}_{\mathrm{L}}, {E}_{\mathrm{R}}\right)=(-\mathrm{0.1, 0.1}) $ . (a), (c) Disorder strength$ W=0 $ ; (b), (d)$ W= $ 1. Here distribution function F is averaged up to 400 configurations when$ W\ne 0 $ . -
[1] von Klitzing K, Dorda G, Pepper M 1980 Phys. Rev. Lett. 45 494
Google Scholar
[2] Tsui D C, Stormer H L, Gossard A C 1982 Phys. Rev. Lett. 48 1559
Google Scholar
[3] Qi X L, Zhang S C 2011 Rev. Mod. Phys. 83 1057
Google Scholar
[4] Hasan M Z, Kane C L 2010 Rev. Mod. Phys. 82 3045
Google Scholar
[5] Halperin 1982 Phys. Rev. B 25 2185
Google Scholar
[6] von Klitzing K 2019 Phys. Rev. Lett. 122 200001
Google Scholar
[7] Tang F, Ren Y, Wang P, Zhong R, Schneeloch J, Yang S A, Yang K, Lee P A, Gu G, Qiao Z, Zhang L 2019 Nature 569 537
Google Scholar
[8] Datta S 1995 Electronic Transport in Mesoscopic System (Cambridge: Cambridge University Press) p293
[9] Büttiker M 1988 Phys. Rev. B 38 9375
Google Scholar
[10] Laughlin 1981 Phys. Rev. B 23 5632
Google Scholar
[11] Laughlin 1983 Phys. Rev. Lett. 50 1395
Google Scholar
[12] Halbertal D, Cuppens J, Shalom M B, Embon L, Shadmi N, Anahory Y, Naren H R, Sarkar J, Uri A, Ronen Y, Myasoedov Y, Levitov L S, Joselevich E, Geim A K, Zeldov E 2016 Nature 539 407
Google Scholar
[13] Halbertal D, Shalom M B, Uri A, Bagani K, Meltzer A Y, Marcus I, Myasoedov Y, Birkbeck J, Levitov L S, Geim A K, Zeldov E 2017 Science 358 1303
Google Scholar
[14] Castro Neto A H, Guinea F, Peres N M R, Novoselov K S, Geim A K 2009 Rev. Mod. Phys. 81 109
Google Scholar
[15] Beenakker C W J 2008 Rev. Mod. Phys. 80 1337
Google Scholar
[16] Novoselov K S, Geim A K, Morozov S V, Jiang D, Zhang Y, Dubonos S V, Grigorieva I V, Firsov A A 2004 Science 306 666
Google Scholar
[17] Novoselov K S, Geim A K, Morozov S V, Jiang D, Katsnelson M I, Grigorieva I V, Dubonos S V, Firsov A A 2005 Nature 438 197
Google Scholar
[18] Zhang Y, Tan Y W, Stormer H L, Kim P 2005 Nature 438 201
Google Scholar
[19] Novoselov K S, Jiang Z, Zhang Y, Morozov S V, Stormer H L, Zeitler U, Maan J C, Boebinger G S, Kim P, Geim A K 2007 Science 315 1379
Google Scholar
[20] Semenoff G W 1984 Phys. Rev. Lett. 53 2449
Google Scholar
[21] Gusynin V P, Sharapov S G 2005 Phys. Rev. Lett. 95 146801
Google Scholar
[22] Marguerite A, Birkbeck J, Aharon-Steinberg A, Halbertal D, Bagani K, Marcus I, Myasoedov Y, Geim K, Perello D J, Zeldov E 2019 Nature 575 628
Google Scholar
[23] Zhang G, Gornyi I V, Mirlin A D 2020 Phys. Rev. Res. 2 013337
Google Scholar
[24] Fang J Y, Yang N X, Yan Q, Guo A M, Sun Q F 2021 Phys. Rev. B 104 115411
Google Scholar
[25] Aharon-Steinberg A, Marguerite A, Perello D J, Bagani K, Holder T, Myasoedov Y, Levitov L S, Geim A K, Zeldov E 2021 Nature 593 528
Google Scholar
[26] Anahory Y, Naren H R, Lachman E O, Buhbut Sinai S, Uri A, Embon L, Yaakobi E, Myasoedov Y, Huber M E, Klajn R, Zeldov E 2020 Nanoscale 12 3174
Google Scholar
[27] Huard B, Sulpizio J A, Stander N, Todd K, Yang B, Goldhaber-Gordon D 2007 Phys. Rev. Lett. 98 236803
Google Scholar
[28] Heersche H B, Jarillo-Herrero P, Oostinga J B, Vandersypen L M K, Morpurgo A F 2007 Nature 446 56
Google Scholar
[29] Williams J R, DiCarlo L, Marcus C M 2007 Science 317 638
Google Scholar
[30] Özyilmaz B, Jarillo-Herrero P, Efetov D, Abanin D A, Levitov L S, Kim P 2007 Phys. Rev. Lett. 99 166804
Google Scholar
[31] Katsnelson M I, Novoselov K S, Geim A K 2006 Nat. Phys. 2 620
Google Scholar
[32] Stander N, Huard B, Goldhaber-Gordon D 2009 Phys. Rev. Lett. 102 026807
Google Scholar
[33] Cheianov V V, Fal’ko V, Altshuler B L 2007 Science 315 1252
Google Scholar
[34] Rycerz A, Tworzydlo Beenakker C W J 2007 Nat. Phys. 3 172
Google Scholar
[35] Abanin D A, Levitov L S 2007 Science 317 641
Google Scholar
[36] Lohmann T, von Klitzing K, Smet J H 2009 Nano Lett. 9 1973
Google Scholar
[37] Wang K, Harzheim A, Taniguchi T, Watanabei K, Lee J U, Kim P 2019 Phys. Rev. Lett. 122 146801
Google Scholar
[38] Meir Y, Wingreen N S 1992 Phys. Rev. Lett. 68 2512
Google Scholar
[39] Jauho A P, Wingreen N S, Meir Y 1994 Phys. Rev. B 50 5528
Google Scholar
[40] Büttiker M 1986 Phys. Rev. Lett. 57 1761
Google Scholar
[41] Sheng D N, Sheng L, Weng Z Y 2006 Phys. Rev. B 73 233406
Google Scholar
[42] Sun Q F, Xie X C 2010 Phys. Rev. Lett. 104 066805
Google Scholar
[43] Long W, Sun Q F, Wang J 2008 Phys. Rev. Lett. 101 166806
Google Scholar
[44] Li J, Shen S Q 2008 Phys. Rev. B 78 205308
Google Scholar
[45] Sancho M P L, Sancho J M L, Rubio J 1985 J. Phys. F: Met. Phys. 15 851
Google Scholar
[46] Tian J, Jiang Y, Childres I, Cao H, Hu J, Chen Y P 2013 Phys. Rev. B 88 125410
Google Scholar
[47] Chen J C, Au Yeung T C, Sun Q F 2010 Phys. Rev. B 81 245417
Google Scholar
下载:
计量
- 文章访问数: 4571
- PDF下载量: 206
- 被引次数: 0
