-
晶界控制的调幅分解对材料微观组织及性能有着十分重要的影响, 然而, 限于研究手段, 我们对晶界与调幅分解间相互作用过程及机制的认识仍存在不足. 本文采用相场法模拟了实际多晶体系的调幅分解过程, 研究了晶界曲率及晶界处原子扩散速率对调幅组织形貌的影响, 并讨论了调幅分解与晶界迁移的相互作用关系. 结果表明, 晶界能够促进并调制调幅组织形貌, 晶界附近为各向异性调幅组织, 晶粒内部为各向同性双连通调幅组织; 随着晶界曲率增大, 调幅组织由垂直晶界转变为平行晶界; 调幅分解速度随着晶界原子扩散系数的增大而增大, 而调幅分解过程中的晶界迁移速度则随着晶界原子扩散系数的增大表现为先减小后增大; 三维模拟结果与二维模拟结果相一致.The grain boundary-directed spinodal decomposition has a substantial effect on the microstructure and properties of polycrystalline materials. However, due to the fact that the spinodal decomposition is usually too fast to be captured in experiments, our understanding of the grain boundary-directed spinodal decomposition process is still very limited. In this work, we simulate the spinodal decomposition process of a polycrystalline system by the phase-field model, check the influences of the curvature and the atom diffusion constant inside the grain boundary (Mt) on the phase decomposition patterns, and discuss the interaction between the moving grain boundaries and spinodal decomposition. The simulation results indicate that the velocity of spinodal decomposition near the grain boundary is faster, and the spinodal morphology at the grain boundary presents the anisotropic bicontinuous microstructures different from the isotropic continuous microstructures in the bulk phase. Further, we find that the spinodal pattern is parallel to the grain boundaries with larger curvatures, and it will perpendicular to the grain boundaries with smaller curvatures. We also find that the spinodal decomposition velocity increases with the augment of Mt , while the grain boundary migration velocity will first decrease and then increase with the augment of Mt under the effect of spinodal decomposition. Finally, we simulate the spinodal decomposition process of two-grain system in three dimensions, and we obtain the results consistent with the two-dimensional simulations.
-
Keywords:
- spinodal decomposition /
- grain boundary /
- curvature /
- grain boundary migration
[1] Cahn J W, Hilliard J E 1958 J. Chem. Phys. 28 258
Google Scholar
[2] Liu X, Li R, Lu Y, Zhang Y, Yu P, Li G 2021 Mater. Sci. Eng. A 822 141674
Google Scholar
[3] Peng Y, Wang N 2020 J. Mater. Sci. Technol. 38 64
Google Scholar
[4] Das A, Basak C B 2018 Philos. Mag. 98 3007
Google Scholar
[5] Rajeshwari K S, Sankaran S, Hari Kumar K C, Rösner H, Peterlechner M, Esin V A, Divinski S, Wilde G 2020 Acta Mater. 195 501
Google Scholar
[6] Grönhagen K, Ågren J, Odén M 2015 Scr. Mater. 95 42
Google Scholar
[7] Wise S M, Kim J S, Johnson W C 2005 Thin Solid Films 473 151
Google Scholar
[8] Li L, Li Z, Kwiatkowski da Silva A, Peng Z, Zhao H, Gault B, Raabe D 2019 Acta Mater. 178 1
Google Scholar
[9] Liu J, Wu X, Lennard W N, Landheer D, Dharma-Wardana M W C 2010 J. Appl. Phys. 107 123510
Google Scholar
[10] Li Y S, Li S X, Zhang T Y 2009 J. Nucl. Mater. 395 120
Google Scholar
[11] Li Y, Katsui H, Goto T 2017 Mater. Today:Proc. 4 11449
Google Scholar
[12] Deng Y Y, Guo C, Wang J C, Liu Q, Zhao Y P, Yang Q 2021 Chin. Phys. B 30 088101
Google Scholar
[13] 孙佳, 李学雄, 张金虎, 王刚, 杨梅, 王皞, 徐东生 2020 金属学报 56 1113
Sun J, Li X X, Zhang J H, Wang G, Yang M, Wang H, Xu D S 2020 Acta Metall. Sin. 56 1113
[14] 王锦程, 郭春文, 李俊杰, 王志军 2018 金属学报 54 657
Google Scholar
Wang J C, Guo C W, Li J J, Wang Z J 2018 Acta Metall. Sin. 54 657
Google Scholar
[15] Guo C, Kang C, Xu C, Wang J 2021 Comput. Mater. Sci. 196 110536
Google Scholar
[16] Guo C, Wang J, Li J, Wang Z, Huang Y, Gu J, Lin X 2018 Acta Mater. 145 175
Google Scholar
[17] Tu Z, Zhou J, Tong L, Guo Z 2020 J. Cryst. Growth 532 125439
Google Scholar
[18] 祁科武, 赵宇宏, 田晓林, 彭敦维, 孙远洋, 侯华 2020 69 140504
Google Scholar
Qi K W, Zhao Y H, Tian X L, Peng D W, Sun Y Y, Hou H 2020 Acta Phys. Sin. 69 140504
Google Scholar
[19] Zhu C S, Hu Z, Wang K M 2020 Chin. Phys. B 29 034702
Google Scholar
[20] Ramanarayan H, Abinandanan T A 2003 Acta Mater. 51 4761
Google Scholar
[21] Razumov I K, Gornostyrev Y N, Yermakov A Y 2007 J. Alloys Compd. 434–435 535
[22] Yang T, Chen Z, Zhang J, Dong W P, Wu L 2012 Chin. Phys. Lett. 29 078103
[23] Seol D J, Hu S Y, Li Y L, Shen J, Oh K H, Chen L Q 2003 Acta Mater. 51 5173
Google Scholar
[24] Lee J, Chang K 2019 Comput. Mater. Sci. 169 109088
Google Scholar
[25] Guo C, Wang J, Wang Z, Li J, Guo Y, Huang Y 2016 Soft Matter 12 4666
Google Scholar
[26] Kwiatkowski da Silva A, Kamachali R D, Ponge D, Gault B, Neugebauer J, Raabe D 2019 Acta Mater. 168 109
Google Scholar
[27] 郭耀麟 2015 博士学位论文 (西安: 西北工业大学)
Guo Y L 2015 Ph. D. Dissertation (Xi'an: Northwestern Polytechnical University) (in Chinese)
-
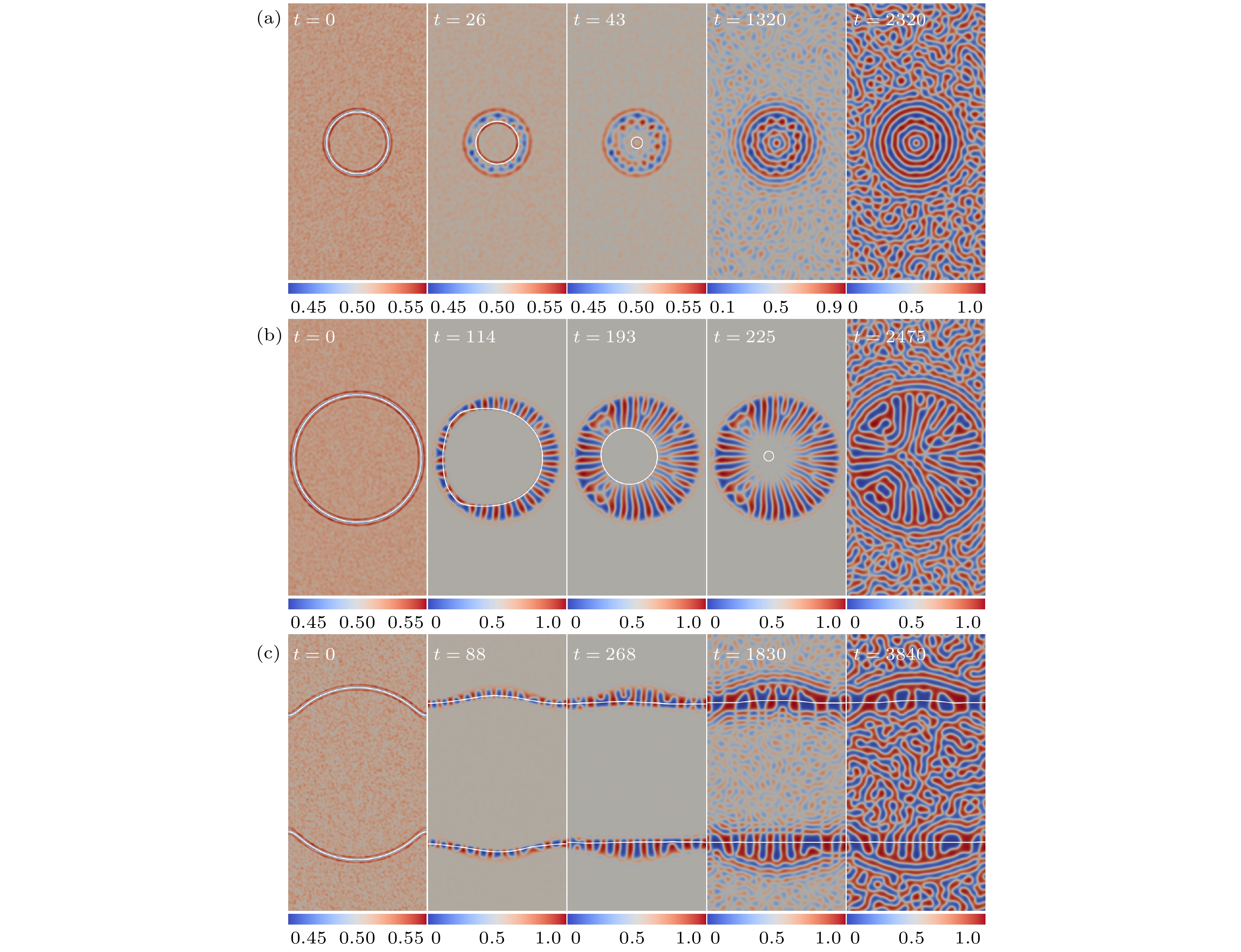
图 1 不同时刻多晶粒调幅分解过程 (a) t = 0; (b) t = 40; (c) t = 100; (d) t = 240; (e) t = 705; (f) t = 1805. 图中白色线条为晶界, 蓝色与红色区域分别代表相分离后的α相和β相
Fig. 1. The snapshots of the spinodal decomposition process of a polycrystalline system: (a) t = 0; (b) t = 40; (c) t = 100; (d) t = 240; (e) t = 705; (f) t = 1805. Note, the white line is the grain boundary, blue and red regions represent α and β phases, respectively.
图 2 不同初始曲率半径条件下的调幅分解过程 (a) r = 30; (b) r = 60; (c) r = 70
Fig. 2. Snapshots of the spinodal decomposition processes with different radius of curvatures: (a) r = 30; (b) r = 70; (c) r = 70.
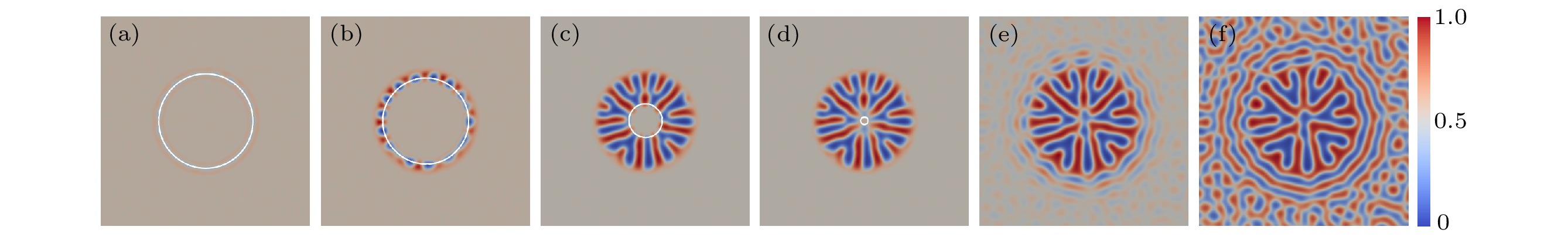
图 3 初始晶粒半径为30, 晶界原子扩散常数Mt = 5000时的调幅分解过程 (a) t = 0; (b) t = 10; (c) t = 36; (d) t = 41; (e) t = 1062; (f) t = 2008
Fig. 3. The snapshots of the spinodal decomposition processes with initial r = 30 (radius of curvature) and Mt = 5000 (the diffusional constant inside the grain boundary): (a) t = 0; (b) t = 10; (c) t = 36; (d) t = 41; (e) t = 1062; (f) t = 2008.
图 4 不同Mt条件下晶界迁移速率(V)与晶界半径倒数(1/r)间的关系曲线
Fig. 4. The grain boundary migration velocity (V) versus the grain boundary curvature (1/r) with different Mt.
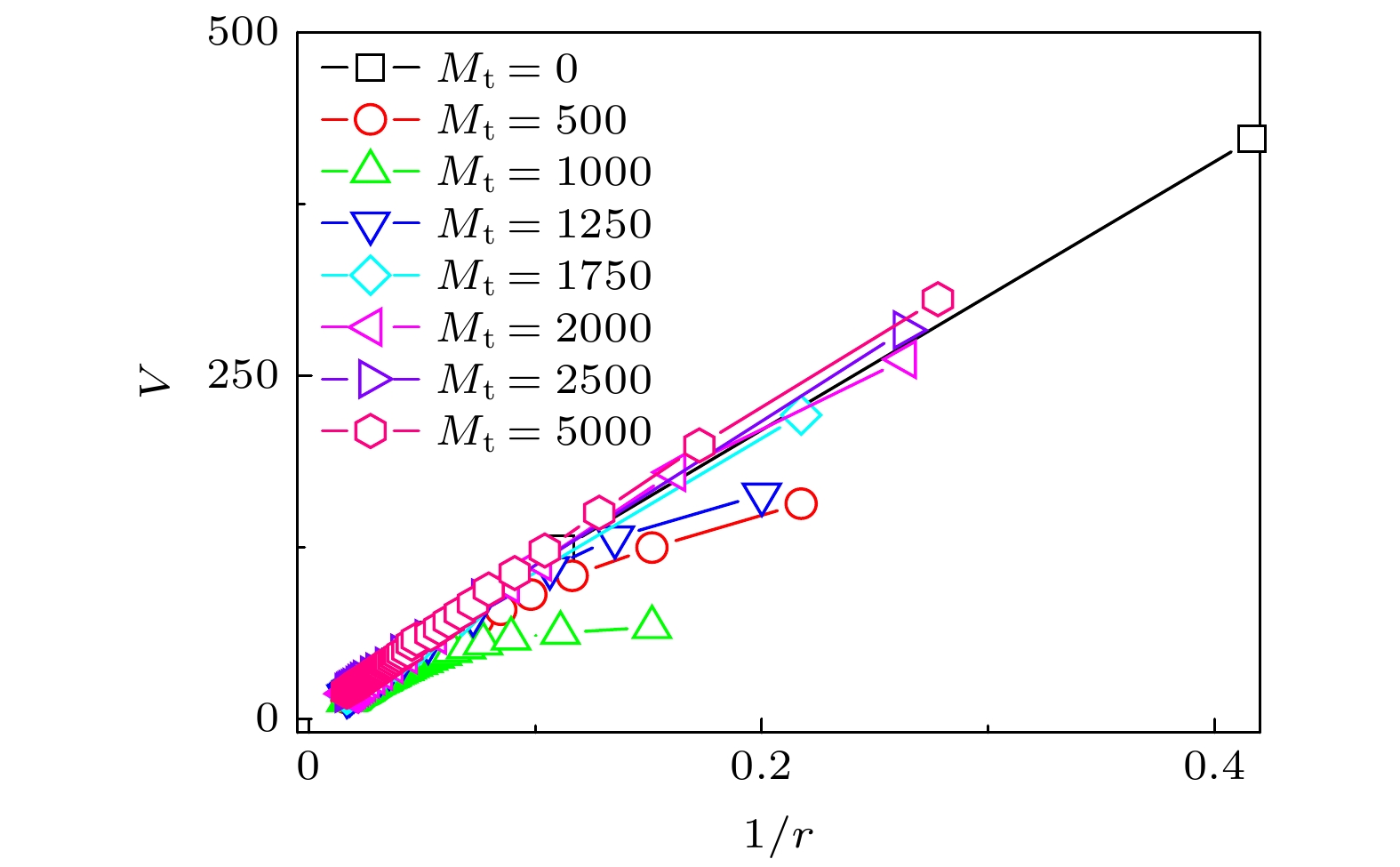
图 5 不同扩散系数(Mt)条件下穿过曲率中心某直线上的成分场随时间演化曲线, 图中红色曲线代表中心晶粒成分场, 黑色曲线代表外部晶粒成分场
Fig. 5. Temporal evolution of the composition fields along the line that across the center of the grain with different initial Mt, where the red and black lines represent the composition field of central grain and external grain, respectively.
图 6 中心晶粒半径为35, 不同Mt条件下双晶体系调幅分解过程 (a) Mt = 5000; (b) Mt = 500. 其中, 每组图片中上一行为三维视图, 第二行为x = 40Δx处的二维视图, 灰色球面为晶界
Fig. 6. Snapshots of the spinodal decomposition processes with different Mt for r = 35: (a) Mt = 5000; (b) Mt = 500. Where the 5 figures in the upper row of Fig. 6(a) and Fig. 6(b) are the time evolution of the composition field in three-dimension, the 5 figures in the lower row of Fig. 6(a) and Fig. 6(b) are the two-dimensional cross-sectional view of the composition field at x = 40Δx, and the gray sphere is the grain boundary.
-
[1] Cahn J W, Hilliard J E 1958 J. Chem. Phys. 28 258
Google Scholar
[2] Liu X, Li R, Lu Y, Zhang Y, Yu P, Li G 2021 Mater. Sci. Eng. A 822 141674
Google Scholar
[3] Peng Y, Wang N 2020 J. Mater. Sci. Technol. 38 64
Google Scholar
[4] Das A, Basak C B 2018 Philos. Mag. 98 3007
Google Scholar
[5] Rajeshwari K S, Sankaran S, Hari Kumar K C, Rösner H, Peterlechner M, Esin V A, Divinski S, Wilde G 2020 Acta Mater. 195 501
Google Scholar
[6] Grönhagen K, Ågren J, Odén M 2015 Scr. Mater. 95 42
Google Scholar
[7] Wise S M, Kim J S, Johnson W C 2005 Thin Solid Films 473 151
Google Scholar
[8] Li L, Li Z, Kwiatkowski da Silva A, Peng Z, Zhao H, Gault B, Raabe D 2019 Acta Mater. 178 1
Google Scholar
[9] Liu J, Wu X, Lennard W N, Landheer D, Dharma-Wardana M W C 2010 J. Appl. Phys. 107 123510
Google Scholar
[10] Li Y S, Li S X, Zhang T Y 2009 J. Nucl. Mater. 395 120
Google Scholar
[11] Li Y, Katsui H, Goto T 2017 Mater. Today:Proc. 4 11449
Google Scholar
[12] Deng Y Y, Guo C, Wang J C, Liu Q, Zhao Y P, Yang Q 2021 Chin. Phys. B 30 088101
Google Scholar
[13] 孙佳, 李学雄, 张金虎, 王刚, 杨梅, 王皞, 徐东生 2020 金属学报 56 1113
Sun J, Li X X, Zhang J H, Wang G, Yang M, Wang H, Xu D S 2020 Acta Metall. Sin. 56 1113
[14] 王锦程, 郭春文, 李俊杰, 王志军 2018 金属学报 54 657
Google Scholar
Wang J C, Guo C W, Li J J, Wang Z J 2018 Acta Metall. Sin. 54 657
Google Scholar
[15] Guo C, Kang C, Xu C, Wang J 2021 Comput. Mater. Sci. 196 110536
Google Scholar
[16] Guo C, Wang J, Li J, Wang Z, Huang Y, Gu J, Lin X 2018 Acta Mater. 145 175
Google Scholar
[17] Tu Z, Zhou J, Tong L, Guo Z 2020 J. Cryst. Growth 532 125439
Google Scholar
[18] 祁科武, 赵宇宏, 田晓林, 彭敦维, 孙远洋, 侯华 2020 69 140504
Google Scholar
Qi K W, Zhao Y H, Tian X L, Peng D W, Sun Y Y, Hou H 2020 Acta Phys. Sin. 69 140504
Google Scholar
[19] Zhu C S, Hu Z, Wang K M 2020 Chin. Phys. B 29 034702
Google Scholar
[20] Ramanarayan H, Abinandanan T A 2003 Acta Mater. 51 4761
Google Scholar
[21] Razumov I K, Gornostyrev Y N, Yermakov A Y 2007 J. Alloys Compd. 434–435 535
[22] Yang T, Chen Z, Zhang J, Dong W P, Wu L 2012 Chin. Phys. Lett. 29 078103
[23] Seol D J, Hu S Y, Li Y L, Shen J, Oh K H, Chen L Q 2003 Acta Mater. 51 5173
Google Scholar
[24] Lee J, Chang K 2019 Comput. Mater. Sci. 169 109088
Google Scholar
[25] Guo C, Wang J, Wang Z, Li J, Guo Y, Huang Y 2016 Soft Matter 12 4666
Google Scholar
[26] Kwiatkowski da Silva A, Kamachali R D, Ponge D, Gault B, Neugebauer J, Raabe D 2019 Acta Mater. 168 109
Google Scholar
[27] 郭耀麟 2015 博士学位论文 (西安: 西北工业大学)
Guo Y L 2015 Ph. D. Dissertation (Xi'an: Northwestern Polytechnical University) (in Chinese)
下载:
计量
- 文章访问数: 5764
- PDF下载量: 109
- 被引次数: 0
