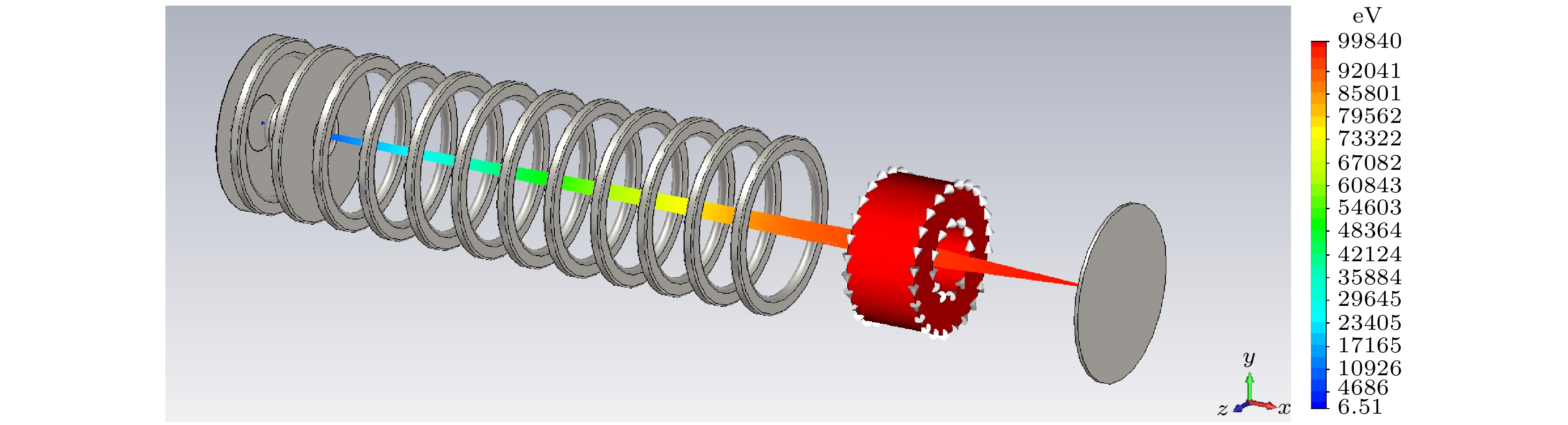
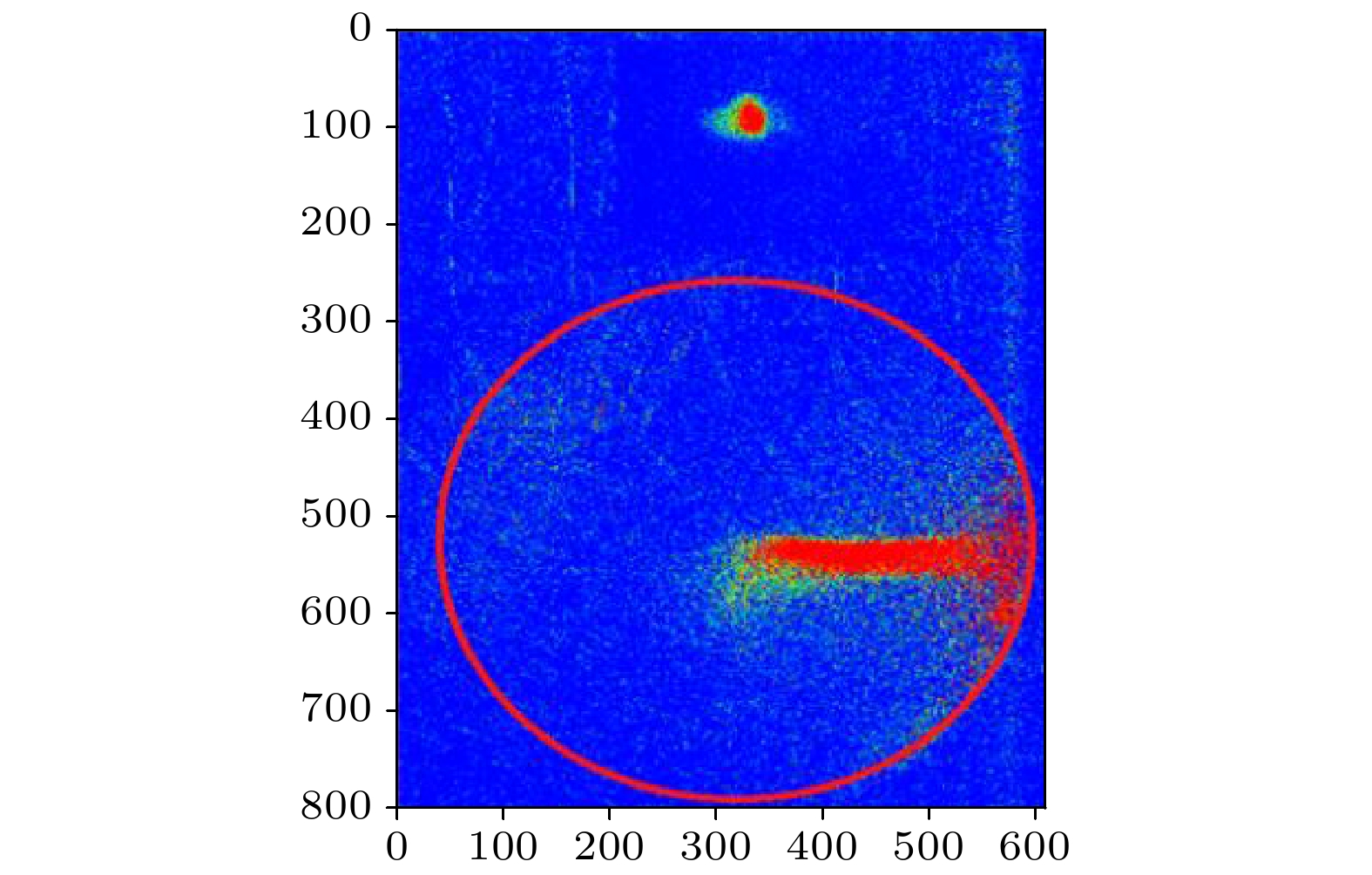
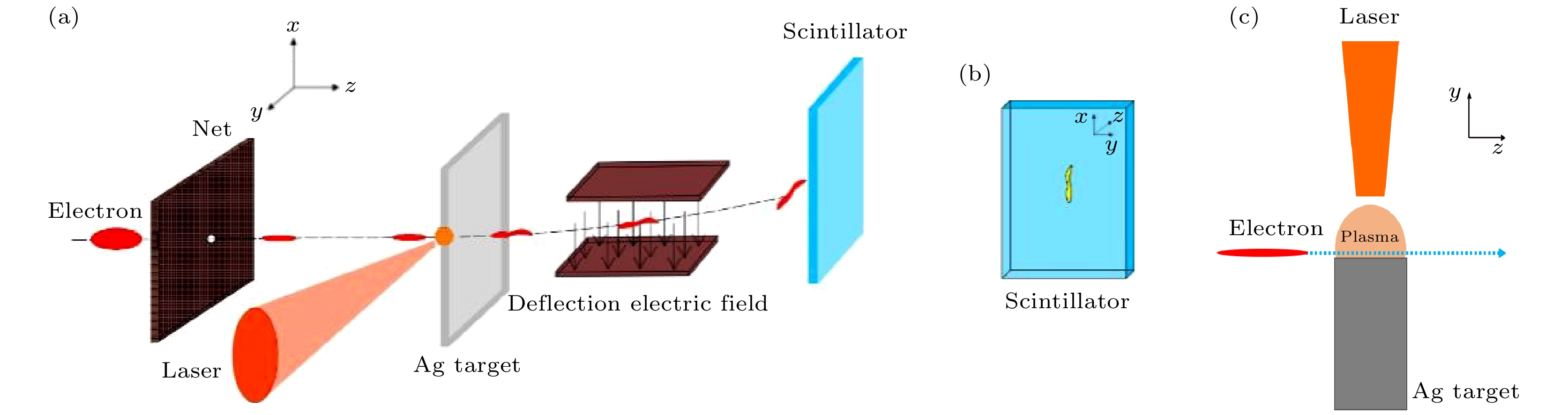
-
惯性约束核聚变研究最近取得可喜成果, 美国国家点火装置NIF装置实验上聚变增益达到了输入激光能量的三分之二. 但是, 这一成果与人们的预期还有较大差距, 需要更深入研究激光与等离子体相互作用初期的动力学过程. 我们发展了一种新方法, 用单发长脉冲电子束团为探针, 测量激光等离子体内电磁场在整个等离子体持续时间内的演化过程. 实验中, 高压静电电子源产生能量0—100 keV 连续可调、脉宽10ns的电子束团. 1 J, 532 nm, 脉宽约4 ns的激光脉冲聚焦到银靶上, 激发产生等离子体. 电子束团穿过激光等离子体, 被其中的电磁场调制后成像, 单发电子束团时间宽度会覆盖整个等离子体持续时间, 通过分析电子束团的调制强度, 推得等离子体内电磁场的变化. 实验上成功实现了单发电子束团对整个激光等离子体内电场的诊断测量, 获得了演化曲线, 推算出实验条件下电子束通过路径上平均电场的最大值约为
$ 7.74\times {10}^{5}\;\mathrm{V}/\mathrm{m} $ .Laser fusion research needs much more high-time-resolved diagnostic technologies to study the dynamic process in laser plasma. We develop a special method and setup a device to measure the electromagnetic field in the plasma by using a single electron bunch. The measurement covers the whole-time window of the plasma process driven by a 3.6 ns laser pulse. An electron source can generate a single electron bunch with 0–100 keV energy and 10ns bunch length. A laser pulse with 1 J energy and 532 nm wavelength irradiates on the edge of a silver target, the target nearby the irradiated spot is ionized into plasma. At the beginning of plasma generation, the head of the electron beam begins to pass through the plasma. Electromagnetic field in plasma pushes the electrons transversely. A high voltage pulse at a good time is used to deflect the electrons linearly in the transverse direction to avoid overlapping of the different electrons on the scintillator downstream. By analyzing the deflection distances of the different electrons in this single bunch, we succesfully achieve an average electronic field along the trajectory in the plasma in the whole plasma process. The maximum value of this electronic field is$ 7.74\times {10}^{5}\;\mathrm{V}/\mathrm{m} $ .-
Keywords:
- electron beam /
- laser Plasma /
- electromagnetic field /
- synchronization
[1] Lindl J D, Hammel B A, Logan B G, Meyerhofer D D, Payne S A, Sethian J D 2003 Plasma Phys. Controlled Fusion 45 A217
Google Scholar
[2] Edwards C B, Danson C N 2015 High Power Laser Sci. Eng. 3 e4
Google Scholar
[3] Zohuri B 2017 Inertial Confinement Fusion Driven Thermonuclear Energy (Albuquerque: Springer International Publishing) pp133−192
[4] Craxton R S, Anderson K S, Boehly T R, Goncharov V N, Harding D R, Knauer J P, Mccrory R L, Mckenty P W, Meyerhofer D D, Myatt J F 2015 Phys. Plasmas 22 139
[5] 王天泽, 雷弘毅, 孙方正, 王丹, 廖国前, 李玉同 2021 70 085205
Google Scholar
Wang T Z, Lei H Y, Sun F Z, Wang D, Liao G Q, Li Y T 2021 Acta Phys. Sin. 70 085205
Google Scholar
[6] 刘家合, 鲁佳哲, 雷俊杰, 高勋, 林 景全 2020 69 057401
Google Scholar
[7] Liu L B, Deng H X, Zu X T Yuan X D Zheng W G 2020 Chin. Phys. B 29 507
[8] 杜报, 蔡洪波, 张文帅, 陈京, 邹士阳, 朱少平 2019 68 185205
Google Scholar
Du B, Cai H B, Zhang W S, Chen J, Zou S Y, Zhu S P 2019 Acta Phys. Sin. 68 185205
Google Scholar
[9] Eliezer S 2010 45 181
[10] Li C K, Seguin F H, Frenje J A, Rosenberg M J, Knauer J 2009 Phys. Rev. Lett. 102(20)
[11] Li C K, Zylstra A B, Frenje J A, Séguin F H, Sinenian N, Petrasso R D, Amendt P A, Bionta R, Friedrich S, Collins G W 2013 New J. Phys. 15 025040
Google Scholar
[12] Chen Y, Zhang W, Bao J, Lin Z, Dong C, Cao J 2020 Chin. Phys. Lett. 37 095201
Google Scholar
[13] 曹柱荣, 张海鹰, 董建军, 袁铮, 刘慎业, 江少恩, 丁永坤 2011 60 045212
Google Scholar
Cao Z R, Zhang H Y, Dong J J, Yuan Z, Miao W Y, Liu S Y, Jiang S E, Ding Y K 2011 Acta Phys. Sin. 60 045212
Google Scholar
[14] Glenzer S H, Lee H J, Davis P, Doppner T, Falcone R W, Fortmann C, Hanmmel B A, Kritcher A L, Landen O L, Lee R W, Munro D H, Redmer R 2010 High Energy Density Phys. 6 1
Google Scholar
[15] Fahad M, Ali S, Iqbal Y 2019 Plasma Sci. Technol. 21 2058
[16] Azechi H, Shiraga H, Miyanaga N, Nishimura H 1997 Fusion Eng. Des. 34−35 37
[17] Borghesi M 2002 Phys. Plasma 9 2214
Google Scholar
[18] Li C K, Seguin F H, Frenje J A, Rygg J R, Petrasso R D, Town R P J, Amendt P A, Hatchett S P, Landen O L, Mackinnon A J 2006 Phys. Rev. Lett. 97 135003
Google Scholar
[19] Kugland N L, Ryutov D D, Plechaty C, Ross J S, Park H S 2012 Rev. Sci. Instrum. 83 101301
Google Scholar
[20] Li C K, Seguin F H, Frenje J A, Petrasso R D, Amendt P A, Town R P J, Landen O L, Rygg J R, Betti R, Knauer J P, Meyerhofer D D, Soures J M, Back C A, Kilkenny J D, Nikroo A 2009 Phys. Rev. Lett. 102 205001
Google Scholar
[21] Patel P K, Mackinnon A J, Key M H, Cowan T E, Stephens R 2003 Phys. Rev. Lett. 91 125004
Google Scholar
[22] 马文君, 刘志鹏, 王鹏业, 赵家瑞, 颜学庆 2021 70 084102
Google Scholar
Ma W J, Liu Z P, Wang P J, Zhao J R, Yan X Q 2021 Acta Phys. Sin. 70 084102
Google Scholar
[23] Zhu P, Zhang Z, Chen L, Zheng J, Li R, Wang W, Li J, Wang X, Cao J, Qian D 2010 Appl. Phys. Lett. 97 155
[24] Chen L, Li R, Chen J, Zhu P, Liu F, Cao J, Sheng Z, Zhang J 2016 Proc. Natl. Acad. Sci. U.S.A. 112 47
[25] Du B, Cai H B, Zhang W S, Wang X F, Zhu S P 2021 Matter Radiat. Extrem. 6 035903
Google Scholar
-
图 1 实验原理和装置示意图 (a) 装置总体布局; (b) 被等离子体内电磁场调制后的电子束在闪烁体上成像示意图; (c) 靶附近的局部放大图
Fig. 1. The experimental principle: (a) The set-up of the whole system; (b) imaging principle of the electron beam on the scintillator after being modulated in the plasma; (c) the enlarged setup nearby the target.
图 2 电子枪束流模拟, 能量100 keV的电子束在靶位置聚焦
Fig. 2. Simulation result on electron source, beam with 100 keV energy focused on the target.
图 3 实验测量到的电子束分布 (a) 电子束团的纵向分布; (b) 电子束团在成像板上得到的束斑
Fig. 3. Electron bunch from Gun: (a) The longitudinal distribution of electron bunch; (b) the beam profile at imaging plate.
图 4 激光的时间和空间分布 (a) 激光的时间分布; (b)激光在银靶处束腰光斑
Fig. 4. The time and space distribution of laser pulse: (a) The time distribution; (b) the laser waist at the target.
图 5 带上负载测得的两偏转极板间的高压脉冲信号. 平顶宽度5 μs, 最高电压6.32 kV, 脉冲电压信号有一段线性上升沿5.44 kV/10 ns
Fig. 5. The HV signal between the two deflecting plates with load, HV pulse with 5 μs flattop and 6.32 kV maximum, a linear rise edge at the slope of 5.44 kV/10 ns.
图 6 没有激光时的电子束斑. 上面的束斑, 偏转极板间没有电压; 下面的束斑, 偏转极板间有电压
Fig. 6. Beam profile without laser pulse. The above one is beam profile without deflecting HV; The below one is beam profile with deflecting HV.
图 7 10 ns电子束被等离子体内电场调制后分布
Fig. 7. Distribution of the electron bunch with 10 ns length modulated by the electric field of the laser plasma.
-
[1] Lindl J D, Hammel B A, Logan B G, Meyerhofer D D, Payne S A, Sethian J D 2003 Plasma Phys. Controlled Fusion 45 A217
Google Scholar
[2] Edwards C B, Danson C N 2015 High Power Laser Sci. Eng. 3 e4
Google Scholar
[3] Zohuri B 2017 Inertial Confinement Fusion Driven Thermonuclear Energy (Albuquerque: Springer International Publishing) pp133−192
[4] Craxton R S, Anderson K S, Boehly T R, Goncharov V N, Harding D R, Knauer J P, Mccrory R L, Mckenty P W, Meyerhofer D D, Myatt J F 2015 Phys. Plasmas 22 139
[5] 王天泽, 雷弘毅, 孙方正, 王丹, 廖国前, 李玉同 2021 70 085205
Google Scholar
Wang T Z, Lei H Y, Sun F Z, Wang D, Liao G Q, Li Y T 2021 Acta Phys. Sin. 70 085205
Google Scholar
[6] 刘家合, 鲁佳哲, 雷俊杰, 高勋, 林 景全 2020 69 057401
Google Scholar
[7] Liu L B, Deng H X, Zu X T Yuan X D Zheng W G 2020 Chin. Phys. B 29 507
[8] 杜报, 蔡洪波, 张文帅, 陈京, 邹士阳, 朱少平 2019 68 185205
Google Scholar
Du B, Cai H B, Zhang W S, Chen J, Zou S Y, Zhu S P 2019 Acta Phys. Sin. 68 185205
Google Scholar
[9] Eliezer S 2010 45 181
[10] Li C K, Seguin F H, Frenje J A, Rosenberg M J, Knauer J 2009 Phys. Rev. Lett. 102(20)
[11] Li C K, Zylstra A B, Frenje J A, Séguin F H, Sinenian N, Petrasso R D, Amendt P A, Bionta R, Friedrich S, Collins G W 2013 New J. Phys. 15 025040
Google Scholar
[12] Chen Y, Zhang W, Bao J, Lin Z, Dong C, Cao J 2020 Chin. Phys. Lett. 37 095201
Google Scholar
[13] 曹柱荣, 张海鹰, 董建军, 袁铮, 刘慎业, 江少恩, 丁永坤 2011 60 045212
Google Scholar
Cao Z R, Zhang H Y, Dong J J, Yuan Z, Miao W Y, Liu S Y, Jiang S E, Ding Y K 2011 Acta Phys. Sin. 60 045212
Google Scholar
[14] Glenzer S H, Lee H J, Davis P, Doppner T, Falcone R W, Fortmann C, Hanmmel B A, Kritcher A L, Landen O L, Lee R W, Munro D H, Redmer R 2010 High Energy Density Phys. 6 1
Google Scholar
[15] Fahad M, Ali S, Iqbal Y 2019 Plasma Sci. Technol. 21 2058
[16] Azechi H, Shiraga H, Miyanaga N, Nishimura H 1997 Fusion Eng. Des. 34−35 37
[17] Borghesi M 2002 Phys. Plasma 9 2214
Google Scholar
[18] Li C K, Seguin F H, Frenje J A, Rygg J R, Petrasso R D, Town R P J, Amendt P A, Hatchett S P, Landen O L, Mackinnon A J 2006 Phys. Rev. Lett. 97 135003
Google Scholar
[19] Kugland N L, Ryutov D D, Plechaty C, Ross J S, Park H S 2012 Rev. Sci. Instrum. 83 101301
Google Scholar
[20] Li C K, Seguin F H, Frenje J A, Petrasso R D, Amendt P A, Town R P J, Landen O L, Rygg J R, Betti R, Knauer J P, Meyerhofer D D, Soures J M, Back C A, Kilkenny J D, Nikroo A 2009 Phys. Rev. Lett. 102 205001
Google Scholar
[21] Patel P K, Mackinnon A J, Key M H, Cowan T E, Stephens R 2003 Phys. Rev. Lett. 91 125004
Google Scholar
[22] 马文君, 刘志鹏, 王鹏业, 赵家瑞, 颜学庆 2021 70 084102
Google Scholar
Ma W J, Liu Z P, Wang P J, Zhao J R, Yan X Q 2021 Acta Phys. Sin. 70 084102
Google Scholar
[23] Zhu P, Zhang Z, Chen L, Zheng J, Li R, Wang W, Li J, Wang X, Cao J, Qian D 2010 Appl. Phys. Lett. 97 155
[24] Chen L, Li R, Chen J, Zhu P, Liu F, Cao J, Sheng Z, Zhang J 2016 Proc. Natl. Acad. Sci. U.S.A. 112 47
[25] Du B, Cai H B, Zhang W S, Wang X F, Zhu S P 2021 Matter Radiat. Extrem. 6 035903
Google Scholar
下载:
计量
- 文章访问数: 4143
- PDF下载量: 62
- 被引次数: 0
