激光混沌并行串联同步及其在中继器保密通信系统中的应用

颜森林

doi:10.7498/aps.68.20190212

摘要

研究了两个不同半导体激光器的相互耦合与其他激光器之间的混沌并行同步和多周期并行同步. 提出激光并行串联复杂动力学系统与网络, 给出光学光路与数学物理模型. 由此提出中继器运行原则, 给出了信道编码方程. 成功实现了激光混沌再生与发送, 分别完成了有中继器系统的两个异路混沌加密通信. 这是一种新型的混沌编码网络系统, 具有中继器核心技术要素, 它具有多变量、高维度、多密钥以及两路不同混沌载波特点, 具有高度的安全性、不易被破解等特性. 其研究结果对混沌在保密通信应用、光网络和激光技术的研究具有重要的参考价值.

关键词:

混沌 /
同步 /
激光器 /
网络

Abstract

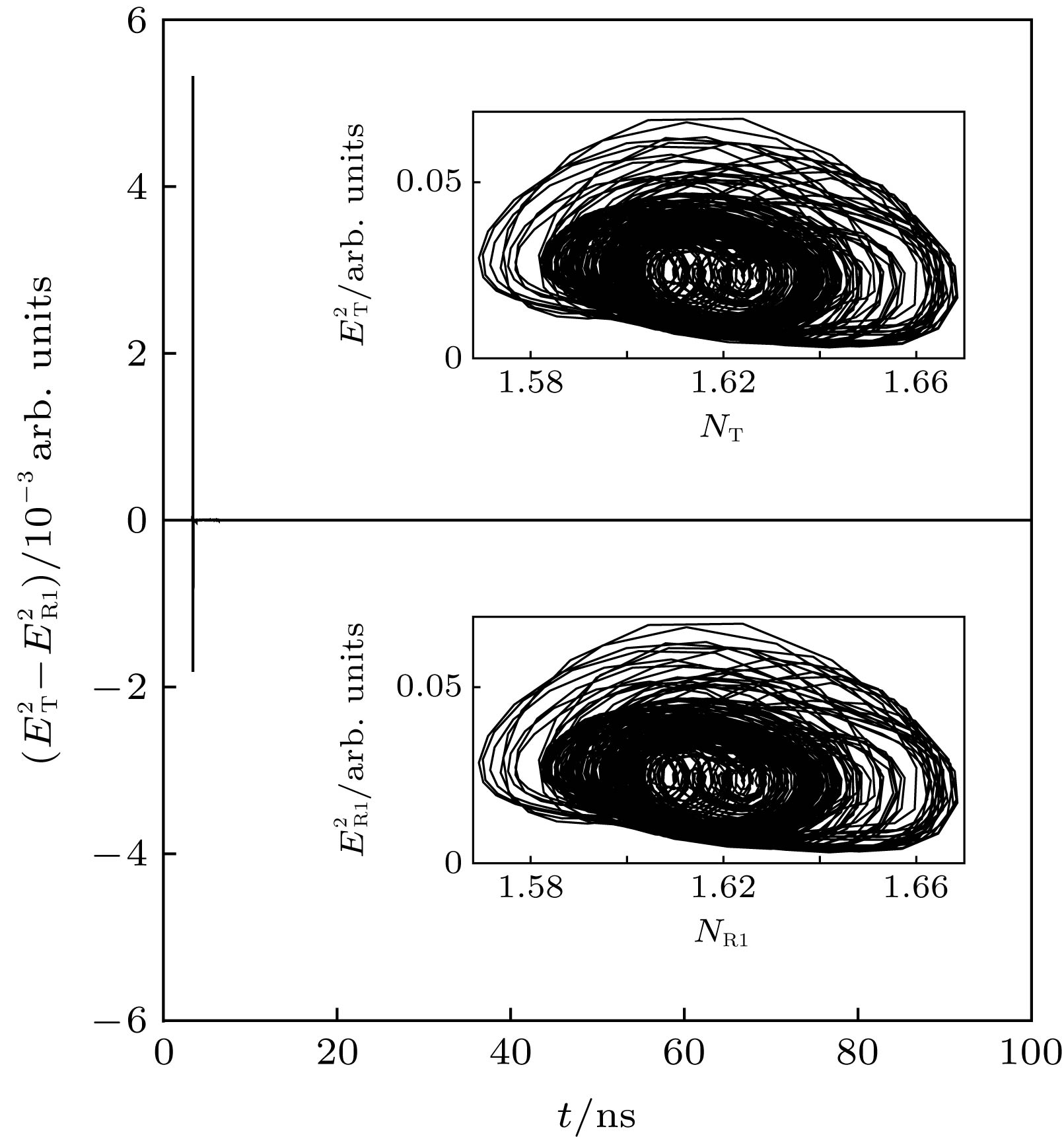
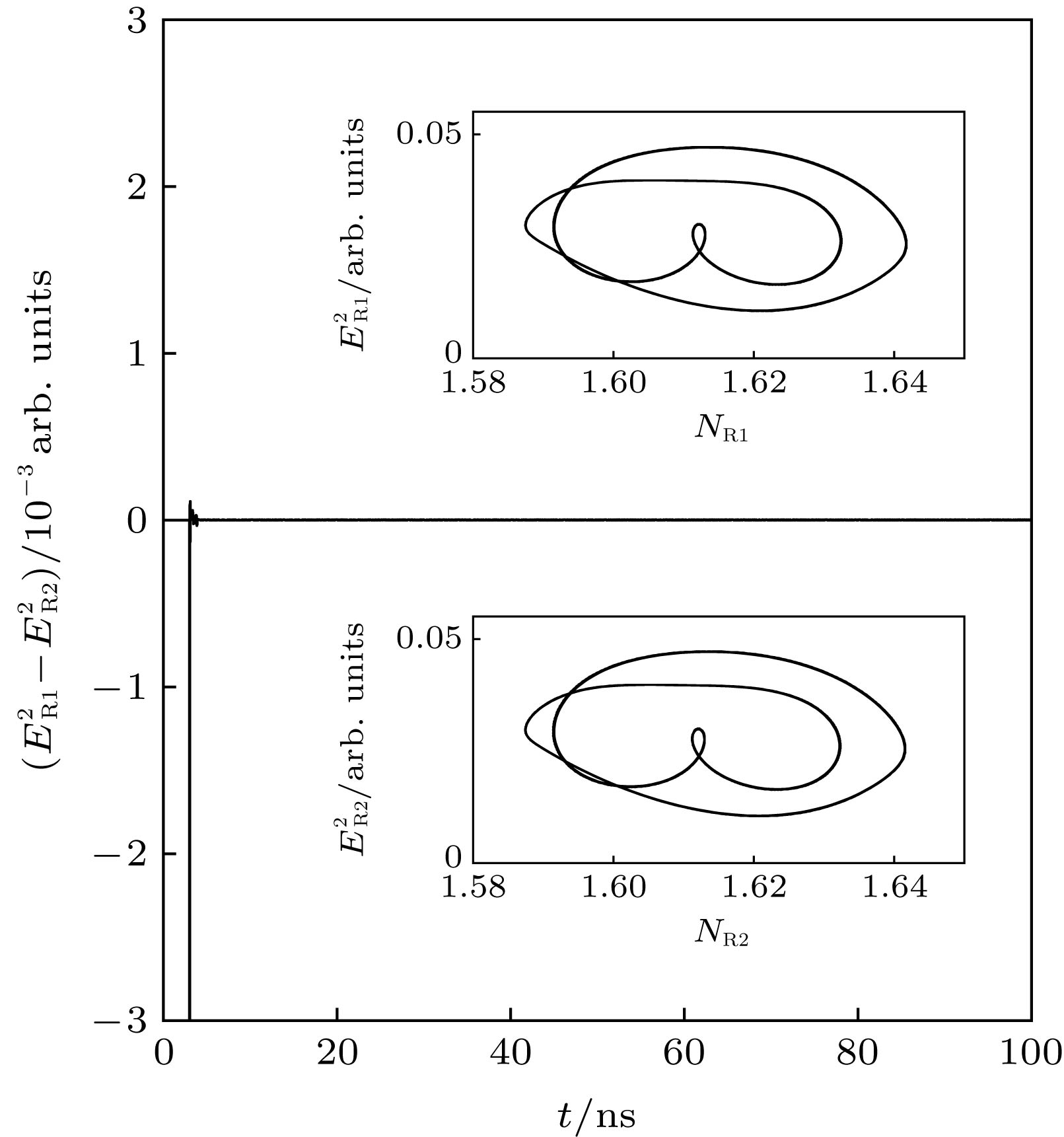
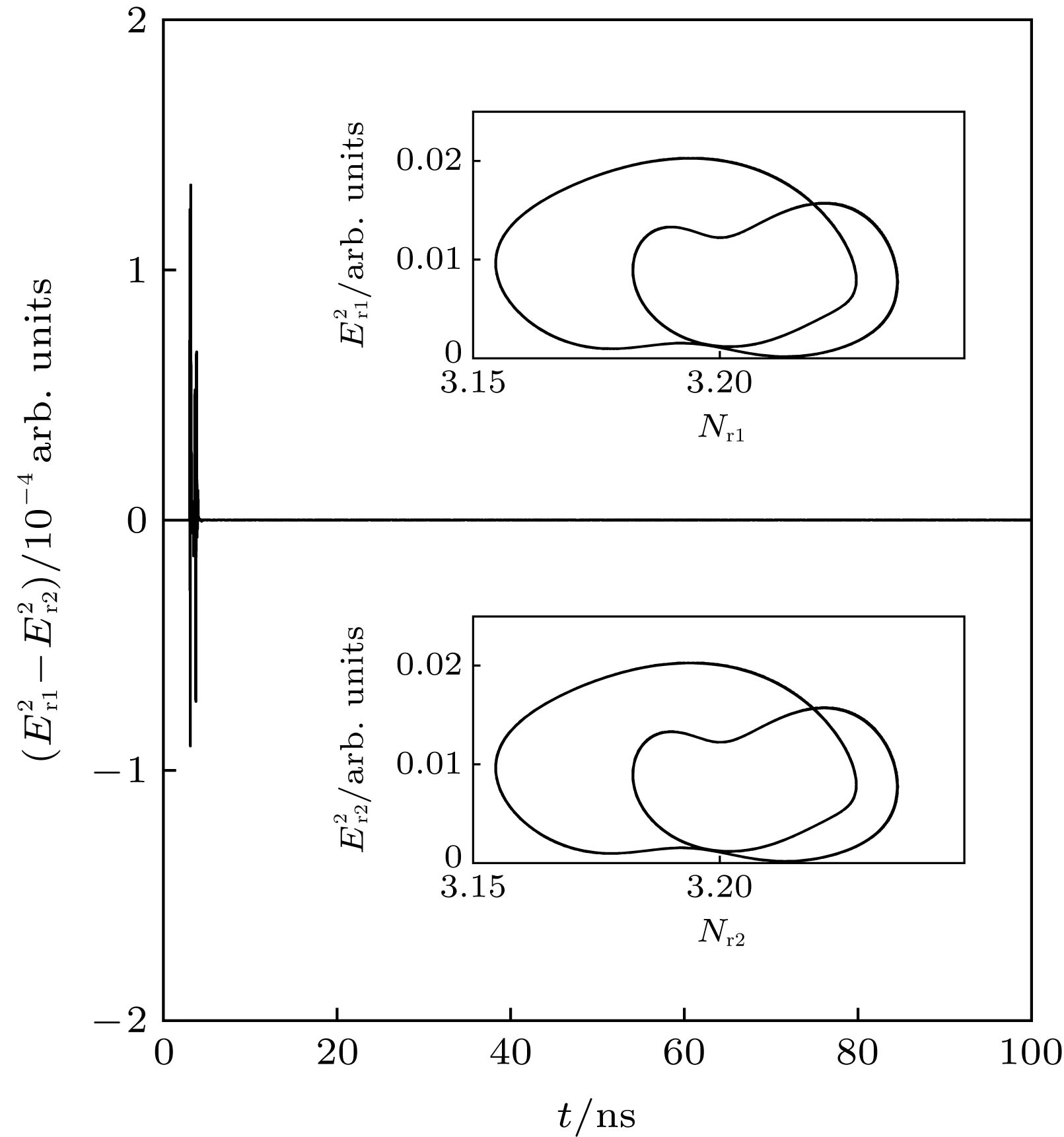
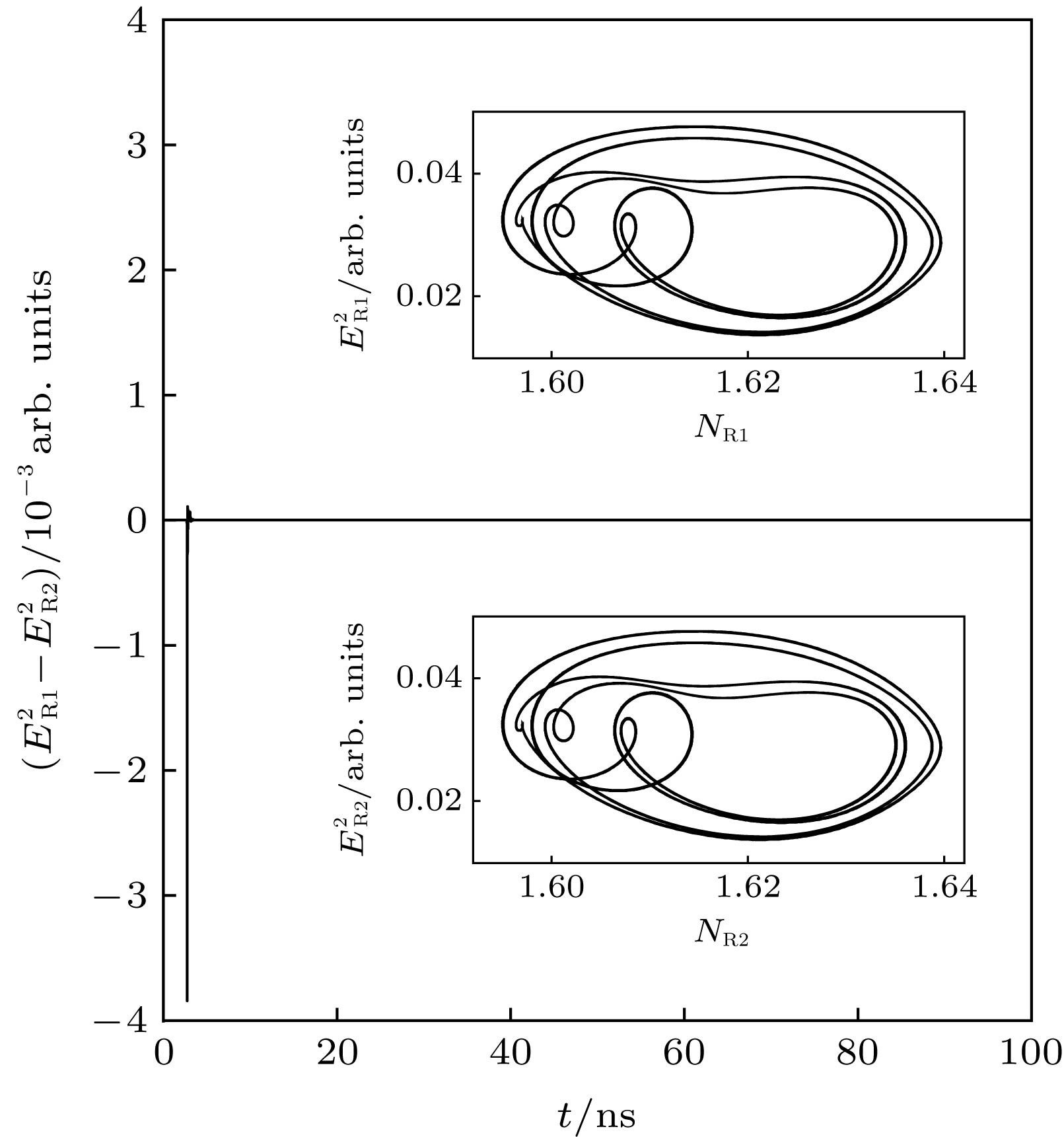
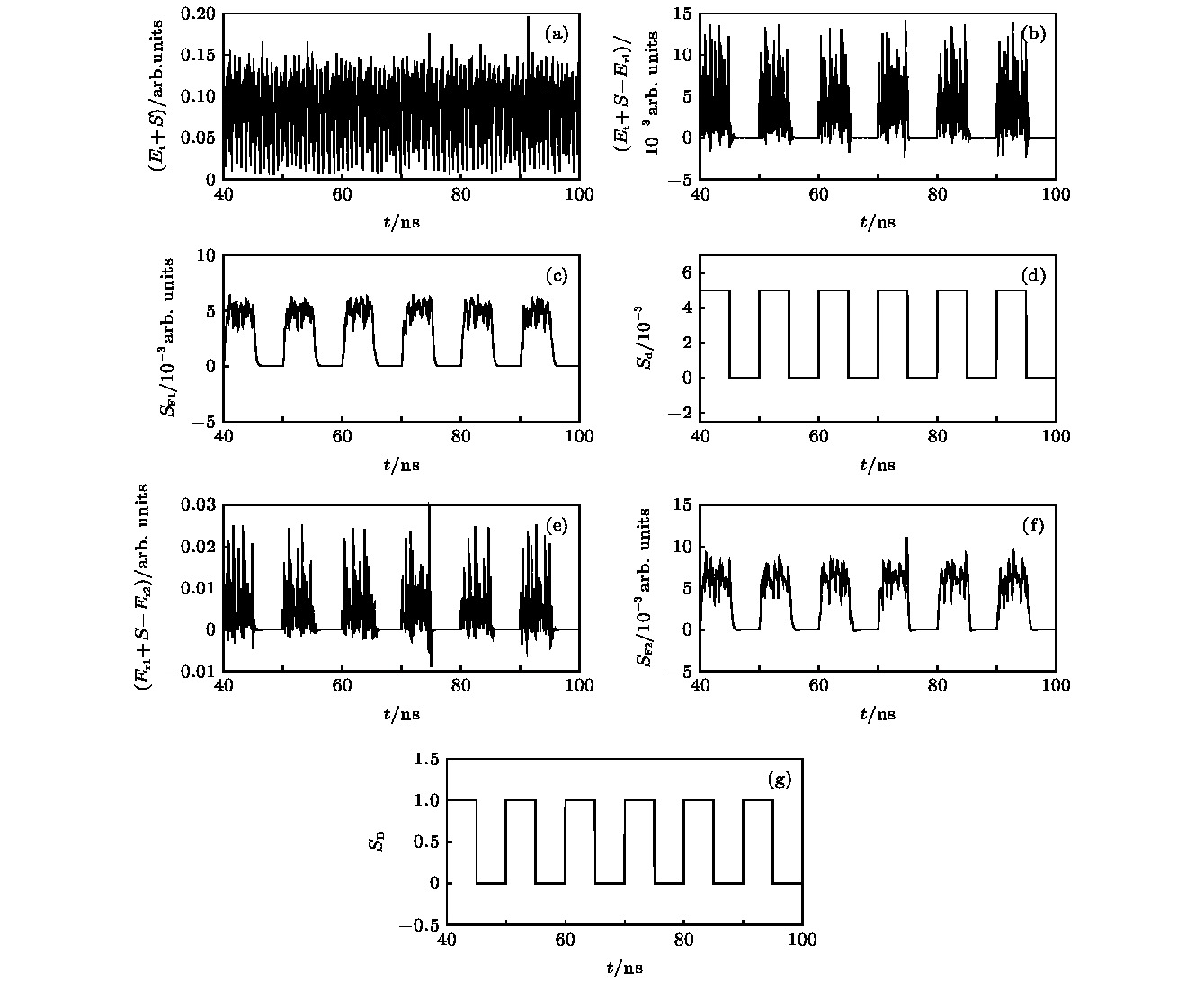
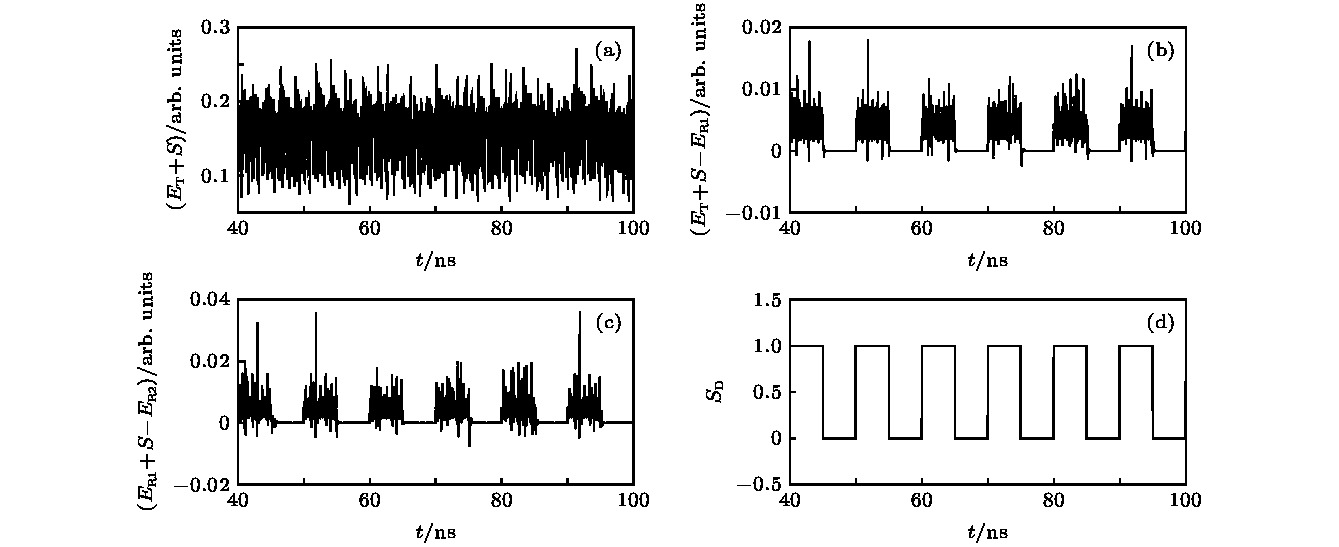
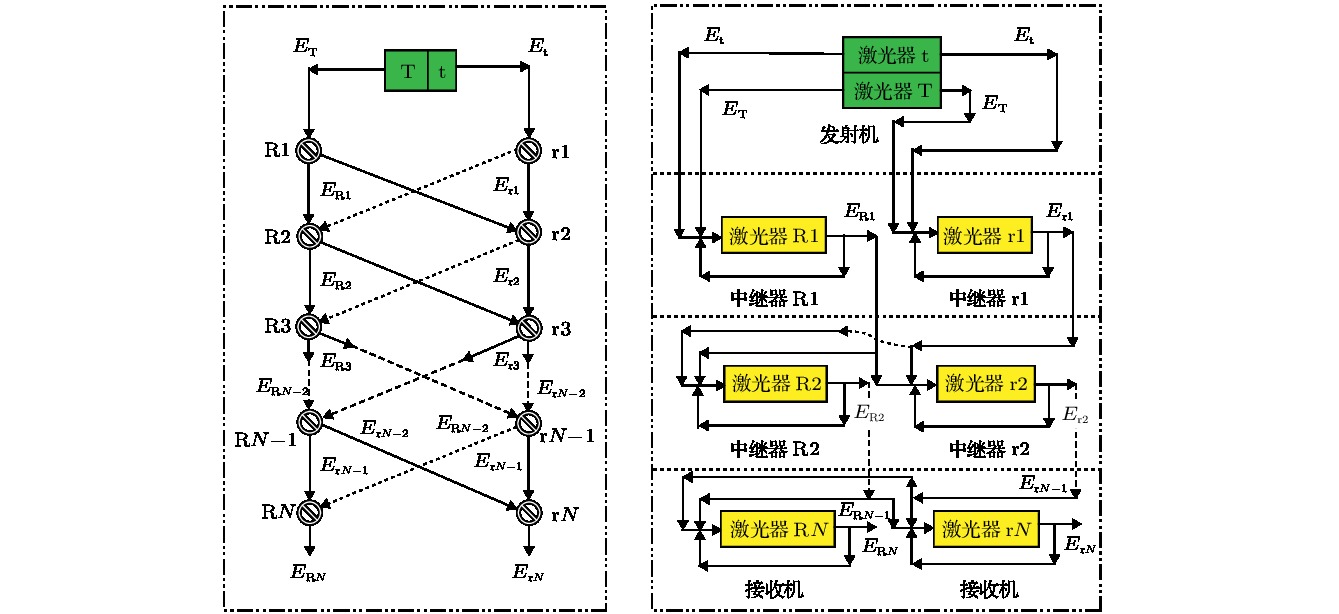
In this paper, chaotic parallel synchronization and quasi-periodic parallel synchronization between two mutually coupled different semiconductor lasers and other lasers are studied, and the regeneration of chaotic laser and key technology of repeater are discussed. The complex dynamic system and network of laser parallel series are presented in mathematics and in physics, and the network topology diagram and optics path are specified. A mathematical-physical model is given to study how to obtain parallel synchronization via the coupled driving nonlinear equations. The operating principle of the repeater is put forward for chaotic secure communication, and the channel equation of repeater is established because the laser chaotic behavior is extremely sensitive to external influences and system parameter changes. The laser’s chaotically regenerating and transmitting is successfully realized via two sets of repeaters. The chaotic encoding communication with repeaters is successfully completed while the encoding information signal is accurately extracted from the chaotic carrier by a filter and calculating the synchronous difference. We adopt three sets of lasers as a research case to simulate and verify the theory of laser parallel series network we put forward to fit perfectly the obtained numerical results. We study the parameter mismatch problem of the system, where the synchronous difference is numerically calculated by varying some parameters of the lasers. In the case of smaller parameter mismatch, the system has a highly synchronous capability to a certain degree. This is a novel laser chaotic encoding network in chaotic secure communication and characterizes the core technical elements of the repeater. The laser transmitter has four nonlinear interaction variables, where the nonlinear interaction between the amplitude and phase of the two optical fields results in highly nonlinear dynamics. The system has the characteristics of high nonlinearity, multi-variable, high-dimension, and multi-key. So it is highly secure and not easy to crack. The results have an important reference value for the chaos applications in remote secure communication, optical network and laser technology.

Keywords:

chaos /
synchronization /
laser /
network

作者及机构信息

颜森林

南京晓庄学院电子工程学院, 南京　211171

通信作者: 颜森林, senlinyan@163.com

Authors and contacts

Yan Sen-Lin

Electronic Engineering School, Nanjing Xiaozhuang University, Nanjing 211171, China

Corresponding author: Yan Sen-Lin, senlinyan@163.com

文章全文 : translate this paragraph

参考文献

[1]	Bayati B M A, Ahmad K A, Naimee M A 2018 J. Opt. Soc. Am. B 35 918 Google Scholar
[2]	Kang Z, Sun J, Ma L, Qi Y, Jian S 2014 IEEE J. Quantum Electron. 50 148 Google Scholar
[3]	王顺天, 吴正茂, 吴加贵, 周立, 夏光琼 2015 64 154205 Google Scholar Wang S T, Wu Z M, Wu J G, Zhou L, Xia G Q 2015 Acta Phys. Sin. 64 154205 Google Scholar
[4]	钟东洲, 邓涛, 郑国梁 2015 63 070504 Google Scholar Zhong D Z, Deng T, Zheng G L 2015 Acta Phys. Sin. 63 070504 Google Scholar
[5]	Mulet J, Masoller C, Mirasso C R 2002 Phys. Rev. A 65 063815 Google Scholar
[6]	Erzgräbera D, Lenstraa D, Krauskopfc B 2006 Proc. SPIE 6184 618407 Google Scholar
[7]	Arroyo-Almanza D A, Pisarchik A N, Fischer I, Mirasso C R, Soriano M C 2013 Opt. Commun. 301 67
[8]	Erzgräber H, Wieczorek S 2009 Phys. Rev. E 80 026212 Google Scholar
[9]	刘庆喜, 潘炜, 张力月, 李念强, 阎娟 2015 64 024209 Google Scholar Liu Q X, Pan W, Zhang L Y, Li N Q, Yan J 2015 Acta Phys. Sin. 64 024209 Google Scholar
[10]	Wunsche H J, Bauer S, Kreissl J, Ushakov O, Korneyev N, Henneberger F, Wille E, Erzgräber H, Peil M, Elsaor W, Fischer I 2005 Phys. Rev. Lett. 94 163901 Google Scholar
[11]	Mulet J, Mirasso C R, Heil T, Fischer I 2004 J. Opt. B: Quantum Semiclass. Opt. 6 97 Google Scholar
[12]	Hill M T, Waardt H D, Dorren H J S 2001 IEEE J. Quantum Electron. 37 405 Google Scholar
[13]	Tang X, Wu Z M, Wu J G, Deng T, Fan L, Zhong Z Q, Chen J J, Xia G Q 2015 Laser Phys. Lett. 12 015003 Google Scholar
[14]	Quirce A, Valle A, Thienpont H, Panajotov K 2016 J. Opt. Soc. Am. B 33 90 Google Scholar
[15]	Zhang W L, Pan W, Luo B, Li X F, Zou X H, Wang M Y 2007 J. Opt. Soc. Am. B 24 1276
[16]	Hong Y H 2015 IEEE J. Select. Topics Quantum Electron. 21 1801007
[17]	Wang A B, Wang Y C, Wang J F 2009 Opt. Lett. 34 1144 Google Scholar
[18]	李增, 冯玉玲, 王晓茜, 姚治海 2018 67 140501 Google Scholar Li Z, Feng Y L, Wang X Q, Yao Z H 2018 Acta Phys. Sin. 67 140501 Google Scholar
[19]	张浩, 郭星星, 项水英 2018 67 204202 Google Scholar Zhang H, Guo X X, Xiang S Y 2018 Acta Phys. Sin. 67 204202 Google Scholar
[20]	Liu J, Wu Z M, Xia G Q 2009 Opt. Express 17 12619 Google Scholar
[21]	Wu J, Wu Z, Liu Y, Fan L, Tang X, Xia G 2013 IEEE/OSA J. Lightwave Technol. 31 461 Google Scholar
[22]	穆鹏华, 潘炜, 李念强, 闫连山, 罗斌, 邹喜华, 徐明峰 2015 64 124206 Google Scholar Mu P H, Pan W, Li N Q, Yan L S, Luo B, Zou X H, Xu M F 2015 Acta Phys. Sin. 64 124206 Google Scholar
[23]	Li N Q, Pan W, Luo B, Yan L S, Zou X H, Jiang N, Xiang S Y 2012 IEEE Photon. Technol. Lett. 24 1072 Google Scholar

施引文献

图 1 并行串联复杂动力学网络及中继器光路图　(a) 网络拓扑图; (b)光路图

Fig. 1. Parallel series complex dynamical network and optical path of repeater: (a) Network topology; (b) optics path.

下载: 全尺寸图片幻灯片

图 2 激光器t与r1取得混沌同步过程, 其中内插图分别是两个激光器的混沌吸引子

Fig. 2. The laser t synchronizes with the laser r1. The ininserted illustrations show the chaotic attractors of two lasers.

下载: 全尺寸图片幻灯片

图 5 激光器R1与R2取得混沌同步　(a)同步过程; (b)互相关函数曲线

Fig. 5. The laser R1 synchronizes with the laser R2: (a) The synchronous process; (b) the cross-correlation function curve.

下载: 全尺寸图片幻灯片

图 3 激光器r1与r2取得混沌同步　(a)同步过程; (b)互相关函数曲线

Fig. 3. The laser t synchronizes with the laser r2: (a) The synchronous process; (b) the cross-correlation function curve.

下载: 全尺寸图片幻灯片

图 4 激光器T与R1的混沌同步过程

Fig. 4. The laser T synchronizes with the laser R1.

下载: 全尺寸图片幻灯片

图 6 激光器t与r1取得4周期同步

Fig. 6. Period-4 synchronization between the lasers t and r1

下载: 全尺寸图片幻灯片

图 9 激光器R1与R2取得3周期同步

Fig. 9. Period-3 synchronization between the lasers R1 and R2

下载: 全尺寸图片幻灯片

图 7 激光器r1与r2取得4周期同步

Fig. 7. Period-4 synchronization between the lasers r1 and r2

下载: 全尺寸图片幻灯片

图 8 激光器T与R1取得3周期同步

Fig. 8. Period-3 synchronization between the lasers T and R1

下载: 全尺寸图片幻灯片

图 10 激光器t与r1取得10周期同步

Fig. 10. Period-10 synchronization between the lasers t and r1

下载: 全尺寸图片幻灯片

图 13 激光器R1与R2的另一个10周期同步

Fig. 13. Another period-10 synchronization between the lasers R1 and R2.

下载: 全尺寸图片幻灯片

图 11 激光器r1与r2取得10周期同步

Fig. 11. Period-10 synchronization between the lasers r1 and r2.

下载: 全尺寸图片幻灯片

图 12 激光器T与R1的另一个10周期同步

Fig. 12. Another period-10 synchronization between the lasers T and R1.

下载: 全尺寸图片幻灯片

图 14 同步调制解调过程

Fig. 14. Synchronous decoding process.

下载: 全尺寸图片幻灯片

图 15 另一路同步调制解调过程

Fig. 15. Another synchronous decoding process.

下载: 全尺寸图片幻灯片

表 1 激光器参量

Table 1. Laser parameters.

参量	值	参量	值
腔长L/ μm	350	俄歇复合因子C/ cm⁶·s^–1	3.5 × 10^–29
腔宽w/ μm	2	饱和光子场振幅\|E_s\| / m^–3/2	1.6619 × 10¹¹
腔厚d/ μm	0.15	增益常数α/ cm²	2.3 × 10^–16
压缩和限制因子Γ	0.29	光线宽增强因子β_c	6
群速度折射率n_g	3.8	耦合驱动系数k	0.1
光子损耗系数α_m/ cm^–1	49	频率ω/ Rad·s^–1	1438 × 10¹²
非辐射复合速率A_nr/ s^–1	1.0 × 10⁸	激光透明时载流子密度n_th/ cm^–3	1.2 × 10¹⁸
辐射复合因子B/ cm³·s^–1	1.2 × 10^–10

下载: 导出CSV

map

[1]	Bayati B M A, Ahmad K A, Naimee M A 2018 J. Opt. Soc. Am. B 35 918 Google Scholar
[2]	Kang Z, Sun J, Ma L, Qi Y, Jian S 2014 IEEE J. Quantum Electron. 50 148 Google Scholar
[3]	王顺天, 吴正茂, 吴加贵, 周立, 夏光琼 2015 64 154205 Google Scholar Wang S T, Wu Z M, Wu J G, Zhou L, Xia G Q 2015 Acta Phys. Sin. 64 154205 Google Scholar
[4]	钟东洲, 邓涛, 郑国梁 2015 63 070504 Google Scholar Zhong D Z, Deng T, Zheng G L 2015 Acta Phys. Sin. 63 070504 Google Scholar
[5]	Mulet J, Masoller C, Mirasso C R 2002 Phys. Rev. A 65 063815 Google Scholar
[6]	Erzgräbera D, Lenstraa D, Krauskopfc B 2006 Proc. SPIE 6184 618407 Google Scholar
[7]	Arroyo-Almanza D A, Pisarchik A N, Fischer I, Mirasso C R, Soriano M C 2013 Opt. Commun. 301 67
[8]	Erzgräber H, Wieczorek S 2009 Phys. Rev. E 80 026212 Google Scholar
[9]	刘庆喜, 潘炜, 张力月, 李念强, 阎娟 2015 64 024209 Google Scholar Liu Q X, Pan W, Zhang L Y, Li N Q, Yan J 2015 Acta Phys. Sin. 64 024209 Google Scholar
[10]	Wunsche H J, Bauer S, Kreissl J, Ushakov O, Korneyev N, Henneberger F, Wille E, Erzgräber H, Peil M, Elsaor W, Fischer I 2005 Phys. Rev. Lett. 94 163901 Google Scholar
[11]	Mulet J, Mirasso C R, Heil T, Fischer I 2004 J. Opt. B: Quantum Semiclass. Opt. 6 97 Google Scholar
[12]	Hill M T, Waardt H D, Dorren H J S 2001 IEEE J. Quantum Electron. 37 405 Google Scholar
[13]	Tang X, Wu Z M, Wu J G, Deng T, Fan L, Zhong Z Q, Chen J J, Xia G Q 2015 Laser Phys. Lett. 12 015003 Google Scholar
[14]	Quirce A, Valle A, Thienpont H, Panajotov K 2016 J. Opt. Soc. Am. B 33 90 Google Scholar
[15]	Zhang W L, Pan W, Luo B, Li X F, Zou X H, Wang M Y 2007 J. Opt. Soc. Am. B 24 1276
[16]	Hong Y H 2015 IEEE J. Select. Topics Quantum Electron. 21 1801007
[17]	Wang A B, Wang Y C, Wang J F 2009 Opt. Lett. 34 1144 Google Scholar
[18]	李增, 冯玉玲, 王晓茜, 姚治海 2018 67 140501 Google Scholar Li Z, Feng Y L, Wang X Q, Yao Z H 2018 Acta Phys. Sin. 67 140501 Google Scholar
[19]	张浩, 郭星星, 项水英 2018 67 204202 Google Scholar Zhang H, Guo X X, Xiang S Y 2018 Acta Phys. Sin. 67 204202 Google Scholar
[20]	Liu J, Wu Z M, Xia G Q 2009 Opt. Express 17 12619 Google Scholar
[21]	Wu J, Wu Z, Liu Y, Fan L, Tang X, Xia G 2013 IEEE/OSA J. Lightwave Technol. 31 461 Google Scholar
[22]	穆鹏华, 潘炜, 李念强, 闫连山, 罗斌, 邹喜华, 徐明峰 2015 64 124206 Google Scholar Mu P H, Pan W, Li N Q, Yan L S, Luo B, Zou X H, Xu M F 2015 Acta Phys. Sin. 64 124206 Google Scholar
[23]	Li N Q, Pan W, Luo B, Yan L S, Zou X H, Jiang N, Xiang S Y 2012 IEEE Photon. Technol. Lett. 24 1072 Google Scholar

[1]	颜森林. 激光局域网络的混沌控制及并行队列同步. , 2021, 70(8): 080501. doi: 10.7498/aps.70.20201251
[2]	颜森林. 半导体激光器混沌法拉第效应控制方法. , 2015, 64(24): 240505. doi: 10.7498/aps.64.240505
[3]	李雨珊, 吕翎, 刘烨, 刘硕, 闫兵兵, 常欢, 周佳楠. 复杂网络时空混沌同步的Backstepping设计. , 2013, 62(2): 020513. doi: 10.7498/aps.62.020513
[4]	颜森林. 激光混沌并联同步及其在全光逻辑门中的应用研究. , 2013, 62(23): 230504. doi: 10.7498/aps.62.230504
[5]	胡文, 李俊平, 张弓, 刘文波, 赵广浩. 自调频混沌系统及其调频码耦合同步. , 2012, 61(1): 010504. doi: 10.7498/aps.61.010504
[6]	冯野, 杨毅彪, 王安帮, 王云才. 利用半导体激光器环产生27 GHz的平坦宽带混沌激光. , 2011, 60(6): 064206. doi: 10.7498/aps.60.064206
[7]	颜森林. 激光混沌耦合反馈光电及全光逻辑门研究. , 2011, 60(5): 050509. doi: 10.7498/aps.60.050509
[8]	韩敏, 牛志强, 韩冰. 一种参数摄动的混沌异结构同步方法. , 2008, 57(11): 6824-6829. doi: 10.7498/aps.57.6824
[9]	颜森林. 外腔延时反馈半导体激光器混沌偏振可调控制方法研究. , 2008, 57(11): 6878-6882. doi: 10.7498/aps.57.6878
[10]	颜森林. 半导体激光器混沌光电延时负反馈控制方法研究. , 2008, 57(4): 2100-2106. doi: 10.7498/aps.57.2100
[11]	于洪洁, 郑宁. 非线性函数耦合的Chen吸引子网络的混沌同步. , 2008, 57(8): 4712-4720. doi: 10.7498/aps.57.4712
[12]	桑新柱, 余重秀, 王葵如. 波长可调谐混沌产生和同步的实验研究. , 2006, 55(11): 5728-5732. doi: 10.7498/aps.55.5728
[13]	颜森林. 注入半导体激光器混沌相位周期控制方法研究. , 2006, 55(10): 5109-5114. doi: 10.7498/aps.55.5109
[14]	姚利娜, 高金峰, 廖旎焕. 实现混沌系统同步的非线性状态观测器方法. , 2006, 55(1): 35-41. doi: 10.7498/aps.55.35
[15]	颜森林. 注入半导体激光器混沌调制性能与内部相位键控编码方法研究. , 2006, 55(12): 6267-6274. doi: 10.7498/aps.55.6267
[16]	颜森林, 汪胜前. 激光混沌串联同步以及混沌中继器系统理论研究. , 2006, 55(4): 1687-1695. doi: 10.7498/aps.55.1687
[17]	于洪洁, 刘延柱. 对称非线性耦合混沌系统的同步. , 2005, 54(7): 3029-3033. doi: 10.7498/aps.54.3029
[18]	陶朝海, 陆君安. 混沌系统的速度反馈同步. , 2005, 54(11): 5058-5061. doi: 10.7498/aps.54.5058
[19]	颜森林. 量子阱激光器混沌相位控制同步以及编码研究. , 2005, 54(3): 1098-1104. doi: 10.7498/aps.54.1098
[20]	颜森林, 迟泽英, 陈文建, 王泽农. 激光混沌同步和解码以及优化. , 2004, 53(6): 1704-1709. doi: 10.7498/aps.53.1704

计量

文章访问数: 7431
PDF下载量: 75
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

激光混沌并行串联同步及其在中继器保密通信系统中的应用