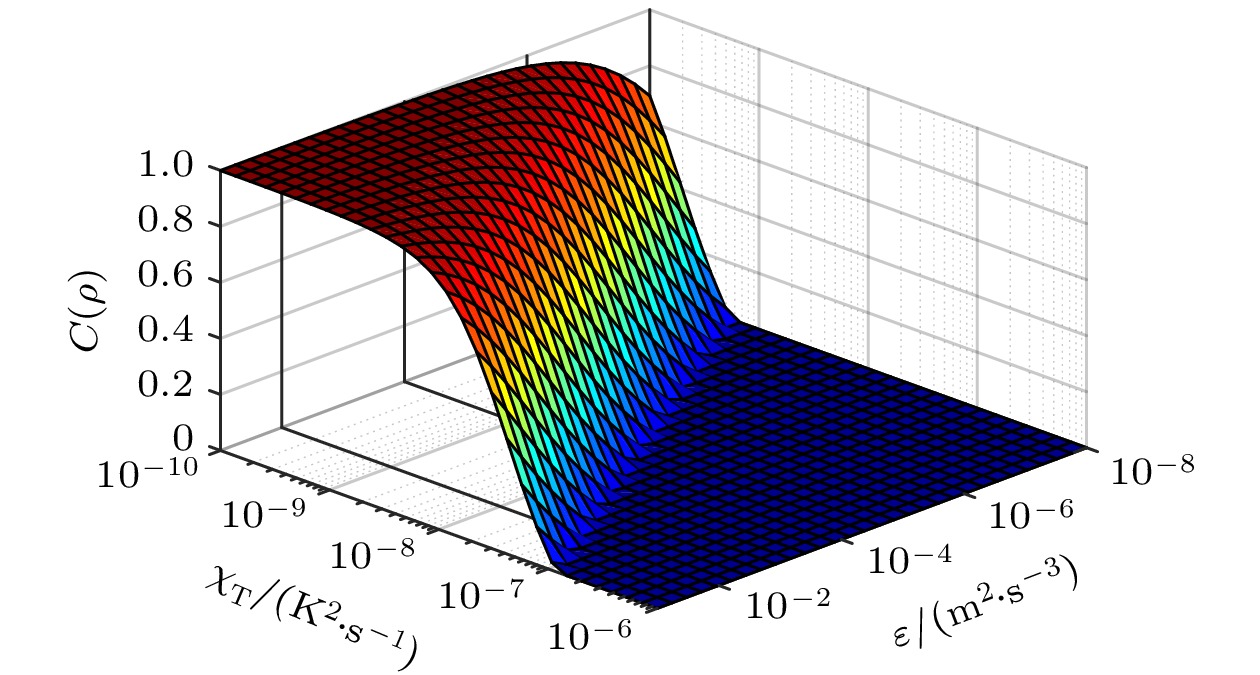
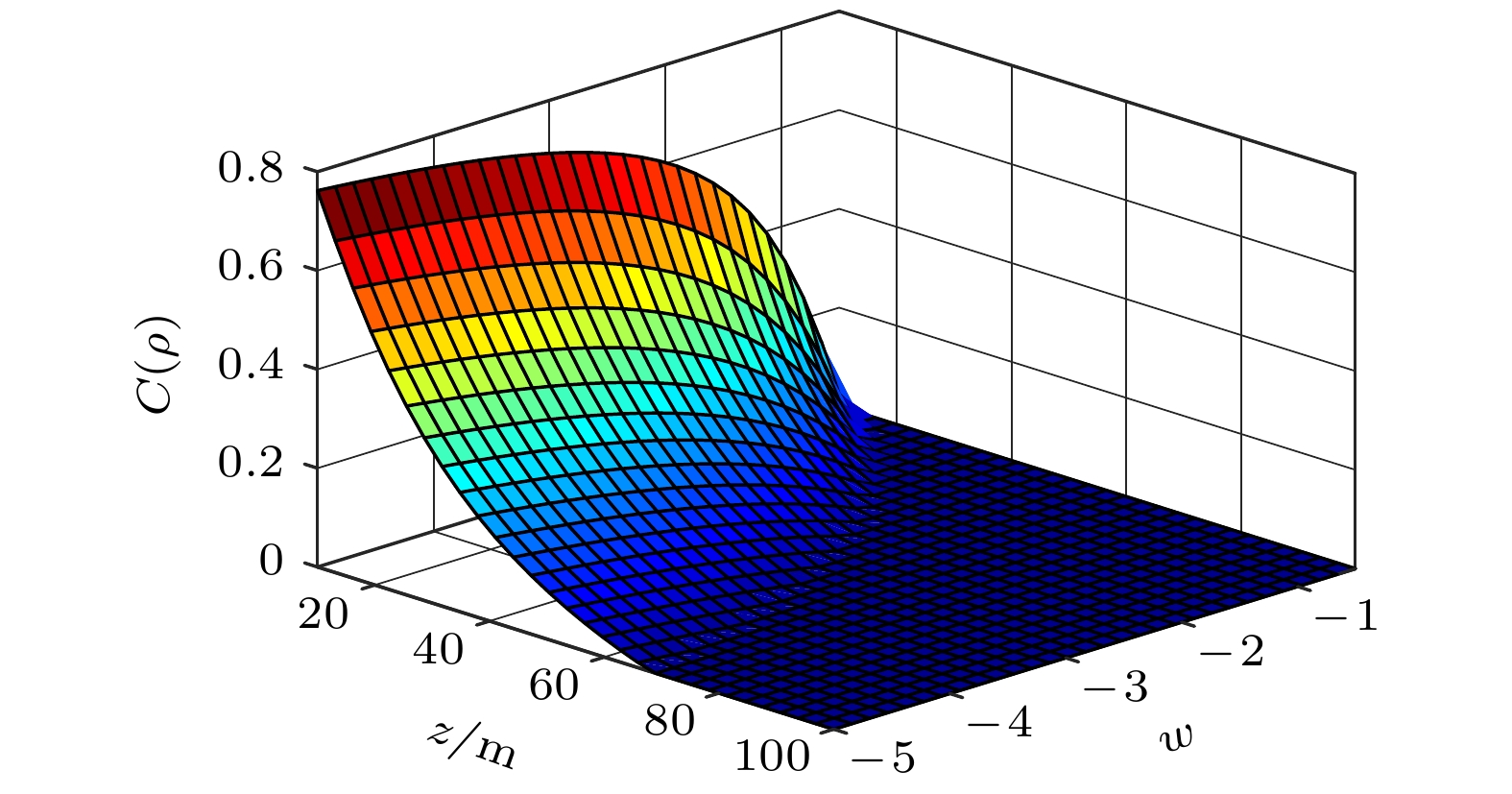
-
本文研究了基于光子轨道角动量的量子通信在水下量子信道中受海洋湍流运动的影响. 基于Elamassie等提出的海洋湍流功率谱模型, 本文建立了不同海洋湍流参数与光子轨道角动量量子通信的单光子探测概率、信道容量、密钥产生率以及双光子共生纠缠度的定量关系, 并利用纠缠光子对的共生纠缠度在海洋湍流中的普适衰减特性进一步研究了轨道角动量纠缠光子对在海洋湍流中的最大纠缠距离. 研究结果表明: 水下量子通信性能和纠缠光子对的共生纠缠度都随海洋湍流的湍流动能耗散率的增大或温度方差耗散率的减小而降低; 温度和盐度因素对海洋湍流贡献的比值对水下量子通信的影响在海水是否稳定分层的条件下具有显著的区别; 在通过海洋湍流进行量子通信时, 增加信号光子的初始轨道角动量量子数可以提高量子密钥分发的密钥产生率和纠缠光子的纠缠衰减抵抗性.The effect of the turbulent motion of ocean on the quantum communication based on the orbital angular momentum in an underwater quantum channel is studied in this work. Based on the power spectrum model of ocean turbulence proposed by Elamassie, the quantitative relationships of different ocean turbulence parameters with the single photon detection probability of orbital angular momentum photons, the channel capacity, the key generation rate, the concurrence of two entangled photons are proposed. The maximum entanglement distance of the orbital angular momentum entangled photon-pairs in the ocean turbulence is further studied by the universal entanglement decay of the concurrence of entangled photon-pairs in the ocean turbulence. The results show that the detection probability of single photon, the channel capacity, the key generation rate, and the concurrence of entangled photon-pairs decrease with the increase of the dissipation rate of turbulent kinetic energy and the decrease of the rate of dissipation of mean-squared temperature. The influence of the temperature and salinity balance parameter of ocean turbulence on the performance of underwater quantum communication are significantly different under the condition of whether the stable stratification of seawater is assumed or not. In the ocean turbulent environment, the increasing of the initial orbital angular momentum quantum number of signal photons can improve the key generation rate of quantum key distribution and the resistance of entangled photons to entanglement decay.
[1] Jin X M, Ren J G, Yang B, Yi Z H, Zhou F, Xu X F, Wang S K, Yang D, Hu Y F, Jiang S, Yang T, Yin H, Chen K, Peng C Z, Pan J W 2010 Nat. Photonics 4 376
 Google Scholar
Google Scholar
[2] Ji L, Gao J, Yang A L, Feng Z, Lin X F, Li Z G, Jin X M 2017 Opt. Express 25 19795
 Google Scholar
Google Scholar
[3] 聂敏, 王允, 杨光, 张美玲, 裴昌幸 2016 65 020303
 Google Scholar
Google Scholar
Nie M, Wang Y, Yang G, Zhang M L, Pei C X 2016 Acta Phys. Sin. 65 020303
 Google Scholar
Google Scholar
[4] 聂敏, 王超旭, 杨光, 张美玲, 孙爱晶, 裴昌幸 2021 70 030301
Nie M, Wang C X, Yang G, Zhang M L, Sun A J, Pei C X 2021 Acta Phys. Sin. 70 030301
[5] 聂敏, 尚鹏钢, 杨光, 张美玲, 裴昌幸 2014 63 240303
 Google Scholar
Google Scholar
Nie M, Shang P G, Yang G, Zhang M L, Pei C X 2014 Acta Phys. Sin. 63 240303
 Google Scholar
Google Scholar
[6] 聂敏, 任杰, 杨光, 张美玲, 裴昌幸 2015 64 150301
 Google Scholar
Google Scholar
Nie M, Ren J, Yang G, Zhang M L, Pei C X 2015 Acta Phys. Sin. 64 150301
 Google Scholar
Google Scholar
[7] Zhao S C, Li W D, Shen Y, Yu Y H, Han X H, Zeng H, Cai M Q, Qian T, Wang S, Wang Z M, Xiao Y, Gu Y J 2019 Appl. Opt. 58 3902
 Google Scholar
Google Scholar
[8] Li D D, Shen Q, Chen W, Li Y, Han X, Yang K X, Xu Y, Lin J, Wang C Z, Yong H L, Liu W Y, Cao Y, Yin J, Liao S K, Ren J G 2019 Opt. Commun. 452 220
 Google Scholar
Google Scholar
[9] Allen L, Beijersbergen M W, Spreeuw R J C, Woerdman J P 1992 Phys. Rev. A 45 8185
 Google Scholar
Google Scholar
[10] Molina-Terriza G, Torres J P, Torner L 2002 Phys. Rev. Lett. 88 013601
[11] Gibson G, Courtial J, Padgett M J, Vasnetsov M, Pas'ko V, Barnett S M, Franke-Arnold S 2004 Opt. Express 12 5448
 Google Scholar
Google Scholar
[12] Barreiro J T, Wei T C, Kwiat P G 2008 Nature Phys. 4 282
 Google Scholar
Google Scholar
[13] Bechmann-Pasquinucci H, Peres A 2000 Phys. Rev. Lett. 85 3313
 Google Scholar
Google Scholar
[14] Spedalieri F M 2006 Opt. Commun. 260 340
 Google Scholar
Google Scholar
[15] Paterson C 2005 Phys. Rev. Lett. 94 153901
 Google Scholar
Google Scholar
[16] Ibrahim A H, Roux F S, McLaren M, Konrad T, Forbes A 2013 Phys. Rev. A 88 012312
 Google Scholar
Google Scholar
[17] Bouchard F, Sit A, Hufnagel F, Abbas A, Zhang Y W, Heshami K, Fickler R, Marquardt C, Leuchs G, Boyd R W, Karimi E 2018 Opt. Express 26 22563
 Google Scholar
Google Scholar
[18] 胡涛, 潘孙翔, 王乐, 赵生妹 2018 量子电子学报 35 499
Hu T, Pan S X, Wang L, Zhang S M Chin. J. Quantum Electron. 35 499 (in Chinese)
[19] Cheng M J, Guo L X, Li J T, Huang Q Q, Cheng Q, Zhang D 2016 Appl. Opt. 55 4642
 Google Scholar
Google Scholar
[20] Elamassie M, Uysal M, Baykal Y, Abdallah M, Qaraqe K 2017 J. Opt. Soc. Am. A 34 1969
 Google Scholar
Google Scholar
[21] Andrews L C, Phillips R L 2005 Laser Beam Propagation through Random Media (Bellingham, Washington USA: SPIE Press) pp192−206
[22] 吴彤, 季小玲, 李晓庆, 王欢, 邓宇, 丁洲林 2018 67 224206
 Google Scholar
Google Scholar
Wu T, Ji X L, Li X Q, Wang H, Deng Y, Ding Z L 2018 Acta Phys. Sin. 67 224206
 Google Scholar
Google Scholar
[23] Fried D L 1966 J. Opt. Soc. Am. 56 1372
 Google Scholar
Google Scholar
[24] Alonso J R G, Brun T A 2013 Phys. Rev. A 88 022326
 Google Scholar
Google Scholar
[25] Lo H K, Curty M, Qi B 2012 Phys. Rev. Lett. 108 130503
 Google Scholar
Google Scholar
[26] Wang L, Zhao S M, Gong L Y, Cheng W W 2015 Chin. Phys. B 24 120307
 Google Scholar
Google Scholar
[27] Fickler R, Lapkiewicz R, Plick W N, Krenn M, Schaeff C, Ramelow S, Zeilinger A 2012 Science 338 640
 Google Scholar
Google Scholar
[28] Leonhard N D, Shatokhin V N, Buchleitner A 2015 Phys. Rev. A 91 012345
 Google Scholar
Google Scholar
[29] Wootters W K 1998 Phys. Rev. Lett. 80 2245
 Google Scholar
Google Scholar
[30] Yu T, Eberly J H 2006 Opt. Commun. 264 393
 Google Scholar
Google Scholar
[31] Gao F, Qin S J, Huang W, Wen Q Y 2019 Sci. China-Phys. Mech. Astron. 62 070301
 Google Scholar
Google Scholar
-
表 1 密钥分发系统的仿真参数值
Table 1. Simulation parameters of key distribution system
Parameter $ {P_d} $ f $ {e_d} $ $ \mu (\upsilon ) $ Value $ 3.0 \times {10^{ - 6}} $ $ 1.16 $ 1.5% $ 0.1 $ -
[1] Jin X M, Ren J G, Yang B, Yi Z H, Zhou F, Xu X F, Wang S K, Yang D, Hu Y F, Jiang S, Yang T, Yin H, Chen K, Peng C Z, Pan J W 2010 Nat. Photonics 4 376
 Google Scholar
Google Scholar
[2] Ji L, Gao J, Yang A L, Feng Z, Lin X F, Li Z G, Jin X M 2017 Opt. Express 25 19795
 Google Scholar
Google Scholar
[3] 聂敏, 王允, 杨光, 张美玲, 裴昌幸 2016 65 020303
 Google Scholar
Google Scholar
Nie M, Wang Y, Yang G, Zhang M L, Pei C X 2016 Acta Phys. Sin. 65 020303
 Google Scholar
Google Scholar
[4] 聂敏, 王超旭, 杨光, 张美玲, 孙爱晶, 裴昌幸 2021 70 030301
Nie M, Wang C X, Yang G, Zhang M L, Sun A J, Pei C X 2021 Acta Phys. Sin. 70 030301
[5] 聂敏, 尚鹏钢, 杨光, 张美玲, 裴昌幸 2014 63 240303
 Google Scholar
Google Scholar
Nie M, Shang P G, Yang G, Zhang M L, Pei C X 2014 Acta Phys. Sin. 63 240303
 Google Scholar
Google Scholar
[6] 聂敏, 任杰, 杨光, 张美玲, 裴昌幸 2015 64 150301
 Google Scholar
Google Scholar
Nie M, Ren J, Yang G, Zhang M L, Pei C X 2015 Acta Phys. Sin. 64 150301
 Google Scholar
Google Scholar
[7] Zhao S C, Li W D, Shen Y, Yu Y H, Han X H, Zeng H, Cai M Q, Qian T, Wang S, Wang Z M, Xiao Y, Gu Y J 2019 Appl. Opt. 58 3902
 Google Scholar
Google Scholar
[8] Li D D, Shen Q, Chen W, Li Y, Han X, Yang K X, Xu Y, Lin J, Wang C Z, Yong H L, Liu W Y, Cao Y, Yin J, Liao S K, Ren J G 2019 Opt. Commun. 452 220
 Google Scholar
Google Scholar
[9] Allen L, Beijersbergen M W, Spreeuw R J C, Woerdman J P 1992 Phys. Rev. A 45 8185
 Google Scholar
Google Scholar
[10] Molina-Terriza G, Torres J P, Torner L 2002 Phys. Rev. Lett. 88 013601
[11] Gibson G, Courtial J, Padgett M J, Vasnetsov M, Pas'ko V, Barnett S M, Franke-Arnold S 2004 Opt. Express 12 5448
 Google Scholar
Google Scholar
[12] Barreiro J T, Wei T C, Kwiat P G 2008 Nature Phys. 4 282
 Google Scholar
Google Scholar
[13] Bechmann-Pasquinucci H, Peres A 2000 Phys. Rev. Lett. 85 3313
 Google Scholar
Google Scholar
[14] Spedalieri F M 2006 Opt. Commun. 260 340
 Google Scholar
Google Scholar
[15] Paterson C 2005 Phys. Rev. Lett. 94 153901
 Google Scholar
Google Scholar
[16] Ibrahim A H, Roux F S, McLaren M, Konrad T, Forbes A 2013 Phys. Rev. A 88 012312
 Google Scholar
Google Scholar
[17] Bouchard F, Sit A, Hufnagel F, Abbas A, Zhang Y W, Heshami K, Fickler R, Marquardt C, Leuchs G, Boyd R W, Karimi E 2018 Opt. Express 26 22563
 Google Scholar
Google Scholar
[18] 胡涛, 潘孙翔, 王乐, 赵生妹 2018 量子电子学报 35 499
Hu T, Pan S X, Wang L, Zhang S M Chin. J. Quantum Electron. 35 499 (in Chinese)
[19] Cheng M J, Guo L X, Li J T, Huang Q Q, Cheng Q, Zhang D 2016 Appl. Opt. 55 4642
 Google Scholar
Google Scholar
[20] Elamassie M, Uysal M, Baykal Y, Abdallah M, Qaraqe K 2017 J. Opt. Soc. Am. A 34 1969
 Google Scholar
Google Scholar
[21] Andrews L C, Phillips R L 2005 Laser Beam Propagation through Random Media (Bellingham, Washington USA: SPIE Press) pp192−206
[22] 吴彤, 季小玲, 李晓庆, 王欢, 邓宇, 丁洲林 2018 67 224206
 Google Scholar
Google Scholar
Wu T, Ji X L, Li X Q, Wang H, Deng Y, Ding Z L 2018 Acta Phys. Sin. 67 224206
 Google Scholar
Google Scholar
[23] Fried D L 1966 J. Opt. Soc. Am. 56 1372
 Google Scholar
Google Scholar
[24] Alonso J R G, Brun T A 2013 Phys. Rev. A 88 022326
 Google Scholar
Google Scholar
[25] Lo H K, Curty M, Qi B 2012 Phys. Rev. Lett. 108 130503
 Google Scholar
Google Scholar
[26] Wang L, Zhao S M, Gong L Y, Cheng W W 2015 Chin. Phys. B 24 120307
 Google Scholar
Google Scholar
[27] Fickler R, Lapkiewicz R, Plick W N, Krenn M, Schaeff C, Ramelow S, Zeilinger A 2012 Science 338 640
 Google Scholar
Google Scholar
[28] Leonhard N D, Shatokhin V N, Buchleitner A 2015 Phys. Rev. A 91 012345
 Google Scholar
Google Scholar
[29] Wootters W K 1998 Phys. Rev. Lett. 80 2245
 Google Scholar
Google Scholar
[30] Yu T, Eberly J H 2006 Opt. Commun. 264 393
 Google Scholar
Google Scholar
[31] Gao F, Qin S J, Huang W, Wen Q Y 2019 Sci. China-Phys. Mech. Astron. 62 070301
 Google Scholar
Google Scholar
计量
- 文章访问数: 7650
- PDF下载量: 147
- 被引次数: 0













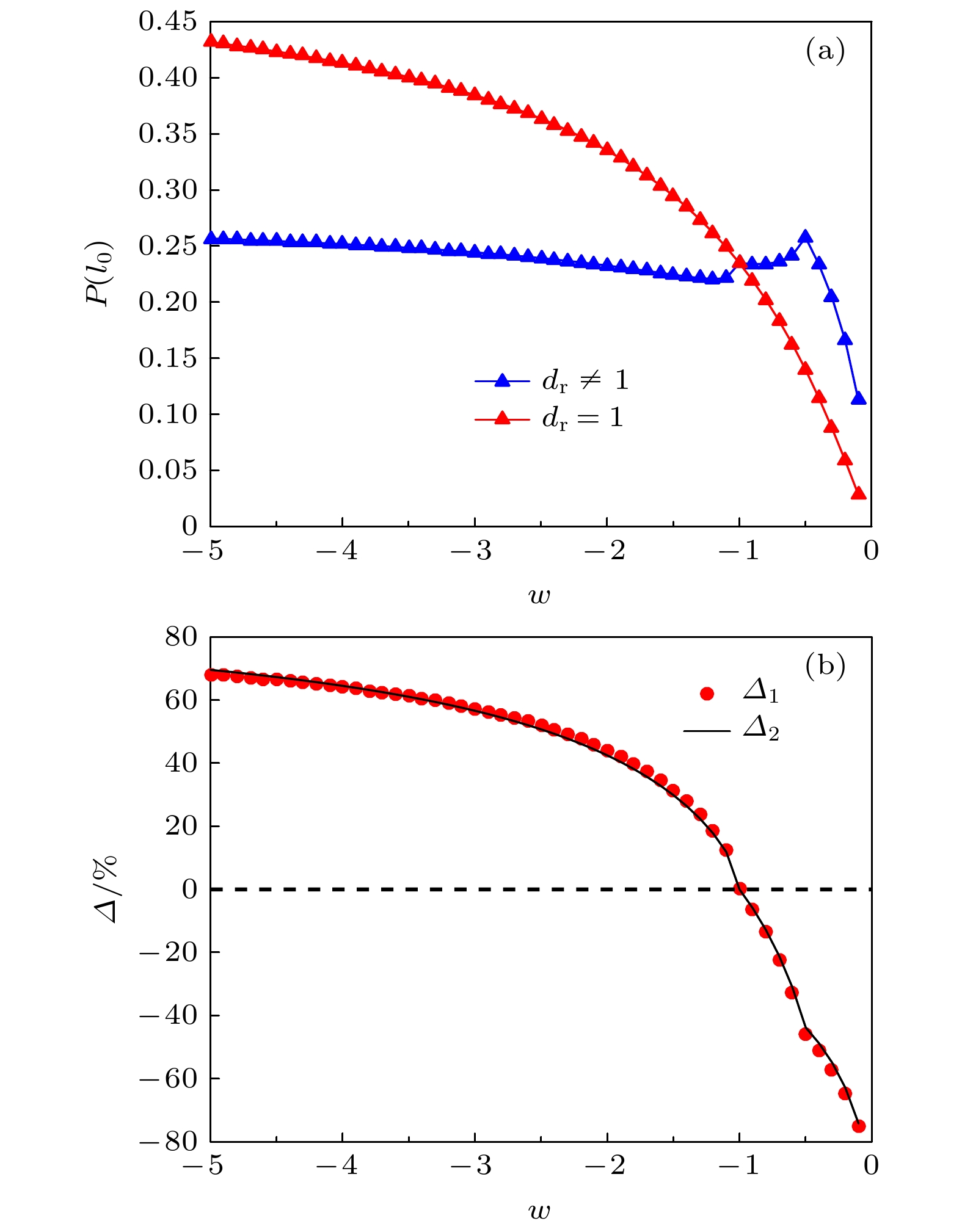
 下载:
下载: