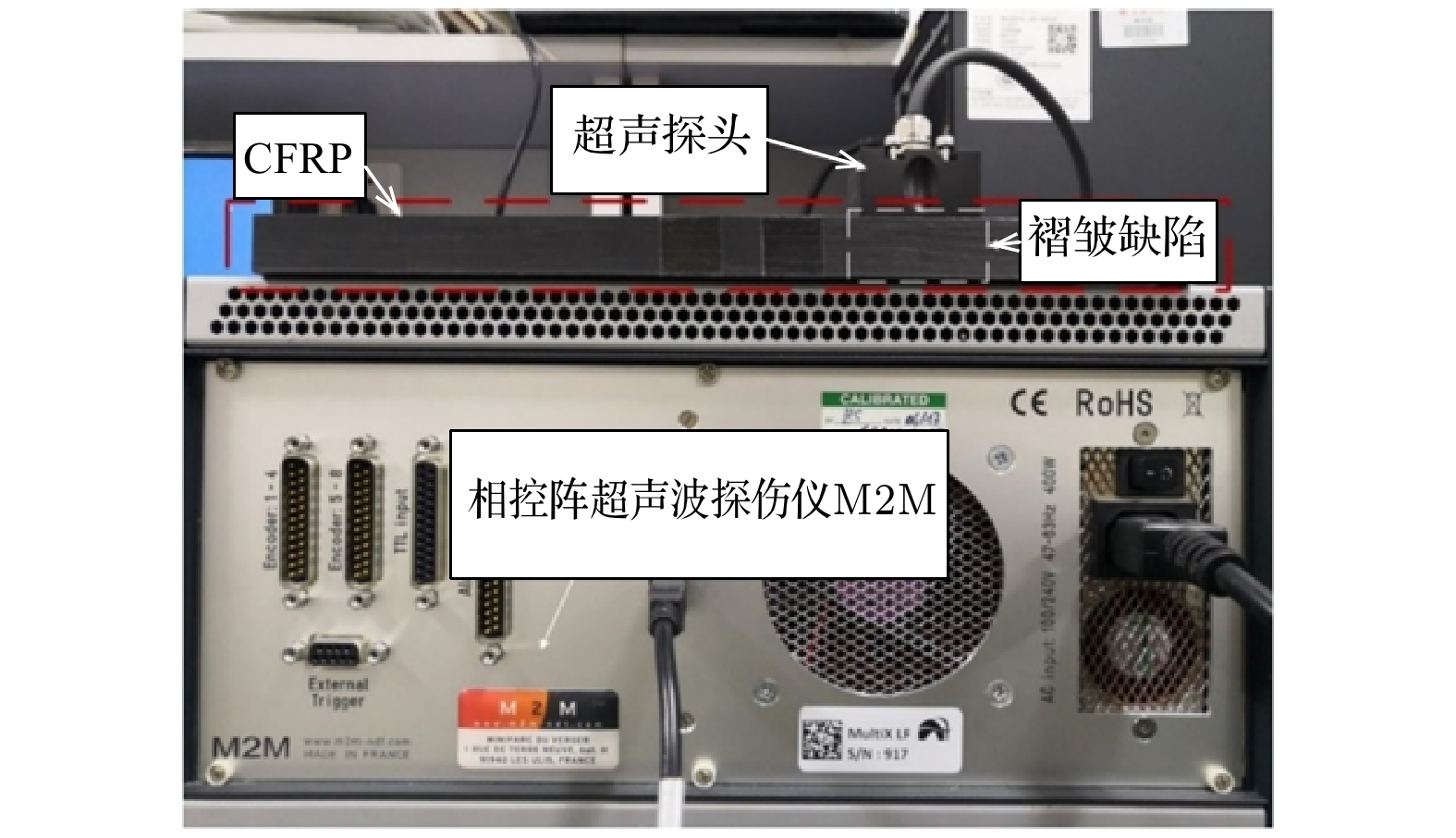
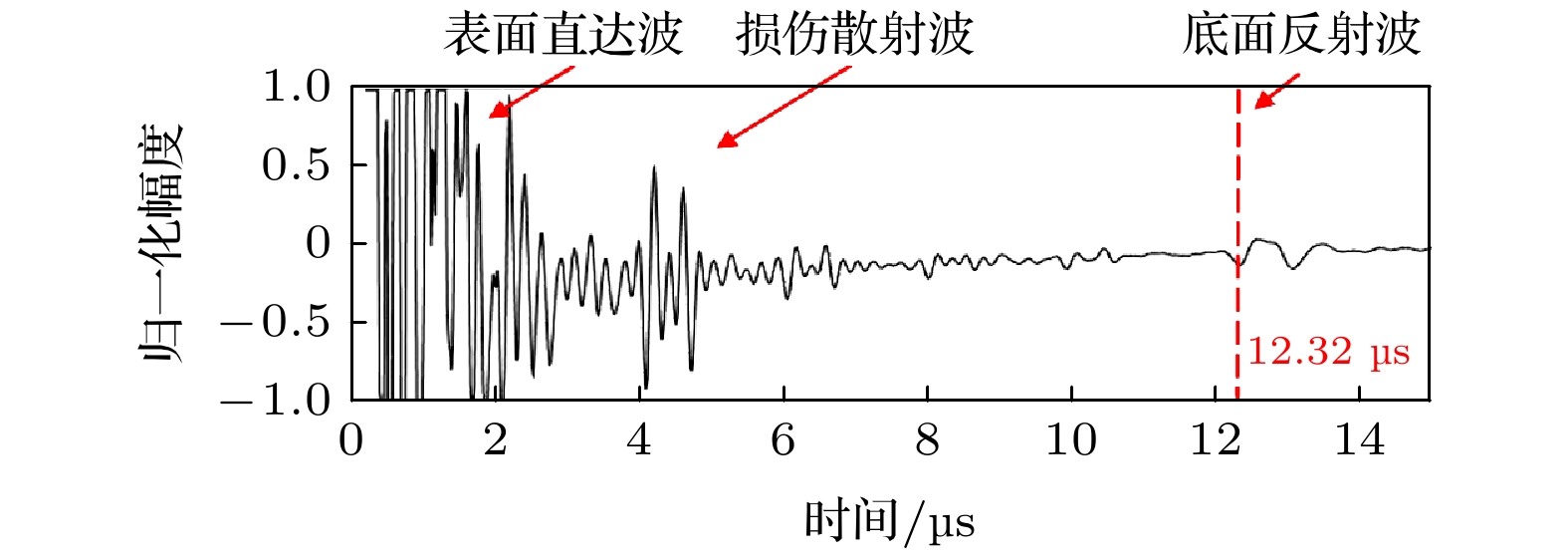
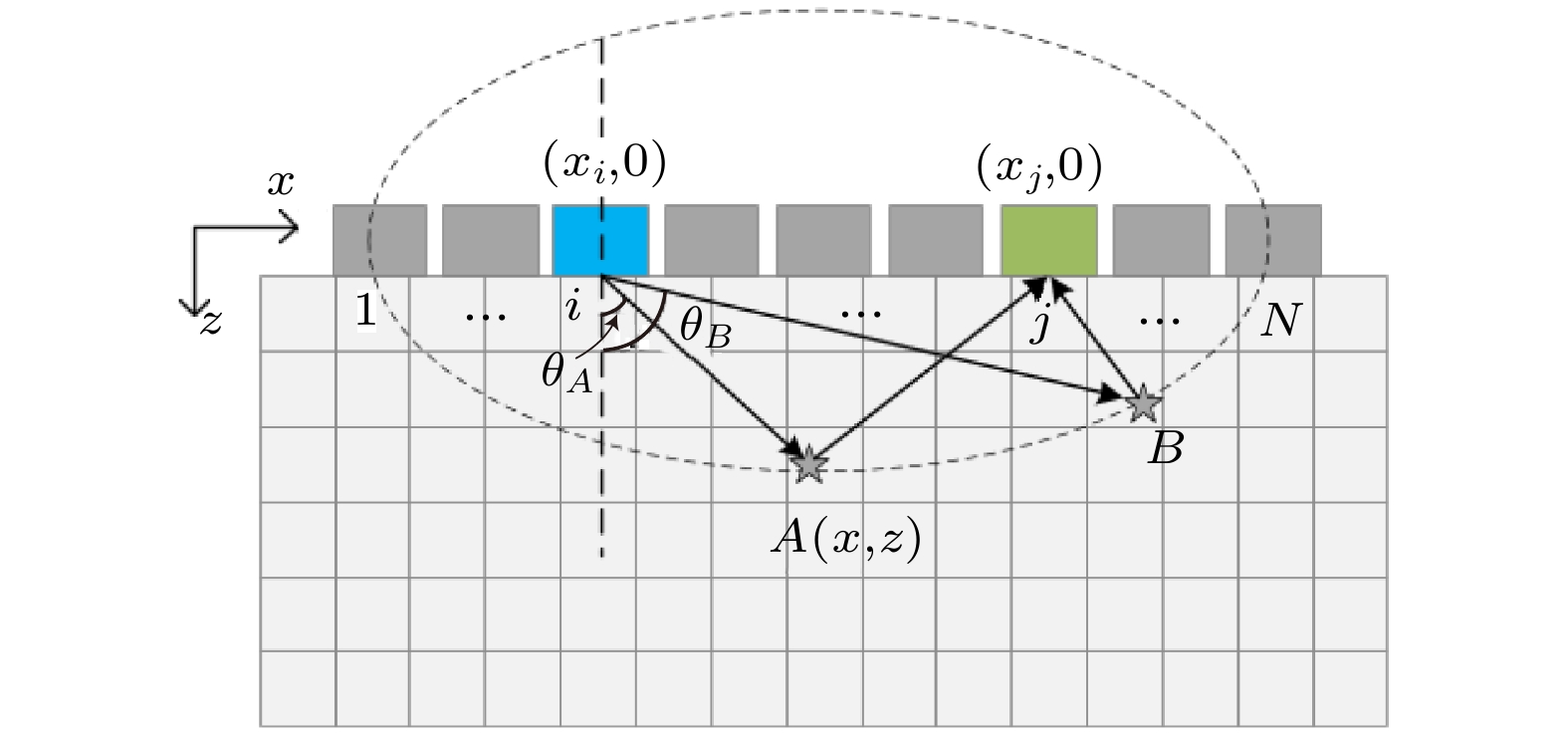
-
碳纤维增强复合材料在制造和使用过程中会产生褶皱, 褶皱缺陷的存在使得纤维层起伏不平, 超声波传播至纤维界面时不同声束方向的声能量存在差异. 针对此问题, 提出一种基于声束指向性函数校正的全聚焦阵列成像方法: 制备了含褶皱缺陷的碳纤维增强复合材料试样, 建立超声阵列数据采集实验系统, 捕获全矩阵数据; 考虑超声发射/接收阵元不同声束方向的声能量差异, 提出全聚焦成像方法的校正模型; 将校正前后的全聚焦成像结果与试样的实物图和光学显微镜图像进行对比分析. 实验结果表明: 基于指向性函数校正的全聚焦成像方法能够有效降低背景噪声, 恢复出被检试样的褶皱缺陷和铺层结构等细节信息, 为碳纤维增强复合材料褶皱缺陷的精确表征提供了理论指导.
Carbon-fiber-reinforced-polymer (CFRP) composites are widely used in aerospace, automobile and other industries because of their excellent performances. However, wrinkles will be formed during the manufacturing and service life process of composites, which is detrimental to the fatigue limit and tensile strength. Due to the fiber waviness, the presence of such defects leads to the acoustic energy difference in different beam directions during ultrasonic nondestructive testing. The directly obtained imaging amplitude cannot characterize the wrinkle properly. In order to solve this problem, the total focusing method (TFM) imaging combing the acoustic beam directivity function correction is presented. Firstly, the longitudinal wave signal is excited by an ultrasonic phased array, from which the echo data is collected by full matrix capture (FMC) in both the healthy and defected samples prepared. Then, considering the difference in acoustic energy from different beam directions of each ultrasonic transmitting/receiving array element, a correction TFM is proposed. Finally, the echo signals are post processed while the results are compared with the optical images. No wrinkle information can be indicated from the direct TFM imaging. In contrast, the wrinkles and layer-up information can be recovered from the corrected TFM imaging with an improved signal-to-noise ratio and resolution. Furthermore, owing to the directivity function, the near-surface noise can be effectively reduced and the near-surface fibrous layer information is restored. This method paves the way for the accurate, quantitative and rapid characterizing of wrinkles in real CFRP structures. -
Keywords:
- ultrasonic phased array /
- wrinkle defects /
- total focus method imaging /
- directional correction
[1] 彭金涛, 任天斌 2014 中国胶粘剂 23 48
Peng J T, Ren J T 2014 China Adhes. 23 48
[2] 魏莹莹 2016 博士学位论文 (上海: 上海交通大学)
Wei Y Y 2016 Ph. D. Dissertation (Shanghai: Shanghai Jiao Tong University) (in Chinese)
[3] 周典瑞, 高亮, 霍红宇, 张宝艳 2020 复合材料学报 37 1785
Zhou D R, Gao L, Huo H Y, Zhang B Y 2020 Acta Mater. Compos. Sin. 37 1785
[4] 邢丽英, 冯志海, 包建文, 礼嵩明 2020 复合材料学报 37 2700
Xing L Y, Feng Z H, Bao J W, Li S M 2020 Acta Mater. Compos. Sin. 37 2700
[5] Sun S P, Liu F L, Xue S, ZengM, Zeng F X 2015 Renew. Sust. Energ. Rev. 45 589
Google Scholar
[6] Hagstrand P O, Bonjour F, Manson J A E 2004 Composites Part A 36 705
[7] 张学辉, 乔英杰, 徐宏涛, 刘瑞良 2019 中国海洋平台 34 9
Google Scholar
Zhang X H, Qiao Y J, Xu H T, Liu R L 2019 China Offshore Platform 34 9
Google Scholar
[8] Zeng Z W, Liao Y F, Liu X H, Lin J M, Dai Y H 2020 IEEE Trans. Instrum. Meas. 69 5755
Google Scholar
[9] Hsiao H M, Daniel I M 1996 Compos. Sci. Technol. 56 581
Google Scholar
[10] Dattoma V, Gambino B, Nobile R, Panella F W 2018 Procedia Struct. Integrity 8 444
Google Scholar
[11] Zhen Z, Shifeng G, Qian L, Fangsen C, Andrew A M, Zhongqing S, Menglong L 2020 Compos. Sci. Technol. 189 1
[12] Wang X Y, He J J, Guo W H, Guan X F 2020 Ultrasonics 110 1
[13] Nelson L J, Smith R A 2019 Composites Part A 118 1
Google Scholar
[14] Holmes C, Drinkwater B W, Wilcox P D 2005 NDT&E Int. 38 701
[15] Pain D, Drinkwater B W 2013 Nondestruct Eval 32 215
Google Scholar
[16] Beatriz L V, Smith R A, Tayong R B, Antonio F L, Alfredo G 2018 Composites Part A 114 225
Google Scholar
[17] Wilcox P D, Holmes C, Drinkwater B W 2007 IEEE Trans. Ultrason. Ferroelectr. Freq. Control 54 1541
Google Scholar
[18] 周正干, 彭地, 李洋, 胡宏伟 2015 机械工程学报 51 1
Zhou Z G, Peng D, Li Y, Hu H W 2015 J. Mech. Eng. 51 1
[19] Lin L, Cao H Q, Luo Z B 2018 NDT and E Int. 97 51
Google Scholar
[20] 贾乐成, 陈世利, 白志亮, 曾周末, 杨晓霞 2017 仪器仪表学报 38 1589
Google Scholar
Jia L C, Chen S L, Bai Z L, Zeng Z M, Yang X X 2017 Chinese Journal of Scientific Instrument 38 1589
Google Scholar
[21] Selfridge A R, Kino G S, Khuri-Yakub B T 1980 Appl. Phys. Lett. 37 35
Google Scholar
[22] Tasinkevych Y, Trots I, Nowicki A, Lewin P A 2012 Ultrasonics 52 333
Google Scholar
[23] 滕国阳 2019 博士学位论文 (浙江: 浙江大学)
Teng G Y 2019 Ph. D. Dissertation (Zhejiang: Zhejiang University) (in Chinese)
[24] Smith R A, Nelson L J, Mienczakowski M J, Wilcox P D 2018 IEEE Trans. Ultrason. Ferroelectr. Freq. Control 65 231
Google Scholar
-
图 3 CFRP显微镜图 (a)无褶皱; (b)有褶皱
Fig. 3. Microscopic image of CFRP: (a) No wrinkles; (b) wrinkles.
图 6 试样A及其成像结果 (a)实物图; (b)图6(a)的局部放大图; (c)全聚焦成像结果; (d)图6(c)的灰度图; (e)用指向性函数校正的全聚焦成像结果; (f)图6(e)的灰度图
Fig. 6. Sample A and imaging results: (a) Sample A; (b) partial enlarged view of Fig.6 (a); (c) total focus method; (d) gray scale of Fig.6 (c); (e) total focus method corrected by directivity function; (f) gray scale of Fig.6 (e).
图 7 试样B及其成像结果 (a)实物图; (b)图7(a)的局部放大图; (c)全聚焦成像结果; (d)图7(c)的灰度图; (e)用指向性函数校正的全聚焦成像结果; (f)图7(e)的灰度图
Fig. 7. Sample B and its imaging results: (a) Sample B; (b) partial enlarged view of Fig.7(a); (c) total focus method; (d) gray scale of Fig.7 (c); (e) total focus method corrected by directivity function; (f) gray scale of Fig.7 (e).
图 8 试样C及其成像结果 (a)实物图; (b)图8(a)的局部放大图; (c)全聚焦成像结果; (d)图8(c)的灰度图; (e)用指向性函数校正的全聚焦成像结果; (f)图8(e)的灰度图
Fig. 8. Sample C and its imaging results: (a) Sample C; (b) partial enlarged view of Fig. 8 (a); (c) total focus method; (d) gray scale of Fig. 8 (c); (e) total focus method corrected by directivity function; (f) gray scale of Fig. 8 (e).
表 1 超声相控阵参数配置
Table 1. Parameters configuration of ultrasonic phased array.
参数 值 参数 值 阵元个数 32 阵元宽度/mm 0.9 阵元中心距/mm 1.0 采样频率/MHz 50 中心频率/MHz 5 激励方式 纵波 
下载: 导出CSV
-
[1] 彭金涛, 任天斌 2014 中国胶粘剂 23 48
Peng J T, Ren J T 2014 China Adhes. 23 48
[2] 魏莹莹 2016 博士学位论文 (上海: 上海交通大学)
Wei Y Y 2016 Ph. D. Dissertation (Shanghai: Shanghai Jiao Tong University) (in Chinese)
[3] 周典瑞, 高亮, 霍红宇, 张宝艳 2020 复合材料学报 37 1785
Zhou D R, Gao L, Huo H Y, Zhang B Y 2020 Acta Mater. Compos. Sin. 37 1785
[4] 邢丽英, 冯志海, 包建文, 礼嵩明 2020 复合材料学报 37 2700
Xing L Y, Feng Z H, Bao J W, Li S M 2020 Acta Mater. Compos. Sin. 37 2700
[5] Sun S P, Liu F L, Xue S, ZengM, Zeng F X 2015 Renew. Sust. Energ. Rev. 45 589
Google Scholar
[6] Hagstrand P O, Bonjour F, Manson J A E 2004 Composites Part A 36 705
[7] 张学辉, 乔英杰, 徐宏涛, 刘瑞良 2019 中国海洋平台 34 9
Google Scholar
Zhang X H, Qiao Y J, Xu H T, Liu R L 2019 China Offshore Platform 34 9
Google Scholar
[8] Zeng Z W, Liao Y F, Liu X H, Lin J M, Dai Y H 2020 IEEE Trans. Instrum. Meas. 69 5755
Google Scholar
[9] Hsiao H M, Daniel I M 1996 Compos. Sci. Technol. 56 581
Google Scholar
[10] Dattoma V, Gambino B, Nobile R, Panella F W 2018 Procedia Struct. Integrity 8 444
Google Scholar
[11] Zhen Z, Shifeng G, Qian L, Fangsen C, Andrew A M, Zhongqing S, Menglong L 2020 Compos. Sci. Technol. 189 1
[12] Wang X Y, He J J, Guo W H, Guan X F 2020 Ultrasonics 110 1
[13] Nelson L J, Smith R A 2019 Composites Part A 118 1
Google Scholar
[14] Holmes C, Drinkwater B W, Wilcox P D 2005 NDT&E Int. 38 701
[15] Pain D, Drinkwater B W 2013 Nondestruct Eval 32 215
Google Scholar
[16] Beatriz L V, Smith R A, Tayong R B, Antonio F L, Alfredo G 2018 Composites Part A 114 225
Google Scholar
[17] Wilcox P D, Holmes C, Drinkwater B W 2007 IEEE Trans. Ultrason. Ferroelectr. Freq. Control 54 1541
Google Scholar
[18] 周正干, 彭地, 李洋, 胡宏伟 2015 机械工程学报 51 1
Zhou Z G, Peng D, Li Y, Hu H W 2015 J. Mech. Eng. 51 1
[19] Lin L, Cao H Q, Luo Z B 2018 NDT and E Int. 97 51
Google Scholar
[20] 贾乐成, 陈世利, 白志亮, 曾周末, 杨晓霞 2017 仪器仪表学报 38 1589
Google Scholar
Jia L C, Chen S L, Bai Z L, Zeng Z M, Yang X X 2017 Chinese Journal of Scientific Instrument 38 1589
Google Scholar
[21] Selfridge A R, Kino G S, Khuri-Yakub B T 1980 Appl. Phys. Lett. 37 35
Google Scholar
[22] Tasinkevych Y, Trots I, Nowicki A, Lewin P A 2012 Ultrasonics 52 333
Google Scholar
[23] 滕国阳 2019 博士学位论文 (浙江: 浙江大学)
Teng G Y 2019 Ph. D. Dissertation (Zhejiang: Zhejiang University) (in Chinese)
[24] Smith R A, Nelson L J, Mienczakowski M J, Wilcox P D 2018 IEEE Trans. Ultrason. Ferroelectr. Freq. Control 65 231
Google Scholar
下载:
计量
- 文章访问数: 6421
- PDF下载量: 151
- 被引次数: 0
