基于飞秒光梳多路同步锁相的多波长干涉实时绝对测距及其非模糊度量程分析

王国超; 李星辉; 颜树华; 谭立龙; 管文良

doi:10.7498/aps.70.20201225

摘要

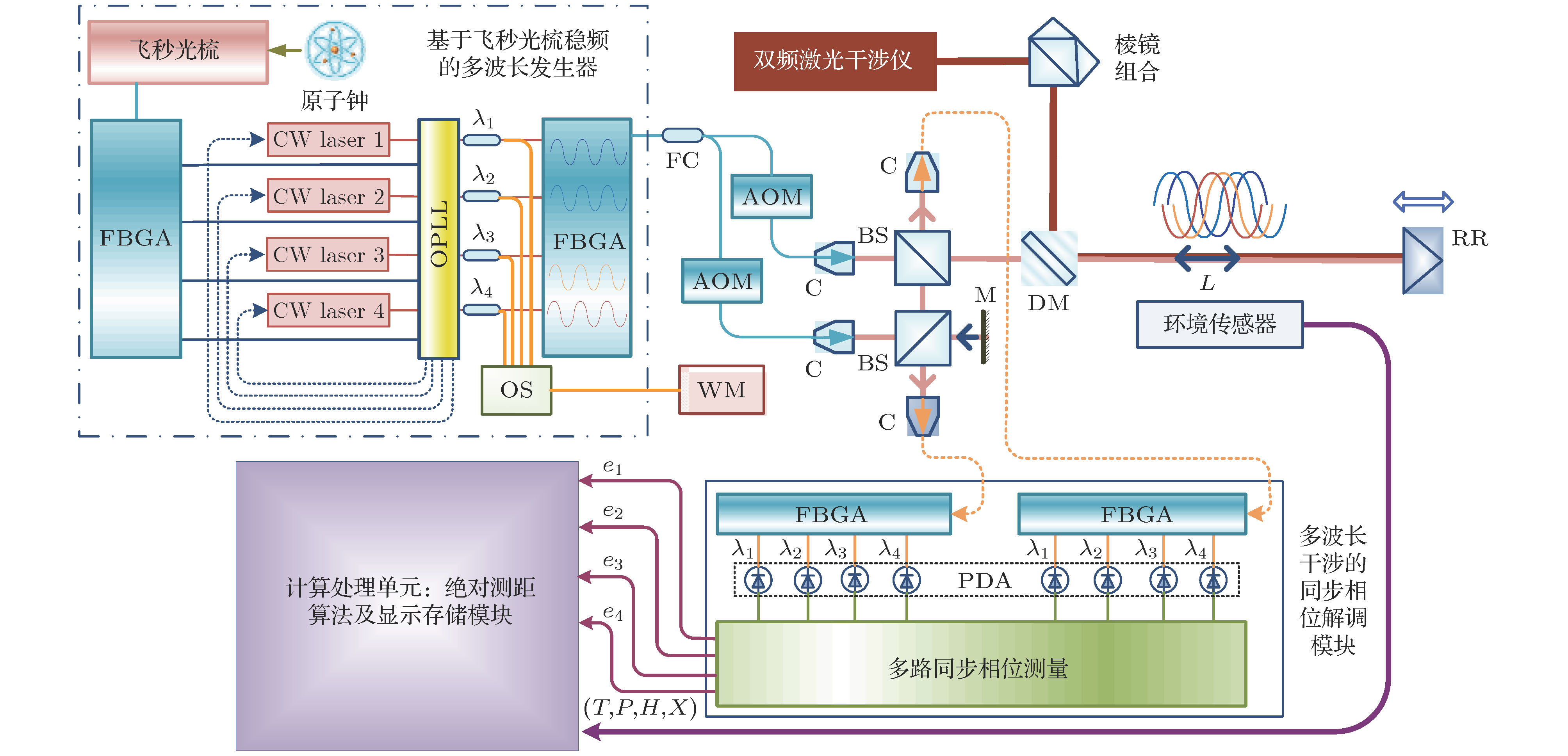
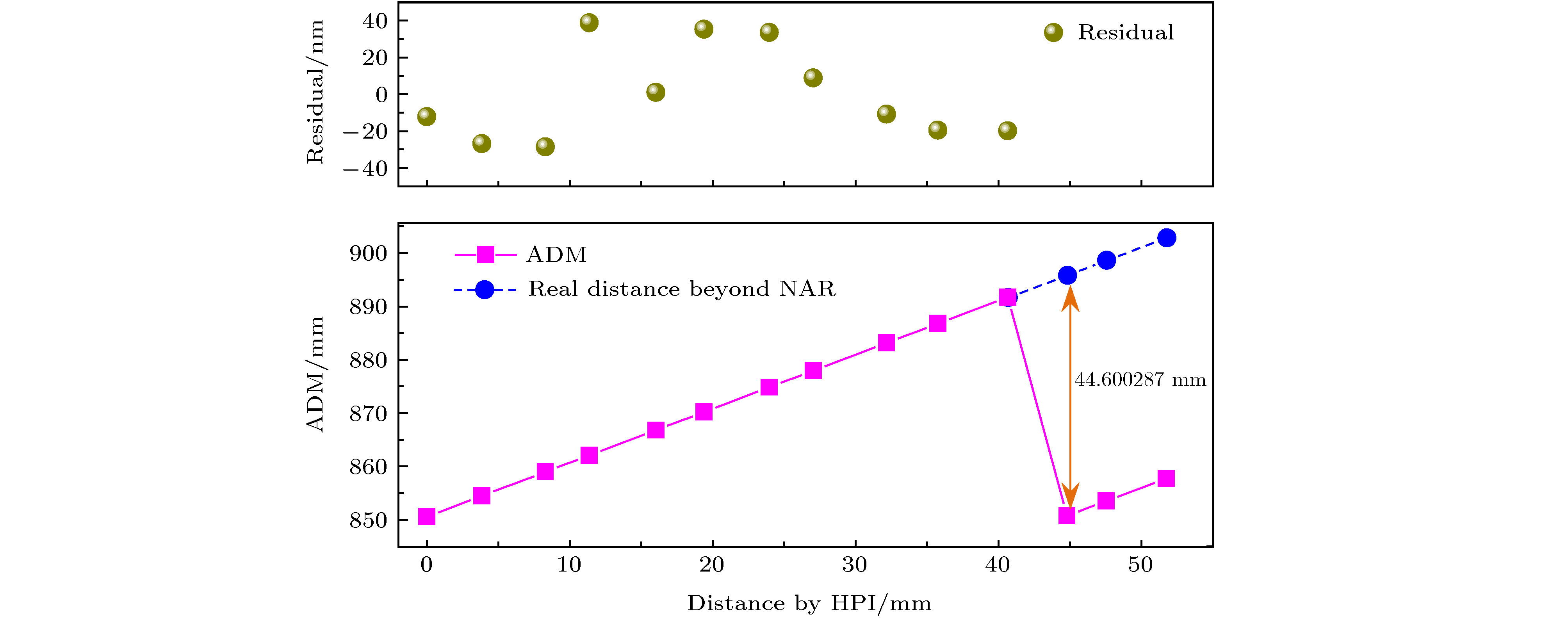
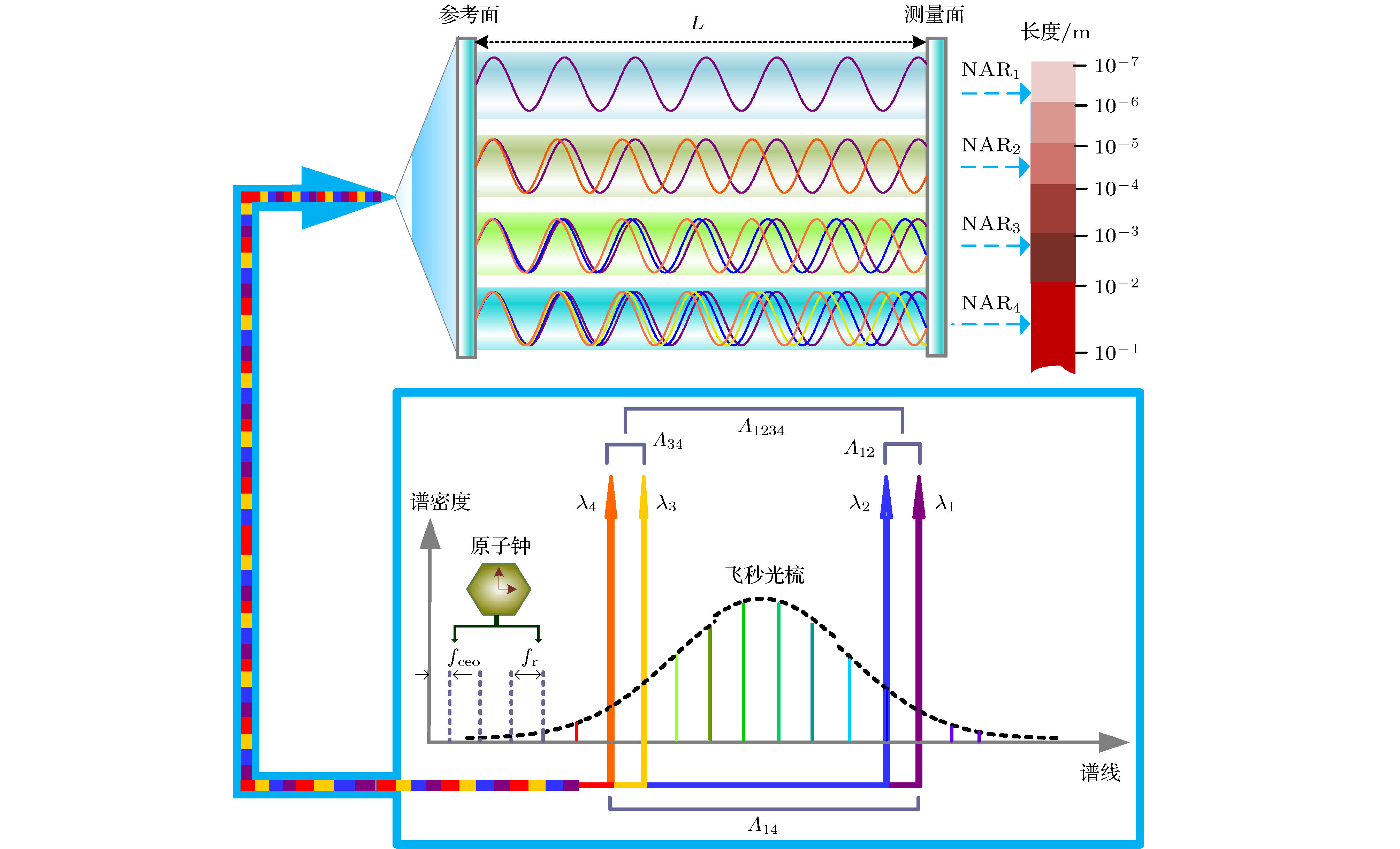
飞秒光梳被广泛用于时间频率技术和精密光谱测量, 由其时频特性所衍生的绝对测距技术以可溯源、大尺寸、高精度等优点有望成为未来长度计量的最重要手段. 本文提出了一种基于飞秒光梳多路同步锁相的多波长干涉实时绝对测距方法, 使多个连续波激光器通过光学锁相环技术同步锁定到飞秒光梳梳模上, 通过多路同步相位测量和小数重合算法最终实现绝对距离测量. 所提测量方法不仅能保留传统激光干涉测距的高分辨力和精度, 而且可溯源至时间频率基准, 对高精度长度测量、尤其是对物理复现“米”的定义具有重要计量意义. 测距实验证明, 四波长干涉测距的非模糊度量程达到44.6 mm, 折射率波动导致非模糊度量程变化为纳米量级; 多波长干涉测距的非模糊度量程也受制于空气折射率的测量误差, 多波长干涉绝对测距的非模糊度量程在实验室环境下可达数米、甚至几十米, 并通过2米线性位移实验证明了多波长绝对测距的大量程和线性测量性能.

关键词:

Abstract

Optical frequency combs of the femtosecond laser have been widely used in time-frequency technology and precision spectrum measurement. The absolute ranging technology derived from time-frequency characteristics of the optical frequency comb is expected to become the incomparable means of length metrology and distance measurement in the future due to its traceability to time-frequency standard and capability of large scale and high precision. This paper proposes a real-time absolute ranging method with multi-wavelength interferometry referenced to optical frequency comb, which enables multiple continuous-wave lasers to be synchronously calibrated to selected modes of the frequency comb by means of optical phase-locked loop. With synchronous phase measurement and calculation with excess fraction algorithm, absolute distance measurement by multi-wavelength interferometry is ultimately fulfilled. The proposed measurement method can not only retain high resolution and high accuracy of traditional laser interferometry, but also can be traced to a time-frequency reference, which is of metrological significance to high-precision length and distance measurement, especially to the definition of “meter” for physical reproduction. Measured results for ranging experiments have proved that the non-ambiguity range of the four-wavelength interferometer reaches 44.6 mm, and fluctuations of air refractive index cause the non-ambiguity range change with the order of nanometers. Through theoretical analysis, it is pointed out that the non-ambiguity range of the multi-wavelength interferometer in the actual measurement environment is restricted by the calculated error of air refractive index, especially the estimation accuracy and fluctuation degree of the refractive index relationship between wavelengths. And in a good laboratory conditions, the non-ambiguity range of real-time absolute ranging by frequency-comb-calibrated multi-wavelength interferometry can reach several meters or even tens of meters. At the same time, a 2-meter linear displacement comparison has been carried out, the P.V. value of the residual errors for linear fitting is 36.1 nm, and such residual errors match the magnitude of uncertainty of air refractive index calculated by empirical formula, which prove that the multi-wavelength interferometry can perform meter-level absolute ranging. The proposed research can be directly applied to precision manufacturing of large-scale semiconductors up to several meters, and is beneficial to promoting the accuracy of laser ranging for space mission.

Keywords:

absolute distance measurement /
optical frequency comb of the femtosecond laser /
multi-wavelength interferometry /
non-ambiguous range

作者及机构信息

1.
西安高技术研究所, 西安　710025

2.
清华大学深圳国际研究生院, 深圳　518055

3.
国防科技大学智能科学学院, 长沙　410073

4.
国防科技大学, 量子信息学科交叉中心, 长沙　410073

通信作者: 王国超, wgc.19850414@163.com ; 李星辉, li.xinghui@sz.tsinghua.edu.cn ;

基金项目: 国家自然科学基金(批准号: 51705523)和陕西省自然科学基础研究计划资质项目(批准号: 2018JQ5026)资助的课题

Authors and contacts

1.
High-tec Institute of Xi’an, Xi’an 710025, China

2.
Shenzhen International Graduate School, Tsinghua University, Shenzhen 518055, China

3.
College of Intelligence Science and Technology, National University of Defense Technology, Changsha 410073, China

4.
Interdisciplinary Center for Quantum Information, National University of Defense Technology, Changsha 410073, China

Corresponding author: Wang Guo-Chao, wgc.19850414@163.com ; Li Xing-Hui, li.xinghui@sz.tsinghua.edu.cn ;

Funds: Project supported by the National Natural Science Foundation of China (Grant No. 51705523) and the Natural Science Basic Research Plan of Shaanxi Province, China (Grant No. 2018JQ5026)

文章全文 : translate this paragraph

参考文献

[1]	Berkovic G, Shafir E 2012 Adv. Opt. Photon. 4 441 Google Scholar
[2]	Estler W T, Edmundson K L, Peggs G N, Parker D H 2002 CIRP Ann. Manuf. Technol. 51 587 Google Scholar
[3]	Manske E, Jager G, Hausotte T, Fub R 2012 Meas. Sci. Technol. 23 074001 Google Scholar
[4]	Bobroff N 1993 Meas. Sci. Technol. 4 907 Google Scholar
[5]	Lay O P, Dubovitsky S, Peters R D, Burger J P, Steier W H 2003 Opt. Lett. 28 890 Google Scholar
[6]	Bourdet G L, Orszag A G 1979 Appl. Opt. 18 225 Google Scholar
[7]	Zimmermann E, Salvadé Y, Dändliker R 1996 Opt. Lett. 21 531 Google Scholar
[8]	Meiners-Hagen K, Schoedel R, Pollinger F, Abou-Zeid A 2009 Meas. Sci. Rev. 9 16 Google Scholar
[9]	Dändliker R, Thalmann R, Prongue D 1988 Opt. Lett. 13 339 Google Scholar
[10]	Groot P 2001 Opt. Eng. 40 28 Google Scholar
[11]	时光, 张福民, 曲兴华, 孟祥松 2014 63 184209 Google Scholar Shi G, Zhang F M, Qu X H, Meng X S 2014 Acta Phys. Sin. 63 184209 Google Scholar
[12]	Coe P A, Howell D F, Nickerson R B 2004 Meas. Sci. Technol. 15 2175 Google Scholar
[13]	Williams C C, Wickramasinghe H K 1989 Opt. Lett. 145 42 Google Scholar
[14]	Diddams S A, Jones D J, Ye J, Cundiff S T, Hall J L, Ranka J K, Windeler R S, Holzwarth R, Udem T, Hansch T W 2000 Phys. Rev. Lett. 84 5102 Google Scholar
[15]	Udem T, Holzwarth R, Hansch T W 2002 Nature 416 233 Google Scholar
[16]	Jones D J, Diddams S A, Ranka J K, Stentz A, Windeler R W, Hall J L, Cundiff S T 2000 Science 288 635 Google Scholar
[17]	Minoshima K, Matsumoto H 2000 Appl. Opt. 39 5512 Google Scholar
[18]	王国超, 颜树华, 杨俊, 林存宝, 杨东兴, 邹鹏飞 2013 62 070601 Google Scholar Wang G C, Yan S H, Yang J, Lin C B, Yang D X, Zou P F 2013 Acta Phys. Sin. 62 070601 Google Scholar
[19]	张晓声, 易旺民, 胡明皓, 杨再华, 吴冠豪 2016 65 080602 Google Scholar Zhang X S, Yi W M, Hu M H, Yang Z H, Wu G H 2016 Acta Phys. Sin. 65 080602 Google Scholar
[20]	Lee J, Kim Y J, Lee K, Lee S, Kim S W 2010 Nat. Photonics 4 716 Google Scholar
[21]	秦鹏, 陈伟, 宋有建, 胡明列, 柴路, 王清月 2012 61 240601 Google Scholar Qin P, Chen W, Song Y J, Hu M L, Chai L, Wang C Y 2012 Acta Phys. Sin. 61 240601 Google Scholar
[22]	Coddington I, Swann W C, Nenadovic L, Newbury N R 2009 Nat. Photonics 3 351 Google Scholar
[23]	Lee J, Han S, Lee K, Bae E, Kim S, Lee S, Kim S W, Kim Y J 2013 Meas. Sci. Technol. 24 045201 Google Scholar
[24]	Joo K N, Kim S W 2006 Opt. Express 14 5954 Google Scholar
[25]	Van den Berg S A, Persijn S T, Kok G, Zeitouny M G, Bhattacharya N 2012 Phys. Rev. Lett. 108 183901 Google Scholar
[26]	Wang G C, Jang Y S, Hyun S, Chun B J, Kang H J, Yan S H, Kim S W, Kim Y J 2015 Opt. Express 23 9121 Google Scholar
[27]	Hyun S, Kim Y J, Kim Y, Jin J, Kim S W 2009 Meas. Sci. Technol. 20 095302 Google Scholar
[28]	Jang Y S, Wang G C, Hyun S, Kang H J, Chun B J, Kim Y J, Kim S W 2016 Sci. Rep. 6 31770 Google Scholar
[29]	Ye J 2004 Opt. Lett. 29 1153 Google Scholar
[30]	Wei D, Takahashi S, Takamasu K, Matsumoto H 2009 Opt. Lett. 34 2775 Google Scholar
[31]	孟祥松, 张福民, 曲兴华 2015 23 230601 Google Scholar Meng X S, Zhang F M, Qu X H 2015 Acta Phys. Sin. 23 230601 Google Scholar
[32]	Kim S W 2009 Nat. Photonics 3 313 Google Scholar
[33]	姜海峰 2018 67 160602 Google Scholar Jiang H F 2018 Acta Phys. Sin. 67 160602 Google Scholar
[34]	Chun B J, Hyun S, Kim S, Kim S W, Kim Y J 2013 Opt. Express 21 29179 Google Scholar
[35]	Felder R 2003 Metrologia 42 323 Google Scholar
[36]	Wei D, Takamasu K, Matsumoto H 2013 Precis. Eng. 37 694 Google Scholar
[37]	Tilford C R 1977 Appl. Opt. 16 1857 Google Scholar
[38]	王国超, 魏春华, 颜树华 2014 光学学报 34 111 Google Scholar Wang G C, Wei C H, Yan S H 2014 Acta Optic. Sin. 34 111 Google Scholar
[39]	Falaggis K, Towers D P, Towers C E 2013 Appl. Opt. 52 5758 Google Scholar
[40]	Towers C E, Towers D P, Julian D C 2004 Opt. Express 12 1136 Google Scholar
[41]	Ma L, Zucco M, Picard S 2003 IEEE J. Sel. Top. Quantum Electron. 9 1066 Google Scholar
[42]	王国超, 谭立龙, 颜树华, 魏春华 2017 光学学报 37 160 Google Scholar Wang G C, Wei C H, Yan S H 2017 Acta Optic. Sin. 37 160 Google Scholar
[43]	Hyun S, Kim Y J, Kim Y, Kim S W 2010 CIRP Ann.-Manuf. Techn. 59 555 Google Scholar
[44]	王国超 2015 博士学位论文 (长沙: 国防科技大学) Wang G C 2015 Ph. D. Dissertation (Changsha: National University of Defense Technology) (in Chinese)
[45]	Ciddor P E 1996 Appl. Opt. 35 1566 Google Scholar
[46]	Birch K P, Downs M J 1993 Metrologia 30 155 Google Scholar
[47]	Wu G H, Takahashi M, Arai K, Inaba H, Minoshima K 2013 Sci. Rep. 3 1894 Google Scholar
[48]	Minoshima K, Arai K, Inaba H 2011 Opt. Express 19 26095 Google Scholar

施引文献

图 1 基于飞秒光梳多路同步锁相的多波长干涉测距原理及非模糊度量程示意图

Fig. 1. Schematic diagram of the synthetic wavelengths and measuring NAR of Frequency-Comb-Referenced Multi-Wavelength Interferometry.

下载: 全尺寸图片幻灯片

图 2 多波长干涉实时绝对测距系统. FBGA, 光纤光栅滤波阵列; OPLL, 光学锁相环; OS, 光开关; WM, 波长计; FC, 光纤耦合器; AOM, 声光调制器; C, 准直器; M, 反射镜; BS, 分光棱镜; DM, 双色镜; RR, 角锥反射镜; PDA, 光电探测器阵列

Fig. 2. Schematic configuration diagram of real-time absolute distance measurement by Frequency-Comb-Referenced Multi-Wavelength Interferometry.

下载: 全尺寸图片幻灯片

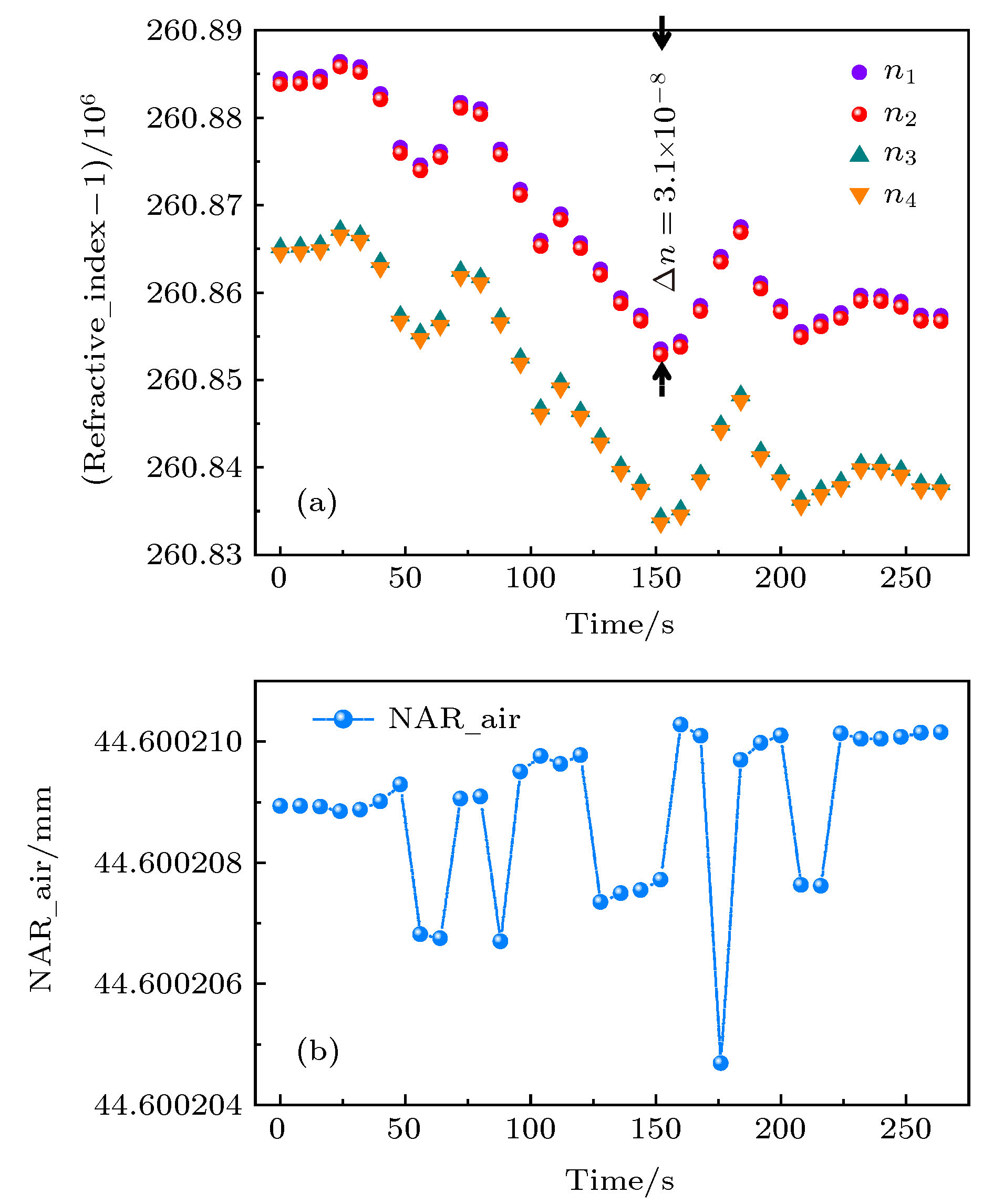
图 3 多波长光源和相位测量结果　(a) 多波长发生器光谱测量结果; (b) 锁频激光的频率稳定度分析结果; (c) 多路同步相位解调实时测量结果

Fig. 3. Test results for preparation of real-time and meter-scale absolute distance measurement: (a) Parallel generated four wavelengths for MWI; (b) frequency stability evaluation; (c) simultaneously detected phases for MWI in real time.

下载: 全尺寸图片幻灯片

图 4 验证NAR的线性位移对比实验

Fig. 4. Linear comparison between ADM by MWI and displacement by HPI for NAR demonstration.

下载: 全尺寸图片幻灯片

图 5 空气折射率变化对NAR的影响　(a) 空气折射率的波动情况; (b) 理论NAR受空气折射率影响的计算结果

Fig. 5. Influence of air refractive index on NAR: (a) Fluctuation of air refractive index for observation of 272 s;. (b) calculated result of theoretical NAR under the fluctuation of air refractive index.

下载: 全尺寸图片幻灯片

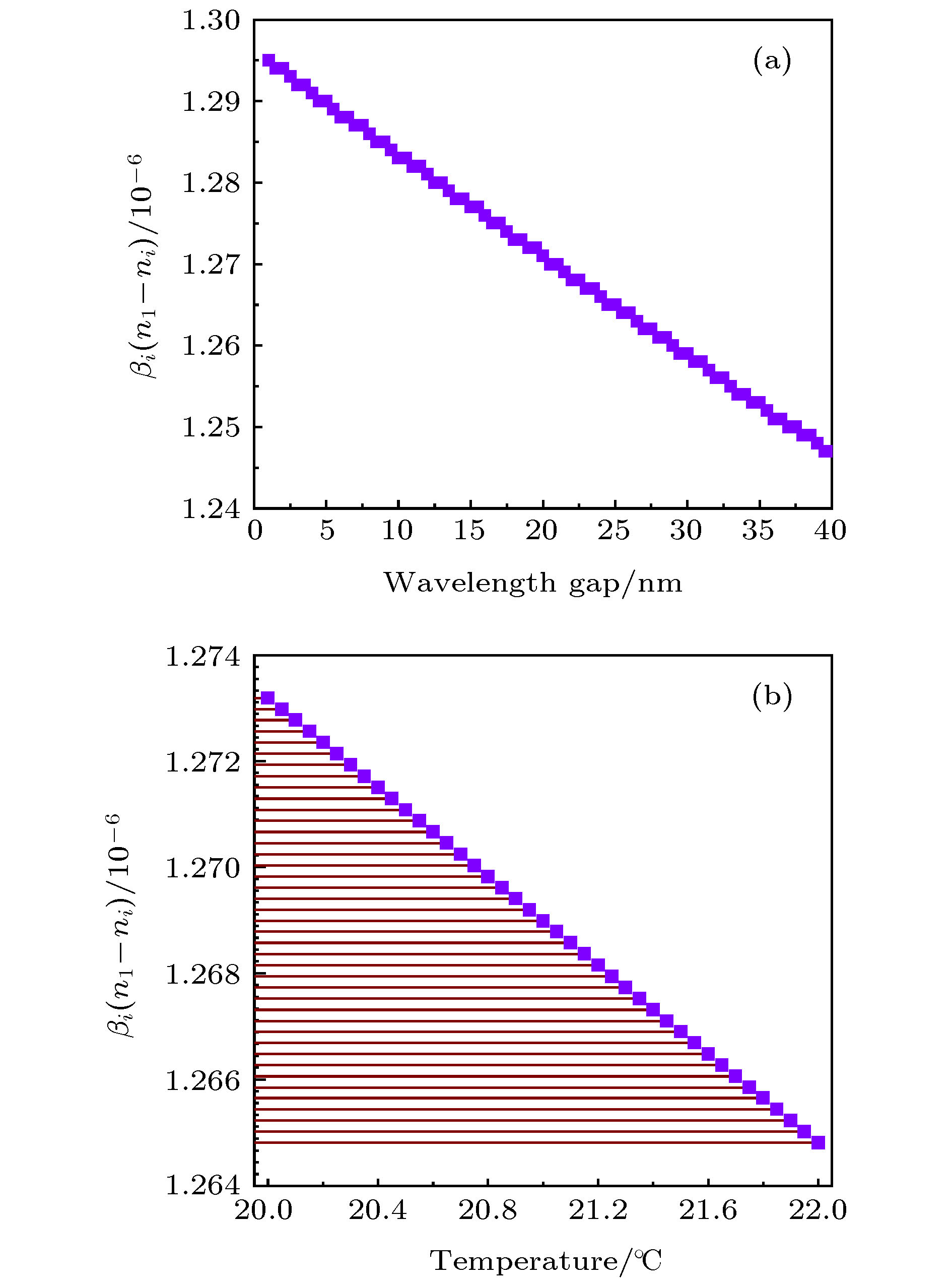
图 6 $\alpha = {\beta _i} \cdot \left( {{n_1} - {n_i}} \right)$随参数变化的波动大小　(a) $ {\beta _i} \cdot \left( {{n_1} - {n_i}} \right) $随波长间距变化的波动仿真结果; (b)${\beta _i} \cdot \left( {{n_1} - {n_i}} \right)$随温度变化时的波动仿真结果, 波长间隔为25 nm, 波长选定为1555 nm

Fig. 6. Influences of the parameter variations on the value of ${\beta _i} \cdot \left( {{n_1} - {n_i}} \right)$: (a) Fluctuation of ${\beta _i} \cdot \left( {{n_1} - {n_i}} \right)$ as variations of wavelength gap; (b) fluctuation of ${\beta _i} \cdot \left( {{n_1} - {n_i}} \right)$ as variations of ambient temperature.

下载: 全尺寸图片幻灯片

图 7 MWI与商用激光干涉仪的2 m线性位移对比实验结果

Fig. 7. Linear comparison between MWI and the commercial laser interferometer over a 2.0-meter displacement.

下载: 全尺寸图片幻灯片

map

[1]	Berkovic G, Shafir E 2012 Adv. Opt. Photon. 4 441 Google Scholar
[2]	Estler W T, Edmundson K L, Peggs G N, Parker D H 2002 CIRP Ann. Manuf. Technol. 51 587 Google Scholar
[3]	Manske E, Jager G, Hausotte T, Fub R 2012 Meas. Sci. Technol. 23 074001 Google Scholar
[4]	Bobroff N 1993 Meas. Sci. Technol. 4 907 Google Scholar
[5]	Lay O P, Dubovitsky S, Peters R D, Burger J P, Steier W H 2003 Opt. Lett. 28 890 Google Scholar
[6]	Bourdet G L, Orszag A G 1979 Appl. Opt. 18 225 Google Scholar
[7]	Zimmermann E, Salvadé Y, Dändliker R 1996 Opt. Lett. 21 531 Google Scholar
[8]	Meiners-Hagen K, Schoedel R, Pollinger F, Abou-Zeid A 2009 Meas. Sci. Rev. 9 16 Google Scholar
[9]	Dändliker R, Thalmann R, Prongue D 1988 Opt. Lett. 13 339 Google Scholar
[10]	Groot P 2001 Opt. Eng. 40 28 Google Scholar
[11]	时光, 张福民, 曲兴华, 孟祥松 2014 63 184209 Google Scholar Shi G, Zhang F M, Qu X H, Meng X S 2014 Acta Phys. Sin. 63 184209 Google Scholar
[12]	Coe P A, Howell D F, Nickerson R B 2004 Meas. Sci. Technol. 15 2175 Google Scholar
[13]	Williams C C, Wickramasinghe H K 1989 Opt. Lett. 145 42 Google Scholar
[14]	Diddams S A, Jones D J, Ye J, Cundiff S T, Hall J L, Ranka J K, Windeler R S, Holzwarth R, Udem T, Hansch T W 2000 Phys. Rev. Lett. 84 5102 Google Scholar
[15]	Udem T, Holzwarth R, Hansch T W 2002 Nature 416 233 Google Scholar
[16]	Jones D J, Diddams S A, Ranka J K, Stentz A, Windeler R W, Hall J L, Cundiff S T 2000 Science 288 635 Google Scholar
[17]	Minoshima K, Matsumoto H 2000 Appl. Opt. 39 5512 Google Scholar
[18]	王国超, 颜树华, 杨俊, 林存宝, 杨东兴, 邹鹏飞 2013 62 070601 Google Scholar Wang G C, Yan S H, Yang J, Lin C B, Yang D X, Zou P F 2013 Acta Phys. Sin. 62 070601 Google Scholar
[19]	张晓声, 易旺民, 胡明皓, 杨再华, 吴冠豪 2016 65 080602 Google Scholar Zhang X S, Yi W M, Hu M H, Yang Z H, Wu G H 2016 Acta Phys. Sin. 65 080602 Google Scholar
[20]	Lee J, Kim Y J, Lee K, Lee S, Kim S W 2010 Nat. Photonics 4 716 Google Scholar
[21]	秦鹏, 陈伟, 宋有建, 胡明列, 柴路, 王清月 2012 61 240601 Google Scholar Qin P, Chen W, Song Y J, Hu M L, Chai L, Wang C Y 2012 Acta Phys. Sin. 61 240601 Google Scholar
[22]	Coddington I, Swann W C, Nenadovic L, Newbury N R 2009 Nat. Photonics 3 351 Google Scholar
[23]	Lee J, Han S, Lee K, Bae E, Kim S, Lee S, Kim S W, Kim Y J 2013 Meas. Sci. Technol. 24 045201 Google Scholar
[24]	Joo K N, Kim S W 2006 Opt. Express 14 5954 Google Scholar
[25]	Van den Berg S A, Persijn S T, Kok G, Zeitouny M G, Bhattacharya N 2012 Phys. Rev. Lett. 108 183901 Google Scholar
[26]	Wang G C, Jang Y S, Hyun S, Chun B J, Kang H J, Yan S H, Kim S W, Kim Y J 2015 Opt. Express 23 9121 Google Scholar
[27]	Hyun S, Kim Y J, Kim Y, Jin J, Kim S W 2009 Meas. Sci. Technol. 20 095302 Google Scholar
[28]	Jang Y S, Wang G C, Hyun S, Kang H J, Chun B J, Kim Y J, Kim S W 2016 Sci. Rep. 6 31770 Google Scholar
[29]	Ye J 2004 Opt. Lett. 29 1153 Google Scholar
[30]	Wei D, Takahashi S, Takamasu K, Matsumoto H 2009 Opt. Lett. 34 2775 Google Scholar
[31]	孟祥松, 张福民, 曲兴华 2015 23 230601 Google Scholar Meng X S, Zhang F M, Qu X H 2015 Acta Phys. Sin. 23 230601 Google Scholar
[32]	Kim S W 2009 Nat. Photonics 3 313 Google Scholar
[33]	姜海峰 2018 67 160602 Google Scholar Jiang H F 2018 Acta Phys. Sin. 67 160602 Google Scholar
[34]	Chun B J, Hyun S, Kim S, Kim S W, Kim Y J 2013 Opt. Express 21 29179 Google Scholar
[35]	Felder R 2003 Metrologia 42 323 Google Scholar
[36]	Wei D, Takamasu K, Matsumoto H 2013 Precis. Eng. 37 694 Google Scholar
[37]	Tilford C R 1977 Appl. Opt. 16 1857 Google Scholar
[38]	王国超, 魏春华, 颜树华 2014 光学学报 34 111 Google Scholar Wang G C, Wei C H, Yan S H 2014 Acta Optic. Sin. 34 111 Google Scholar
[39]	Falaggis K, Towers D P, Towers C E 2013 Appl. Opt. 52 5758 Google Scholar
[40]	Towers C E, Towers D P, Julian D C 2004 Opt. Express 12 1136 Google Scholar
[41]	Ma L, Zucco M, Picard S 2003 IEEE J. Sel. Top. Quantum Electron. 9 1066 Google Scholar
[42]	王国超, 谭立龙, 颜树华, 魏春华 2017 光学学报 37 160 Google Scholar Wang G C, Wei C H, Yan S H 2017 Acta Optic. Sin. 37 160 Google Scholar
[43]	Hyun S, Kim Y J, Kim Y, Kim S W 2010 CIRP Ann.-Manuf. Techn. 59 555 Google Scholar
[44]	王国超 2015 博士学位论文 (长沙: 国防科技大学) Wang G C 2015 Ph. D. Dissertation (Changsha: National University of Defense Technology) (in Chinese)
[45]	Ciddor P E 1996 Appl. Opt. 35 1566 Google Scholar
[46]	Birch K P, Downs M J 1993 Metrologia 30 155 Google Scholar
[47]	Wu G H, Takahashi M, Arai K, Inaba H, Minoshima K 2013 Sci. Rep. 3 1894 Google Scholar
[48]	Minoshima K, Arai K, Inaba H 2011 Opt. Express 19 26095 Google Scholar

[1]	周强, 吴腾飞, 曾周末, 邾继贵. 基于双向吸收光谱精准标定的光频扫描干涉绝对测距. , 2024, 73(17): 170601. doi: 10.7498/aps.73.20240840
[2]	梁旭, 林嘉睿, 吴腾飞, 赵晖, 邾继贵. 重复频率倍增光频梳时域互相关绝对测距. , 2022, 71(9): 090602. doi: 10.7498/aps.71.20212073
[3]	饶冰洁, 张攀, 李铭坤, 杨西光, 闫露露, 陈鑫, 张首刚, 张颜艳, 姜海峰. 用于光腔衰荡光谱测量的多支路掺铒光纤飞秒光梳系统. , 2022, 71(8): 084203. doi: 10.7498/aps.71.20212162
[4]	赵显宇, 曲兴华, 陈嘉伟, 郑继辉, 王金栋, 张福民. 一种基于电光调制光频梳光谱干涉的绝对测距方法. , 2020, 69(9): 090601. doi: 10.7498/aps.69.20200081
[5]	陈嘉伟, 王金栋, 曲兴华, 张福民. 光频梳频域干涉测距主要参数分析及一种改进的数据处理方法. , 2019, 68(19): 190602. doi: 10.7498/aps.68.20190836
[6]	曹辉, 宋有建, 于佳禾, 师浩森, 胡明列, 王清月. 奇异谱分析用于提升双光梳激光测距精度. , 2018, 67(1): 010601. doi: 10.7498/aps.67.20171922
[7]	吕志国, 杨直, 李峰, 李强龙, 王屹山, 杨小君. 基于光纤中超短脉冲非线性传输机理与特定光谱选择技术的多波长飞秒激光的产生. , 2018, 67(18): 184205. doi: 10.7498/aps.67.20181026
[8]	彭博, 曲兴华, 张福民, 张天宇, 张铁犁, 刘晓旭, 谢阳. 飞秒脉冲非对称互相关绝对测距. , 2018, 67(21): 210601. doi: 10.7498/aps.67.20181274
[9]	姜海峰. 超稳光生微波源研究进展. , 2018, 67(16): 160602. doi: 10.7498/aps.67.20180751
[10]	廖磊, 易旺民, 杨再华, 吴冠豪. 基于合成波长法的飞秒激光外差干涉测距方法. , 2016, 65(14): 140601. doi: 10.7498/aps.65.140601
[11]	刘欢, 曹士英, 孟飞, 林百科, 方占军. 覆盖可见光波长的掺Er光纤飞秒光学频率梳. , 2015, 64(9): 094204. doi: 10.7498/aps.64.094204
[12]	赵冠凯, 刘军, 李儒新. 基于多光子脉冲内干涉相位扫描法对飞秒激光脉冲进行相位测量和补偿的研究. , 2014, 63(16): 164207. doi: 10.7498/aps.63.164207
[13]	吴翰钟, 曹士英, 张福民, 邢书剑, 曲兴华. 一种光学频率梳绝对测距的新方法. , 2014, 63(10): 100601. doi: 10.7498/aps.63.100601
[14]	张晓青, 贺号, 胡明列, 颜鑫, 张霞, 任晓敏, 王清月. 多波长飞秒激光激发下GaAs纳米线SHG特性研究. , 2013, 62(7): 076102. doi: 10.7498/aps.62.076102
[15]	邢书剑, 张福民, 曹士英, 王高文, 曲兴华. 飞秒光频梳的任意长绝对测距. , 2013, 62(17): 170603. doi: 10.7498/aps.62.170603
[16]	王国超, 颜树华, 杨俊, 林存宝, 杨东兴, 邹鹏飞. 一种双光梳多外差大尺寸高精度绝对测距新方法的理论分析. , 2013, 62(7): 070601. doi: 10.7498/aps.62.070601
[17]	吴学健, 尉昊赟, 朱敏昊, 张继涛, 李岩. 基于飞秒光频梳的双频He-Ne激光器频率测量. , 2012, 61(18): 180601. doi: 10.7498/aps.61.180601
[18]	孟飞, 曹士英, 蔡岳, 王贵重, 曹建平, 李天初, 方占军. 光纤飞秒光学频率梳的研制及绝对光学频率测量. , 2011, 60(10): 100601. doi: 10.7498/aps.60.100601
[19]	刘华刚, 胡明列, 刘博文, 宋有建, 柴路, 王清月. 高功率高重复频率多波长飞秒激光系统的研究. , 2010, 59(6): 3979-3985. doi: 10.7498/aps.59.3979
[20]	方占军, 王强, 王民明, 孟飞, 林百科, 李天初. 飞秒光梳和碘稳频532nm Nd:YAG激光频率的测量. , 2007, 56(10): 5684-5690. doi: 10.7498/aps.56.5684

计量

文章访问数: 6862
PDF下载量: 154
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

基于飞秒光梳多路同步锁相的多波长干涉实时绝对测距及其非模糊度量程分析