-
The reflection and transmission of plane electromagnetic waves on monolayer graphene are studied theoretically in this paper. From an electromagnetic point of view, monolayer graphene is described as an “infinitely thin” graphene sheet characterized by a surface conductivity, and based on a microscopic quantum dynamical approach, the graphene sheet becomes anisotropic in the presence of both an electrostatic and a magnetic bias. In this work, starting from boundary conditions and phase-matching conditions, the propagation matrix for the analysis of the interaction between an electromagnetic field and thin graphene sheet which is biased electrostatically and magnetostatically, and then characterized by an anisotropic conductivity, is derived. Furthermore, the analytical solutions of co- and cross-polarization reflective and transmittance coefficients through an anisotropic graphene planar surface are obtained from the proposal matrix above, which couples the fundamental transverse electric (TE) polarization and transverse magnetic (TM) polarization and includes the possible effects of electrostatic and/or magnetostatic bias. In conclusion, the cross-polarization reflective coefficient of TE wave and that of TM wave are equal, and their cross-polarization transmittance coefficients have opposite phase. Finally, a new propagation matrix for stratified medium containing anisotropic graphene interfaces is deduced by embedding the matrix across graphene sheet mentioned above into the traditional propagation matrix for isotropic stratified medium. The proposed new matrix can be used to investigate the propagation properties of plane wave in a complex structure of layered medium and anisotropic conductivity interfaces (including graphene sheet) analytically and quickly, and represents a very simple tool for the relevant analysis and design.
-
Keywords:
- anisotropic graphene /
- electrostatically and magnetostatically bias /
- stratified media /
- propagation matrix
[1] Lovat G 2012 IEEE Trans. Electromagn. Compat. 54 101
 Google Scholar
Google Scholar
[2] Geim A K 2009 Science 324 1530
 Google Scholar
Google Scholar
[3] Das T, Sharma B K, Katiyar A K, Ahn J H 2018 J. Semicond. 39 011007
 Google Scholar
Google Scholar
[4] Nimbalkar A, Kim H 2020 Nano-Micro Lett. 12 126
 Google Scholar
Google Scholar
[5] Moshizi S A, Azadi S, Belford A, Razmjou A, Wu S, Han Z J, Asadnia M 2020 Nano-Micro Lett. 12 109
 Google Scholar
Google Scholar
[6] Chen X Y, Tian Z, Li Q, Li S X, Zhang X Q, Ouyang C M, Gu J Q, Han J G, Zhang W L 2020 Chin. Phys. B 29 077803
 Google Scholar
Google Scholar
[7] Chaea M S, Leea T H, Sona K R, Parka T H, Hwangb K S, Kim T G 2020 J Mater. Sci. Technol. 40 72
 Google Scholar
Google Scholar
[8] Novoselov K S, Geim A K, Morozov S V, Jiang D, Zhang Y, Dubonos S V, Grigorieva I V, Firsove A A 2004 Science 306 666
 Google Scholar
Google Scholar
[9] Novoselov K S, Geim A K, Morozov S V, Jiang D, Katsnelson M I, Grigorieva I V, Dubonos S V, Firsove A A 2005 Nature 438 197
 Google Scholar
Google Scholar
[10] Zhang Y, Tan Y W, Stormer H L, Kim P 2005 Nature 438 201
 Google Scholar
Google Scholar
[11] Zhang Y, Small J P, Pontius W V, Kim P 2005 Appl. Phys. Lett. 86 073104
 Google Scholar
Google Scholar
[12] Slepyan G Y, Maksimenko S A, Lakhtakia A, Yevtushenko O, Gusakov A V 1999 Phys. Rev. B 60 17136
 Google Scholar
Google Scholar
[13] Hanson G W 2008 J. Appl. Phys. 103 064302
 Google Scholar
Google Scholar
[14] Gusynin V P, Sharapov S G, Carbotte J P 2007 J. Phys. Condens. Matter 19 026222
 Google Scholar
Google Scholar
[15] Hanson G W 2008 IEEE Trans. Antennas Propag. 56 747
 Google Scholar
Google Scholar
[16] 葛德彪, 魏兵 2011 电磁波理论 (北京: 科学出版社) 第5, 6, 39, 40, 56−73页
Ge D B, Wei B 2011 Electromagnetic wave theory (Beijing: Science Press) pp5, 6, 39, 40, 56−73 (in Chinese)
[17] 王飞, 魏兵 2019 68 244101
 Google Scholar
Google Scholar
Wang F, Wei B 2019 Acta Phys. Sin. 68 244101
 Google Scholar
Google Scholar
[18] Gusynin V P, Sharapov S G 2006 Phys. Rev. B 73 245411
 Google Scholar
Google Scholar
[19] Gusynin V P, Sharapov S G, Carbotte J P 2006 Phys. Rev. Lett. 96 256802
 Google Scholar
Google Scholar
[20] Peres N M R, Guiner F, Castro Neto A H 2006 Phys. Rev. B 73 125411
 Google Scholar
Google Scholar
[21] Ziegler K 2007 Phys. Rev. B 75 233407
 Google Scholar
Google Scholar
[22] George W H 2008 Journal of Applied Physics 103 064302
[23] Balanis C A 1989 Advanced Engineering Electromagnetics (New York: Wiley) pp168−170
-
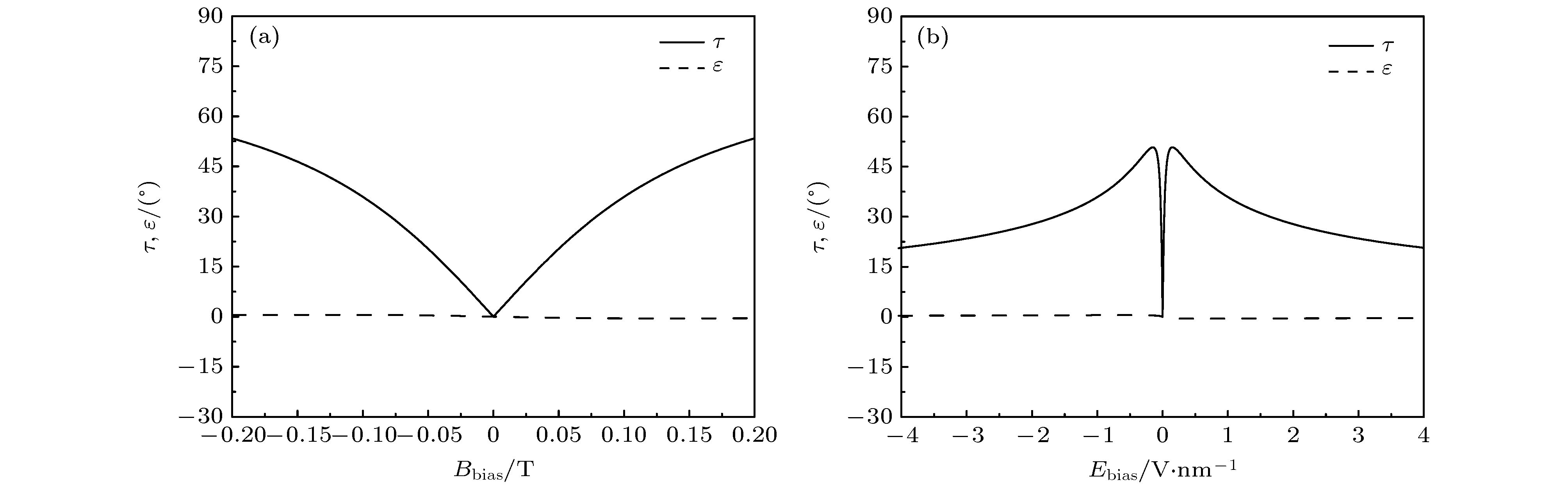
图 5 透射波极化状态参量
$\tau, \varepsilon $ 与偏置磁场、偏置电场关系 (a) 偏置电场${E_{{\rm{bias}}}} = 1\;{\rm{V}} \cdot {\rm{n}}{{\rm{m}}^{{{ - }}1}}$ ; (b) 偏置磁场${B_{{\rm{bias}}}} = 0.5\;{\rm{T}}$ Fig. 5. Angles
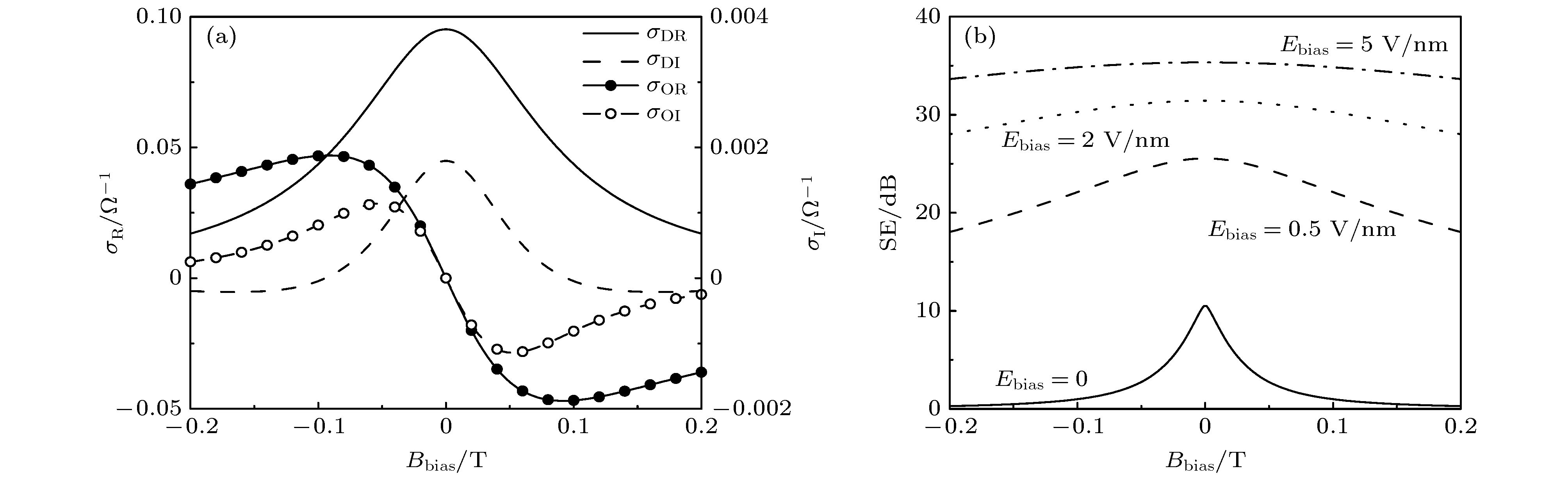
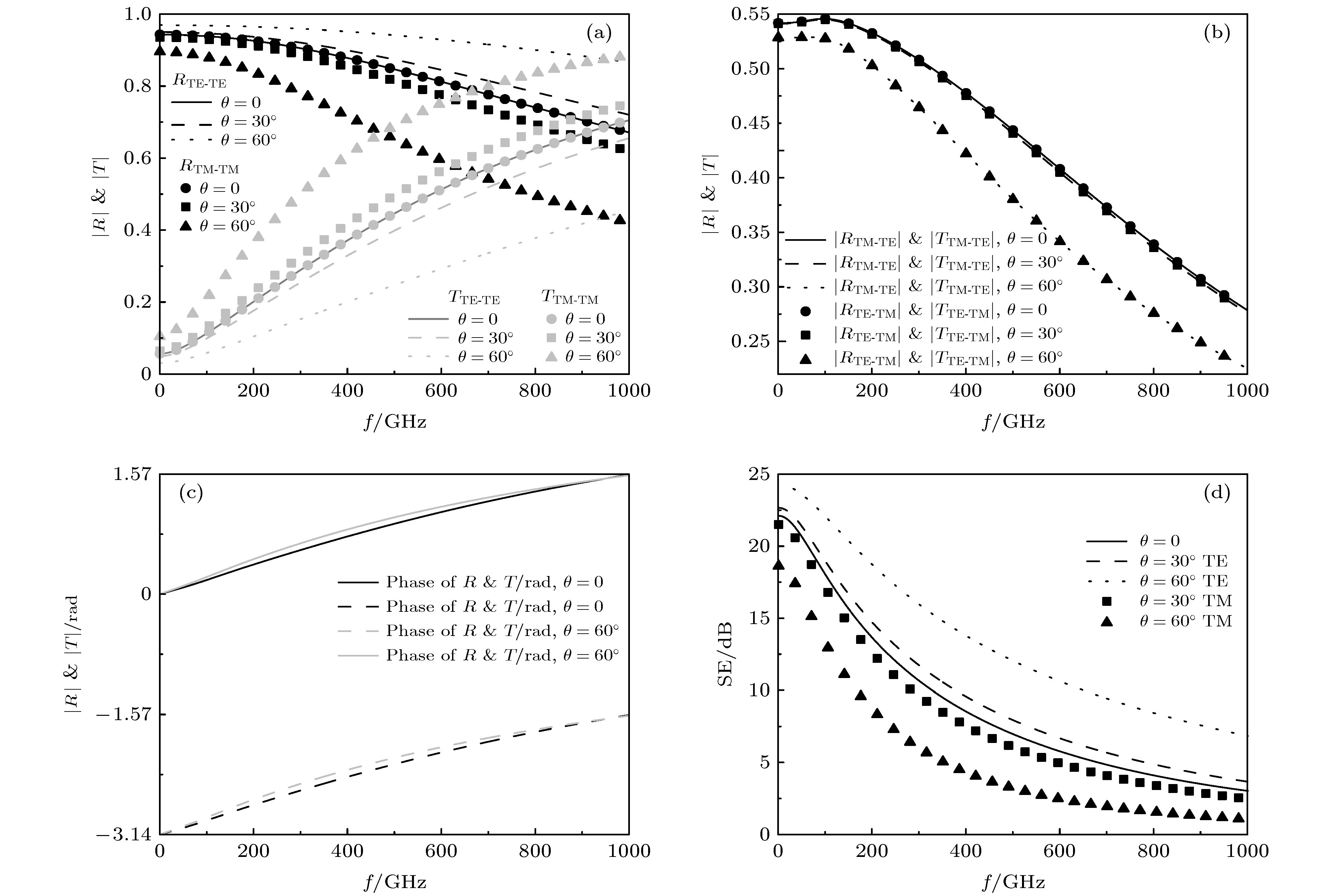
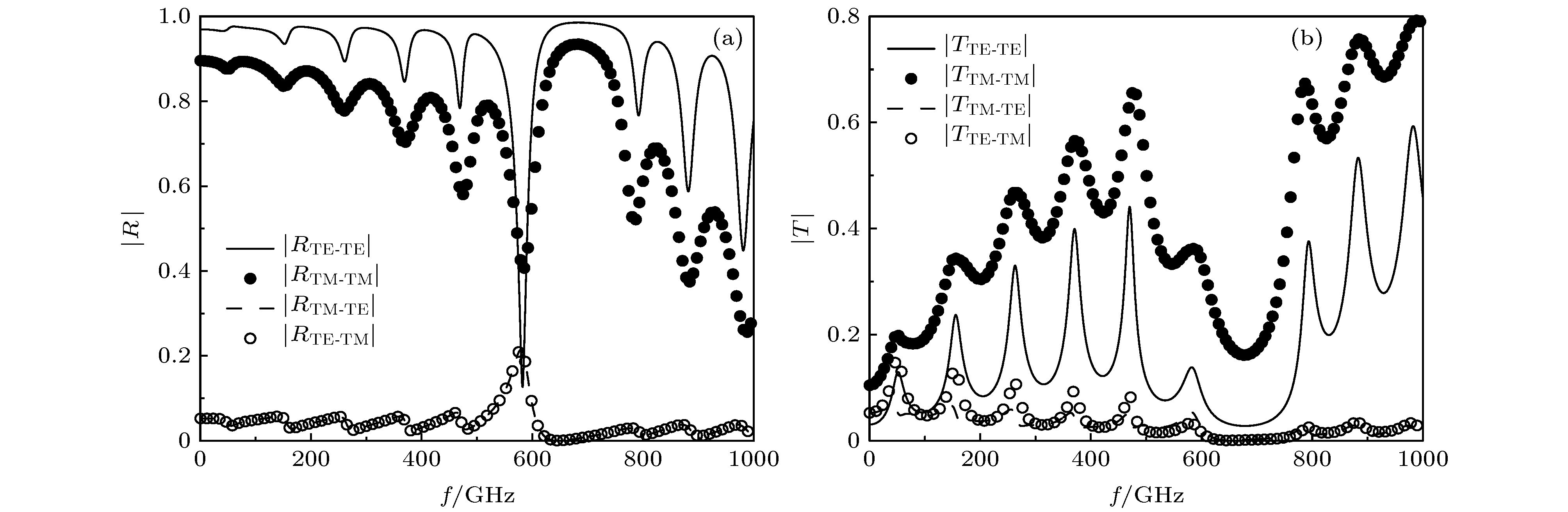
$\tau, \;\varepsilon $ describing the polarization state of the transmitted wave as functions of the magnetostatic bias and the electrostatic bias: (a)${E_{{\rm{bias}}}} = 1\;{\rm{V}} \cdot {\rm{n}}{{\rm{m}}^{{{ - }}1}}$ ; (b)${B_{{\rm{bias}}}} = 0.5\;{\rm{T}}$ .图 6 各向异性石墨烯界面的反透射及屏蔽效率随频率变化关系 (a) 同极化反透射系数模值; (b) 交叉极化反透射系数模值; (c) 交叉极化反透射系数相位; (d) 屏蔽效率
Fig. 6. Reflection/transmission and the SE of an isotropic graphene sheet as functions of frequency: (a) Modulus of co-polarization reflective and transmittance coefficients; (b) modulus of cross-polarization reflective and transmittance coefficients; (c) phase of cross-polarization reflective and transmittance coefficients; (d) SE.
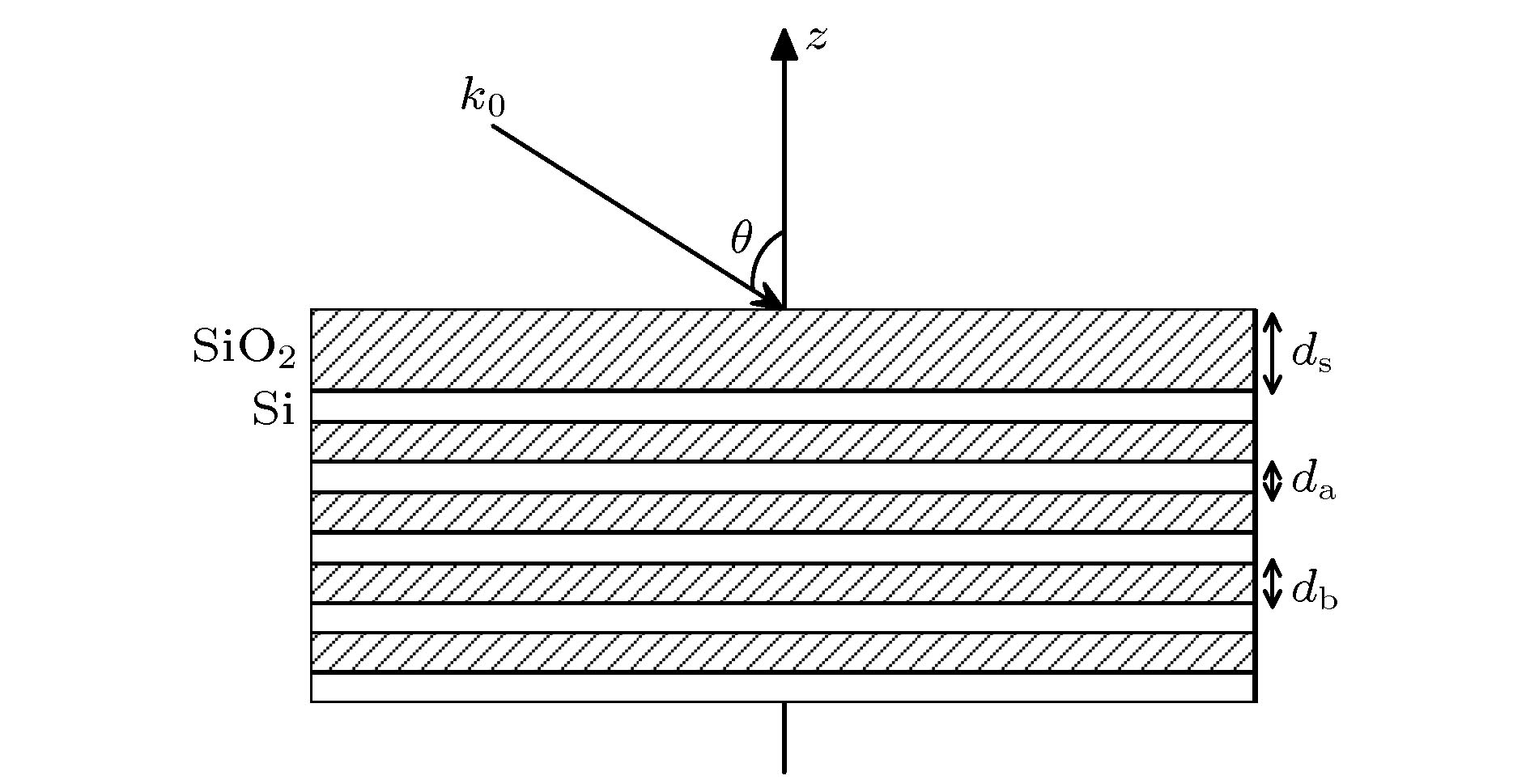
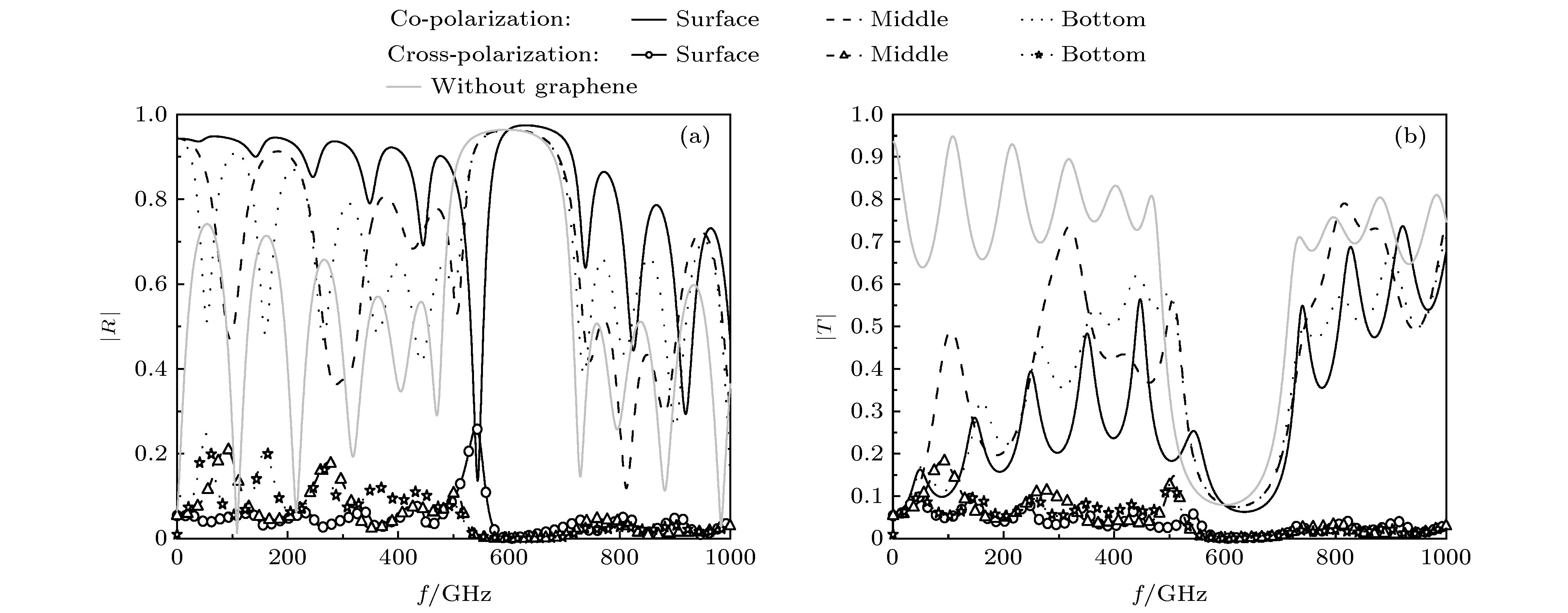
图 8 各向异性石墨烯界面位于不同位置时层状结构对垂直入射波的反透射随频率变化关系 (a) 反射系数模值; (b) 透射系数模值
Fig. 8. Reflection/transmission of normally incident waves from a periodic layered structure with an isotropic graphene at different interface as functions of frequency: (a) Modulus of reflective coefficient; (b) modulus of transmittance coefficient.
-
[1] Lovat G 2012 IEEE Trans. Electromagn. Compat. 54 101
 Google Scholar
Google Scholar
[2] Geim A K 2009 Science 324 1530
 Google Scholar
Google Scholar
[3] Das T, Sharma B K, Katiyar A K, Ahn J H 2018 J. Semicond. 39 011007
 Google Scholar
Google Scholar
[4] Nimbalkar A, Kim H 2020 Nano-Micro Lett. 12 126
 Google Scholar
Google Scholar
[5] Moshizi S A, Azadi S, Belford A, Razmjou A, Wu S, Han Z J, Asadnia M 2020 Nano-Micro Lett. 12 109
 Google Scholar
Google Scholar
[6] Chen X Y, Tian Z, Li Q, Li S X, Zhang X Q, Ouyang C M, Gu J Q, Han J G, Zhang W L 2020 Chin. Phys. B 29 077803
 Google Scholar
Google Scholar
[7] Chaea M S, Leea T H, Sona K R, Parka T H, Hwangb K S, Kim T G 2020 J Mater. Sci. Technol. 40 72
 Google Scholar
Google Scholar
[8] Novoselov K S, Geim A K, Morozov S V, Jiang D, Zhang Y, Dubonos S V, Grigorieva I V, Firsove A A 2004 Science 306 666
 Google Scholar
Google Scholar
[9] Novoselov K S, Geim A K, Morozov S V, Jiang D, Katsnelson M I, Grigorieva I V, Dubonos S V, Firsove A A 2005 Nature 438 197
 Google Scholar
Google Scholar
[10] Zhang Y, Tan Y W, Stormer H L, Kim P 2005 Nature 438 201
 Google Scholar
Google Scholar
[11] Zhang Y, Small J P, Pontius W V, Kim P 2005 Appl. Phys. Lett. 86 073104
 Google Scholar
Google Scholar
[12] Slepyan G Y, Maksimenko S A, Lakhtakia A, Yevtushenko O, Gusakov A V 1999 Phys. Rev. B 60 17136
 Google Scholar
Google Scholar
[13] Hanson G W 2008 J. Appl. Phys. 103 064302
 Google Scholar
Google Scholar
[14] Gusynin V P, Sharapov S G, Carbotte J P 2007 J. Phys. Condens. Matter 19 026222
 Google Scholar
Google Scholar
[15] Hanson G W 2008 IEEE Trans. Antennas Propag. 56 747
 Google Scholar
Google Scholar
[16] 葛德彪, 魏兵 2011 电磁波理论 (北京: 科学出版社) 第5, 6, 39, 40, 56−73页
Ge D B, Wei B 2011 Electromagnetic wave theory (Beijing: Science Press) pp5, 6, 39, 40, 56−73 (in Chinese)
[17] 王飞, 魏兵 2019 68 244101
 Google Scholar
Google Scholar
Wang F, Wei B 2019 Acta Phys. Sin. 68 244101
 Google Scholar
Google Scholar
[18] Gusynin V P, Sharapov S G 2006 Phys. Rev. B 73 245411
 Google Scholar
Google Scholar
[19] Gusynin V P, Sharapov S G, Carbotte J P 2006 Phys. Rev. Lett. 96 256802
 Google Scholar
Google Scholar
[20] Peres N M R, Guiner F, Castro Neto A H 2006 Phys. Rev. B 73 125411
 Google Scholar
Google Scholar
[21] Ziegler K 2007 Phys. Rev. B 75 233407
 Google Scholar
Google Scholar
[22] George W H 2008 Journal of Applied Physics 103 064302
[23] Balanis C A 1989 Advanced Engineering Electromagnetics (New York: Wiley) pp168−170
计量
- 文章访问数: 6719
- PDF下载量: 89
- 被引次数: 0














 下载:
下载: