-
在香蕉形液晶分子B4相态中, 非手性香蕉形液晶分子自组装形成层状结构, 分子在层内倾斜, 形成层手性和自发极化, 并且造成层内不匹配, 最终形成纳米螺旋丝. 本文设计了NOBOW/十六烷混合体系, 在高温时, 香蕉形液晶分子溶解于十六烷, 在低温时, 香蕉形液晶分子自组装形成纳米螺旋丝, 并最终形成三维网络, 变成有机凝胶. 为深入理解纳米螺旋丝有机凝胶的特性, 拓展其在软物质领域的应用, 本文通过流变实验对该有机凝胶的黏弹性质进行了系统研究. 实验表明纳米螺旋丝有机凝胶与传统凝胶不同, 纳米螺旋丝有机凝胶可以随温度变化形成凝胶-流体的可逆变化, 并且通过测量NOBOW/十六烷混合体系在不同液晶分子浓度、温度、应变大小和应变速率下的流变特征, 揭示了该有机凝胶的流变特性与纳米螺旋丝的性质密切相关.In the B4 phase of bent-core liquid crystals, smectic layers of tilted achiral bent-core molecules are chiral and polar, which, driven by intra-layer structural mismatch, eventually twist into helical nanofilaments. We design a NOBOW/hexadecane organogel system, which is different from traditional organogel system, and the studied organogels show reversible gel-liquid transitions under temperature cycles. At high temperature, the NOBOW molecules dissolve in hexadecane and the storage modulus and viscous modulus show typical liquid characteristics. At low temperature, the mobility of NOBOW molecules decreases and the storage modulus of the organogels increases as the temperature decreases. We conduct a rheology experiment to systematically investigate the viscoelasticity of the organogel to understand the property of the organogel and develop the application in soft matter. The viscoelastic studies of the organogels reveal that the helical nanofilaments are internally strained and their 3D networks are relatively stiff, which provides an in-depth insight into the properties of the organogels and paves the way for their applications in soft matter.
-
Keywords:
- bent-core liquid crystal /
- helical nanofilament /
- organogel /
- viscoelastic property
[1] 乔小溪, 张向军, 田煜, 孟永钢, 温诗铸 2013 62 176101
Google Scholar
Qiao X X, Zhang X J, Tian Y, Meng Y G, Wen S Z 2013 Acta Phys. Sin. 62 176101
Google Scholar
[2] Shen Z X, Tang M J, Chen P, Zhou S H, Ge S J, Duan W, Wei T, Liang X, Hu W, Lu Y Q 2020 Adv. Opt. Mater. 8 1902124
Google Scholar
[3] Qin J, Wang X Q, Yuan C, Zheng Z, Shen D 2019 Liq. Cryst. 47 255
Google Scholar
[4] Liu L, Wang M, Guo L X, Sun Y, Zhang X Q, Lin B P, Yang H 2018 Macromolecules 51 4516
Google Scholar
[5] Wang L, Chen D, Gutierrez Cuevas K G, Bisoyi H K, Fan J, Zola R S, Li G, Urbas A M, Bunning T J, Weitz D A 2017 Mater. Horiz. 4 1190
Google Scholar
[6] Ye F F, Mukhopadhyay R, Stenull O, Lubensky T C 2007 Phys. Rev. Lett. 98 147801.1
Google Scholar
[7] Ye F F, Lubensky T C 2009 JPCB 113 3853
Google Scholar
[8] Hough L E, Jung H T, Kruerke D, Heberling M S, Nakata M, Jones C D, Chen D, Link D R, Zasadzinski J, Heppke G 2009 Science 325 456
Google Scholar
[9] Dierking I 2010 Angew. Chem. Int. Ed. 49 29
Google Scholar
[10] Sekine T, Niori T, Sone M, Watanabe J, Takezoe H 1997 Jpn. J. Appl. Phys. 36 6455
Google Scholar
[11] Chen D, Madennan J E, Shao R, Dong K Y, Wang H, Korblova E, Walba D M, Glaser M A, Clark N A 2011 J. Am. Chem. Soc. 133 12656
Google Scholar
[12] Coleman D A, Fernsler J, Chattham N, Nakata M, Takanishi Y, Körblova E, Link D R, Shao R F, Jang W G, Maclennan J E 2003 Science 301 1204
Google Scholar
[13] Thisayukta J, Takezoe H, Watanabe J 2001 Jpn. J. Appl. Phys. 40 3277
Google Scholar
[14] Tschierske C, Dantlgraber G 2003 Pramana 61 455
Google Scholar
[15] Kondepudi D K, Kaufman R J, Singh N 1990 Science 250 975
Google Scholar
[16] Zep A, Salamonczyk M, Vaupotič N, Pociecha D, Gorecka E 2013 Chem. Commun. 49 3119
Google Scholar
[17] Chen D, Zhu C, Wang H, Maclennan J E, Glaser M A, Korblova E, Walba D M, Rego J A, Soto-Bustamante E A, Clark N A 2013 Soft Matter 9 462
Google Scholar
[18] Gleeson H F, Liu H, Kaur S, Srigengan S, Görtz V, Mandle R, Lydon J E 2018 Soft Matter 14 9159
Google Scholar
[19] Matraszek J, Topnani N, Vaupotic N, Takezoe H, Mieczkowski J, Pociecha D, Gorecka E 2015 Angew Chem. Int. Ed. Engl. 128 3529
[20] Shen Z, Jiang Y, Wang T, Liu M 2015 J. Am. Chem. Soc. 137 16109
Google Scholar
[21] Shen Z, Wang T, Shi L, Tang Z, Liu M 2015 Chem. Sci. 6 4267
Google Scholar
[22] Zheludev N I 2010 Science 328 582
Google Scholar
[23] Chen D, Tuchband M R, Horanyi B, Korblova E, Walba D M, Glaser M A, Maclennan J E, Clark N A 2015 Nat. Commun. 6 1
[24] 刘立伟, 王作维, 周鲁卫, 王冶金, 高广君, 刘晓君 2000 49 1887
Google Scholar
Liu L W, Wang Z W, Zhou L W, Wang Z J, Gao G J, Liu X J 2000 Acta Phys. Sin. 49 1887
Google Scholar
[25] Macosko C W 1994 RHEOLOGY Principles, Measurements and Applications (Canada: Wiley-VCH) pp65–106
[26] Mason T G, Weitz D A 1995 Phys. Rev. Lett. 74 1250
Google Scholar
[27] Chen D, Zhu C, Shoemaker R K, Korblova E, Walba D M, Glaser M A, Maclennan J E, Clark N A 2010 Langmuir 26 15541
Google Scholar
-
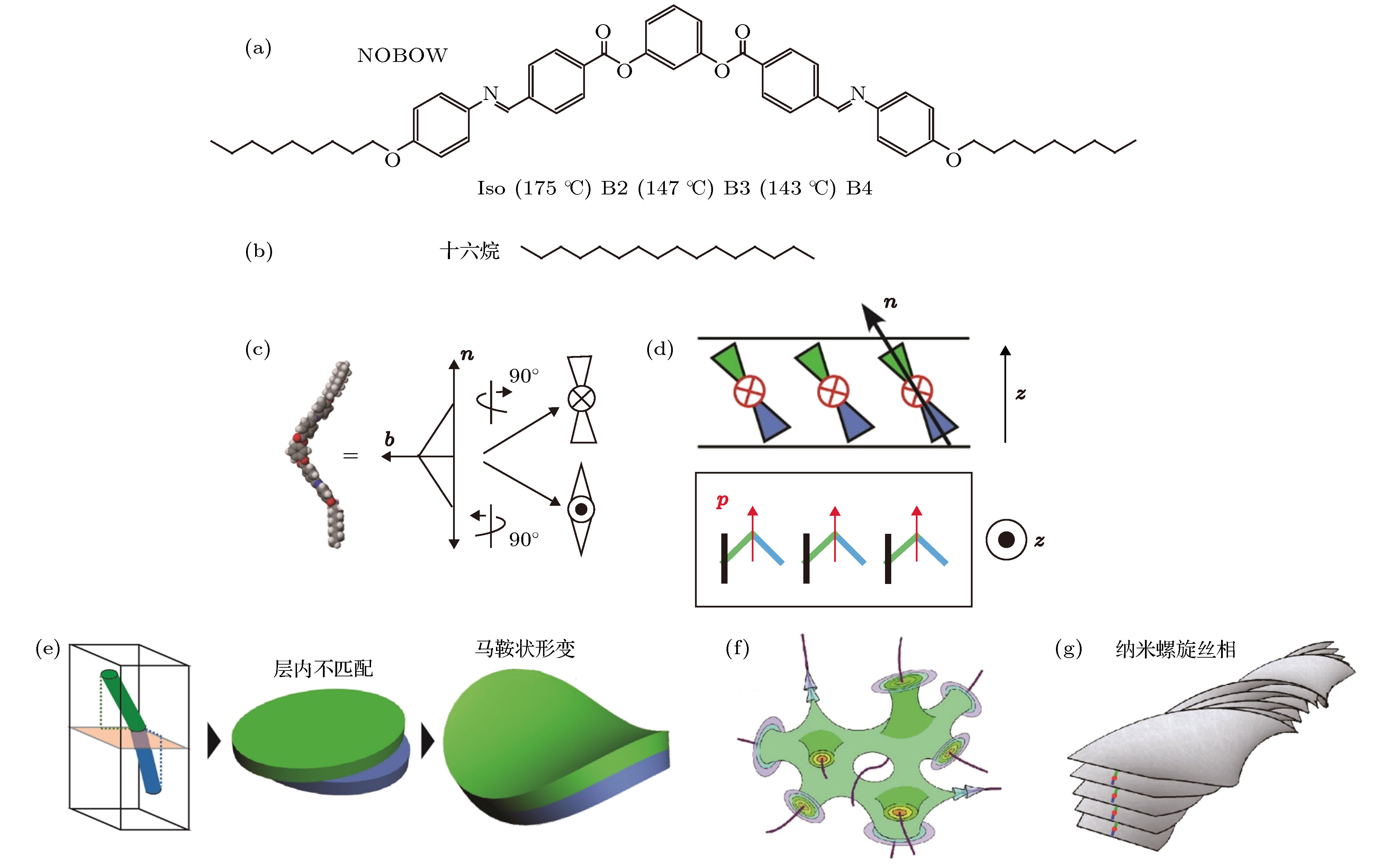
图 1 香蕉形液晶分子的自组装结构 (a) NOBOW和 (b)十六烷的化学结构; (c) 香蕉形液晶分子的指向矢n沿长轴方向, 极化p沿弓形方向; (d) 非手性香蕉形液晶分子自组装形成层状结构, 分子在层内倾斜, 形成层手性和极化, 极化方向p同时垂直于指向矢n和层法线z, 即垂直于指向矢n和层法线z所在的平面; (e) 香蕉形液晶分子上下两臂的倾斜方向相互垂直, 对应的扩张方向也相互垂直, 造成层内不匹配. 为了消除这种层内不匹配, 香蕉形液晶分子的层状结构会自发形成马鞍状弹性形变. 当马鞍状弹性形变足够大时, 液晶分子可以自组装形成 (f)双连续相或 (g)纳米螺旋丝相
Fig. 1. Self-assembly of bent-core liquid crystal. Chemical structures of (a) NOBOW and (b) hexadecane molecules; (c) the director, n, which is along the molecular long axis, and the polarization, p, which is along the bow direction; (d) smectic layers of tilted bent-core molecules. The molecules are tilted from the layer normal z, and the macroscopic polarization p, is orthogonal to both n and z; (e) the tilt directions of the top and bottom molecular arms of bent-core liquid crystals are essentially orthogonal to each other. If each molecular arm is regarded as an elastic slab, the two elastic slabs dilate along their local molecular tilt directions, which are orthogonal to each other, thus resulting in internal structural mismatch. The internal structural mismatch could be released by the formation of saddle-splay deformations; (f) bicontinuous smectic layer of the dark conglomerate (DC) phase, in which smectic layers organize into disordered focal conics; (g) helical nanofilaments of the B4 phase, in which smectic layers of finite width form uniformly twisted ribbons.
图 2 基于香蕉形液晶分子自组装形成纳米螺旋丝网络的有机凝胶 (a)在120 ℃高温下, 1 wt.% NOBOW香蕉形液晶分子可以溶解于十六烷, 体系呈透明流体; 在室温25 ℃下, 香蕉形液晶分子自组装形成纳米螺旋丝网络, 体系变成有机凝胶. 该流体-凝胶转变可通过加热和冷却实现可逆变化; (b) 有机凝胶中纳米螺旋丝网络的速冻断层电子显微镜(FFTEM)图像; (c) 纳米螺旋丝的透射电子显微镜(TEM)图像, 插图展示了纳米螺旋丝的应力分布, 应力从螺旋轴中心往两边逐渐增加, 对应颜色逐渐加深
Fig. 2. Organogels formed by self-assembled 3D helical nanofilament networks of bent-core liquid crystals: (a) At 25 ℃, bent-core liquid crystal molecules self-assemble into helical nanofilament networks and the 1 wt.% NOBOW/hexadecane mixture forms organogels. At 120 ℃, NOBOW dissolves in the hexadecane solvent and the system appears as transparent fluid, showing reversible gel-sol transitions upon heating and cooling; (b) freeze-fracture transmission electron microscopy (FFTEM) image of helical nanofilament networks in the organogels; (c) transmission electron microscopy (TEM) image of individual helical nano-filaments. The inset shows the distribution of internal stress in the helical nanofilaments. The internal stress gradually increases from the helical axis towards the edges, accompanied by the color increase.
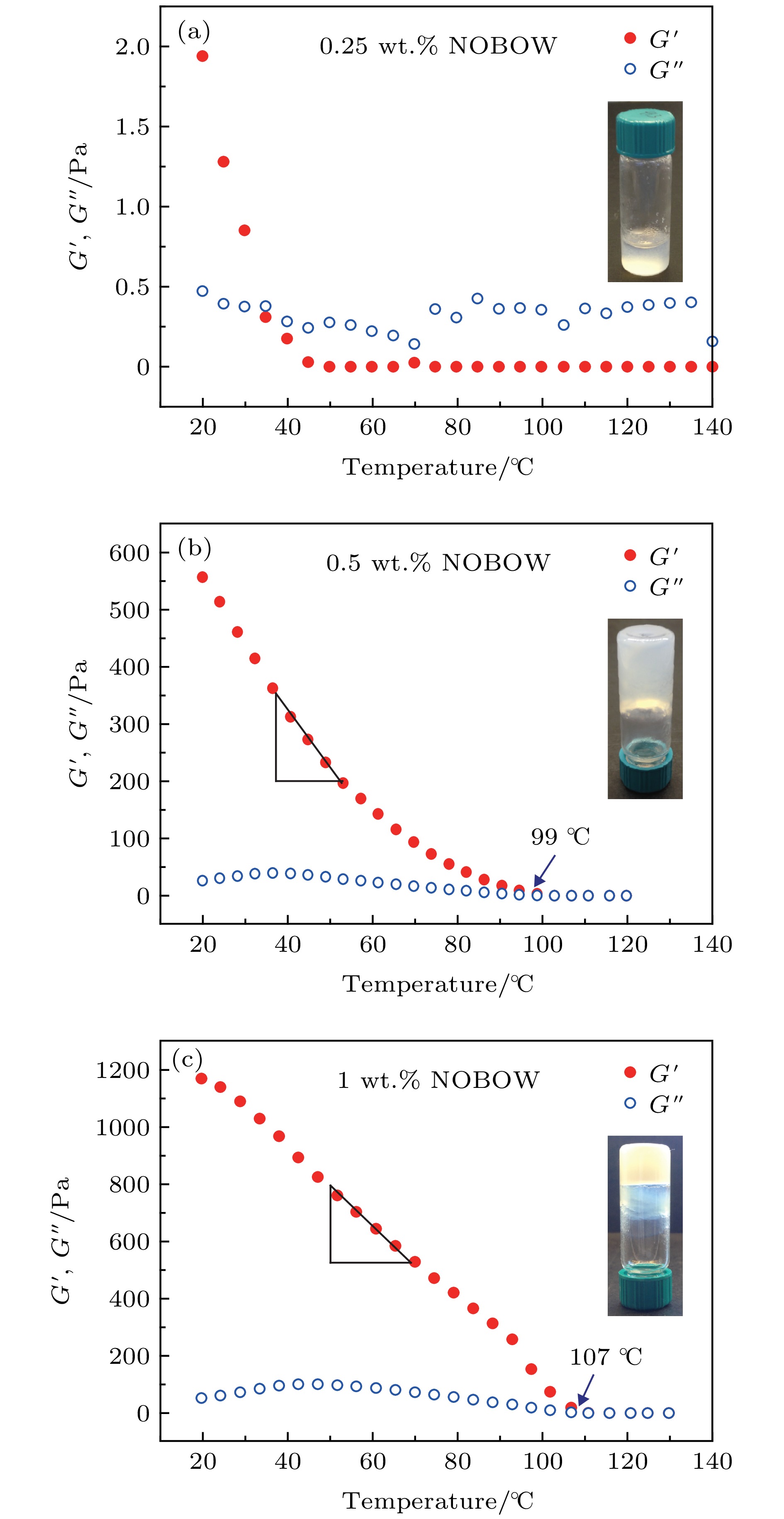
图 3 NOBOW/十六烷混合体系储能模量(G' )和损耗模量(G'' )随温度变化的关系 (a)当NOBOW的质量分数为0.25 wt.%时, 体系不能形成凝胶, 损耗模量略大于储能模量; (b), (c)当NOBOW的质量分数分别为0.5 wt.%和1 wt.%且温度分别低于99和107 ℃时, 香蕉形液晶分子自组装形成纳米螺旋丝网络, 体系储能模量显著升高. 0.5 wt.%体系储能模量增加的速率为10.3 Pa/℃, 而1 wt.%体系储能模量增加的速率为14.2 Pa/℃. 实验中, 应变保持0.5%, 应变速率保持1 Hz
Fig. 3. Temperature sweeps of the elastic (G', solid symbols) and viscous moduli (G'', open symbols) of NOBOW/ hexadecane mixtures: (a) 0.25 wt.% NOBOW/hexadecane mixture could not form organogels and its G'' is larger than G'; (b), (c) 0.5 wt.% and 1 wt.% NOBOW/hexadecane mixtures could self-assemble into helical nanofilament networks, forming organogels. The increase rate of the elastic modulus of 0.5 wt.% NOBOW/hexadecane is 10.3 Pa/℃ and that of 1 wt.% NOBOW/hexadecane is 14.2 Pa/℃. The measurements are taken at a fixed strain of 0.5% and a fixed strain rate of 1 Hz.
图 4 不同温度下, NOBOW/十六烷混合体系储能模量(G' )和损耗模量(G'' )随应变大小变化的规律 (a) 0.25 wt.%, (b) 0.5 wt.%和(c) 1 wt.% NOBOW/十六烷混合体系储能模量(G' )和损耗模量(G'' )分别在20, 60和90 ℃随应变大小变化的关系. 实验中, 应变速率保持1 Hz
Fig. 4. Strain sweeps of the elastic (G', solid symbols) and viscous moduli (G'', open symbols) of (a) 0.25 wt.%, (b) 0.5 wt.% and (c) 1 wt.% NOBOW/hexadecane mixtures measured at different temperatures of 20 ℃, 60 ℃ and 90 ℃. The mea-surements are taken at a fixed strain rate of 1 Hz.
图 5 不同温度下, NOBOW/十六烷混合体系储能模量(G' )和损耗模量(G'' )随应变速率变化的规律 (a) 0.25 wt.%, (b) 0.5 wt.%和(c) 1 wt.% NOBOW/十六烷混合体系储能模量(G' )和损耗模量(G'' )分别在20, 90, 100和120 ℃随应变速率变化的关系. 实验中, 应变大小保持0.5%
Fig. 5. Strain rate sweeps of the elastic (G', solid symbols) and viscous moduli (G'', open symbols) of (a) 0.25 wt.%, (b) 0.5 wt.% and (c) 1 wt.% NOBOW/hexadecane mixtures measured at different temperatures of 20, 90, 100 and 120 ℃. The measurements are taken at a fixed strain of 0.5%.
-
[1] 乔小溪, 张向军, 田煜, 孟永钢, 温诗铸 2013 62 176101
Google Scholar
Qiao X X, Zhang X J, Tian Y, Meng Y G, Wen S Z 2013 Acta Phys. Sin. 62 176101
Google Scholar
[2] Shen Z X, Tang M J, Chen P, Zhou S H, Ge S J, Duan W, Wei T, Liang X, Hu W, Lu Y Q 2020 Adv. Opt. Mater. 8 1902124
Google Scholar
[3] Qin J, Wang X Q, Yuan C, Zheng Z, Shen D 2019 Liq. Cryst. 47 255
Google Scholar
[4] Liu L, Wang M, Guo L X, Sun Y, Zhang X Q, Lin B P, Yang H 2018 Macromolecules 51 4516
Google Scholar
[5] Wang L, Chen D, Gutierrez Cuevas K G, Bisoyi H K, Fan J, Zola R S, Li G, Urbas A M, Bunning T J, Weitz D A 2017 Mater. Horiz. 4 1190
Google Scholar
[6] Ye F F, Mukhopadhyay R, Stenull O, Lubensky T C 2007 Phys. Rev. Lett. 98 147801.1
Google Scholar
[7] Ye F F, Lubensky T C 2009 JPCB 113 3853
Google Scholar
[8] Hough L E, Jung H T, Kruerke D, Heberling M S, Nakata M, Jones C D, Chen D, Link D R, Zasadzinski J, Heppke G 2009 Science 325 456
Google Scholar
[9] Dierking I 2010 Angew. Chem. Int. Ed. 49 29
Google Scholar
[10] Sekine T, Niori T, Sone M, Watanabe J, Takezoe H 1997 Jpn. J. Appl. Phys. 36 6455
Google Scholar
[11] Chen D, Madennan J E, Shao R, Dong K Y, Wang H, Korblova E, Walba D M, Glaser M A, Clark N A 2011 J. Am. Chem. Soc. 133 12656
Google Scholar
[12] Coleman D A, Fernsler J, Chattham N, Nakata M, Takanishi Y, Körblova E, Link D R, Shao R F, Jang W G, Maclennan J E 2003 Science 301 1204
Google Scholar
[13] Thisayukta J, Takezoe H, Watanabe J 2001 Jpn. J. Appl. Phys. 40 3277
Google Scholar
[14] Tschierske C, Dantlgraber G 2003 Pramana 61 455
Google Scholar
[15] Kondepudi D K, Kaufman R J, Singh N 1990 Science 250 975
Google Scholar
[16] Zep A, Salamonczyk M, Vaupotič N, Pociecha D, Gorecka E 2013 Chem. Commun. 49 3119
Google Scholar
[17] Chen D, Zhu C, Wang H, Maclennan J E, Glaser M A, Korblova E, Walba D M, Rego J A, Soto-Bustamante E A, Clark N A 2013 Soft Matter 9 462
Google Scholar
[18] Gleeson H F, Liu H, Kaur S, Srigengan S, Görtz V, Mandle R, Lydon J E 2018 Soft Matter 14 9159
Google Scholar
[19] Matraszek J, Topnani N, Vaupotic N, Takezoe H, Mieczkowski J, Pociecha D, Gorecka E 2015 Angew Chem. Int. Ed. Engl. 128 3529
[20] Shen Z, Jiang Y, Wang T, Liu M 2015 J. Am. Chem. Soc. 137 16109
Google Scholar
[21] Shen Z, Wang T, Shi L, Tang Z, Liu M 2015 Chem. Sci. 6 4267
Google Scholar
[22] Zheludev N I 2010 Science 328 582
Google Scholar
[23] Chen D, Tuchband M R, Horanyi B, Korblova E, Walba D M, Glaser M A, Maclennan J E, Clark N A 2015 Nat. Commun. 6 1
[24] 刘立伟, 王作维, 周鲁卫, 王冶金, 高广君, 刘晓君 2000 49 1887
Google Scholar
Liu L W, Wang Z W, Zhou L W, Wang Z J, Gao G J, Liu X J 2000 Acta Phys. Sin. 49 1887
Google Scholar
[25] Macosko C W 1994 RHEOLOGY Principles, Measurements and Applications (Canada: Wiley-VCH) pp65–106
[26] Mason T G, Weitz D A 1995 Phys. Rev. Lett. 74 1250
Google Scholar
[27] Chen D, Zhu C, Shoemaker R K, Korblova E, Walba D M, Glaser M A, Maclennan J E, Clark N A 2010 Langmuir 26 15541
Google Scholar
下载:
计量
- 文章访问数: 9089
- PDF下载量: 156
- 被引次数: 0
