利用X射线衍射技术对压电材料本征与非本征起源探究的研究进展

张冠杰; 杨豪; 张楠

doi:10.7498/aps.69.20200301

摘要

钙钛矿铁电压电材料具有高介电压电常数和高机电耦合系数等特点, 在工业、消费电子和军事等领域具有广泛的应用, 其压电性能起源的机理及与材料多尺度结构之间的关系一直是凝聚态物理和材料科学领域的研究热点. 铁电材料的压电效应主要来源于本征的场致晶格畸变以及非本征的畴翻转和畴壁运动, 理解并区分这两种压电效应的贡献机制对研究材料压电性能的起源具有重要意义. 本文综述了近年来通过电场原位X射线衍射技术分析电场作用下材料晶格结构和畴结构变化的技术手段和研究方法, 重点介绍了自第三代同步辐射光源和高速探测器获得长足发展以来, 通过时间分辨衍射技术、单双峰拟合、全谱拟合、质心计算等方法开展压电材料本征和非本征贡献, 以及电场诱导相变对其宏观性能影响的研究进展, 期望通过对各类方法的介绍和回顾为多种压电材料的机理分析提供研究方法和技术支持.

关键词:

Abstract

Ferroelectric/piezoelectric perovskites are an important class of functional material and have broad application prospects in commercial, industrial, military and other areas because of their high dielectric constants, high piezoelectric coefficients, and high electromechanical coupling coefficients. Their structures, applications, and physical mechanisms have been intensively studied in condensed matter physics and material science. The piezoelectric properties of ferroelectric materials mainly originate from the intrinsic field-induced lattice distortion and extrinsic domain inversion and domain wall motion. Therefore, the understanding of and the distinguishing between these mechanisms are important for ascertaining the origin of the high-piezoelectric properties and developing new functional materials. In this article, we review the research progress of technical means and methodology of analyzing the changes of crystal lattices and magnetic domains of materials under the action of an externally applied electric field through the high-energy synchrotron X-ray diffraction experiments. The techniques and analysis methods involved in the review cover the time-resolved X-ray diffraction, single/double-peak analysis, full-pattern refinement, center-of-mass calculation, and field-induced phase transformation analysis, which are used to study the intrinsic and extrinsic contributions to sample’s macroscopic properties. It is expected to provide the research methods, which fulfill the individual experimental requirements, and the technical support for the mechanism analysis of various piezoelectric materials through the introduction and review of various methods.

Keywords:

作者及机构信息

西安交通大学, 电子科学与工程学院, 电子陶瓷与器件教育部重点实验室、国际电介质研究中心, 西安　710049

通信作者: 张楠, nzhang1@xjtu.edu.cn

Authors and contacts

Electronic Materials Research Laboratory, Key Laboratory of the Ministry of Education & International Center for Dielectric Research, School of Electronic Science and Engineering, Xi’an Jiaotong University, Xi’an 710049, China

Corresponding author: Zhang Nan, nzhang1@xjtu.edu.cn

文章全文 : translate this paragraph

参考文献

[1]	Berlincourt D 1992 J. Acoust. Soc. Am. 91 3034 Google Scholar
[2]	King T G, Preston M E, Murphy B J M, Cannell D S 1990 Precis. Eng. 12 131 Google Scholar
[3]	Uchino K 2015 Sci. Technol. Adv. Mater. 16 46001 Google Scholar
[4]	Haertling G H 1999 J. Am. Ceram. Soc. 82 797 Google Scholar
[5]	Bellaiche L, Vanderbilt D 1999 Phys. Rev. Lett. 83 1347 Google Scholar
[6]	Li F, Lin D, Chen Z, Cheng Z, Wang J, Li C, Xu Z, Huang Q, Liao X, Chen L Q, Shrout T R, Zhang S 2018 Nat. Mater. 17 349 Google Scholar
[7]	Park S E, Shrout T R 1997 J. Appl. Phys. 82 1804 Google Scholar
[8]	Zhang N, Yokota H, Glazer A M, Ren Z, Keen D A, Keeble D S, Thomas P A, Ye Z G 2014 Nat. Commun. 5 5231 Google Scholar
[9]	Guo R, Cross L E, Park S E, Noheda B, Cox D E, Shirane G 2000 Phys. Rev. Lett. 84 5423 Google Scholar
[10]	Hollenstein E, Davis M, Damjanovic D, Setter N 2005 Appl. Phys. Lett. 87 182905 Google Scholar
[11]	Xu K, Li J, Lv X, Wu J, Zhang X, Xiao D, Zhu J 2016 Adv. Mater. 28 8519 Google Scholar
[12]	Wang X, Wu J, Xiao D, Zhu J, Cheng X, Zheng T, Zhang B, Lou X, Wang X 2014 J. Am. Chem. Soc. 136 2905 Google Scholar
[13]	Wang K, Li J F 2010 Adv. Funct. Mater. 20 1924 Google Scholar
[14]	Saito Y, Takao H, Tani T, Nonoyama T, Takatori K, Homma T, Nagaya T, Nakamura M 2004 Nature 432 84 Google Scholar
[15]	McQuade R R, Dolgos M R 2016 J. Solid State Chem. 242 140 Google Scholar
[16]	Paterson A R, Nagata H, Tan X, Daniels J E, Hinterstein M, Ranjan R, Groszewicz P B, Jo W, Jones J L 2018 MRS Bull. 43 600 Google Scholar
[17]	Du X H, Zheng J, Belegundu U, Uchino K 1998 Appl. Phys. Lett. 72 2421 Google Scholar
[18]	Fu H, Cohen R E 2000 Nature 403 281 Google Scholar
[19]	Noheda B, Cox D E 2006 Phase Transitions 79 5 Google Scholar
[20]	Ye Z G, Noheda B, Dong M, Cox D, Shirane G 2001 Phys. Rev. B 64 184114 Google Scholar
[21]	Li F, Zhang S, Damjanovic D, Chen L Q, Shrout T R 2018 Adv. Funct. Mater. 28 1801504 Google Scholar
[22]	Clegg W 2015 X-ray Crystallography (New York: Oxford University Press) pp1–31
[23]	Rietveld H M 1969 J. Appl. Crystallogr. 2 65 Google Scholar
[24]	Hammond C 2009 The Basics of Crystallography and Diffraction Struct. Chem. (New York: Oxford University Press) pp252–267
[25]	David W I F, Shankland K, Baerlocher C, McCusker L B 2002 Structure Determination from Powder Diffraction Data (New York: Oxford University Press) pp88–93
[26]	Tagantsev A K, Cross L E, Fousek J 2010 Domains in Ferroic Crystals and Thin Films (New York: Springer New York) pp11–74
[27]	Viehland D D, Salje E K H 2014 Adv. Phys. 63 267 Google Scholar
[28]	Jones J L, Aksel E, Tutuncu G, Usher T M, Chen J, Xing X, Studer A J 2012 Phys. Rev. B 86 024104 Google Scholar
[29]	Als-Nielsen J, McMorrow D 2011 Elements of Modern X-ray Physics (Chichester: A John Wiley & Sons, Ltd Publication) pp33–42
[30]	Broennimann C 2008 Acta Crystallogr. Sect. A Found. Crystallogr. 64 C162 Google Scholar
[31]	Daniels J E, Finlayson T R, Studer A J, Hoffman M, Jones J L 2007 J. Appl. Phys. 101 094104 Google Scholar
[32]	Daniels J, Pramanick A, Jones J 2009 IEEE Trans. Ultrason. Ferroelectr. Freq. Control 56 1539 Google Scholar
[33]	Choe H, Bieker J, Zhang N, Glazer A M, Thomas P A, Gorfman S 2018 IUCrJ 5 417 Google Scholar
[34]	Eckold G, Schober H, Nagler S E 2010 Studying Kinetics with Neutrons (Berlin, Heidelberg: Springer Berlin Heidelberg) pp149–173
[35]	Jiang A Q, Lee H J, Hwang C S, Scott J F 2012 Adv. Funct. Mater. 22 192 Google Scholar
[36]	Bai F, Wang N, Li J, Viehland D, Gehring P M, Xu G, Shirane G 2004 J. Appl. Phys. 96 1620 Google Scholar
[37]	Ehara Y, Yasui S, Nagata J, Kan D, Anbusathaiah V, Yamada T, Sakata O, Funakubo H, Nagarajan V 2011 Appl. Phys. Lett. 99 182906 Google Scholar
[38]	Rana D S, Kawayama I, Mavani K, Takahashi K, Murakami H, Tonouchi M 2009 Adv. Mater. 21 2881 Google Scholar
[39]	Eckold G, Gibhardt H, Caspary D, Elter P, Elisbihani K 2003 Z. Kristallogr. 218 144 Google Scholar
[40]	Choe H, Heidbrink S, Ziolkowski M, Pietsch U, Dyadkin V, Gorfman S, Chernyshov D 2017 J. Appl. Crystallogr. 50 975 Google Scholar
[41]	Usher T M, Levin I, Daniels J E, Jones J L 2015 Sci. Rep. 5 14678 Google Scholar
[42]	Vergentev T, Bronwald I, Chernyshov D, Gorfman S, Ryding S H M, Thompson P, Cernik R J 2016 J. Appl. Crystallogr. 49 1501 Google Scholar
[43]	Kitanaka Y, Noguchi Y, Miyayama M, Kagawa Y, Moriyoshi C, Kuroiwa Y 2013 Ferroelectrics 443 1 Google Scholar
[44]	Moriyoshi C, Hiramoto S, Ohkubo H, Kuroiwa Y, Osawa H, Sugimoto K, Kimura S, Takata M, Kitanaka Y, Noguchi Y, Miyayama M 2011 Jpn. J. Appl. Phys. 50 09NE05 Google Scholar
[45]	Gorfman S, Keeble D S, Glazer A M, Long X, Xie Y, Ye Z G, Collins S, Thomas P A 2011 Phys. Rev. B 84 020102 Google Scholar
[46]	Gorfman S, Thomas P A 2010 J. Appl. Crystallogr. 43 1409 Google Scholar
[47]	Datta K, Gorfman S, Thomas P A 2009 Appl. Phys. Lett. 95 251901 Google Scholar
[48]	Daymond M R 2004 J. Appl. Phys. 96 4263 Google Scholar
[49]	Pramanick A, Damjanovic D, Daniels J E, Nino J C, Jones J L 2011 J. Am. Ceram. Soc. 94 293 Google Scholar
[50]	Ehmke M C, Khansur N H, Daniels J E, Blendell J E, Bowman K J 2014 Acta Mater. 66 340 Google Scholar
[51]	Jones J L, Slamovich E B, Bowman K J 2005 J. Appl. Phys. 97 034113 Google Scholar
[52]	Jones J L, Hoffman M, Bowman K J 2005 J. Appl. Phys. 98 024115 Google Scholar
[53]	Kungl H, Theissmann R, Knapp M, Baehtz C, Fuess H, Wagner S, Fett T, Hoffmann M J 2007 Acta Mater. 55 1849 Google Scholar
[54]	Hall D A, Steuwer A, Cherdhirunkorn B, Mori T, Withers P J 2004 J. Appl. Phys. 96 4245 Google Scholar
[55]	Fan L, Chen J, Ren Y, Pan Z, Zhang L, Xing X 2016 Phys. Rev. Lett. 116 027601 Google Scholar
[56]	Hinterstein M, Lee K Y, Esslinger S, Glaum J, Studer A J, Hoffman M, Hoffmann M J 2019 Phys. Rev. B 99 174107 Google Scholar
[57]	Matthies S, Lutteroti L, Wenk H R 1997 J. Appl. Crystallogr. 30 31 Google Scholar
[58]	Lutterotti L, Bortolotti M, Ischia G, Lonardelli I, Wenk H R 2007 Z. Kristallogr. Suppl. 26 125 Google Scholar
[59]	Hinterstein M, Hoelzel M, Rouquette J, Haines J, Glaum J, Kungl H, Hoffman M 2015 Acta Mater. 94 319 Google Scholar
[60]	Khansur N H, Hinterstein M, Wang Z, Groh C, Jo W, Daniels J E 2015 Appl. Phys. Lett. 107 242902 Google Scholar
[61]	Zhao C, Hou D, Chung C-C, Zhou H, Kynast A, Hennig E, Liu W, Li S, Jones J L 2018 Acta Mater. 158 369 Google Scholar
[62]	Fu J, Zuo R, Xu Y, Li J F, Shi M 2017 J. Eur. Ceram. Soc. 37 975 Google Scholar
[63]	Ochoa D A, Esteves G, Iamsasri T, Rubio-Marcos F, Fernández J F, García J E, Jones J L 2016 J. Eur. Ceram. Soc. 36 2489 Google Scholar
[64]	Zheng T, Wu H, Yuan Y, Lv X, Li Q, Men T, Zhao C, Xiao D, Wu J, Wang K, Li J F, Gu Y, Zhu J, Pennycook S J 2017 Energy Environ. Sci. 10 528 Google Scholar
[65]	Tutuncu G, Li B, Bowman K, Jones J L 2014 J. Appl. Phys. 115 144104 Google Scholar
[66]	Khansur N H, Rojac T, Damjanovic D, Reinhard C, Webber K G, Kimpton J A, Daniels J E 2015 J. Am. Ceram. Soc. 98 3884 Google Scholar
[67]	Li Y, Chen Y, Zhang Z, Kleppe A, Hall D A 2019 Acta Mater. 168 411 Google Scholar
[68]	Zuo R, Qi H, Fu J, Li J F, Li L 2017 Appl. Phys. Lett. 111 132901 Google Scholar
[69]	Hu C, Meng X, Zhang M H, Tian H, Daniels J E, Tan P, Huang F, Li L, Wang K, Li J F, Lu Q, Cao W, Zhou Z 2020 Sci. Adv. 6 eaay5979 Google Scholar
[70]	Zhang N, Gorfman S, Choe H, Vergentev T, Dyadkin V, Yokota H, Chernyshov D, Wang B, Glazer A M, Ren W, Ye Z G 2018 J. Appl. Crystallogr. 51 1396 Google Scholar
[71]	Hinterstein M, Knapp M, Hölzel M, Jo W, Cervellino A, Ehrenberg H, Fuess H 2010 J. Appl. Crystallogr. 43 1314 Google Scholar
[72]	Kling J, Tan X, Jo W, Kleebe H J, Fuess H, Rödel J 2010 J. Am. Ceram. Soc. 93 2452 Google Scholar
[73]	Daniels J E, Jo W, Rödel J, Honkimäki V, Jones J L 2010 Acta Mater. 58 2103 Google Scholar
[74]	Durbin M K, Jacobs E W, Hicks J C, Park S E 1999 Appl. Phys. Lett. 74 2848 Google Scholar
[75]	Damjanovic D 2005 J. Am. Ceram. Soc. 88 2663 Google Scholar
[76]	Noheda B, Cox D E, Shirane G, Park S E, Cross L E, Zhong Z 2001 Phys. Rev. Lett. 86 3891 Google Scholar
[77]	Fu J, Zuo R, Gao X 2013 Appl. Phys. Lett. 103 182907 Google Scholar
[78]	Li P, Zhai J, Shen B, Zhang S, Li X, Zhu F, Zhang X 2018 Adv. Mater. 30 1705171 Google Scholar
[79]	Yao F Z, Wang K, Jo W, Webber K G, Comyn T P, Ding J X, Xu B, Cheng L Q, Zheng M P, Hou Y D, Li J F 2016 Adv. Funct. Mater. 26 1217 Google Scholar
[80]	Simons H, Daniels J E, Glaum J, Studer A J, Jones J L, Hoffman M 2013 Appl. Phys. Lett. 102 062902 Google Scholar
[81]	Ren P, Liu Z, Liu H, Sun S, Wan Y, Long C, Shi J, Chen J, Zhao G 2019 J. Eur. Ceram. Soc. 39 994 Google Scholar
[82]	Wang G, Fan Z, Murakami S, Lu Z, Hall D A, Sinclair D C, Feteira A, Tan X, Jones J L, Kleppe A K, Wang D, Reaney I M 2019 J. Mater. Chem. A 7 21254 Google Scholar
[83]	Xu G, Zhong Z, Bing Y, Ye Z G, Shirane G 2006 Nat. Mater. 5 134 Google Scholar
[84]	Xu G, Wen J, Stock C, Gehring P M 2008 Nat. Mater. 7 562 Google Scholar
[85]	Paściak M, Welberry T R, Kulda J, Kempa M, Hlinka J 2012 Phys. Rev. B 85 224109 Google Scholar
[86]	Li F, Zhang S, Yang T, Xu Z, Zhang N, Liu G, Wang J, Wang J, Cheng Z, Ye Z G, Luo J, Shrout T R, Chen L Q 2016 Nat. Commun. 7 13807 Google Scholar
[87]	Polinger V, Bersuker I B 2018 Phys. Rev. B 98 214102 Google Scholar
[88]	Bokov A A, Ye Z G 2006 J. Mater. Sci. 41 31 Google Scholar
[89]	Xu G, Zhong Z, Hiraka H, Shirane G 2004 Phys. Rev. B 70 174109 Google Scholar
[90]	Welberry T R 2004 Diffuse X-Ray Scattering and Models of Disorder (New York: Oxford University Press) pp4−20

施引文献

图 1 频闪数据收集原理

Fig. 1. Principle of stroboscopic data-acquisition

下载: 全尺寸图片幻灯片

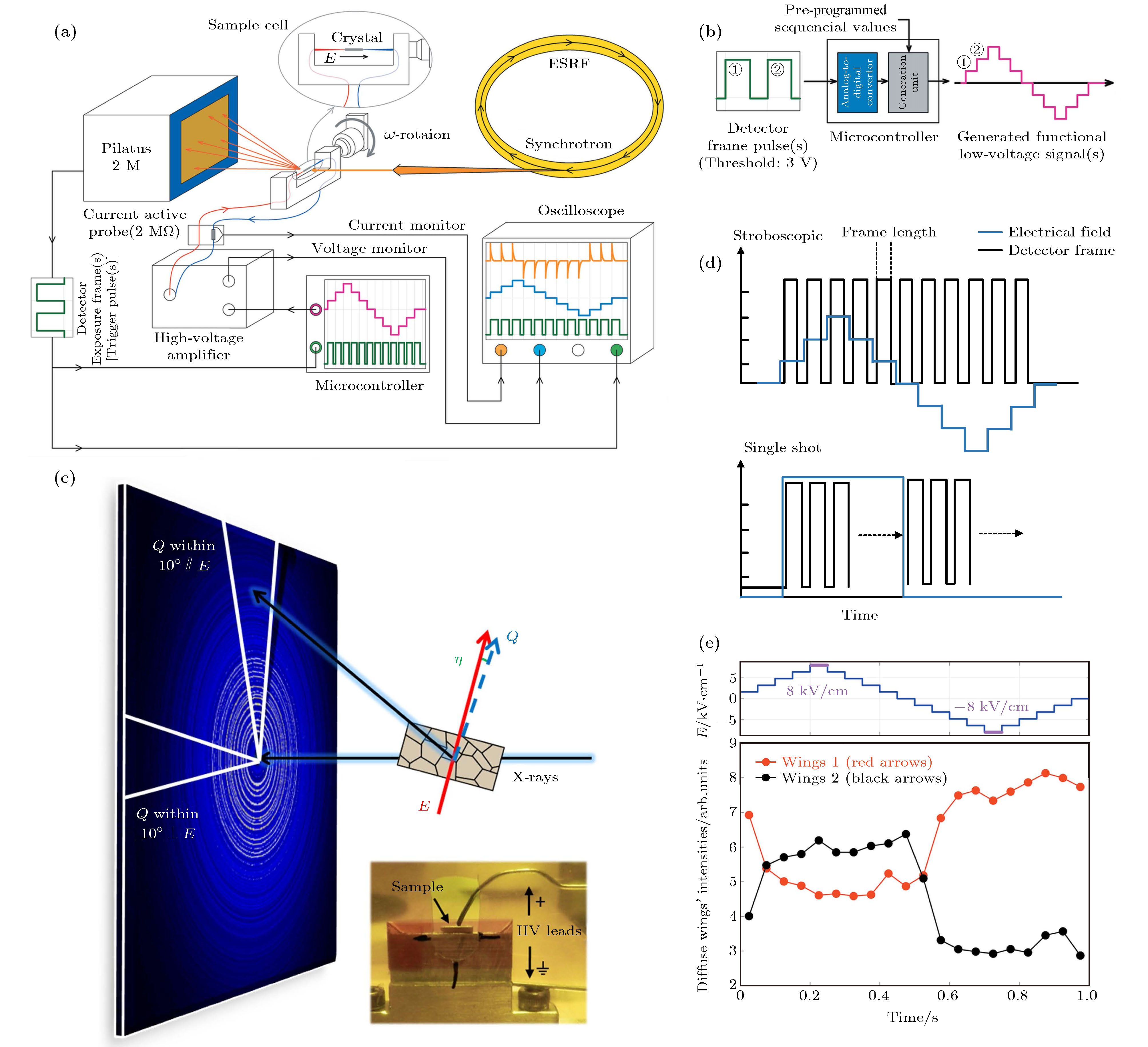
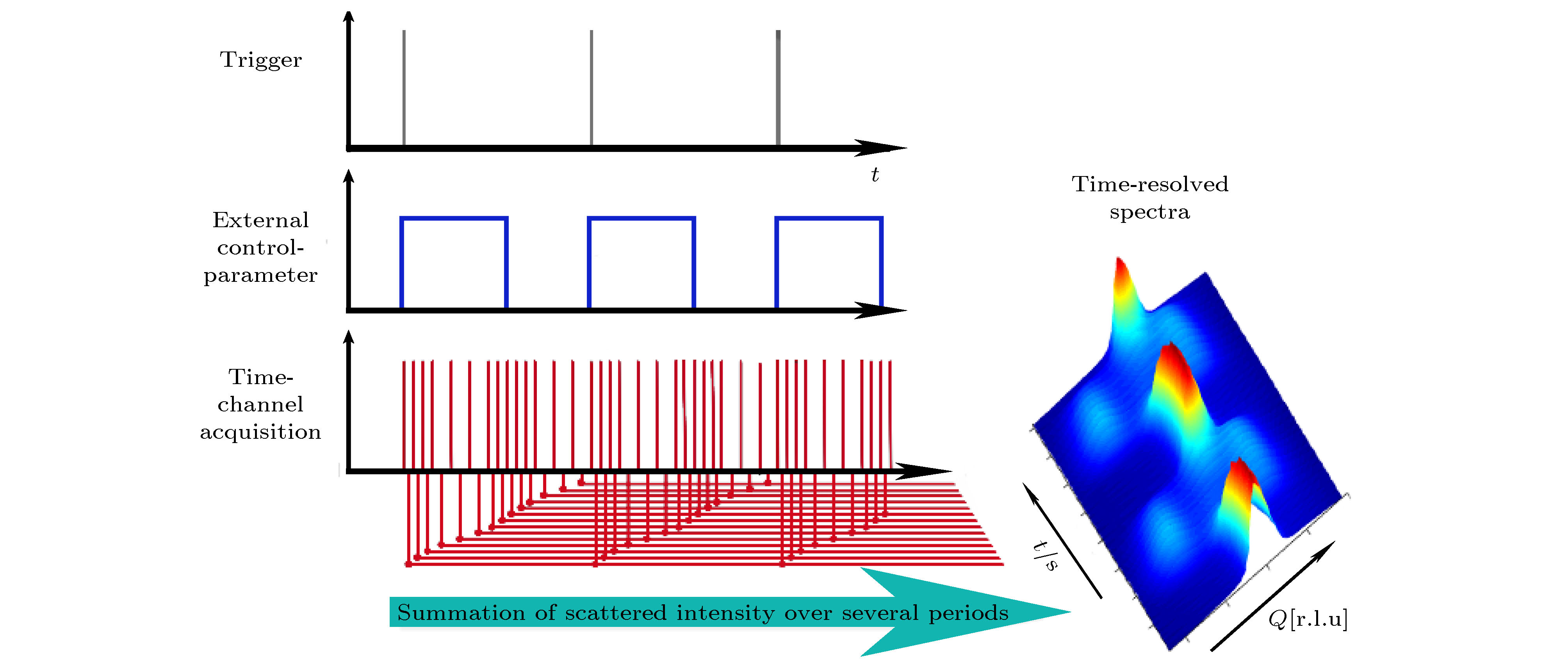
图 2 (a) Choe等^[40]的数据采集系统; (b) Choe等^[40]的系统中信号同步过程; (c) Daniels等^[41]的数据采集系统; (d) Daniels等的系统中数据采集的时间序列; (e)频闪技术中样品所施加电场与时间的关系, 以及相关衍射强度随电场变化趋势^[40]

Fig. 2. (a) Data acquisition system by Choe et al.^[40]; (b) signal synchronization process in the system of Choe et al.^[40]; (c) data acquisition system by Daniels et al.^[41]; (d) timing sequences for data acquisition processes in the system of Daniels et al.; (e) time dependence of the AC electric field and the collected intensity of diffraction wings, showing the field-induced intensity exchange between the two wings^[40]. (a) (b) (e) Copyright © 2017 International Union of Crystallography. Reproduced with permission of the International Union of Crystallography.

下载: 全尺寸图片幻灯片

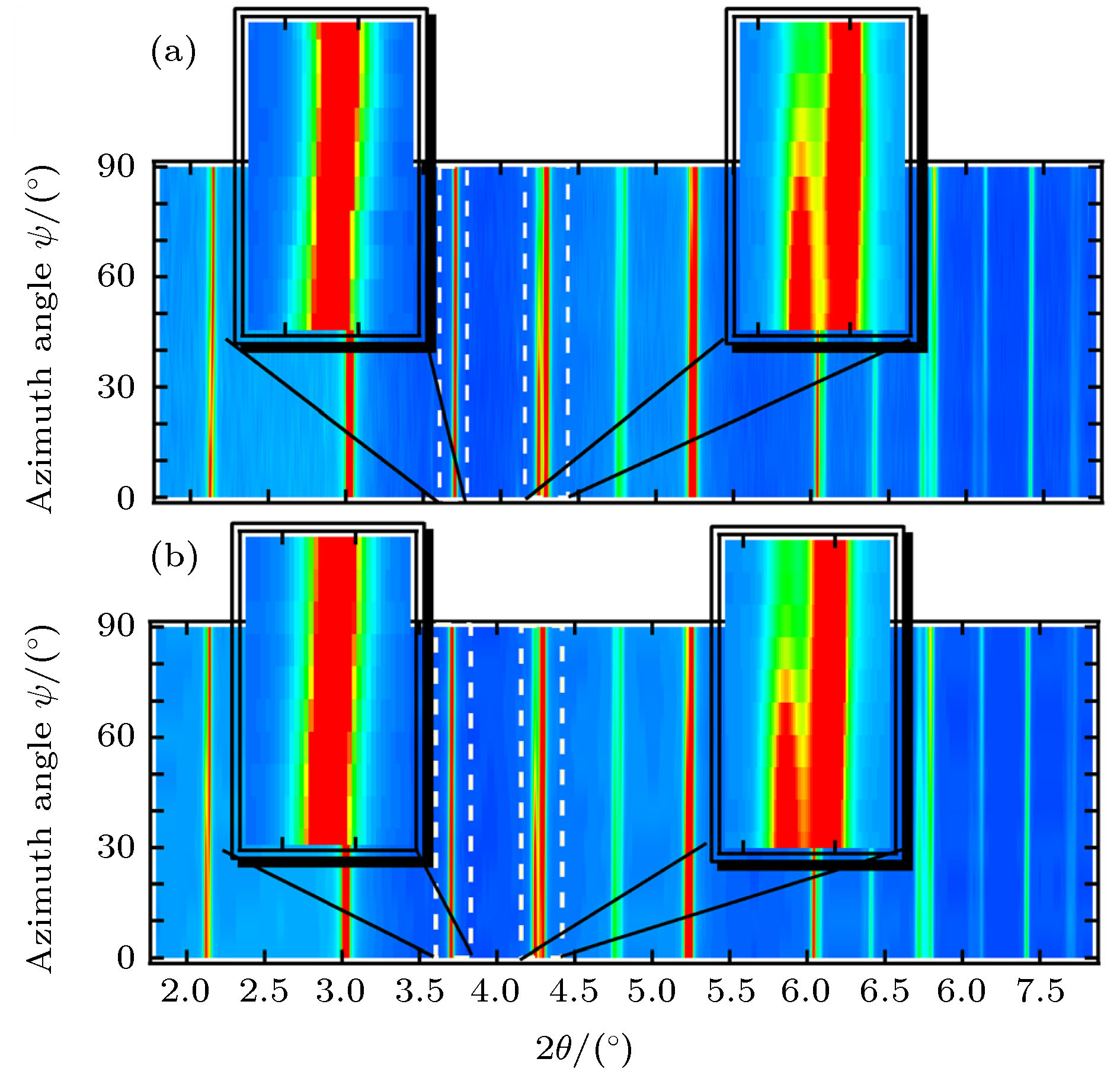
图 3 NBT单晶{00h}衍射峰X-ray衍射峰强度　(a) {002}衍射峰的静态ω-2θ二维衍射图像; (b)外加电场(沿[001]方向)与时间的关系; (c)−(e)使用频闪技术收集到的{004}衍射峰的ω-2θ二维衍射图像, 分别对应不同的时间通道与电场^[33]

Fig. 3. Diffraction intensity of the X-ray around the {00h} family of reflections of NBT single crystal: (a) Static ω versus 2θ mesh of the {002} reflections family; (b) the time-dependence of the applied external electric field (along [001]); (c)−(e) stroboscopically collected versus 2θ meshes of the {004} family of reflections, corresponding to different time channels and electric fields^[33]

下载: 全尺寸图片幻灯片

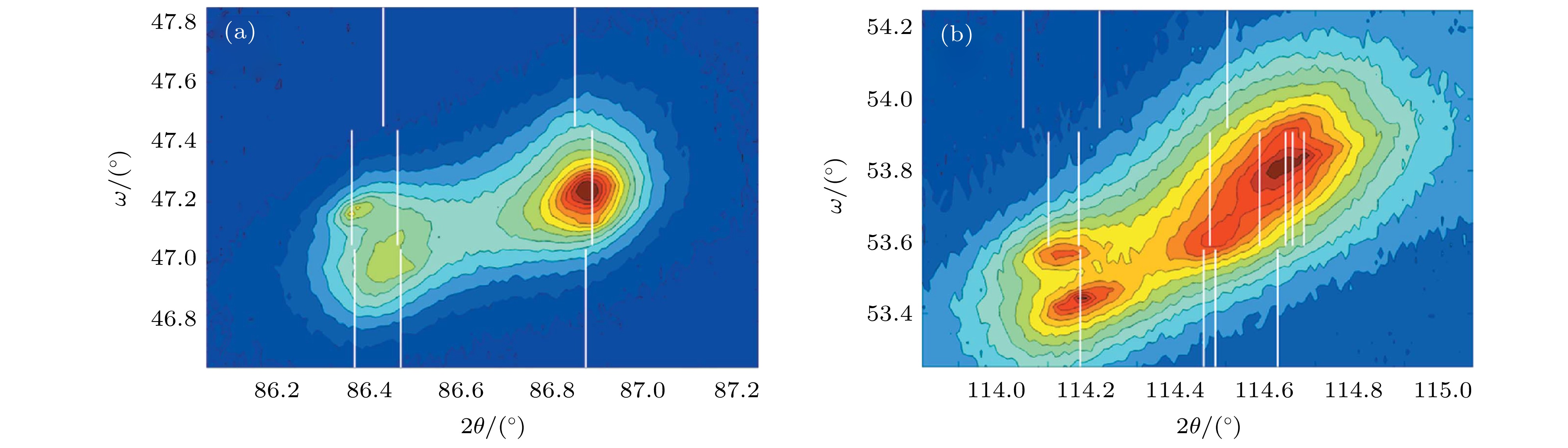
图 4 NBT单晶中不同{hkl}_pc衍射峰的ω-2θ二维衍射图像, 其中从上到下的白线表示三方相, 单斜相C_c和三方-四方混合相可能的分峰$ 2\theta $位置　 (a) {222}; (b) {114}^[46]

Fig. 4. Two ω versus 2θ maps for different {hkl}_pc of NBT single crystal collected on the high-resolution diffractometer. The lines indicate the simulated position of the scattering angle: from top to bottom, rhombohedral, monoclinic, and a combination of rhombohedral and tetragonal: (a) {222}; (b) {114}^[46] (Copyright © 2010 International Union of Crystallography. Reproduced with permission of the International Union of Crystallography)

下载: 全尺寸图片幻灯片

图 5 时间分辨高能X射线衍射装置以及德拜环不同区域分别对应晶粒方向与电场不同夹角的衍射图谱^[49]

Fig. 5. Experimental set-up for time-resolved high-energy X-ray diffraction. Different sections in the Debye ring correspond to grains with specific angles respect to the applied E field^[49] (Copyright © 2011 John Wiley and Sons).

下载: 全尺寸图片幻灯片

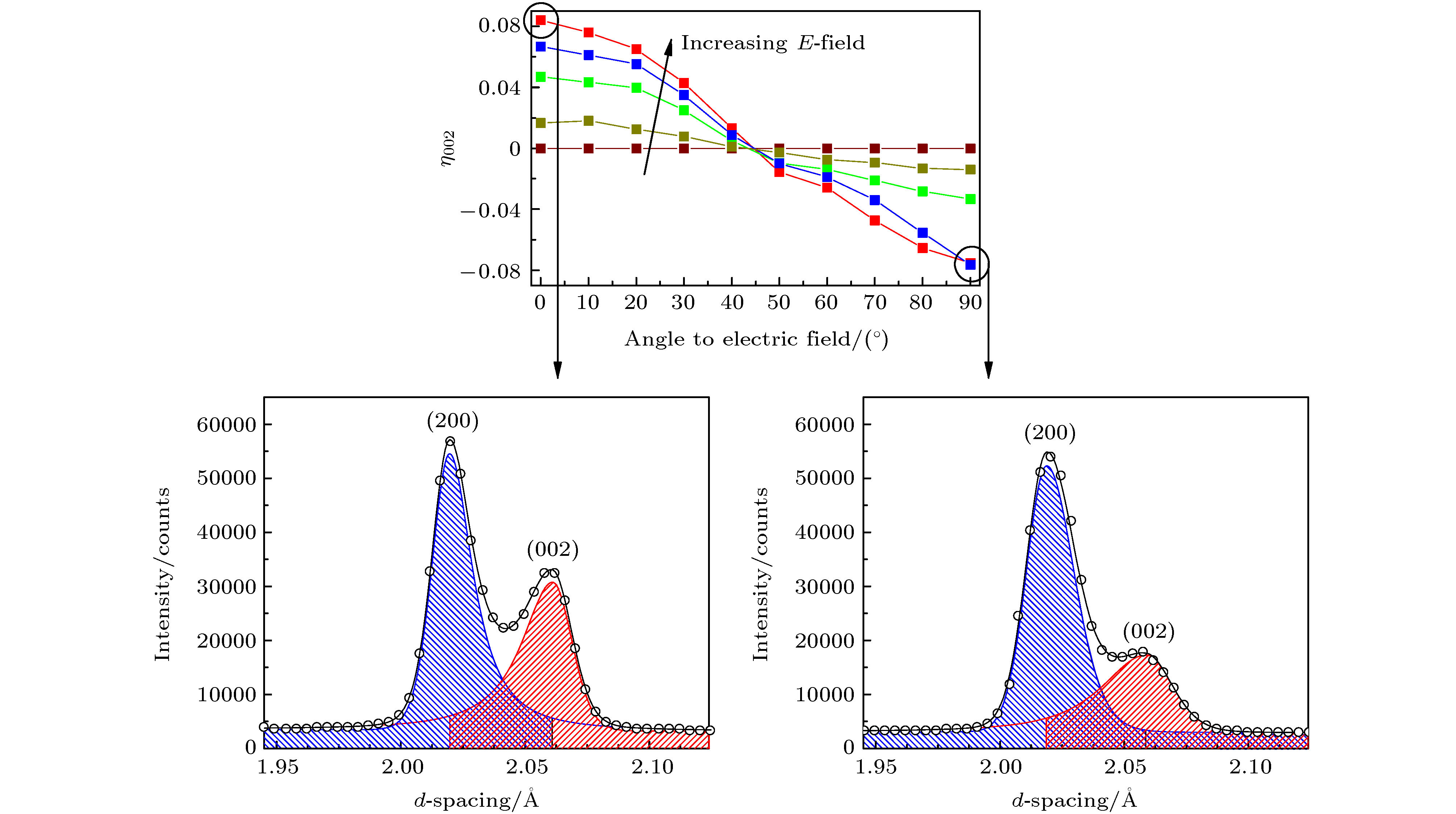
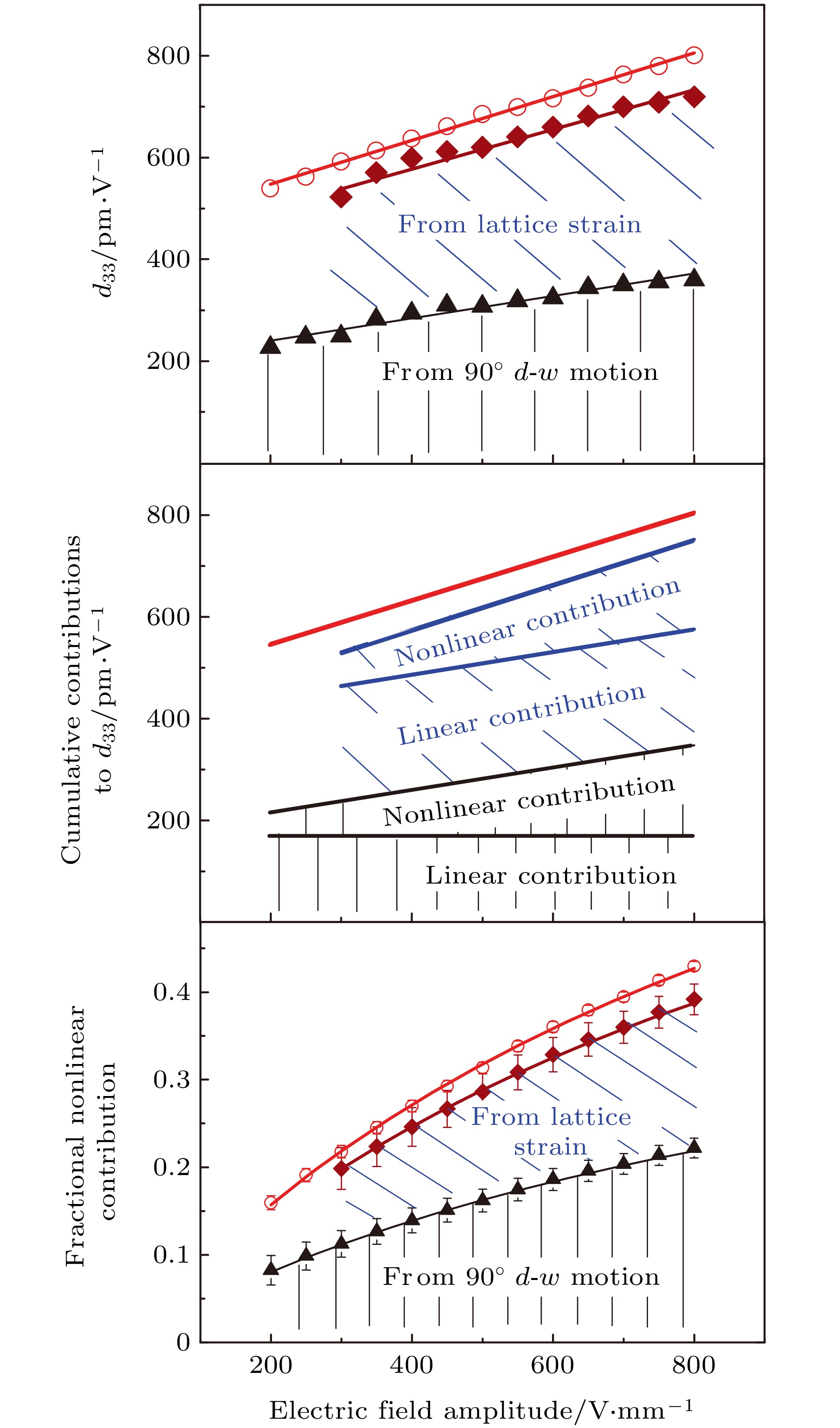
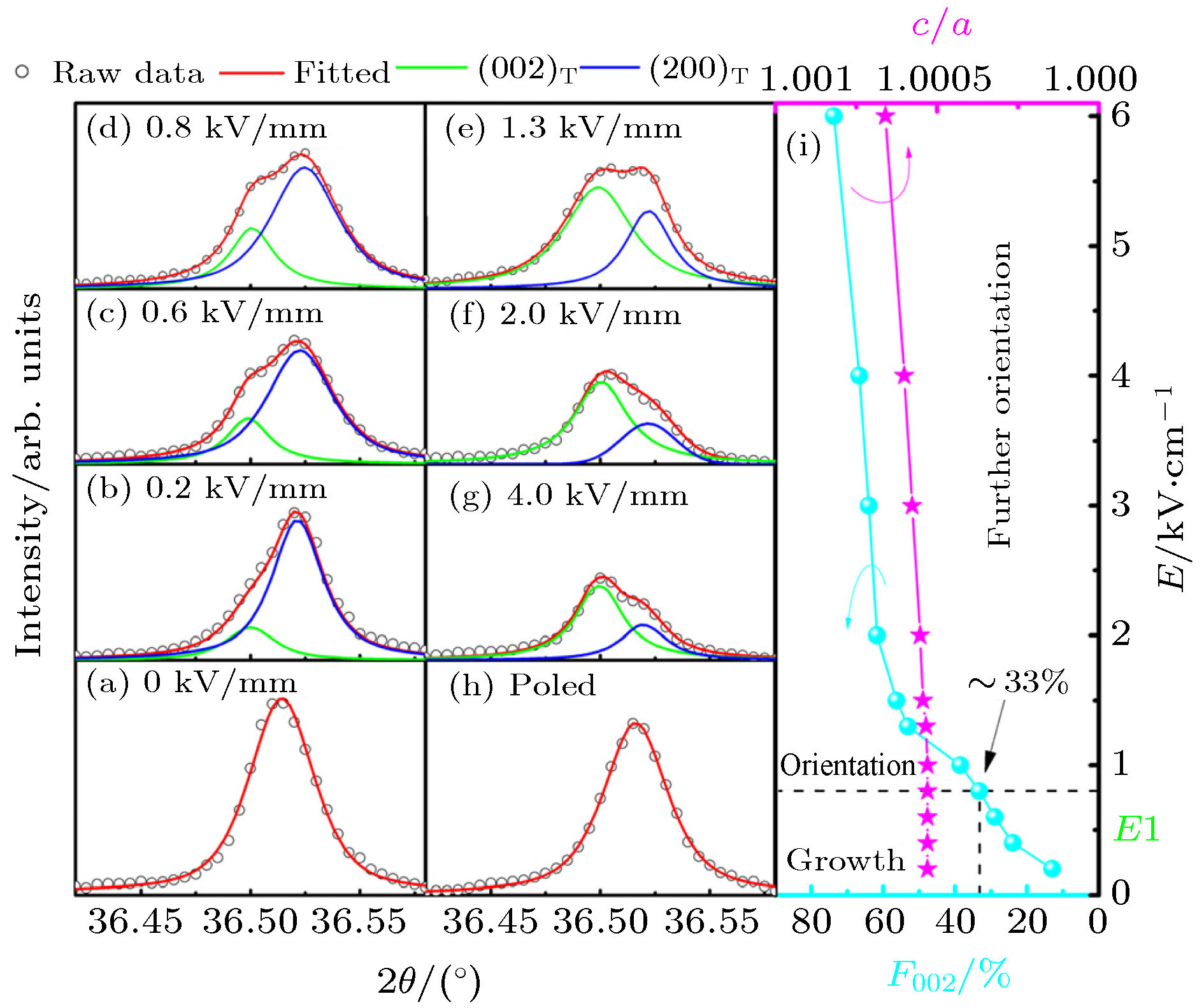
图 6 La掺杂PZT陶瓷中002畴体积分数与电场不同夹角的关系(底图分别显示与电场呈0°与90°条件下(002)与(200)衍射峰体积分数的变化)^[49]

Fig. 6. η₀₀₂ as a function of the field amplitude as well as orientation with respect to the direction of applied field, for an unpoled La-doped tetragonal PZT ceramic under the application of static electric fields. The measured and fitted (002)-type diffraction peaks corresponding to the particular values of η₀₀₂ (marked by circles and indicated by arrows) are shown in the bottom section of the figure. For the fitted diffraction patterns, the deconvoluted (200) and (002) peaks are shown in black solid lines. The integration of individual (002) and (200) peaks are terminated beyond the peak position of the adjacent peak, as indicated by the color-shaded areas^[49] (Copyright © 2011 John Wiley and Sons).

下载: 全尺寸图片幻灯片

图 7 NBT-BT陶瓷在施加最大电场E_max = 4 kV/mm下的实验(a)和模拟(b)所得的取向相关衍射图样^[60]

Fig. 7. Measured (a) and modelled (b) orientation dependent diffraction patterns of NBT-BT at maximum field E_max = 4 kV/mm^[60] (Copyright © 2015 AIP Publishing).

下载: 全尺寸图片幻灯片

图 8 La掺杂的PbZr_0.52Ti_0.48O₃陶瓷中晶格应变与畴壁运动对宏观压电常数及非线性压电常数的贡献^[49]

Fig. 8. Contributions of lattice strain and domain wall motion to macroscopic piezoelectric coefficient and non-linear piezoelectric coefficient in La-doped PbZr_0.52Ti_0.48O₃ ceramics^[49] (Copyright © 2011 John Wiley and Sons)

下载: 全尺寸图片幻灯片

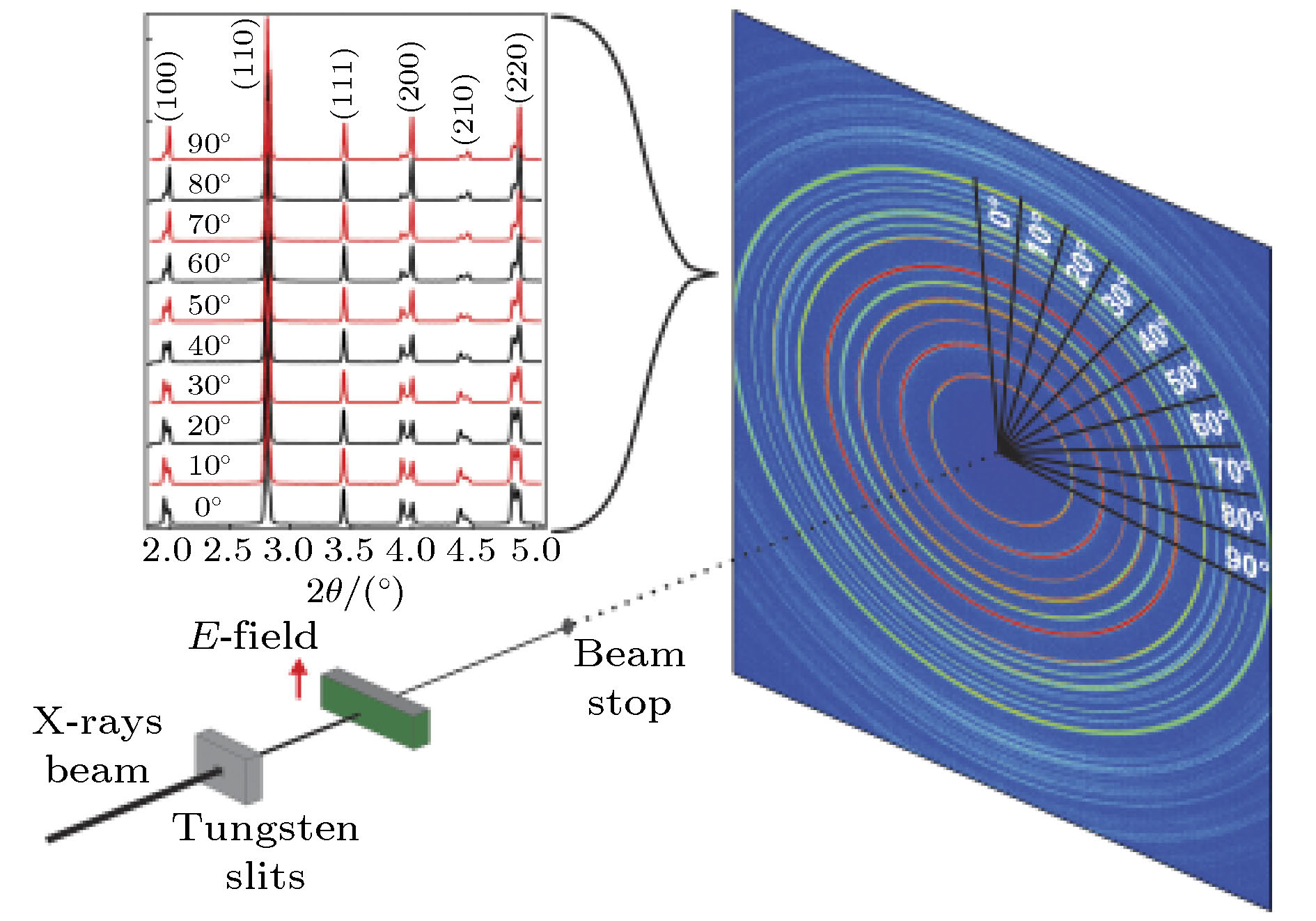
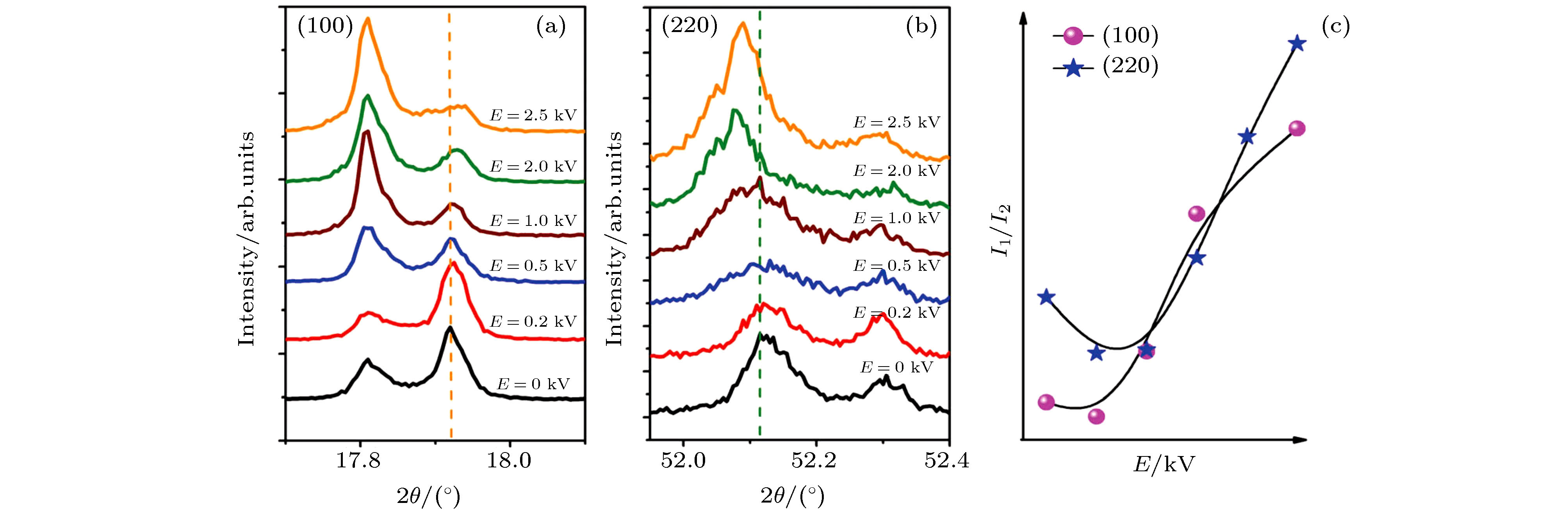
图 9 (1–x)(K_1–yNa_y)(Nb_1–zSb_z)O₃-xBi_0.5(Na_1–wK_w)_0.5HfO₃ (x = 0.035, y = 0.52, z = 0.05, w = 0.18)陶瓷　(a), (b) (100)和(220)衍射峰随电场的演变过程; (c) (100)和(220)衍射峰中低角度衍射峰与高角度衍射峰的强度之比(I₁/I₂)随电场的变化^[64]

Fig. 9. (1–x)(K_1–yNa_y)(Nb_1–zSb_z)O₃-xBi_0.5(Na_1–wK_w)_0.5HfO₃ ceramic with x = 0.035, y = 0.52, z = 0.05 and w = 0.18: (a), (b) Evolution of the (100) and (220) pseudocubic reflections as a function of the electric field; (c) ratio of low angle peak intensity to high angle intensity (I₁/I₂) for (100) and (220) pseudocubic reflections as a function of the electric field^[64] (Copyright © 2017 The Royal Society of Chemistry)

下载: 全尺寸图片幻灯片

图 10 NN-BT陶瓷{200}衍射峰在电场作用下的重新分布现象^[68]

Fig. 10. {200} reflections and their redistributions under electric field for NN-BT^[68] (Copyright © 2017 AIP Publishing)

下载: 全尺寸图片幻灯片

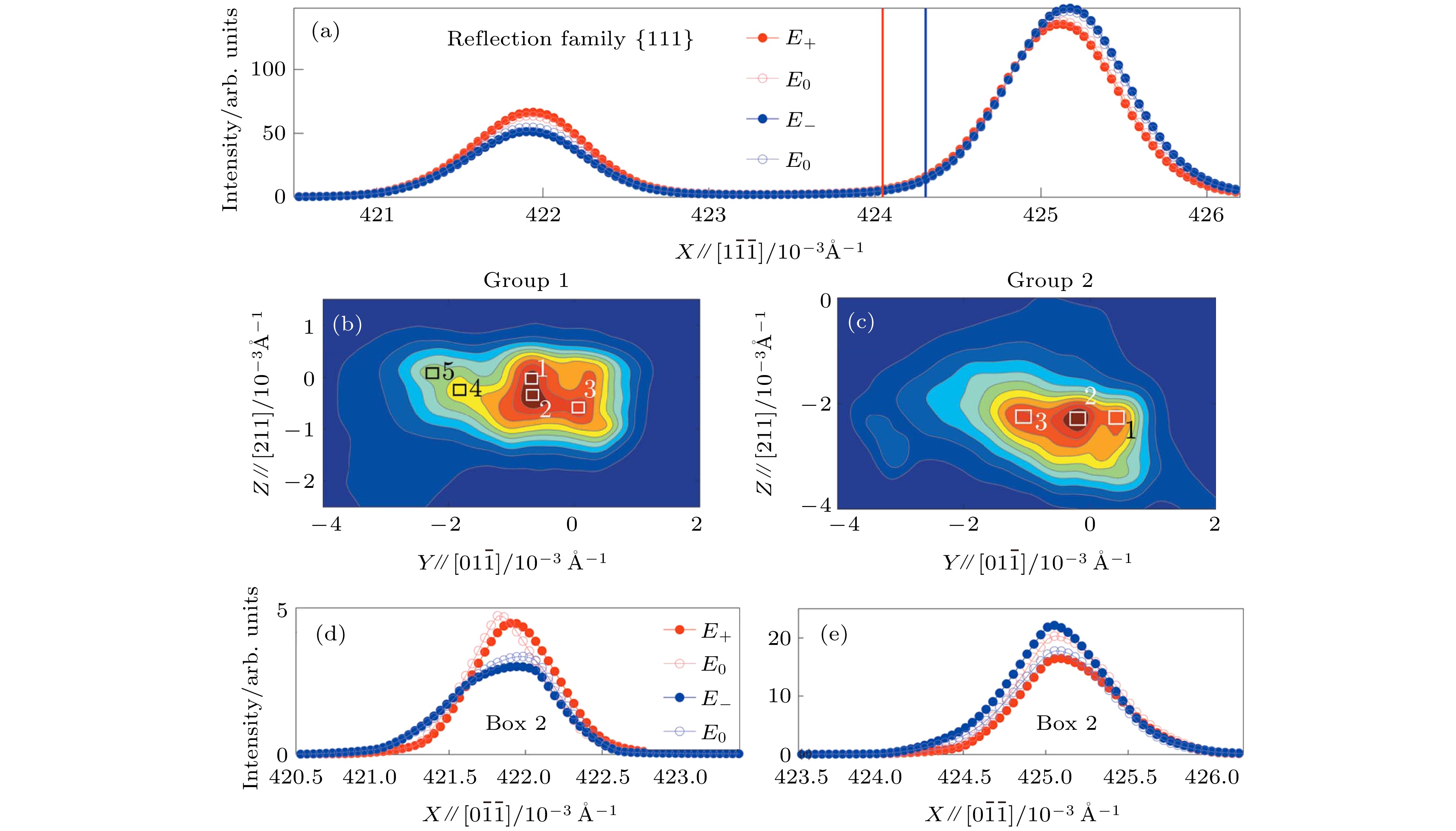
图 11 (a) {111}衍射峰的衍射强度(沿YZ方向积分)与X的关系曲线, 垂直的红蓝线分别对应$ {E}_{+}\backslash {E}_{-} $状态下的质心位置; (b), (c)沿不同X范围积分的二维衍射强度分布图, 分别对应图(a)中的Group 1和Group 2; (d), (e)一个YZ Box范围内积分的衍射强度与X的关系曲线, 其中(d), (e)分别对应Group 1中的Box 2和Group 2中的Box 2 ^[70]

Fig. 11. (a) The X dependence of the diffraction intensity around {111} reflections, integrated within the full YZ range. The vertical red and blue lines mark the center of mass positions corresponding to the E₊ and ${E_ - } $ states. (b), (c) YZ dependence of the diffraction intensity integrated within two ranges of X, corresponding to Group 1 and Group 2 in panel (a). Several boxes are marked to show the positions of Bragg peak sub-components. (d), (e) Integrated intensities within one YZ box against X under four states of field. (d) Corresponds to Box 2 in Group 1 and (e) to Box 2 in Group 2^[70] (Copyright © 2018 International Union of Crystallography. Reproduced with permission of the International Union of Crystallography)

下载: 全尺寸图片幻灯片

图 12 三方相-四方相相变中可能的极化矢量旋转路径^[18,76]

Fig. 12. The two possible paths for the polarization direction to change from [111] in the rhombohedral (R) phase to [001] in the tetragonal (T) phase^[18,76] (Copyright © 2001 American Physical Society)

下载: 全尺寸图片幻灯片

图 13 KNN基陶瓷中的三方相-单斜相-正交相的极化旋转路径

Fig. 13. Polarization rotation path of rhombohedral-monoclinic-orthorhombic phase in KNN-based ceramic

下载: 全尺寸图片幻灯片

图 14 对于0.94NBT-0.06BT陶瓷　(a) 在25, 50, 75和100 ℃下的单极电场-应变曲线; (b) 可恢复应变(S_max – S_rem)的温度依赖关系 ^[80]

Fig. 14. For 0.94NBT-0.06BT ceramic, (a) unipolar strain hysteresis at 25, 50, 75, and 100 ℃; (b) temperature-dependence of recoverable strain (S_max – S_rem)^[80] (Copyright © 2013 AIP Publishing)

下载: 全尺寸图片幻灯片

图 15 对BF-0.3 BT-0.03 NLN陶瓷在 ± 60 kV/cm的电场下进行了两个电场循环观察到的{111}, {200}和{220}峰的等高线图 (a)平行电场方向; (b)垂直电场方向^[82]

Fig. 15. Contour plots of the {111}, {200} and {220} peak profiles for (a) $ \beta $ = 0° and (b) $ \beta $ = 90° obtained from the in situ X-ray diffraction experiment for BF-0.3 BT-0.03 NLN, with two cycles of electric field poling under ± 60 kV/cm^[82] (Copyright © 2019 The Royal Society of Chemistry)

下载: 全尺寸图片幻灯片

map

[1]	Berlincourt D 1992 J. Acoust. Soc. Am. 91 3034 Google Scholar
[2]	King T G, Preston M E, Murphy B J M, Cannell D S 1990 Precis. Eng. 12 131 Google Scholar
[3]	Uchino K 2015 Sci. Technol. Adv. Mater. 16 46001 Google Scholar
[4]	Haertling G H 1999 J. Am. Ceram. Soc. 82 797 Google Scholar
[5]	Bellaiche L, Vanderbilt D 1999 Phys. Rev. Lett. 83 1347 Google Scholar
[6]	Li F, Lin D, Chen Z, Cheng Z, Wang J, Li C, Xu Z, Huang Q, Liao X, Chen L Q, Shrout T R, Zhang S 2018 Nat. Mater. 17 349 Google Scholar
[7]	Park S E, Shrout T R 1997 J. Appl. Phys. 82 1804 Google Scholar
[8]	Zhang N, Yokota H, Glazer A M, Ren Z, Keen D A, Keeble D S, Thomas P A, Ye Z G 2014 Nat. Commun. 5 5231 Google Scholar
[9]	Guo R, Cross L E, Park S E, Noheda B, Cox D E, Shirane G 2000 Phys. Rev. Lett. 84 5423 Google Scholar
[10]	Hollenstein E, Davis M, Damjanovic D, Setter N 2005 Appl. Phys. Lett. 87 182905 Google Scholar
[11]	Xu K, Li J, Lv X, Wu J, Zhang X, Xiao D, Zhu J 2016 Adv. Mater. 28 8519 Google Scholar
[12]	Wang X, Wu J, Xiao D, Zhu J, Cheng X, Zheng T, Zhang B, Lou X, Wang X 2014 J. Am. Chem. Soc. 136 2905 Google Scholar
[13]	Wang K, Li J F 2010 Adv. Funct. Mater. 20 1924 Google Scholar
[14]	Saito Y, Takao H, Tani T, Nonoyama T, Takatori K, Homma T, Nagaya T, Nakamura M 2004 Nature 432 84 Google Scholar
[15]	McQuade R R, Dolgos M R 2016 J. Solid State Chem. 242 140 Google Scholar
[16]	Paterson A R, Nagata H, Tan X, Daniels J E, Hinterstein M, Ranjan R, Groszewicz P B, Jo W, Jones J L 2018 MRS Bull. 43 600 Google Scholar
[17]	Du X H, Zheng J, Belegundu U, Uchino K 1998 Appl. Phys. Lett. 72 2421 Google Scholar
[18]	Fu H, Cohen R E 2000 Nature 403 281 Google Scholar
[19]	Noheda B, Cox D E 2006 Phase Transitions 79 5 Google Scholar
[20]	Ye Z G, Noheda B, Dong M, Cox D, Shirane G 2001 Phys. Rev. B 64 184114 Google Scholar
[21]	Li F, Zhang S, Damjanovic D, Chen L Q, Shrout T R 2018 Adv. Funct. Mater. 28 1801504 Google Scholar
[22]	Clegg W 2015 X-ray Crystallography (New York: Oxford University Press) pp1–31
[23]	Rietveld H M 1969 J. Appl. Crystallogr. 2 65 Google Scholar
[24]	Hammond C 2009 The Basics of Crystallography and Diffraction Struct. Chem. (New York: Oxford University Press) pp252–267
[25]	David W I F, Shankland K, Baerlocher C, McCusker L B 2002 Structure Determination from Powder Diffraction Data (New York: Oxford University Press) pp88–93
[26]	Tagantsev A K, Cross L E, Fousek J 2010 Domains in Ferroic Crystals and Thin Films (New York: Springer New York) pp11–74
[27]	Viehland D D, Salje E K H 2014 Adv. Phys. 63 267 Google Scholar
[28]	Jones J L, Aksel E, Tutuncu G, Usher T M, Chen J, Xing X, Studer A J 2012 Phys. Rev. B 86 024104 Google Scholar
[29]	Als-Nielsen J, McMorrow D 2011 Elements of Modern X-ray Physics (Chichester: A John Wiley & Sons, Ltd Publication) pp33–42
[30]	Broennimann C 2008 Acta Crystallogr. Sect. A Found. Crystallogr. 64 C162 Google Scholar
[31]	Daniels J E, Finlayson T R, Studer A J, Hoffman M, Jones J L 2007 J. Appl. Phys. 101 094104 Google Scholar
[32]	Daniels J, Pramanick A, Jones J 2009 IEEE Trans. Ultrason. Ferroelectr. Freq. Control 56 1539 Google Scholar
[33]	Choe H, Bieker J, Zhang N, Glazer A M, Thomas P A, Gorfman S 2018 IUCrJ 5 417 Google Scholar
[34]	Eckold G, Schober H, Nagler S E 2010 Studying Kinetics with Neutrons (Berlin, Heidelberg: Springer Berlin Heidelberg) pp149–173
[35]	Jiang A Q, Lee H J, Hwang C S, Scott J F 2012 Adv. Funct. Mater. 22 192 Google Scholar
[36]	Bai F, Wang N, Li J, Viehland D, Gehring P M, Xu G, Shirane G 2004 J. Appl. Phys. 96 1620 Google Scholar
[37]	Ehara Y, Yasui S, Nagata J, Kan D, Anbusathaiah V, Yamada T, Sakata O, Funakubo H, Nagarajan V 2011 Appl. Phys. Lett. 99 182906 Google Scholar
[38]	Rana D S, Kawayama I, Mavani K, Takahashi K, Murakami H, Tonouchi M 2009 Adv. Mater. 21 2881 Google Scholar
[39]	Eckold G, Gibhardt H, Caspary D, Elter P, Elisbihani K 2003 Z. Kristallogr. 218 144 Google Scholar
[40]	Choe H, Heidbrink S, Ziolkowski M, Pietsch U, Dyadkin V, Gorfman S, Chernyshov D 2017 J. Appl. Crystallogr. 50 975 Google Scholar
[41]	Usher T M, Levin I, Daniels J E, Jones J L 2015 Sci. Rep. 5 14678 Google Scholar
[42]	Vergentev T, Bronwald I, Chernyshov D, Gorfman S, Ryding S H M, Thompson P, Cernik R J 2016 J. Appl. Crystallogr. 49 1501 Google Scholar
[43]	Kitanaka Y, Noguchi Y, Miyayama M, Kagawa Y, Moriyoshi C, Kuroiwa Y 2013 Ferroelectrics 443 1 Google Scholar
[44]	Moriyoshi C, Hiramoto S, Ohkubo H, Kuroiwa Y, Osawa H, Sugimoto K, Kimura S, Takata M, Kitanaka Y, Noguchi Y, Miyayama M 2011 Jpn. J. Appl. Phys. 50 09NE05 Google Scholar
[45]	Gorfman S, Keeble D S, Glazer A M, Long X, Xie Y, Ye Z G, Collins S, Thomas P A 2011 Phys. Rev. B 84 020102 Google Scholar
[46]	Gorfman S, Thomas P A 2010 J. Appl. Crystallogr. 43 1409 Google Scholar
[47]	Datta K, Gorfman S, Thomas P A 2009 Appl. Phys. Lett. 95 251901 Google Scholar
[48]	Daymond M R 2004 J. Appl. Phys. 96 4263 Google Scholar
[49]	Pramanick A, Damjanovic D, Daniels J E, Nino J C, Jones J L 2011 J. Am. Ceram. Soc. 94 293 Google Scholar
[50]	Ehmke M C, Khansur N H, Daniels J E, Blendell J E, Bowman K J 2014 Acta Mater. 66 340 Google Scholar
[51]	Jones J L, Slamovich E B, Bowman K J 2005 J. Appl. Phys. 97 034113 Google Scholar
[52]	Jones J L, Hoffman M, Bowman K J 2005 J. Appl. Phys. 98 024115 Google Scholar
[53]	Kungl H, Theissmann R, Knapp M, Baehtz C, Fuess H, Wagner S, Fett T, Hoffmann M J 2007 Acta Mater. 55 1849 Google Scholar
[54]	Hall D A, Steuwer A, Cherdhirunkorn B, Mori T, Withers P J 2004 J. Appl. Phys. 96 4245 Google Scholar
[55]	Fan L, Chen J, Ren Y, Pan Z, Zhang L, Xing X 2016 Phys. Rev. Lett. 116 027601 Google Scholar
[56]	Hinterstein M, Lee K Y, Esslinger S, Glaum J, Studer A J, Hoffman M, Hoffmann M J 2019 Phys. Rev. B 99 174107 Google Scholar
[57]	Matthies S, Lutteroti L, Wenk H R 1997 J. Appl. Crystallogr. 30 31 Google Scholar
[58]	Lutterotti L, Bortolotti M, Ischia G, Lonardelli I, Wenk H R 2007 Z. Kristallogr. Suppl. 26 125 Google Scholar
[59]	Hinterstein M, Hoelzel M, Rouquette J, Haines J, Glaum J, Kungl H, Hoffman M 2015 Acta Mater. 94 319 Google Scholar
[60]	Khansur N H, Hinterstein M, Wang Z, Groh C, Jo W, Daniels J E 2015 Appl. Phys. Lett. 107 242902 Google Scholar
[61]	Zhao C, Hou D, Chung C-C, Zhou H, Kynast A, Hennig E, Liu W, Li S, Jones J L 2018 Acta Mater. 158 369 Google Scholar
[62]	Fu J, Zuo R, Xu Y, Li J F, Shi M 2017 J. Eur. Ceram. Soc. 37 975 Google Scholar
[63]	Ochoa D A, Esteves G, Iamsasri T, Rubio-Marcos F, Fernández J F, García J E, Jones J L 2016 J. Eur. Ceram. Soc. 36 2489 Google Scholar
[64]	Zheng T, Wu H, Yuan Y, Lv X, Li Q, Men T, Zhao C, Xiao D, Wu J, Wang K, Li J F, Gu Y, Zhu J, Pennycook S J 2017 Energy Environ. Sci. 10 528 Google Scholar
[65]	Tutuncu G, Li B, Bowman K, Jones J L 2014 J. Appl. Phys. 115 144104 Google Scholar
[66]	Khansur N H, Rojac T, Damjanovic D, Reinhard C, Webber K G, Kimpton J A, Daniels J E 2015 J. Am. Ceram. Soc. 98 3884 Google Scholar
[67]	Li Y, Chen Y, Zhang Z, Kleppe A, Hall D A 2019 Acta Mater. 168 411 Google Scholar
[68]	Zuo R, Qi H, Fu J, Li J F, Li L 2017 Appl. Phys. Lett. 111 132901 Google Scholar
[69]	Hu C, Meng X, Zhang M H, Tian H, Daniels J E, Tan P, Huang F, Li L, Wang K, Li J F, Lu Q, Cao W, Zhou Z 2020 Sci. Adv. 6 eaay5979 Google Scholar
[70]	Zhang N, Gorfman S, Choe H, Vergentev T, Dyadkin V, Yokota H, Chernyshov D, Wang B, Glazer A M, Ren W, Ye Z G 2018 J. Appl. Crystallogr. 51 1396 Google Scholar
[71]	Hinterstein M, Knapp M, Hölzel M, Jo W, Cervellino A, Ehrenberg H, Fuess H 2010 J. Appl. Crystallogr. 43 1314 Google Scholar
[72]	Kling J, Tan X, Jo W, Kleebe H J, Fuess H, Rödel J 2010 J. Am. Ceram. Soc. 93 2452 Google Scholar
[73]	Daniels J E, Jo W, Rödel J, Honkimäki V, Jones J L 2010 Acta Mater. 58 2103 Google Scholar
[74]	Durbin M K, Jacobs E W, Hicks J C, Park S E 1999 Appl. Phys. Lett. 74 2848 Google Scholar
[75]	Damjanovic D 2005 J. Am. Ceram. Soc. 88 2663 Google Scholar
[76]	Noheda B, Cox D E, Shirane G, Park S E, Cross L E, Zhong Z 2001 Phys. Rev. Lett. 86 3891 Google Scholar
[77]	Fu J, Zuo R, Gao X 2013 Appl. Phys. Lett. 103 182907 Google Scholar
[78]	Li P, Zhai J, Shen B, Zhang S, Li X, Zhu F, Zhang X 2018 Adv. Mater. 30 1705171 Google Scholar
[79]	Yao F Z, Wang K, Jo W, Webber K G, Comyn T P, Ding J X, Xu B, Cheng L Q, Zheng M P, Hou Y D, Li J F 2016 Adv. Funct. Mater. 26 1217 Google Scholar
[80]	Simons H, Daniels J E, Glaum J, Studer A J, Jones J L, Hoffman M 2013 Appl. Phys. Lett. 102 062902 Google Scholar
[81]	Ren P, Liu Z, Liu H, Sun S, Wan Y, Long C, Shi J, Chen J, Zhao G 2019 J. Eur. Ceram. Soc. 39 994 Google Scholar
[82]	Wang G, Fan Z, Murakami S, Lu Z, Hall D A, Sinclair D C, Feteira A, Tan X, Jones J L, Kleppe A K, Wang D, Reaney I M 2019 J. Mater. Chem. A 7 21254 Google Scholar
[83]	Xu G, Zhong Z, Bing Y, Ye Z G, Shirane G 2006 Nat. Mater. 5 134 Google Scholar
[84]	Xu G, Wen J, Stock C, Gehring P M 2008 Nat. Mater. 7 562 Google Scholar
[85]	Paściak M, Welberry T R, Kulda J, Kempa M, Hlinka J 2012 Phys. Rev. B 85 224109 Google Scholar
[86]	Li F, Zhang S, Yang T, Xu Z, Zhang N, Liu G, Wang J, Wang J, Cheng Z, Ye Z G, Luo J, Shrout T R, Chen L Q 2016 Nat. Commun. 7 13807 Google Scholar
[87]	Polinger V, Bersuker I B 2018 Phys. Rev. B 98 214102 Google Scholar
[88]	Bokov A A, Ye Z G 2006 J. Mater. Sci. 41 31 Google Scholar
[89]	Xu G, Zhong Z, Hiraka H, Shirane G 2004 Phys. Rev. B 70 174109 Google Scholar
[90]	Welberry T R 2004 Diffuse X-Ray Scattering and Models of Disorder (New York: Oxford University Press) pp4−20

[1]	郑鹏飞, 柳志旭, 王超, 刘卫芳. 基团替代调控无铅有机钙钛矿铁电体的极化和压电特性的第一性原理研究. , 2024, 73(12): 126202. doi: 10.7498/aps.73.20240385
[2]	黄浩, 张侃, 吴明, 李虎, 王敏涓, 张书铭, 陈建宏, 文懋. SiC纤维增强Ti17合金复合材料轴向残余应力的拉曼光谱和X射线衍射法对比研究. , 2018, 67(19): 197203. doi: 10.7498/aps.67.20181157
[3]	李晓东, 李晖, 李鹏善. 同步辐射高压单晶衍射实验技术. , 2017, 66(3): 036203. doi: 10.7498/aps.66.036203
[4]	李佳, 房奇, 罗炳池, 周民杰, 李恺, 吴卫东. Be薄膜应力的X射线掠入射侧倾法分析. , 2013, 62(14): 140701. doi: 10.7498/aps.62.140701
[5]	韩亮, 刘德连, 陈仙, 赵玉清. 氮化铬过渡层对四面体非晶碳薄膜在高速钢基底上附着特性影响的研究. , 2013, 62(9): 096802. doi: 10.7498/aps.62.096802
[6]	徐晓明, 苗伟, 陶琨. X射线衍射多相谱中某一物相点阵参数的直接求解方法. , 2011, 60(8): 086101. doi: 10.7498/aps.60.086101
[7]	曹功勋, 张晓青, 孙转兰, 王学文, 娄可行, 夏钟福. 人工调控微结构压电驻极体的热稳定性和电荷动态特性. , 2010, 59(9): 6514-6520. doi: 10.7498/aps.59.6514
[8]	孙转兰, 张晓青, 曹功勋, 王学文, 夏钟福. 有序结构氟聚合物压电驻极体的制备和压电性研究. , 2010, 59(7): 5061-5066. doi: 10.7498/aps.59.5061
[9]	李永华, 刘常升, 孟繁玲, 王煜明, 郑伟涛. NiTi合金薄膜厚度对相变温度影响的X射线光电子能谱分析. , 2009, 58(4): 2742-2745. doi: 10.7498/aps.58.2742
[10]	张晓青, 黄金峰, 王学文, 夏钟福. 聚四氟乙烯和氟化乙丙烯共聚物复合膜的压电性. , 2009, 58(5): 3525-3531. doi: 10.7498/aps.58.3525
[11]	李洪涛, 罗毅, 席光义, 汪莱, 江洋, 赵维, 韩彦军, 郝智彪, 孙长征. 基于X射线衍射的GaN薄膜厚度的精确测量. , 2008, 57(11): 7119-7125. doi: 10.7498/aps.57.7119
[12]	明保全, 王矜奉, 臧国忠, 王春明, 盖志刚, 杜鹃, 郑立梅. 铌酸钾钠基无铅压电陶瓷的X射线衍射与相变分析. , 2008, 57(9): 5962-5967. doi: 10.7498/aps.57.5962
[13]	谈国太, 陈正豪. La1-xTexMnO3晶格结构的X射线粉末衍射分析. , 2007, 56(3): 1702-1706. doi: 10.7498/aps.56.1702
[14]	钦佩, 娄豫皖, 杨传铮, 夏保佳. 分离X射线衍射线多重宽化效应的新方法和计算程序. , 2006, 55(3): 1325-1335. doi: 10.7498/aps.55.1325
[15]	张鹏锋, 夏钟福, 邱勋林, 王飞鹏, 吴贤勇. 充电参数对聚丙烯蜂窝膜驻极体压电性的影响. , 2006, 55(2): 904-909. doi: 10.7498/aps.55.904
[16]	邱勋林, 夏钟福, 安振连, 吴贤勇. 热膨胀处理的聚丙烯蜂窝膜驻极体的压电性. , 2005, 54(1): 402-406. doi: 10.7498/aps.54.402
[17]	张鹏锋, 夏钟福, 邱勋林, 吴贤勇. 聚丙烯蜂窝膜驻极体压电系数的测量及压电性的改善. , 2005, 54(1): 397-401. doi: 10.7498/aps.54.397
[18]	张晓丹, 赵颖, 高艳涛, 朱锋, 魏长春, 孙建, 耿新华, 熊绍珍. 太阳电池用本征微晶硅材料的制备及其结构研究. , 2005, 54(10): 4874-4878. doi: 10.7498/aps.54.4874
[19]	杜晓松, S. Hak, O. C. Rogojanu, T. Hibma. 氧化铬外延薄膜的x射线研究. , 2004, 53(10): 3510-3514. doi: 10.7498/aps.53.3510
[20]	夏钟福, 马珊珊, 朱伽倩, 邱勋林, 张冶文, Reimund Gerhard-Multhaupt, Wolfgang Kuenstler. 聚四氟乙烯多孔膜的压电活性及其稳定性. , 2003, 52(8): 2075-2080. doi: 10.7498/aps.52.2075

计量

文章访问数: 11134
PDF下载量: 384
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

利用X射线衍射技术对压电材料本征与非本征起源探究的研究进展