-
超短超强激光脉冲在气体等离子体中激发的尾波场加速在过去40年里有了长足的发展, 人们已经在厘米加速距离内获得了数GeV的准单能电子加速, 激光尾波加速的最高电子能量已经达到8 GeV. 为了进一步提升加速电子束的稳定性和品质, 多种电子注入方式先后被提出. 本文研究了基于锐真空-等离子体边界面的密度跃变注入, 着重讨论了不同角度的倾斜边界面对注入电子品质的影响. 二维粒子模拟研究表明, 与倾角为0°的垂直边界面相比, 在合适的倾斜边界角下, 第二个尾波空泡内产生的注入电量可以有近三倍的提升, 同时偏振方向与入射面平行的驱动激光可以增加第一个空泡内注入电子的电量. 根据不同激光入射角度时尾波场中电子自注入的起始位置差异, 分析了电子电量与横向振荡增强的原因. 这些研究有利于提升基于Betatron运动的尾波场辐射及其应用.
-
关键词:
- 激光等离子体相互作用 /
- 激光等离子体尾波加速 /
- 电子注入
Plasma wakefield acceleration driven by ultra short ultra intense laser pulse interacting with gas target has been studied for almost four decades. Monoenergetic electron beams with central energy of multi-giga electron-volt have been achieved in a centimeter-scale acceleration distance. Currently, the highest energy of electrons accelerated by laser wakefield is 8 GeV. In order to further improve the quality of such electrons, many kinds of electron injection schemes have been proposed such as density gradient injection, colliding pulse injection and ionization injection. Electrons under the suitable conditions can be trapped by the strong plasma wakefield. Those trapped electrons are then accelerated in the wakefield. In a nonlinear regime, the wakefield shows a “bubble” structure. Electrons with transverse momentum can oscillate in the wakefield and produce considerably betatron radiation in the ultraviolet and X-ray region. In this paper, we study the electron injection around the sharp plasma-vacuum boundary. The effects of the slant angle of the boundary on the final electron quality are investigated in detail. Our results show that with optimal slant density transition around the vacuum plasma boundary, both the beam quality and the injection charge in the second “bubble” can be improved. Two-dimensional particle-in-cell simulations show that the injection charge in the second wake bucket can be increased three times when an optimal slant angle is used compared with a vertical boundary. The driving pulse’s polarization also affects the injection charge. When the polarization is in the injection plane the injection charge in the first bucket can be triply increased compared with the case when the polarization is out of the plane. The reasons for the enhanced injection charge and transverse oscillation are found by tracing the initial injection positions and trajectories of the electrons. These studies would benefit the electron acceleration and its applications, such as compact betatron radiation source.[1] Tajima T, Dawson J M 1979 Phys. Rev. Lett. 43 267
Google Scholar
[2] Esarey E, Schroeder C B, Leemans W P 2009 Rev. Mod. Phys. 81 1229
Google Scholar
[3] Gonsalves A J, Nakamura K, Daniels J, Benedetti C, Pieronek C, Raadt de T C H, Steinke S, Bin J H, Bulanov S S, Tilborg J van, Geddes C G R, Schroeder C B, Tóth Cs, Esarey E, Swanson K, Fan Chiang L, Bagdasarov G, Bobrova N, Gasilov V, Korn G, Sasorov P, Leemans W P 2019 Phys. Rev. Lett. 122 084801
Google Scholar
[4] Ma Y, Chen L M, Li M H, Li Y F, Wang J G, Tao M Z, Han Y J, Zhao J R, Huang K, Yan W C, Li D Z, Chen Z Y, Ma J L, Li Y T, Sheng Z M, Zhang J 2015 Phys. Plasmas 22 083102
Google Scholar
[5] Wang X, Zgadzaj R, Fazel N, Li Z Y, Yi S A, Zhang X, Henderson Watson, Chang Y Y, Korzekwa R, Tsai H E, Pai C H, Quevedo H, Dyer G, Gaul E, Martinez M, Bernstein A C, Borger T, Spinks M, Donovan M, Khudik V, Shvets G, Ditmire T, Downe M C 2013 Nat. Commun. 4 1988
Google Scholar
[6] Mo M Z, Ali A, Fourmaux S, Lassonde P, Kieffer J C, Fedosejevs R 2012 Appl. Phys. Lett. 100 074101
Google Scholar
[7] Geddes C G R, Nakamura K, Plateau G R, Toth Cs, Cormier-Michel E, Esarey E, Schroeder C B, Cary J R, Leemans W P 2008 Phys. Rev. Lett. 100 215004
Google Scholar
[8] Gonsalves A J, Nakamura K, Lin C, Panasenko D, Shiraishi S, Sokollik T, Benedetti C, Schroeder C B, Geddes C G R, Tilborg J van, Osterhoff J, Esarey E, Toth C, Leemans W P 2011 Nat. Phys. 7 862
Google Scholar
[9] Faure J, Rechatin C, Norlin A, Lifschitz A, Glinec Y, Malka V 2006 Nature 444 737
Google Scholar
[10] Chen M, Sheng Z M, Ma Y Y, Zhang J 2006 J. Appl. Phys. 99 056109
Google Scholar
[11] Pak A, Marsh K A, Martins S F, Lu W, Mori W B, Joshi C 2010 Phys. Rev. Lett. 104 025003
Google Scholar
[12] Liu J S, Xia C Q, Wang W T, Lu H Y, Wang C, Deng A H, Li W T, Zhang H, Liang X Y, Leng Y X, Lu X M, Wang C, Wang J Z, Nakajima K, Li R X, Xu Z Z 2011 Phys. Rev. Lett. 107 035001
Google Scholar
[13] Yu L L, Esarey E, Schroeder C B, Vay J L, Benedetti C, Geddes C G R, Chen M, Leemans W P 2014 Phys. Rev. Lett. 112 125001
Google Scholar
[14] Zeng M, Chen M, Yu L L, Mori W B, Sheng Z M, Hidding B, Jaroszynski D A, Zhang J 2015 Phys. Rev. Lett. 114 084801
Google Scholar
[15] Mirzaie M, Li S, Zeng M, Hafz N A M, Chen M, Li G Y, Zhu Q J, Liao H, Sokollik T, Liu F, Ma Y Y, Chen L M, Sheng Z M, Zhang J 2015 Sci. Rep. 5 14659
Google Scholar
[16] Xu X L, Pai C H, Zhang J C, Li F, Wan Y, Wu Y P, Hua J F, Lu W, An W, Yu P, Joshi C, Mori W B 2016 Phys. Rev. Lett. 117 034801
Google Scholar
[17] Schmid K, Veisz L, Tavella F, Benavides S, Tautz R, Herrmann D, Buck A, Hidding B, Marcinkevicius A, Schramm U, Geissler M, Meyer-ter-Vehn J, Habs D, Krausz F 2009 Phys. Rev. Lett. 102 124801
Google Scholar
[18] Corde S, Ta Phuoc K, Lambert G, Fitour R, Malka V, Rousse A, Beck A, Lefebvre E 2013 Rev. Mod. Phys. 85 1
Google Scholar
[19] Chen M, Luo J, Li F Y, Liu F, Sheng Z M, Zhang J 2016 Light-Sci. Appl. 5 e16015
Google Scholar
[20] Fonseca R 2002 Proceedings of the Second International Conference on Computational Science—ICCS Amsterdam, Netherlands, April 21−24, 2002 p342
[21] Chien T Y, Chang C L, Lee C H, Lin J Y, Wang J, Chen S Y 2005 Phys. Rev. Lett. 94 115003
Google Scholar
[22] Fourmaux S, Ta Phuoc K, Lassonde P, Corde S, Lebrun G, Malka V, Rousse A, Kieffer 2012 Appl. Phys. Lett. 101 111106
[23] Tomassini P, Galimberti M, Giulietti A, Giulietti D, Gizzi L.A, Labate L 2004 Laser. Particle. Beams. 22 423
Google Scholar
[24] Hu R, Lu H, Shou Y, Hu R H, Lu H Y, Shou Y R, Lin C, Zhuo H B, Chen C, Yan X Q 2016 Phys. Rev. Accel. Beams 19 091301
Google Scholar
[25] Geindre J P, Marjoribanks R S, Audebert P 2010 Phys. Rev. Lett. 104 135001
Google Scholar
[26] Brunel F 1987 Phys. Rev. Lett. 59 52
Google Scholar
[27] Steinke S, Tilborg J van, Benedetti1 C, Geddes C G R, Schroeder C B, Daniels J, Swanson K K, Gonsalves A J, Nakamura K, Matlis N H, Shaw B H, Esarey E, Leemans W P 2016 Nature 530 190
Google Scholar
[28] Luo J, Chen M, Wu W Y, Weng S M, Sheng Z M, Schroeder C B, Jaroszynski D A, Esarey E, Leemans W P, Mori W B, Zhang J 2018 Phys. Rev. Lett. 120 154801
Google Scholar
[29] Lemos N, Lopes N, Dias J M 2009 Rev. Sci. Instrum. 80 103301
Google Scholar
[30] Kim G H, Kim C, Hafz N, Kim J U, Lee H J, Suk H 2003 30th International Conference on Plasma Science Jeju, South Korea, June 2−5, 2003 p364
-
图 1 真空等离子体边界激光尾波电子注入示意图
Fig. 1. Schematic of vacuum-plasma boundary injection in laser wakefield acceleration
图 2 倾斜角为0°时激光传播500T0后等离子体密度与注入电子(γ ≥ 15)的位置分布 (a)注入电子的分布; (b)放大后第二个空泡中的电子分布
Fig. 2. Distributions of plasma density and injected electrons after 500T0 propagation when the boundary slant angle is 0°: (a) Injected electrons; (b) electrons in the second bubble
图 3 激光传播过程中不同角度下的电子平均能量增长的情况 (a)第一个空泡内电子平均相对论因子γ的变化; (b) 第二个空泡内电子平均相对论因子γ的变化
Fig. 3. Average energy growth with time: (a) Average gamma factor of electrons in the first bubble; (b) average gamma factor of electrons in the second bubble
图 4 不同角度下电子注入后经过相同加速距离后的加速情况对比 (a)被加速电子的平均能量; (b)被加速电子的总电量(单位归一化到 pC/μm)
Fig. 4. Electrons statistics after same acceleration length: (a) Average energy; (b) total acceleration charge (normalized to pC/μm).
图 5 不同倾斜角度下的第一个空泡(红色)和第二个空泡(蓝色)中注入电子的轨迹(为了显示清晰, 对两种倾角情形, 各自只选取了10个典型的电子) (a) 0°; (b) 45°
Fig. 5. Trajectories of electrons in the first bubble (red) and second bubble (blue): (a) 0°; (b) 45°. Ten electrons’ trajectories have been selected for clearer view
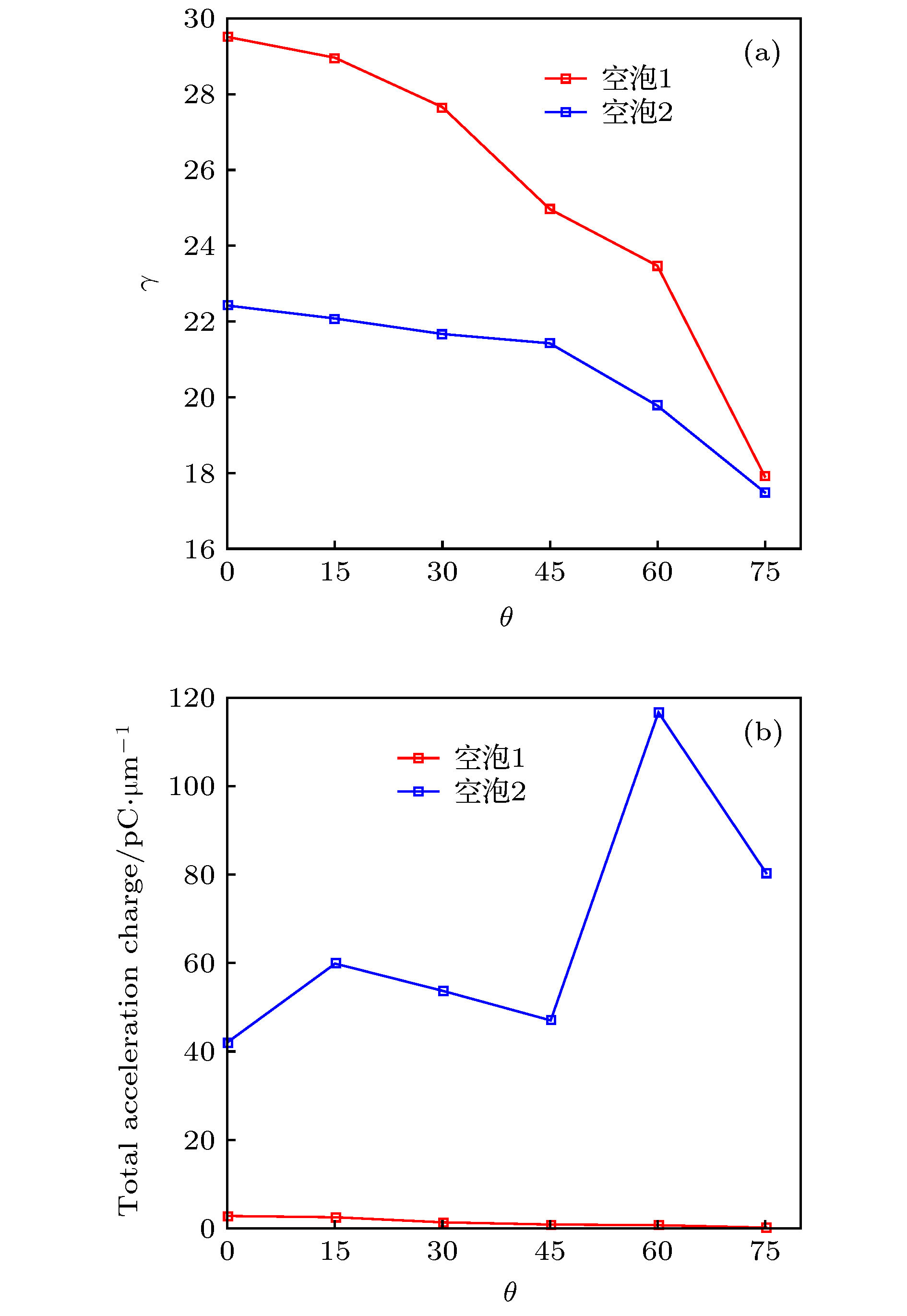
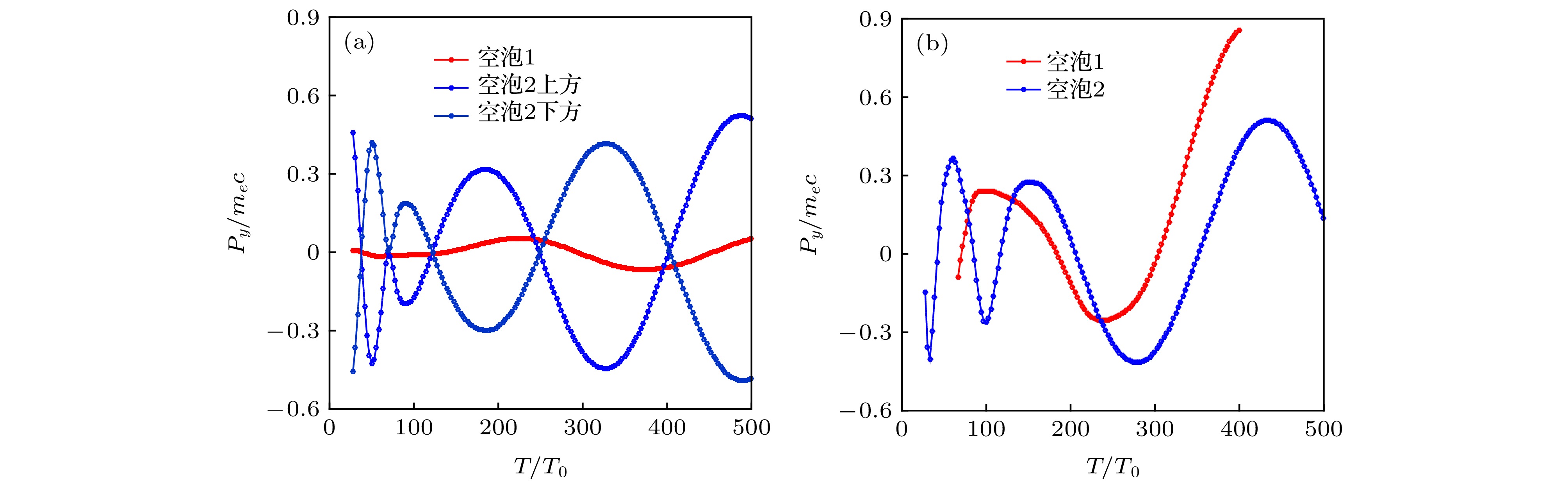
图 6 (a) 0°倾斜边界角时注入空泡1 (红)和空泡2上下两侧注入电子(蓝)的平均动量; (b) 45°倾斜边界角时注入空泡1和空泡2电子的平均动量
Fig. 6. (a) Average transverse momentum of electrons in the first bubble (red) and second bubble (blue) when the boundary slant angle is 0°; (b) average transverse momentum of electrons in the first bubble (red) and second bubble (blue) when the boundary slant angle is 45°
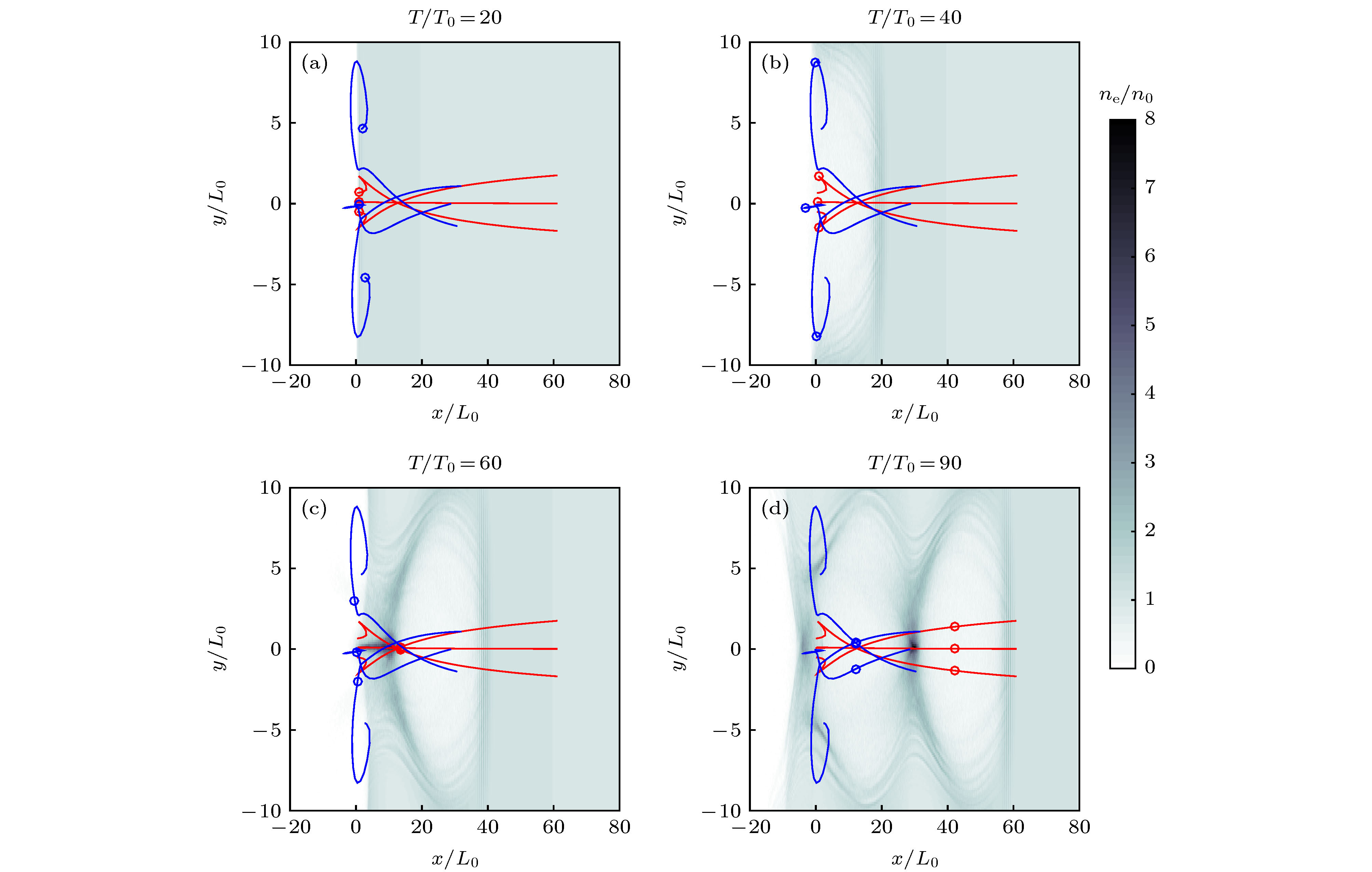
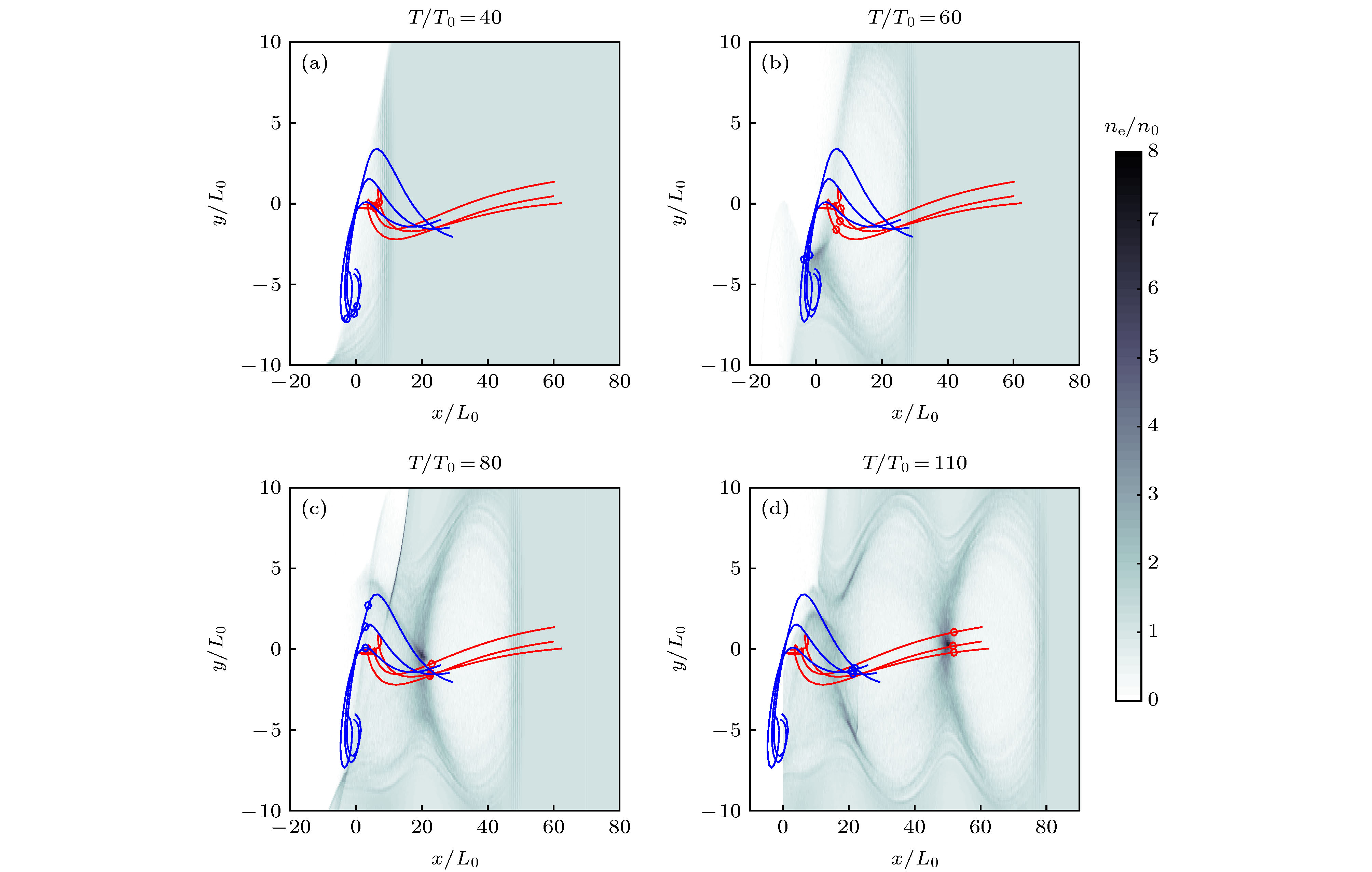
图 7 倾斜边界角为0°时的电子注入过程(等横向间距选取了3个粒子作为示意, 背景为等离子体密度, 线条代表粒子的真实轨迹, 圆圈代表粒子在该时刻的位置) (a) T/T0 = 20; (b) T/T0 = 40; (c) T/T0 = 60; (d) T/T0 = 90
Fig. 7. Electrons’ injection trajectories when the boundary slant angle is 0°: (a) T/T0 = 20; (b) T/T0 = 40; (c) T/T0 = 60; (d) T/T0 = 90. Here the background color bar represents the plasma density, we have selected 3 particles with equally separation along the transverse direction. The blue and red lines represent the injection trajectories and the circles represent the particles’ positions at that time
图 8 45°入射下边界面产生电子注入的过程 (a) T/T0 = 40; (b) T/T0 = 60; (c) T/T0 = 80; (d) T/T0 = 110
Fig. 8. Electrons injection at 45° incidence: (a) T/T0 = 40; (b) T/T0 = 60; (c) T/T0 = 80; (d) T/T0 = 110
图 9 (a)倾斜边界为0°情况下第一个空泡和第二个空泡内注入电子的起始位置; (b) 倾斜边界为45°入射下第一个空泡和第二个空泡内注入电子的起始位置
Fig. 9. (a) Original positions of the trapped electrons when the boundary slant angle is 0°; (b) original positions of the trapped electrons when the boundary slant angle is 45°
表 1 S偏振和P偏振激光45°入射时注入空泡中的电子电量
Table 1. Injection charge of S-polarization and P-polarization incidence at 45°.
电量/pC·μm–1 S偏振 P偏振 第一个空泡 1.24 3.88 第二个空泡 47.21 47.00 
下载: 导出CSV
-
[1] Tajima T, Dawson J M 1979 Phys. Rev. Lett. 43 267
Google Scholar
[2] Esarey E, Schroeder C B, Leemans W P 2009 Rev. Mod. Phys. 81 1229
Google Scholar
[3] Gonsalves A J, Nakamura K, Daniels J, Benedetti C, Pieronek C, Raadt de T C H, Steinke S, Bin J H, Bulanov S S, Tilborg J van, Geddes C G R, Schroeder C B, Tóth Cs, Esarey E, Swanson K, Fan Chiang L, Bagdasarov G, Bobrova N, Gasilov V, Korn G, Sasorov P, Leemans W P 2019 Phys. Rev. Lett. 122 084801
Google Scholar
[4] Ma Y, Chen L M, Li M H, Li Y F, Wang J G, Tao M Z, Han Y J, Zhao J R, Huang K, Yan W C, Li D Z, Chen Z Y, Ma J L, Li Y T, Sheng Z M, Zhang J 2015 Phys. Plasmas 22 083102
Google Scholar
[5] Wang X, Zgadzaj R, Fazel N, Li Z Y, Yi S A, Zhang X, Henderson Watson, Chang Y Y, Korzekwa R, Tsai H E, Pai C H, Quevedo H, Dyer G, Gaul E, Martinez M, Bernstein A C, Borger T, Spinks M, Donovan M, Khudik V, Shvets G, Ditmire T, Downe M C 2013 Nat. Commun. 4 1988
Google Scholar
[6] Mo M Z, Ali A, Fourmaux S, Lassonde P, Kieffer J C, Fedosejevs R 2012 Appl. Phys. Lett. 100 074101
Google Scholar
[7] Geddes C G R, Nakamura K, Plateau G R, Toth Cs, Cormier-Michel E, Esarey E, Schroeder C B, Cary J R, Leemans W P 2008 Phys. Rev. Lett. 100 215004
Google Scholar
[8] Gonsalves A J, Nakamura K, Lin C, Panasenko D, Shiraishi S, Sokollik T, Benedetti C, Schroeder C B, Geddes C G R, Tilborg J van, Osterhoff J, Esarey E, Toth C, Leemans W P 2011 Nat. Phys. 7 862
Google Scholar
[9] Faure J, Rechatin C, Norlin A, Lifschitz A, Glinec Y, Malka V 2006 Nature 444 737
Google Scholar
[10] Chen M, Sheng Z M, Ma Y Y, Zhang J 2006 J. Appl. Phys. 99 056109
Google Scholar
[11] Pak A, Marsh K A, Martins S F, Lu W, Mori W B, Joshi C 2010 Phys. Rev. Lett. 104 025003
Google Scholar
[12] Liu J S, Xia C Q, Wang W T, Lu H Y, Wang C, Deng A H, Li W T, Zhang H, Liang X Y, Leng Y X, Lu X M, Wang C, Wang J Z, Nakajima K, Li R X, Xu Z Z 2011 Phys. Rev. Lett. 107 035001
Google Scholar
[13] Yu L L, Esarey E, Schroeder C B, Vay J L, Benedetti C, Geddes C G R, Chen M, Leemans W P 2014 Phys. Rev. Lett. 112 125001
Google Scholar
[14] Zeng M, Chen M, Yu L L, Mori W B, Sheng Z M, Hidding B, Jaroszynski D A, Zhang J 2015 Phys. Rev. Lett. 114 084801
Google Scholar
[15] Mirzaie M, Li S, Zeng M, Hafz N A M, Chen M, Li G Y, Zhu Q J, Liao H, Sokollik T, Liu F, Ma Y Y, Chen L M, Sheng Z M, Zhang J 2015 Sci. Rep. 5 14659
Google Scholar
[16] Xu X L, Pai C H, Zhang J C, Li F, Wan Y, Wu Y P, Hua J F, Lu W, An W, Yu P, Joshi C, Mori W B 2016 Phys. Rev. Lett. 117 034801
Google Scholar
[17] Schmid K, Veisz L, Tavella F, Benavides S, Tautz R, Herrmann D, Buck A, Hidding B, Marcinkevicius A, Schramm U, Geissler M, Meyer-ter-Vehn J, Habs D, Krausz F 2009 Phys. Rev. Lett. 102 124801
Google Scholar
[18] Corde S, Ta Phuoc K, Lambert G, Fitour R, Malka V, Rousse A, Beck A, Lefebvre E 2013 Rev. Mod. Phys. 85 1
Google Scholar
[19] Chen M, Luo J, Li F Y, Liu F, Sheng Z M, Zhang J 2016 Light-Sci. Appl. 5 e16015
Google Scholar
[20] Fonseca R 2002 Proceedings of the Second International Conference on Computational Science—ICCS Amsterdam, Netherlands, April 21−24, 2002 p342
[21] Chien T Y, Chang C L, Lee C H, Lin J Y, Wang J, Chen S Y 2005 Phys. Rev. Lett. 94 115003
Google Scholar
[22] Fourmaux S, Ta Phuoc K, Lassonde P, Corde S, Lebrun G, Malka V, Rousse A, Kieffer 2012 Appl. Phys. Lett. 101 111106
[23] Tomassini P, Galimberti M, Giulietti A, Giulietti D, Gizzi L.A, Labate L 2004 Laser. Particle. Beams. 22 423
Google Scholar
[24] Hu R, Lu H, Shou Y, Hu R H, Lu H Y, Shou Y R, Lin C, Zhuo H B, Chen C, Yan X Q 2016 Phys. Rev. Accel. Beams 19 091301
Google Scholar
[25] Geindre J P, Marjoribanks R S, Audebert P 2010 Phys. Rev. Lett. 104 135001
Google Scholar
[26] Brunel F 1987 Phys. Rev. Lett. 59 52
Google Scholar
[27] Steinke S, Tilborg J van, Benedetti1 C, Geddes C G R, Schroeder C B, Daniels J, Swanson K K, Gonsalves A J, Nakamura K, Matlis N H, Shaw B H, Esarey E, Leemans W P 2016 Nature 530 190
Google Scholar
[28] Luo J, Chen M, Wu W Y, Weng S M, Sheng Z M, Schroeder C B, Jaroszynski D A, Esarey E, Leemans W P, Mori W B, Zhang J 2018 Phys. Rev. Lett. 120 154801
Google Scholar
[29] Lemos N, Lopes N, Dias J M 2009 Rev. Sci. Instrum. 80 103301
Google Scholar
[30] Kim G H, Kim C, Hafz N, Kim J U, Lee H J, Suk H 2003 30th International Conference on Plasma Science Jeju, South Korea, June 2−5, 2003 p364
下载:
计量
- 文章访问数: 7896
- PDF下载量: 114
- 被引次数: 0
