电路中的拓扑态

罗开发; 余睿

doi:10.7498/aps.68.20191398

摘要

利用凝聚态物理中紧束缚哈密顿量与集中参数电子线路中基尔霍夫方程的对应关系, 可以在电子线路中设计出种类丰富的拓扑物态. 本文详细介绍用电路实现一维SSH模型、三维结线半金属模型和外尔半金属模型的设计方案. 在上述拓扑电路中可探测到端点态、表面鼓膜态、表面费米弧等体拓扑性质对应的界面态. 由于电子线路对应的紧束缚哈密顿量中的跃迁项具有丰富的调控自由度, 如强度、距离、维度等, 容易推广到非厄密系统以及四维或更高维度的系统, 使得人们能在电路中设计和验证传统凝聚态体系中难以实现或无法实现的新物态. 此外, 电子线路具备器件功能多样、制备工艺成熟可靠等优势, 为探索新奇物态提供了一个便利的实验平台.

关键词:

Abstract

Based on the correspondence between tight-binding Hamiltonian in condensed matter physics and the Kirchhoff’s current equations in lumped parameters circuits, profuse topological states can be mapped from the former to the latter. In this article, the electric-circuit realizations of 1D SSH model, 3D nodal-line and Weyl semimetals are devised and elaborated, in which the edge states, surface drum-head and Fermi-arc states are appearing on the surface of the circuit lattice. Of these circuits, the effective hopping terms in Hamiltonian have high degree of freedom. The hopping strength, distance and dimension are easy to tune, and therefore our design is convenient to be extended to non-Hermitian and four or higher dimensional cases, making the fancy states that hard to reach in conventional condensed matter now at our fingertips. Besides, the electric circuit has the advantage of plentiful functional elements and mature manufacture techniques, thus being a promising platform to explore exotic states of matter.

Keywords:

作者及机构信息

罗开发,
余睿

武汉大学, 物理科学与技术学院, 武汉　430072

通信作者: 余睿, yurui@whu.edu.cn

基金项目: 国家自然科学基金(批准号: 11874048)资助的课题

Authors and contacts

Luo Kai-Fa,
Yu Rui

School of Physics and Technology, Wuhan University, Wuhan 430072, China

Corresponding author: Yu Rui, yurui@whu.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant No. 11874048)

文章全文 : translate this paragraph

参考文献

[1]	Qi X L, Zhang S C 2011 Rev. Mod. Phys. 83 1057 Google Scholar
[2]	Hasan M Z, Kane C L 2010 Rev. Mod. Phys. 82 3045 Google Scholar
[3]	Chiu C K, Teo J C, Schnyder A P, Ryu S 2016 Rev. Mod. Phys. 88 035005 Google Scholar
[4]	Xu G, Weng H, Wang Z, Dai X, Fang Z 2011 Phys. Rev. Lett. 107 186806 Google Scholar
[5]	Wan X, Turner A M, Vishwanath A, et al. 2011 Phys. Rev. B 83 205101 Google Scholar
[6]	Burkov A 2018 Ann. Rev. Cond. Mat. Phys. 9 359 Google Scholar
[7]	Weng H M, Fang C, Fang Z, Bernevig B A, Dai X 2015 Phys. Rev. X 5 011029 Google Scholar
[8]	Lv B Q, Weng H M, Fu B B, Wang X P, et al. 2015 Phys. Rev. X 5 031013 Google Scholar
[9]	Xu S Y, Belopolski I, Alidoust N, et al. 2015 Science 349 613 Google Scholar
[10]	Weng H M, Yu R, Hu X, Dai X, Fang Z 2015 Adv. Phys. 64 227 Google Scholar
[11]	Armitage N P, Mele E J, Vishwanath A 2018 Rev. Mod. Phys. 90 015001 Google Scholar
[12]	Yu R, Fang Z, Dai X, Weng H M 2017 Fron. Phy 12 127202 Google Scholar
[13]	Fang C, Weng H M, Dai X, Fang Z 2016 Chinese Phys. B 25 117106 Google Scholar
[14]	Weng H M, Dai X, Fang Z 2016 J. Phys.: Cond. Mat. 28 303001 Google Scholar
[15]	Yan Z, Wang Z 2016 Phys. Rev. Lett. 117 087402 Google Scholar
[16]	Goldman N, Satija I, Nikolic P, et al. 2010 Phys. Rev. Lett. 105 255302 Google Scholar
[17]	Sun K, Liu W V, Hemmerich A 2012 Nature Phys. 8 67 Google Scholar
[18]	Jotzu G, Messer M, Desbuquois R, et al. 2014 Nature 515 237 Google Scholar
[19]	Aidelsburger M, Lohse M, Schweizer C, M. Atala, J. Barreiro, S. Nascimbene, N. Cooper, I. Bloch, N. Goldman 2015 Nature Phys. 11 162 Google Scholar
[20]	Dubcek T, Kennedy C J, L. Lu, W. Ketterle, M. Soljacic, H. Buljan 2015 Phys. Rev. Lett. 114 225301 Google Scholar
[21]	Xu Y, Zhang F, Zhang C 2015 Phys. Rev. Lett. 115 265304 Google Scholar
[22]	Raghu S, Haldane F D M 2008 Phys. Rev. A 78 033834 Google Scholar
[23]	Haldane F D M, Raghu S 2008 Phys. Rev. Lett. 100 013904 Google Scholar
[24]	Rechtsman M C, Zeuner J M, Plotnik Y, Y. Lumer, D. Podolsky, F. Dreisow, S. Nolte, M. Segev, A. Szameit 2013 Nature 496 196 Google Scholar
[25]	Lu L, Fu L, Joannopoulos J D, Soljacic M 2013 Nature Photon. 7 294 Google Scholar
[26]	Lu L, Wang Z D, Ran Y L, Fu L, Joannopoulos J D, Soljacic M 2015 Science 349 622 Google Scholar
[27]	Yan Q, Liu R, Yan Z, B. Liu, H. Chen, Z. Wang, L. Lu 2018 Nature Phys. 14 461 Google Scholar
[28]	Ozawa T, Price H M, Amo A, N. Goldman, M. Hafezi, L. Lu, M. C. Rechtsman, D. Schuster, J. Simon, O. Zilberberg, I. Carusotto 2019 Rev. Mod. Phys. 91 015006 Google Scholar
[29]	Wang P, Lu L, Bertoldi K 2015 Phys. Rev. Lett. 115 104302 Google Scholar
[30]	Po H C, Bahri Y, Vishwanath A 2016 Phys. Rev. B 93 205158 Google Scholar
[31]	Li F, Huang X, Lu J, J. Ma, Z. Liu 2018 Nature Phys. 14 30 Google Scholar
[32]	Xiao Y X, Ma G, Zhang Z Q, Chan C 2017 Phys. Rev. Lett. 118 166803 Google Scholar
[33]	Zhang T, Song Z, Alexandradinata A, H. Weng, C. Fang, L. Lu, Z. Fang 2018 Phys. Rev. Lett. 120 016401 Google Scholar
[34]	Wen X, Qiu C, Y. Qi, L. Ye, M. Ke, F. Zhang, Z. Liu 2019 Nature Phys. 15 352 Google Scholar
[35]	Kane C L, Lubensky T C 2013 Nature Phys. 10 39 Google Scholar
[36]	Gin-ge B, N. Upadhyaya, V. Vitelli 2014 Proc. Nat. Acad. Sci. 111 13004 Google Scholar
[37]	Paulose J, B. G.-g. Chen, V. Vitelli 2015 Nature Phys. 11 153 Google Scholar
[38]	Nash L M, Kleckner D, Read A, Vitelli V,, Turner W T M 2015 Proc. Nat. Acad. Sci. 112 14495 Google Scholar
[39]	Kariyado T, Y. Hatsugai 2015 Sci. Rep. 5 18107 Google Scholar
[40]	Susstrunk R, Huber S D 2015 Science 349 47 Google Scholar
[41]	Wang Y T, Luan P G, S. Zhang 2015 New J. Phys. 17 073031 Google Scholar
[42]	Susstrunk R, Huber S D 2016 Proc. Nat. Aca. Sci. 113 E4767 Google Scholar
[43]	Coulais C, Sounas D, Alu A 2017 Nature 542 461 Google Scholar
[44]	Takahashi Y, Kariyado T, Hatsugai Y 2017 New J. Phys. 19 035003 Google Scholar
[45]	Ronellenfitsch H, Dunkel J, arXiv: 1907.02054(2019).
[46]	Ma G, Xiao M, Chan C T 2019 Nature Rev. Phys. 1 281 Google Scholar
[47]	Ningyuan J, Owens C, Sommer A, D. Schuster, J. Simon 2015 Phys. Rev. X 5 021031 Google Scholar
[48]	Albert V V, L. I. Glazman, L. Jiang 2015 Phys. Rev. Lett. 114 173902 Google Scholar
[49]	Goren T, Plekhanov K, F. Appas, K. Le Hur 2018 Phys. Rev. B 97 041106 Google Scholar
[50]	Li Y, Sun Y, Zhu W, Z. Guo, J. Jiang, T. Kariyado, H. Chen, X. Hu, arXiv: 1801.04395 (2018).
[51]	Lee C H, Imhof S, Berger C, Bayer F, J. Brehm, L. W. Molenkamp, T. Kiessling, R. Thomale 2018 Commun. Phys. 1 39 Google Scholar
[52]	Luo K, Yu R, Weng H 2018 Research 2018 1 Google Scholar
[53]	Luo K, Feng J, Y. X. Zhao, R. Yu, arXiv: 1810.09231 (2018).
[54]	Kotwal T, Ronellenfitsch H, Moseley F, Dunkel J, arXiv: 1903.10130 (2019).
[55]	Lee C H, T. Hofmann, T. Helbig, Y. Liu, X. Zhang, M. Greiter, R. Thomale, arXiv: 1904.10183 (2019).
[56]	Yu R, Zhao Y X, A. P. Schnyder, arXiv: 1906.00883 (2019).
[57]	Lu Y, Jia N, L. Su, C. Owens, G. Juzeliūnas, D. I. Schuster, J. Simon 2019 Phys. Rev. B 99 020302 Google Scholar
[58]	Olekhno N A, Kretov E I, Stepanenko A A, Filonov D S, Yaroshenko V V, Cappello B, Matekovits L, Gorlach M A, arXiv: 1907.01016 (2019).
[59]	Liu S, Gao W, Q. Zhang, S. Ma, L. Zhang, C. Liu, Y. J. Xiang, T. J. Cui, S. Zhang 2019 Research 2019 1 Google Scholar
[60]	Serra-Garcia M, R. Süsstrunk, S. D. Huber 2019 Phys. Rev. B 99 020304 Google Scholar
[61]	Zhu W, Long Y, Chen H, Ren J 2019 Phys. Rev. B 99 115410 Google Scholar
[62]	Hofmann T, T. Helbig, C. H. Lee, M. Greiter, R. Thomale 2019 Phys. Rev. Lett. 122 247702 Google Scholar
[63]	Haenel R, Branch T, Franz M 2019 Phys. Rev. B 99 235110 Google Scholar
[64]	Imhof S, Berger C, Bayer F, Brehm J, et al. 2018 Nature Phys. 14 925 Google Scholar
[65]	Ezawa M 2018 Phys. Rev. B 98 201402 Google Scholar
[66]	Zhang Z Q, Wu B L, Song J, Jiang H, arXiv: 1906.04064 (2019).
[67]	Ezawa M 2019 arXiv: 1907.06911 (2019).
[68]	Nakata Y, Okada T, Nakanishi T, M. Kitano 2012 Phys. Status Solidi B 249 1 Google Scholar
[69]	Tzeng W J, Wu F Y 2006 J. Phys. A: Math. Gen. 39 8579 Google Scholar
[70]	Atala M, Aidelsburger M, Barreiro J T, Abanin D, T. Kitagawa, E. Demler, I. Bloch 2013 Nature Phys. 9 795 Google Scholar
[71]	Wang Q, Xiao M, Liu H, Zhu S, C. T. Chan 2016 Phys. Rev. B 93 041415 Google Scholar
[72]	Xiao M, Zhang Z, Chan C 2014 Phys. Rev. X 4 021017 Google Scholar
[73]	Xiao M, Ma G, Z. Yang, P. Sheng, Z. Q. Zhang, C. T. Chan 2015 Nature Phys. 11 240 Google Scholar
[74]	Wang L, Troyer M, Dai X 2013 Phys. Rev. Lett. 111 026802 Google Scholar
[75]	Bernevig A, Weng H M, Fang Z, Dai X 2018 J. Phys. Soc. Jpn. 87 041001 Google Scholar
[76]	Yu R, Weng H M, Fang Z, Dai X 2015 Phys. Rev. Lett. 115 036807 Google Scholar
[77]	Kim Y, Wieder B J, Kane C L, Rappe A M 2015 Phys. Rev. Lett. 115 036806 Google Scholar
[78]	Chiu C K, Schnyder A P 2014 Phys. Rev. B 90 205136 Google Scholar
[79]	Yu R, Wu Q, Fang Z, Weng H M 2017 Phys. Rev. Lett. 119 036401 Google Scholar
[80]	Fang C, Chen Y, H.-Y. Kee, L. Fu 2015 Phys. Rev. B 92 081201 Google Scholar
[81]	Bzdusek T, Wu Q, Ruegg A, Sigrist M, Soluyanov A A 2016 Nature 538 75 Google Scholar
[82]	Liang Q F, Zhou J, Yu R, Wang Z, Weng H M 2016 Phys. Rev. B 93 085427 Google Scholar
[83]	Heiss W D 2012 J. Phys. A: Math. Theor. 45 444016 Google Scholar
[84]	Zhou H, Peng C, Yoon Y, Hsu C W, Nelson K A, Fu L, Joannopoulos J D, Soljacic M, Zhen B 2018 Science 359 1009 Google Scholar
[85]	Lee T E 2016 Phys. Rev. Lett. 116 133903 Google Scholar
[86]	Yao S, Wang Z 2018 Phys. Rev. Lett. 121 086803 Google Scholar
[87]	Xiao L, Deng T, Wang K, Zhu G, Wang Z, Yi W, Xue P 2019 arXiv: 1907.12566
[88]	Hofmann T, Helbig T, Schindler F, Salgo N, Brzezinska M, M. Greiter, T. Kiessling, D. Wolf, A. Vollhardt, A. Kabasi, C. H. Lee, A. Bilusic, R. Thomale, T. Neupert 2019 arXiv: 1908.02759
[89]	Helbig T, Hofmann T, Imhof S, Abdelghany M, Kiessling T, Molenkamp L W, Lee C H, Szameit A, Greiter M, Thomale R 2019 arXiv: 1907.11562.
[90]	Chen R, Chen C Z, Gao J H, Zhou B, Xu D H 2019 arXiv: 1904.09932
[91]	Mitchell N P, Nash L M, Hexner D, Turner A M, Irvine W T M 2018 Nature Phys. 14 380 Google Scholar

施引文献

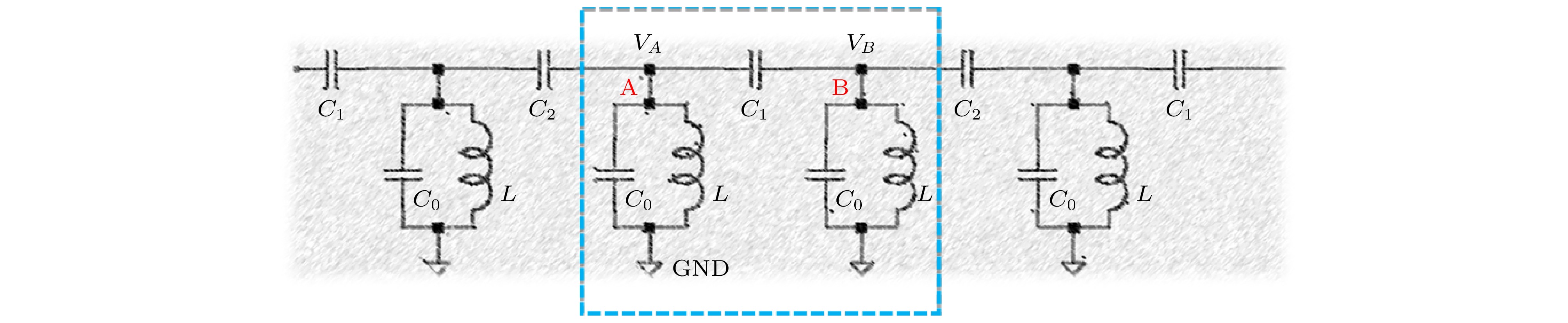
图 1 一维SSH电路. 原胞(蓝色虚线框)内有A和B两个不等价节点, 经并联的电感L和电容$C_{0}$接地. 原胞内节点由电容$C_1$相连, 原胞间节点由电容$C_{2}$相连

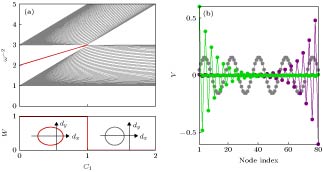
Fig. 1. The 1 D LC chain, in which a unit cell containing two inequivalent nodes A and B labeled by a dashed blue box. Each node A or B is grounded through a parallel connected inductor L and capacitor $C_{0}$. All nodes are connected by $C_{1}$ and $C_{2}$ alternatively.

下载: 全尺寸图片幻灯片

图 2 (a) 上: 电容$C_{1}$从0逐渐增加至超过$C_{2}$, 频谱中两个端点态(红线)在能$C_1=C_2$处消失, 表明系统发生了拓扑相变. 下: 系统缠绕数从1到0的跃变与端点态的消失临界值一致. $C_{1}/C_{2}=0.5$时系统的等效极化矢量在$d_{x}-d_{y}$平面上随动量参数k从0连续变到$2{\text{π}}$时绕原点一周. 红圈对应缠绕数为1; $C_{1}/C_{2}=1.5$时$(d_{x}, d_{y})$绕原点0圈. 黑色圈对应缠绕数为0. (b) 取$C_{1}/C_{2}=0.8$, 端点态(绿色和紫色)以及一个随机挑选的体态(灰色)对应的电势分布V

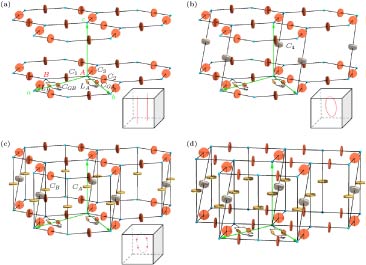
Fig. 2. (a) Upper: increase the parameter of $C_{1}$ from zero to exceed $C_{2}$, the end states (red) converge into the bulk states, indicating the topological transition. Bottom: the transition of winding number is consistent with the appearance and absence of end states. The effective polarization vector $(d_{x}, d_{y})$ winds the original a round when the momentum varies continuously from 0 to $2{\text{π}}$ for $C_{1}=0.5$(left), while zero round for $C_{1}=1.5$(right). (b) The electric potential distributions of two end states (green and puple) and a randomly selected bulk state (grey).

下载: 全尺寸图片幻灯片

图 3 三维LC电路示意图. 　(a) 单层LC蜂窝电路沿c方向上无连接的堆叠起来, 每个原胞内的两个不等价节点A和B在层内由$C_{1, 2, 3}$连接. 每个节点$A(B)$都通过并联的$L_{A}(L_{B})$和$C_{GA}(C_{GB})$回路接地. a, b和c表示格矢. 小图: 单层LC电路频谱中包含两个简并点, 沿着${k}_{c}$方向扩展将在布里渊区中形成两条直线状的结线(红线). (b) 电容$C_{4}$连接最近邻层间的节点A和B, 结线在给定合适的$C_{4}$时将弯曲成闭合环形. (c) 电容$C_{A}(C_{B})$连接最近邻层间的A-A(B-B)节点对, $C_{A}\neq C_{B}$且$C_{GA}\neq C_{GB}$时空间反演对称破缺, 环状结线可能退化成离散的外尔点. 此外, LC网络可以变形成(d), 简化实验装置的同时保证频谱不变

Fig. 3. Schematic setup of the 3 D LC circuit lattice. (a) LC honeycomb layers stacked along c-direction without any interlayer connection. Two inequivalent nodes A and B within a unit cell, linked by capacitors $C_{1, 2, 3}$. Each node A(B) is grounded through the parallel connected inductor $L_{A}$($L_{B}$) and capacitor $C_{GA}$($C_{GB}$). a, b, and c denote lattice vectors. Inset: spectra of a single layer LC lattice includes two band-crossing points, extended uniformly along ${k}_{c}$-direction and form two straight nodal lines (red) in the BZ. (b) Connecting nodes A and B between the nearest neighbor-layers with $C_{4}$. The straight lines could be curved to a closed ring given appropriate $C_{4}$. (c) Connect node-pairs A-A(B-B) between the nearest neighbor-layers with $C_{A}$($C_{B}$), to break space inversion symmetry by setting $C_{A}\neq C_{B}$ and $C_{GA}\neq C_{GB}$, and the nodal ring may be degenerated to discrete Weyl points. The LC network can be deformed into (d), which is convenient to construct circuit elements in experiments while spectrum invariant.

下载: 全尺寸图片幻灯片

图 4 (a) 结线(红色)及其在(001), (010)和(100) 面上的投影(灰色). 此时参数取为$ C_{1}=1~{\rm mF}, C_{2}=2~{\rm mF}, C_{3}=1~{\rm mF}, $$ C_{4}=0.833~{\rm mF}, C_{GA}=C_{GB}=1~{\rm mF} $和$L_{A}=L_{B}=1~{\rm mH}$. (b) 沿Γ-M-A-Y-B-M-Γ路径的频谱, 其中A和B是(a)中结线与$k_{c}=0$平面的交点. 上: (001) 方向的体态(灰色)及表面态(红线), 以及周期边界条件下的能带(紫色). 下: 积分路径在结线内部(外部)的贝里相位$\theta_{k_{\parallel}}$等于拓扑非平庸的π(平庸的0)

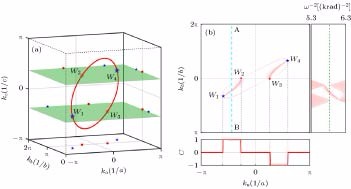
Fig. 4. (a) Nodal line (red) and its projections (grey) on the (001), (010), and (100) planes. The parameters are set as $ C_{1}=1 ~{\rm mF}, C_{2}=2 ~{\rm mF}, C_{3}=1~{\rm mF}, C_{4}=0.833~{\rm mF}, C_{GA}=C_{GB}=1 ~{\rm mF}$, and $L_{A}=L_{B}=1~{\rm mH}$. (b) Bands along Γ-M-A-Y-B-M-Γ, where A and B are two points with $k_{c}=0$ on the nodal line as labeled in (a). Upper: the bulk bands(grey) with the drumhead-like surface states nestled inside the projection of the nodal ring (red) on the (001) surface and the two bands (purple) in periodic boundary condition. Bottom: the Berry phase $\theta_{k_{\parallel}}$ equals π(0) inside (outside) the nodal ring.

下载: 全尺寸图片幻灯片

图 5 (a) 布里渊区中的四个外尔点及它们在(001), (010)和(100)方向的投影. 取$C_{A}=0.2~{\rm mF}$, $C_{B}=0.01~{\rm mF}$和$C_{GA}= 0.77~{\rm mF}$, 其它参数均与图4中相同. 外尔点是由$d_{1, 2}(k)=0$决定的结线与$d_{3}({k})=0$决定的两平面的交点, 它们的手性用蓝色五角星($\chi=+1$)和红色圆点($\chi=-1$)标记. (b) 左上: 在 (001) 表面上, 费米弧连接了手性相反外尔点的投影点. 右上: 开边界时路径AB上(亮青色虚线)的无能隙表面态, 绿色虚线标记外尔点所在频率. 左下: 垂直于$k_{a}$的各平面上的陈数. 沿$k_{a}$方向移动, 经过正(负)手性外尔点时平面上的陈数会增加1(减少1)

Fig. 5. (a) Four Weyl points in the Brillouin zone and their projections on (001), (010) and (100) direction. $C_{A}=0.2~{\rm mF}$, $C_{B}=0.01~{\rm mF}$, and $C_{GA}= 0.77~{\rm mF}$ are used in the calculations. The other parameters are the same as Fig.4. The Weyl points are the intersection points between the nodal ring determined by $d_{1, 2}({k})=0$ and the two planes determined by $d_{3}({k})=0$. The chirality are indicated as blue stars (red points) for $\chi=+1$($-1$). (b) upper left: on the (001) surface, Fermi arcs connect the projections of the bulk Weyl nodes carrying opposite chiralities onto the surface. Upper right: the gapless surface band in the $A-B$ path. Dashed green line denotes the frequency where Weyl points lie. Bottom left: the Chern numbers for planes perpendicular to $k_{a}$. Moving along $k_{a}$, the Chern number increases (decreases) when the plane passing through the Weyl points with +1(–1) chirality

下载: 全尺寸图片幻灯片

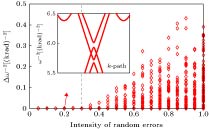
图 6 $3\times 3\times 3$超胞的能隙$\Delta\omega^{-2}\equiv\min\{\omega_{N/2+1}^{2}-\omega_{N/2}^{-2}\}$随误差幅度的变化, 其中N是能带条数. 参数同图5理想情形. 每种误差幅度随机重复计算100次. 数值计算中误差幅度不超过30%时外尔点仍然存在. 小图为电容误差幅度为$\pm 20\%$时两个外尔点所在路径的色散

Fig. 6. The band gap, $\Delta\omega^{-2}\equiv\min\{\omega_{N/2+1}^{2}-\omega_{N/2}^{-2}\}$ as a function of the tolerance values for a $3\times 3\times 3$ super cell, where N is the number of total bands. The parameters are the same as Fig.5 in ideal case. Each range of tolerance is calculated 100 times. Numerical results show that Weyl points survive for ranges less than the critical value 30%. Inset: the bands along the k-path crossing two Weyl points with 20% range of tolerance on the capacitors.

下载: 全尺寸图片幻灯片

map

[1]	Qi X L, Zhang S C 2011 Rev. Mod. Phys. 83 1057 Google Scholar
[2]	Hasan M Z, Kane C L 2010 Rev. Mod. Phys. 82 3045 Google Scholar
[3]	Chiu C K, Teo J C, Schnyder A P, Ryu S 2016 Rev. Mod. Phys. 88 035005 Google Scholar
[4]	Xu G, Weng H, Wang Z, Dai X, Fang Z 2011 Phys. Rev. Lett. 107 186806 Google Scholar
[5]	Wan X, Turner A M, Vishwanath A, et al. 2011 Phys. Rev. B 83 205101 Google Scholar
[6]	Burkov A 2018 Ann. Rev. Cond. Mat. Phys. 9 359 Google Scholar
[7]	Weng H M, Fang C, Fang Z, Bernevig B A, Dai X 2015 Phys. Rev. X 5 011029 Google Scholar
[8]	Lv B Q, Weng H M, Fu B B, Wang X P, et al. 2015 Phys. Rev. X 5 031013 Google Scholar
[9]	Xu S Y, Belopolski I, Alidoust N, et al. 2015 Science 349 613 Google Scholar
[10]	Weng H M, Yu R, Hu X, Dai X, Fang Z 2015 Adv. Phys. 64 227 Google Scholar
[11]	Armitage N P, Mele E J, Vishwanath A 2018 Rev. Mod. Phys. 90 015001 Google Scholar
[12]	Yu R, Fang Z, Dai X, Weng H M 2017 Fron. Phy 12 127202 Google Scholar
[13]	Fang C, Weng H M, Dai X, Fang Z 2016 Chinese Phys. B 25 117106 Google Scholar
[14]	Weng H M, Dai X, Fang Z 2016 J. Phys.: Cond. Mat. 28 303001 Google Scholar
[15]	Yan Z, Wang Z 2016 Phys. Rev. Lett. 117 087402 Google Scholar
[16]	Goldman N, Satija I, Nikolic P, et al. 2010 Phys. Rev. Lett. 105 255302 Google Scholar
[17]	Sun K, Liu W V, Hemmerich A 2012 Nature Phys. 8 67 Google Scholar
[18]	Jotzu G, Messer M, Desbuquois R, et al. 2014 Nature 515 237 Google Scholar
[19]	Aidelsburger M, Lohse M, Schweizer C, M. Atala, J. Barreiro, S. Nascimbene, N. Cooper, I. Bloch, N. Goldman 2015 Nature Phys. 11 162 Google Scholar
[20]	Dubcek T, Kennedy C J, L. Lu, W. Ketterle, M. Soljacic, H. Buljan 2015 Phys. Rev. Lett. 114 225301 Google Scholar
[21]	Xu Y, Zhang F, Zhang C 2015 Phys. Rev. Lett. 115 265304 Google Scholar
[22]	Raghu S, Haldane F D M 2008 Phys. Rev. A 78 033834 Google Scholar
[23]	Haldane F D M, Raghu S 2008 Phys. Rev. Lett. 100 013904 Google Scholar
[24]	Rechtsman M C, Zeuner J M, Plotnik Y, Y. Lumer, D. Podolsky, F. Dreisow, S. Nolte, M. Segev, A. Szameit 2013 Nature 496 196 Google Scholar
[25]	Lu L, Fu L, Joannopoulos J D, Soljacic M 2013 Nature Photon. 7 294 Google Scholar
[26]	Lu L, Wang Z D, Ran Y L, Fu L, Joannopoulos J D, Soljacic M 2015 Science 349 622 Google Scholar
[27]	Yan Q, Liu R, Yan Z, B. Liu, H. Chen, Z. Wang, L. Lu 2018 Nature Phys. 14 461 Google Scholar
[28]	Ozawa T, Price H M, Amo A, N. Goldman, M. Hafezi, L. Lu, M. C. Rechtsman, D. Schuster, J. Simon, O. Zilberberg, I. Carusotto 2019 Rev. Mod. Phys. 91 015006 Google Scholar
[29]	Wang P, Lu L, Bertoldi K 2015 Phys. Rev. Lett. 115 104302 Google Scholar
[30]	Po H C, Bahri Y, Vishwanath A 2016 Phys. Rev. B 93 205158 Google Scholar
[31]	Li F, Huang X, Lu J, J. Ma, Z. Liu 2018 Nature Phys. 14 30 Google Scholar
[32]	Xiao Y X, Ma G, Zhang Z Q, Chan C 2017 Phys. Rev. Lett. 118 166803 Google Scholar
[33]	Zhang T, Song Z, Alexandradinata A, H. Weng, C. Fang, L. Lu, Z. Fang 2018 Phys. Rev. Lett. 120 016401 Google Scholar
[34]	Wen X, Qiu C, Y. Qi, L. Ye, M. Ke, F. Zhang, Z. Liu 2019 Nature Phys. 15 352 Google Scholar
[35]	Kane C L, Lubensky T C 2013 Nature Phys. 10 39 Google Scholar
[36]	Gin-ge B, N. Upadhyaya, V. Vitelli 2014 Proc. Nat. Acad. Sci. 111 13004 Google Scholar
[37]	Paulose J, B. G.-g. Chen, V. Vitelli 2015 Nature Phys. 11 153 Google Scholar
[38]	Nash L M, Kleckner D, Read A, Vitelli V,, Turner W T M 2015 Proc. Nat. Acad. Sci. 112 14495 Google Scholar
[39]	Kariyado T, Y. Hatsugai 2015 Sci. Rep. 5 18107 Google Scholar
[40]	Susstrunk R, Huber S D 2015 Science 349 47 Google Scholar
[41]	Wang Y T, Luan P G, S. Zhang 2015 New J. Phys. 17 073031 Google Scholar
[42]	Susstrunk R, Huber S D 2016 Proc. Nat. Aca. Sci. 113 E4767 Google Scholar
[43]	Coulais C, Sounas D, Alu A 2017 Nature 542 461 Google Scholar
[44]	Takahashi Y, Kariyado T, Hatsugai Y 2017 New J. Phys. 19 035003 Google Scholar
[45]	Ronellenfitsch H, Dunkel J, arXiv: 1907.02054(2019).
[46]	Ma G, Xiao M, Chan C T 2019 Nature Rev. Phys. 1 281 Google Scholar
[47]	Ningyuan J, Owens C, Sommer A, D. Schuster, J. Simon 2015 Phys. Rev. X 5 021031 Google Scholar
[48]	Albert V V, L. I. Glazman, L. Jiang 2015 Phys. Rev. Lett. 114 173902 Google Scholar
[49]	Goren T, Plekhanov K, F. Appas, K. Le Hur 2018 Phys. Rev. B 97 041106 Google Scholar
[50]	Li Y, Sun Y, Zhu W, Z. Guo, J. Jiang, T. Kariyado, H. Chen, X. Hu, arXiv: 1801.04395 (2018).
[51]	Lee C H, Imhof S, Berger C, Bayer F, J. Brehm, L. W. Molenkamp, T. Kiessling, R. Thomale 2018 Commun. Phys. 1 39 Google Scholar
[52]	Luo K, Yu R, Weng H 2018 Research 2018 1 Google Scholar
[53]	Luo K, Feng J, Y. X. Zhao, R. Yu, arXiv: 1810.09231 (2018).
[54]	Kotwal T, Ronellenfitsch H, Moseley F, Dunkel J, arXiv: 1903.10130 (2019).
[55]	Lee C H, T. Hofmann, T. Helbig, Y. Liu, X. Zhang, M. Greiter, R. Thomale, arXiv: 1904.10183 (2019).
[56]	Yu R, Zhao Y X, A. P. Schnyder, arXiv: 1906.00883 (2019).
[57]	Lu Y, Jia N, L. Su, C. Owens, G. Juzeliūnas, D. I. Schuster, J. Simon 2019 Phys. Rev. B 99 020302 Google Scholar
[58]	Olekhno N A, Kretov E I, Stepanenko A A, Filonov D S, Yaroshenko V V, Cappello B, Matekovits L, Gorlach M A, arXiv: 1907.01016 (2019).
[59]	Liu S, Gao W, Q. Zhang, S. Ma, L. Zhang, C. Liu, Y. J. Xiang, T. J. Cui, S. Zhang 2019 Research 2019 1 Google Scholar
[60]	Serra-Garcia M, R. Süsstrunk, S. D. Huber 2019 Phys. Rev. B 99 020304 Google Scholar
[61]	Zhu W, Long Y, Chen H, Ren J 2019 Phys. Rev. B 99 115410 Google Scholar
[62]	Hofmann T, T. Helbig, C. H. Lee, M. Greiter, R. Thomale 2019 Phys. Rev. Lett. 122 247702 Google Scholar
[63]	Haenel R, Branch T, Franz M 2019 Phys. Rev. B 99 235110 Google Scholar
[64]	Imhof S, Berger C, Bayer F, Brehm J, et al. 2018 Nature Phys. 14 925 Google Scholar
[65]	Ezawa M 2018 Phys. Rev. B 98 201402 Google Scholar
[66]	Zhang Z Q, Wu B L, Song J, Jiang H, arXiv: 1906.04064 (2019).
[67]	Ezawa M 2019 arXiv: 1907.06911 (2019).
[68]	Nakata Y, Okada T, Nakanishi T, M. Kitano 2012 Phys. Status Solidi B 249 1 Google Scholar
[69]	Tzeng W J, Wu F Y 2006 J. Phys. A: Math. Gen. 39 8579 Google Scholar
[70]	Atala M, Aidelsburger M, Barreiro J T, Abanin D, T. Kitagawa, E. Demler, I. Bloch 2013 Nature Phys. 9 795 Google Scholar
[71]	Wang Q, Xiao M, Liu H, Zhu S, C. T. Chan 2016 Phys. Rev. B 93 041415 Google Scholar
[72]	Xiao M, Zhang Z, Chan C 2014 Phys. Rev. X 4 021017 Google Scholar
[73]	Xiao M, Ma G, Z. Yang, P. Sheng, Z. Q. Zhang, C. T. Chan 2015 Nature Phys. 11 240 Google Scholar
[74]	Wang L, Troyer M, Dai X 2013 Phys. Rev. Lett. 111 026802 Google Scholar
[75]	Bernevig A, Weng H M, Fang Z, Dai X 2018 J. Phys. Soc. Jpn. 87 041001 Google Scholar
[76]	Yu R, Weng H M, Fang Z, Dai X 2015 Phys. Rev. Lett. 115 036807 Google Scholar
[77]	Kim Y, Wieder B J, Kane C L, Rappe A M 2015 Phys. Rev. Lett. 115 036806 Google Scholar
[78]	Chiu C K, Schnyder A P 2014 Phys. Rev. B 90 205136 Google Scholar
[79]	Yu R, Wu Q, Fang Z, Weng H M 2017 Phys. Rev. Lett. 119 036401 Google Scholar
[80]	Fang C, Chen Y, H.-Y. Kee, L. Fu 2015 Phys. Rev. B 92 081201 Google Scholar
[81]	Bzdusek T, Wu Q, Ruegg A, Sigrist M, Soluyanov A A 2016 Nature 538 75 Google Scholar
[82]	Liang Q F, Zhou J, Yu R, Wang Z, Weng H M 2016 Phys. Rev. B 93 085427 Google Scholar
[83]	Heiss W D 2012 J. Phys. A: Math. Theor. 45 444016 Google Scholar
[84]	Zhou H, Peng C, Yoon Y, Hsu C W, Nelson K A, Fu L, Joannopoulos J D, Soljacic M, Zhen B 2018 Science 359 1009 Google Scholar
[85]	Lee T E 2016 Phys. Rev. Lett. 116 133903 Google Scholar
[86]	Yao S, Wang Z 2018 Phys. Rev. Lett. 121 086803 Google Scholar
[87]	Xiao L, Deng T, Wang K, Zhu G, Wang Z, Yi W, Xue P 2019 arXiv: 1907.12566
[88]	Hofmann T, Helbig T, Schindler F, Salgo N, Brzezinska M, M. Greiter, T. Kiessling, D. Wolf, A. Vollhardt, A. Kabasi, C. H. Lee, A. Bilusic, R. Thomale, T. Neupert 2019 arXiv: 1908.02759
[89]	Helbig T, Hofmann T, Imhof S, Abdelghany M, Kiessling T, Molenkamp L W, Lee C H, Szameit A, Greiter M, Thomale R 2019 arXiv: 1907.11562.
[90]	Chen R, Chen C Z, Gao J H, Zhou B, Xu D H 2019 arXiv: 1904.09932
[91]	Mitchell N P, Nash L M, Hexner D, Turner A M, Irvine W T M 2018 Nature Phys. 14 380 Google Scholar

[1]	刘钊. 莫尔超晶格中的分数化拓扑量子态. , 2024, 73(20): 207303. doi: 10.7498/aps.73.20241029
[2]	魏陆军, 李阳辉, 普勇. 基于外尔半金属WTe₂的自旋-轨道矩驱动磁矩翻转. , 2024, 73(1): 018501. doi: 10.7498/aps.73.20231836
[3]	赖镇鑫, 张也, 仲帆, 王强, 肖彦玲, 祝世宁, 刘辉. 基于合成维度拓扑外尔点的波长选择热辐射超构表面. , 2024, 73(11): 117802. doi: 10.7498/aps.73.20240512
[4]	朱庞栋, 王长昊, 王如志. 节线半金属AlB₂水环境下发生吸附后拓扑表面态变化. , 2024, 73(12): 127101. doi: 10.7498/aps.73.20240404
[5]	初纯光, 王安琦, 廖志敏. 拓扑半金属-超导体异质结的约瑟夫森效应. , 2023, 72(8): 087401. doi: 10.7498/aps.72.20230397
[6]	关欣, 陈刚. 双链超导量子电路中的拓扑非平庸节点. , 2023, 72(14): 140301. doi: 10.7498/aps.72.20230152
[7]	王欢, 何春娟, 徐升, 王义炎, 曾祥雨, 林浚发, 王小艳, 巩静, 马小平, 韩坤, 王乙婷, 夏天龙. 拓扑半金属及磁性拓扑材料的单晶生长. , 2023, 72(3): 038103. doi: 10.7498/aps.72.20221574
[8]	顾梓恒, 臧强, 郑改革. 外尔半金属调制的范德瓦耳斯声子极化激元色散性质. , 2023, 72(19): 197102. doi: 10.7498/aps.72.20230167
[9]	胡军容, 孔鹏, 毕仁贵, 邓科, 赵鹤平. 声学蜂窝结构中的拓扑角态. , 2022, 71(5): 054301. doi: 10.7498/aps.71.20211848
[10]	李牮. 基于Yu-Shiba-Rusinov态的拓扑超导理论. , 2020, 69(11): 117401. doi: 10.7498/aps.69.20200831
[11]	方云团, 王张鑫, 范尔盼, 李小雪, 王洪金. 基于结构反转二维光子晶体的拓扑相变及拓扑边界态的构建. , 2020, 69(18): 184101. doi: 10.7498/aps.69.20200415
[12]	梁奇锋, 王志, 川上拓人, 胡晓. 拓扑超导Majorana束缚态的探索. , 2020, 69(11): 117102. doi: 10.7498/aps.69.20190959
[13]	姜聪颖, 孙飞, 冯子力, 刘世炳, 石友国, 赵继民. 三重简并拓扑半金属磷化钼的时间分辨超快动力学. , 2020, 69(7): 077801. doi: 10.7498/aps.69.20191816
[14]	韦博元, 步海军, 张帅, 宋凤麒. 拓扑半金属ZrSiSe器件中面内霍尔效应的观测. , 2019, 68(22): 227203. doi: 10.7498/aps.68.20191501
[15]	邓韬, 杨海峰, 张敬, 李一苇, 杨乐仙, 柳仲楷, 陈宇林. 拓扑半金属材料角分辨光电子能谱研究进展. , 2019, 68(22): 227102. doi: 10.7498/aps.68.20191544
[16]	许兵, 邱子阳, 杨润, 戴耀民, 邱祥冈. 拓扑半金属的红外光谱研究. , 2019, 68(22): 227804. doi: 10.7498/aps.68.20191510
[17]	喻祥敏, 谭新生, 于海峰, 于扬. 利用超导量子电路模拟拓扑量子材料. , 2018, 67(22): 220302. doi: 10.7498/aps.67.20181857
[18]	伊长江, 王乐, 冯子力, 杨萌, 闫大禹, 王翠香, 石友国. 拓扑半金属材料的单晶生长研究进展. , 2018, 67(12): 128102. doi: 10.7498/aps.67.20180796
[19]	王青, 盛利. 磁场中的拓扑绝缘体边缘态性质. , 2015, 64(9): 097302. doi: 10.7498/aps.64.097302
[20]	张新国, 孙洪涛, 赵金兰, 刘冀钊, 马义德, 韩廷武. 蔡氏电路的功能全同电路与拓扑等效电路及其设计方法. , 2014, 63(20): 200503. doi: 10.7498/aps.63.200503

计量

文章访问数: 15534
PDF下载量: 811
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

电路中的拓扑态