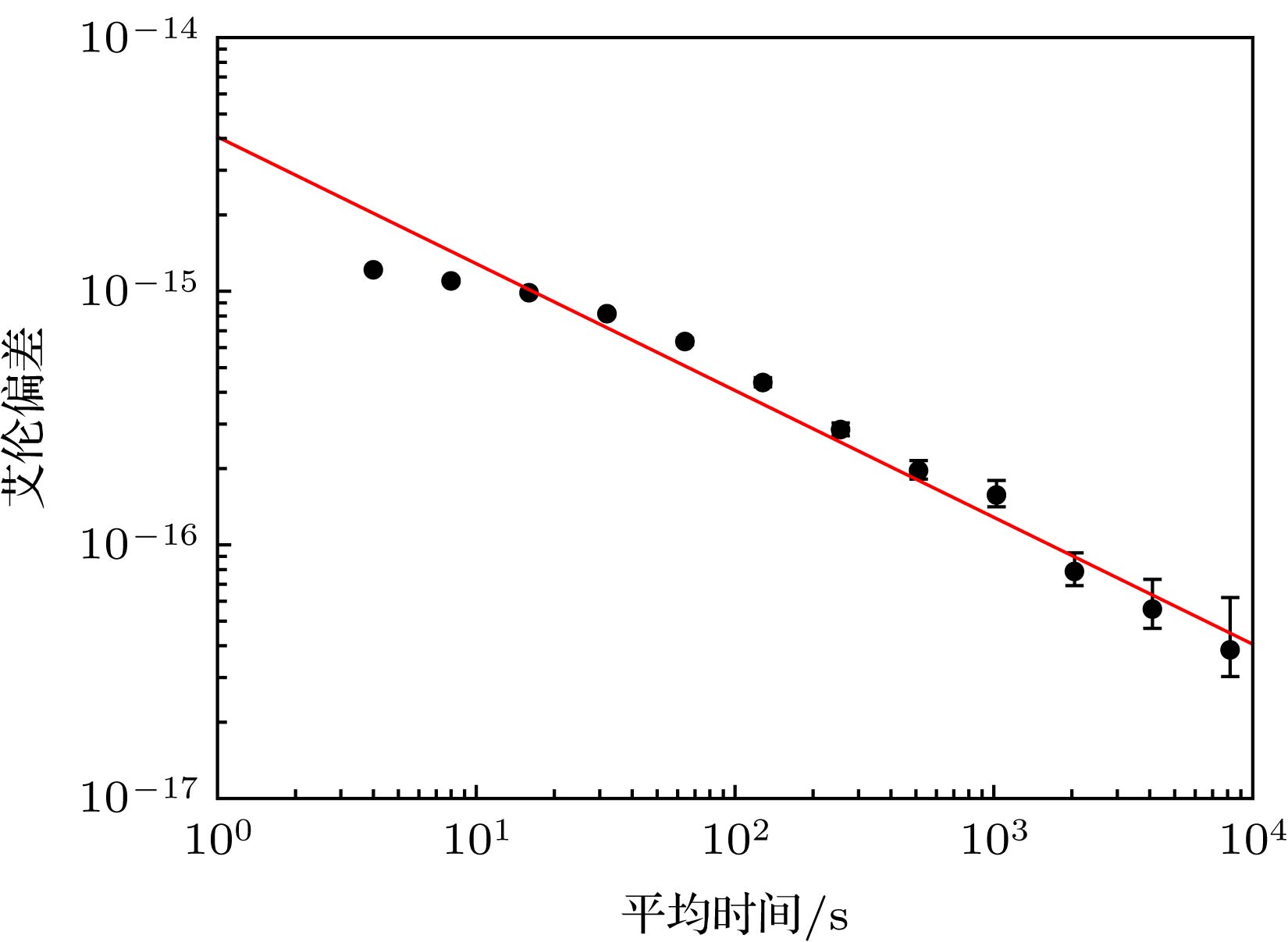
-
中性原子光晶格钟的系统不确定度评估中, 碰撞频移引起的频移修正量和不确定度是其中重要的一项, 且其评估结果将直接影响交流斯塔克频移的评估. 碰撞频移来源于囚禁在同一个格点里面原子间的相互作用, 其大小与原子的密度有关. 本文实验测量了国家授时中心87Sr光晶格钟的碰撞频移. 利用水平方向的一维光晶格囚禁数目在104量级、温度为3.4 μK的冷原子, 用极化光将原子抽运到基态mF = ± 9/2的塞曼子能级上, 获得了钟跃迁自旋极化谱. 通过高低原子密度自比对的方法测量了87Sr光晶格钟系统中与原子密度相关的碰撞频移. 在原子密度差为4 × 1010/cm3的条件下对系统的碰撞频移进行了37次独立测量, 得到系统的碰撞频移为–0.13 Hz, 统计不确定度为3.1 × 10–17. 自比对的艾伦偏差在8000 s时达到了4 × 10–17, 表明系统的测量精度在10–17量级是可靠的, 为锶原子光晶格钟系统不确定度全面评估奠定了基础.In a one-dimensional Fermion optical lattice clock, the p-wave scattering can occur when collision energy is sufficient to overcome the centrifugal barrier of p-wave scattering. According to Pauli exclusion principle, the s-wave scattering is forbidden between two identical Fermions. However, the s-wave scattering may also exist due to inhomogeneous excitation which leads to some difference between two Fermions. In terms of the uncertainty evaluation of a neutral atomic optical lattice clock, the frequency correction and uncertainty caused by atomic interaction cannot be ignored, and it will affect the evaluation of AC stark frequency shift. So the uncertainty evaluation of the collision frequency shift should be as small as possible. Only in this way can a neutral atomic optical lattice clock have a state-of-the-art performance. The collision frequency shift originates from the interaction between atoms trapped in an identical lattice. In this study, the collision frequency shift of 87Sr optical lattice clock at the National Timing Service Center is measured experimentally. A horizontal one-dimensional optical lattice is constructed. The number of tapped atoms is about 104 at a temperature of 3.4 μK. A laser is used to pump the atoms to either of the Zeeman energy levels of mF = ± 9/2 in the ground state, and the clock transition spin polarization spectrum is obtained. In a spin polarized Fermions system, the collision frequency shift relating to atomic density is measured by the method of self-comparison. The method of self-comparison, which takes full advantage of the excellent short-term stability of the clock laser, can be used to measure the frequency difference caused by the variety of system parameters. Owing to the fact that the collision frequency shift is proportional to atomic density, the collision frequency shift can be measured by the method of self-comparison between high and low atomic density. In the experiment, the systematic state is changed between high and low atomic density by periodically changing the loading time of the first stage of cooling. In order to reduce the statistical uncertainty of the measurement, the collision frequency shift is separately measured 37 times. Finally, when the atomic density is 4 × 1010/cm3, the collision frequency shift is –0.13 Hz, and the statistical uncertainty of the measurement is 3.1 × 10–17. The Allan deviation of self-comparison between low and high atomic density reaches 4 × 10–17 after 8000 s averaging time, indicating that the accuracy of the measurement is reliable and on the order of 10–17. This work lays a foundation of the total uncertainty evaluation of 87Sr optical lattice clock.
-
Keywords:
- collision frequency shift /
- strontium optical lattice clock /
- optical lattice /
- spin-polarized spectrum
[1] Margolis H 2014 Nat. Phys. 10 82
Google Scholar
[2] Riehle F 2015 C. R. Phys. 16 506
Google Scholar
[3] Bregolin F, Milani G, Pizzocaro M, Rauf B Thoumany P, Levi F, Calonico D 2017 J. Phys.: Conf. Ser. 841 012015
Google Scholar
[4] Takano T, Takamoto M, Ushijima I, Ohmae N, Akatsuka T, Yamaguchi A, Kuroishi Y, Munekane H, Miyahara B, Katori H 2016 Nat. Photon. 10 662
Google Scholar
[5] Chou C W, Hume D B, Rosenband T, Wineland D J 2010 Science 329 1630
Google Scholar
[6] Delva P, Lodewyck J 2013 Acta Futura 7 67
[7] Lion G, Panet I, Wolf P, Guerlin C, Bize S, Delva P 2017 J. Geod. 91 597
Google Scholar
[8] Grotti J, Koller S, Vogt S, et al. 2018 Nat. Phys. 14 437
Google Scholar
[9] Kolkowitz S, Pikovski I, Langellier N, Lukin M D, Walsworth R L, Ye J 2016 Phys. Rev. D 94 124043
Google Scholar
[10] Delva P, Lodewyck J, Bilicki S, et al. 2017 Phys. Rev. Lett. 118 221102
Google Scholar
[11] Derevianko A, Pospelov M 2014 Nat. Phys. 10 933
Google Scholar
[12] Arvanitaki A, Huang J, van Tilburg K 2015 Phys. Rev. D 91 015015
Google Scholar
[13] Wcisło P, Morzyński P, Bober M, Cygan A, Lisak D, Ciuryło R, Zawada M 2016 Nat. Astron. 1 0009
Google Scholar
[14] Hees A, Guéna J, Abgrall M, Bize S, Wolf P 2016 Phys. Rev. Lett. 117 061301
Google Scholar
[15] Roberts B M, Blewitt G, Dailey C, Murphy M, Pospelov M, Rollings A, Sherman J, Williams W, Derevianko A 2017 Nat. Commun. 8 1195
Google Scholar
[16] Blatt S, Ludlow A D, Campbell G K, et al. 2008 Phys. Rev. Lett. 100 140801
Google Scholar
[17] Godun R M, Nisbet-Jones P B R, Jones J M, King S A, Johnson L A, Margolis H S, Szymaniec K, Lea S N, Bongs K, Gill P 2014 Phys. Rev. Lett. 113 210801
Google Scholar
[18] Huntemann N, Lipphardt B, Tamm C, Gerginov V, Weyers S, Peik E 2014 Phys. Rev. Lett. 113 210802
Google Scholar
[19] Takamoto M, Hong F L, Higashi R, Katori H 2005 Nature 435 321
Google Scholar
[20] Campbell S L, Hutson R B, Marti G E, et al. 2017 Science 358 90
Google Scholar
[21] Lin Y G, Wang Q, Li Y, Meng F, Lin B K, Zang E J, Sun Z, Fang F, Li T C, Fang Z J 2015 Chin. Phys. Lett. 32 090601
Google Scholar
[22] Liu H, Zhang X, Jiang K L, Wang J Q, Zhu Q, Xiong Z X, He L X, Lü B L 2017 Chin. Phys. Lett. 34 20601
Google Scholar
[23] Wang Y B, Yin M J, Ren J, Xu Q F, Lu B Q, Han J X, Guo Y, Chang H 2018 Chin. Phys. B 27 023701
Google Scholar
[24] 李婷, 卢晓同, 张强, 孔德欢, 王叶兵, 常宏 2019 68 093701
Google Scholar
Li T, Lu X T, Zhang Q, Kong D H, Wang Y B, Chang H 2019 Acta Phys. Sin. 68 093701
Google Scholar
[25] Gao Q, Zhou M, Han C, Li S, Zhang S, Yao Y, Li B, Qiao H, Ai D, Lou G, Zhang M, Jiang Y, Bi Z, Ma L, Xu X Y 2018 Sci. Rep. 8 8022
Google Scholar
[26] Ludlow A D, Boyd M M, Ye J, Peik E, Schmidt P O 2015 Rev. Mod. Phys. 87 637
Google Scholar
[27] Lemke N D, Stecher J V, Sherman J A, Rey A M, Oates C W, Ludlow A D 2011 Phys. Rev. Lett. 107 103902
Google Scholar
[28] Zhang X, Bishof M, Bromley S L, Kraus C V, Safronova M S, Zoller P, Rey A M, Ye J 2014 Science 345 1467
Google Scholar
[29] Rey A M, Gorshkov A V, Kraus C V 2014 Ann. Phys. 340 311
Google Scholar
[30] Sang K L, Chang Y P, Won-Kyu L, Dai-Hyuk Y 2016 New J. Phys. 18 033030
Google Scholar
[31] Ludlow A D, Zelevinsky T, Campbell G K 2008 Science 319 1805
Google Scholar
[32] Wang Q, Lin Y G, Meng F 2016 Chin. Phys. Lett. 33 103201
Google Scholar
[33] Nicholson T L, Martin M J, Williams J R, Bloom B J, Bishof M M, Swallows D, Campbell S L, Ye J 2012 Phys. Rev. Lett. 109 230801
Google Scholar
[34] Wang Y B, Lu X T, Lu B Q, Kong D H, Chang H 2018 Appl. Sci. 8 2194
Google Scholar
[35] McDonald M, McGuyer B H, Iwata G Z, Zelevinsky T 2015 Phys. Rev. Lett. 114 023001
Google Scholar
[36] Falke S, Schnatz H, Vellore Winfred J S R, Middelmann T, Vogt S, Weyers S, Lipphardt B, Grosche G, Riehle F, Sterr U, Lisdat C 2011 Metrologia 48 399
Google Scholar
-
图 1 锶原子光晶格钟光晶格和钟跃迁探测结构简图, 其中MS为机械开关; L1为透镜; GP1-2为格兰泰勒棱镜; CR为凹面镜
Fig. 1. Schematic diagram of the optical lattice and clock transition detection of strontium optical lattice clock. L1, lens; CR, concave mirror; GP1-2, Glan prism; MS, mechanical switch.
图 2 87Sr原子钟跃迁谱线 (a)边带可分辨钟跃迁谱线; (b)钟跃迁自旋极化谱
Fig. 2. Spectra of the 87Sr clock transition: (a) Sideband resolvable clock transition line measured in the experiment; (b) spin-polarized spectra of the clock transitions.
图 3 自比对方法 (a)自旋极化峰, fH和fL分别对应高密度和低密度状态下钟跃迁的中心频率, δvC为碰撞频移的值; (b) 锁定反馈原理, fH1 和fL1是初始设定的激光频率,
$f'_{\rm H1} $ 和$f'_{\rm L1} $ 是修正激光频率, Err1和Err2是误差信号, Δf1和Δf2是频率修正量; (c) 时间序列; (d)交替改变原子密度获得的钟跃迁谱线; (e)高、低原子密度状态下原子的跃迁几率Fig. 3. The method of self-comparison: (a) The spin-polarized peaks, fH and fL are the center frequency of locked clock transition, δvC is the value of collision frequency shift; (b) the feedback loop schematic, fH1 and fL1 are initial clock laser frequency of high-density and low-density respectively,
$f'_{\rm H1} $ and$f'_{\rm L1} $ are the frequency of being corrected, Err1 and Err2 are error signals, Δf1 and Δf2 are revisionary frequency; (c) the time sequence; (d) the clock transition spectrum during alternately changing atomic density; (e) the excitation fraction at high and low atomic densities.
图 4 高低密度自比对艾伦偏差
Fig. 4. The Allan deviation obtained by the method of self-comparison between low and high atomic density.
-
[1] Margolis H 2014 Nat. Phys. 10 82
Google Scholar
[2] Riehle F 2015 C. R. Phys. 16 506
Google Scholar
[3] Bregolin F, Milani G, Pizzocaro M, Rauf B Thoumany P, Levi F, Calonico D 2017 J. Phys.: Conf. Ser. 841 012015
Google Scholar
[4] Takano T, Takamoto M, Ushijima I, Ohmae N, Akatsuka T, Yamaguchi A, Kuroishi Y, Munekane H, Miyahara B, Katori H 2016 Nat. Photon. 10 662
Google Scholar
[5] Chou C W, Hume D B, Rosenband T, Wineland D J 2010 Science 329 1630
Google Scholar
[6] Delva P, Lodewyck J 2013 Acta Futura 7 67
[7] Lion G, Panet I, Wolf P, Guerlin C, Bize S, Delva P 2017 J. Geod. 91 597
Google Scholar
[8] Grotti J, Koller S, Vogt S, et al. 2018 Nat. Phys. 14 437
Google Scholar
[9] Kolkowitz S, Pikovski I, Langellier N, Lukin M D, Walsworth R L, Ye J 2016 Phys. Rev. D 94 124043
Google Scholar
[10] Delva P, Lodewyck J, Bilicki S, et al. 2017 Phys. Rev. Lett. 118 221102
Google Scholar
[11] Derevianko A, Pospelov M 2014 Nat. Phys. 10 933
Google Scholar
[12] Arvanitaki A, Huang J, van Tilburg K 2015 Phys. Rev. D 91 015015
Google Scholar
[13] Wcisło P, Morzyński P, Bober M, Cygan A, Lisak D, Ciuryło R, Zawada M 2016 Nat. Astron. 1 0009
Google Scholar
[14] Hees A, Guéna J, Abgrall M, Bize S, Wolf P 2016 Phys. Rev. Lett. 117 061301
Google Scholar
[15] Roberts B M, Blewitt G, Dailey C, Murphy M, Pospelov M, Rollings A, Sherman J, Williams W, Derevianko A 2017 Nat. Commun. 8 1195
Google Scholar
[16] Blatt S, Ludlow A D, Campbell G K, et al. 2008 Phys. Rev. Lett. 100 140801
Google Scholar
[17] Godun R M, Nisbet-Jones P B R, Jones J M, King S A, Johnson L A, Margolis H S, Szymaniec K, Lea S N, Bongs K, Gill P 2014 Phys. Rev. Lett. 113 210801
Google Scholar
[18] Huntemann N, Lipphardt B, Tamm C, Gerginov V, Weyers S, Peik E 2014 Phys. Rev. Lett. 113 210802
Google Scholar
[19] Takamoto M, Hong F L, Higashi R, Katori H 2005 Nature 435 321
Google Scholar
[20] Campbell S L, Hutson R B, Marti G E, et al. 2017 Science 358 90
Google Scholar
[21] Lin Y G, Wang Q, Li Y, Meng F, Lin B K, Zang E J, Sun Z, Fang F, Li T C, Fang Z J 2015 Chin. Phys. Lett. 32 090601
Google Scholar
[22] Liu H, Zhang X, Jiang K L, Wang J Q, Zhu Q, Xiong Z X, He L X, Lü B L 2017 Chin. Phys. Lett. 34 20601
Google Scholar
[23] Wang Y B, Yin M J, Ren J, Xu Q F, Lu B Q, Han J X, Guo Y, Chang H 2018 Chin. Phys. B 27 023701
Google Scholar
[24] 李婷, 卢晓同, 张强, 孔德欢, 王叶兵, 常宏 2019 68 093701
Google Scholar
Li T, Lu X T, Zhang Q, Kong D H, Wang Y B, Chang H 2019 Acta Phys. Sin. 68 093701
Google Scholar
[25] Gao Q, Zhou M, Han C, Li S, Zhang S, Yao Y, Li B, Qiao H, Ai D, Lou G, Zhang M, Jiang Y, Bi Z, Ma L, Xu X Y 2018 Sci. Rep. 8 8022
Google Scholar
[26] Ludlow A D, Boyd M M, Ye J, Peik E, Schmidt P O 2015 Rev. Mod. Phys. 87 637
Google Scholar
[27] Lemke N D, Stecher J V, Sherman J A, Rey A M, Oates C W, Ludlow A D 2011 Phys. Rev. Lett. 107 103902
Google Scholar
[28] Zhang X, Bishof M, Bromley S L, Kraus C V, Safronova M S, Zoller P, Rey A M, Ye J 2014 Science 345 1467
Google Scholar
[29] Rey A M, Gorshkov A V, Kraus C V 2014 Ann. Phys. 340 311
Google Scholar
[30] Sang K L, Chang Y P, Won-Kyu L, Dai-Hyuk Y 2016 New J. Phys. 18 033030
Google Scholar
[31] Ludlow A D, Zelevinsky T, Campbell G K 2008 Science 319 1805
Google Scholar
[32] Wang Q, Lin Y G, Meng F 2016 Chin. Phys. Lett. 33 103201
Google Scholar
[33] Nicholson T L, Martin M J, Williams J R, Bloom B J, Bishof M M, Swallows D, Campbell S L, Ye J 2012 Phys. Rev. Lett. 109 230801
Google Scholar
[34] Wang Y B, Lu X T, Lu B Q, Kong D H, Chang H 2018 Appl. Sci. 8 2194
Google Scholar
[35] McDonald M, McGuyer B H, Iwata G Z, Zelevinsky T 2015 Phys. Rev. Lett. 114 023001
Google Scholar
[36] Falke S, Schnatz H, Vellore Winfred J S R, Middelmann T, Vogt S, Weyers S, Lipphardt B, Grosche G, Riehle F, Sterr U, Lisdat C 2011 Metrologia 48 399
Google Scholar
下载:
计量
- 文章访问数: 9049
- PDF下载量: 85
- 被引次数: 0
