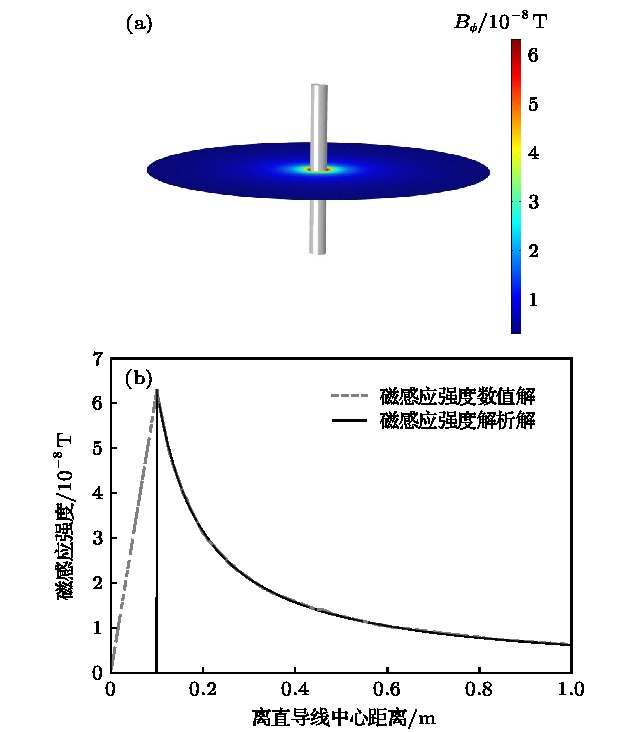
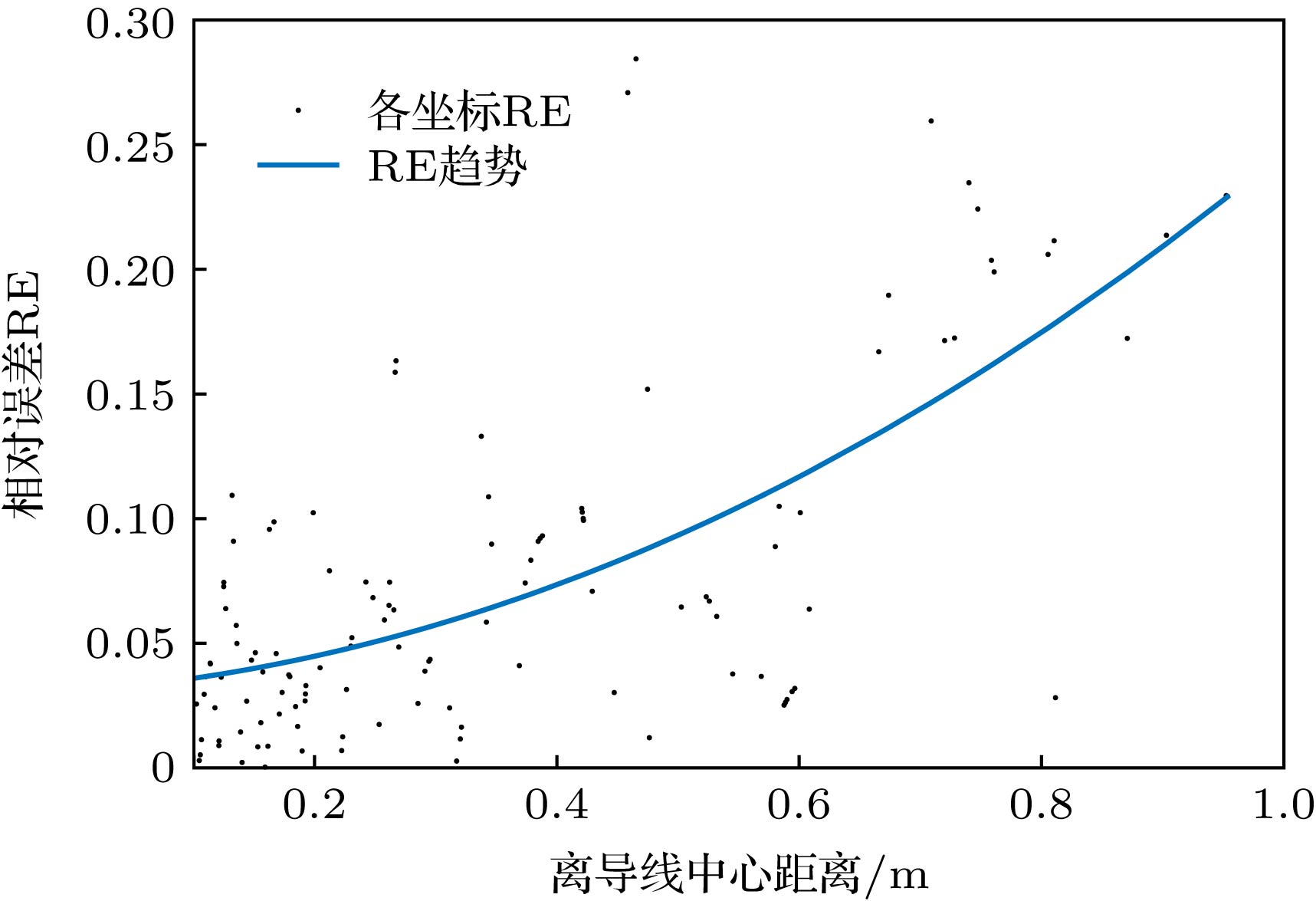
-
建立了基于有限元法(FEM)的三维心磁正问题计算框架, 以研究人体心脏电生理活动产生的磁场问题. 首先对被试的心脏和躯干磁共振影像数据源进行三维个性化建模, 获得心脏-躯干几何模型. 其次结合心脏三维模型与修正的FitzHugh-Nagumo (FHN)方程研究由跨膜电位 (TMP)产生的电兴奋在心脏内部的传导. 随后利用躯干三维模型与准静态麦克斯韦方程, 研究心脏电兴奋产生的生物电磁场在体内的传播过程, 进而获得体表外的心脏磁场分布. 心磁计算框架仿真结果显示, 使用FEM的模型可以较好地模拟体表外磁场分布. 一维FHN方程和直导线的简化模型数值结果分别与解析解呈现出较好的一致性, 验证了该计算框架的可行性. 综上, 该框架成功地仿真了TMP在心脏内部的传播过程及其在体表外投影的磁场分布, 这将有助于未来心磁逆问题求解的研究.
-
关键词:
- 心磁 /
- FitzHugh-Nagumo方程 /
- 有限元法 /
- 跨膜电位
In order to simulate the distribution of magnetic field generated by cardiac electrophysiological activities, a three-dimensional (3D) computing framework of magnetocardiogram forward problem based on a finite element method (FEM) is proposed. First, the 3D heart-torso geometry model is established from the 3D reconstruction of magnetic resonance images. Then the modified FitzHugh-Nagumo (FHN) equation combined with 3D cardiac geometry is used to investigate the propagation of transmembrane potential (TMP). In the end, quasi-static Maxwell equations and 3D torso model are used to explore the propagation of the bioelectromagnetic field produced by TMP. In our calculation, the Galerkin finite element method is used. The results show that the FEM-model can simulate extracorporeal magnetic field. Further, numerical solutions of simplified models with the one-dimensional FHN equation and the straight wire are respectively consistent with the analytical solutions, which verifies the feasibility of the computing framework. In summary, this framework successfully simulates the cardiac TMP and extracorporeal magnetic field, which may conduce to the study of magnetocardiogram inverse problem.-
Keywords:
- magnetocardiogram /
- FitzHugh-Nagumo equation /
- finite element method /
- transmembrane potential
[1] 李勇, 石曦, 陈伟宁 2008 中国医疗设备 23 46
Google Scholar
Li Y, Shi X, Chen W N 2008 Chin. Med. Equip. 23 46
Google Scholar
[2] 周志文, 郑宏超, 缪培智, 朱建民 2016 中国心血管杂志 21 60
Google Scholar
Zhou Z W, Zheng H C, Miao P Z, Zhu J M 2016 Chin. J. Cardiovasc. Med. 21 60
Google Scholar
[3] 张义红, 田青 2012 四川医学 33 452
Google Scholar
Zhang Y Q, Tian Q 2012 Sichuan Medical Journal 33 452
Google Scholar
[4] Wang L M, Wong K C L , Zhang H Y, Liu H F, Shi P C 2011 IEEE Trans. Biomed. Eng. 58 1033
Google Scholar
[5] Dang H B, Maloof A C, Romalis M V 2010 Appl. Phys. Lett. 97 227
[6] 何祥, 黄宇翔, 李曙光, 苏圣然, 郑文强, 胡正珲, 林强 2017 中国医学物理学杂志 34 1167
Google Scholar
He X, Huang Y X, Li S G, Shu S R, Zheng W Q, Hu Z H, Li Q 2017 Chin. J. Med. Phys. 34 1167
Google Scholar
[7] Hodgkin A L, Huxley A F 1952 J. Physiol. 117 500
Google Scholar
[8] Moore J W 2010 Front Comput. Neurosci. 4 20
[9] Schmitt O H 1969 Biological Information Processing Using the Concept of Interpenetrating Domains Information Processing in the Nervous System (New York: Springer) pp21−35
[10] Muler A L, Markin V S 1977 Biofizika 22 671
[11] Nielsen P M, Le G I, Smaill B H, Hunter P J 1991 Am. J. Physiol. 260 H1365
[12] Guo Y L , Heng P A , Zhang S X , Liu Z J 2005 Surg. Radiol. Anat. 27 113
Google Scholar
[13] Xia L, Zhang Y, Zhang H G, Wei Q 2006 Physiol. Meas. 27 1125
Google Scholar
[14] 李心雅, 许亮, 杨啸林, 彭屹 2011 中国生物医学工程学报 30 240
Google Scholar
Li X Y, Xu L, Yang X F, Peng Y 2011 Chin. J. Biomed. Eng. 30 240
Google Scholar
[15] 赵莉娜 2008 硕士学位论文 (山东: 山东大学)
Zhao L N 2008 M. S. Thesis (Shandong: Shangdong University) (in Chinese)
[16] 江贵平, 秦文健, 周寿军, 王昌淼 2015 计算机学报 38 1222
Google Scholar
Jiang G P, Qin W J, Zhou S J, Wang C M 2015 Chin. J. Comput. 38 1222
Google Scholar
[17] 齐丽娜, 张博, 王战凯 2006 无线电工程 36 25
Google Scholar
Qi LN, Zhang B, Wang Z K 2006 Chin. Radio. Eng. 36 25
Google Scholar
[18] Fitzhugh R 1961 Biophys. J. 1 445
Google Scholar
[19] Siregar P, Sinteff J P, Julen N, Beux P L 1998 Comput. Biomed. Res. 31 323
Google Scholar
[20] Aliev R R, Panfilov A V 1996 Chaos, Solitons Fractals 7 293
Google Scholar
[21] Wang L W, Zhang H Y, Wong K C, Liu H F, Shi P C 2010 IEEE Trans. Biomed. Eng. 57 296
Google Scholar
[22] Scher A M , Young A C 1960 Circ. Res. 8 344
Google Scholar
[23] 寿国法 2009 博士学位论文 (杭州: 浙江大学)
Shou G F 2009 Ph. D. Dissertation (Hangzhou: Zhejiang University) (in Chinese)
[24] Fischer G, Tilg B, Wach P, Lafer G, Rucker W 1998 Comput. Methods Programs Biomed. 55 99
Google Scholar
[25] Zhang H Y, Shi P C 2006 IEEE Engineering in Medicine and Biology 27th Annual Conference Shanghai, China, January 17−18, 2006 p349
[26] Zienkiewicz O C, Taylor R L著 (曾攀译 ) 2008 有限元方法 (第一卷)基本原理 (北京: 清华大学出版社)
Zienkiewicz O C, Taylor R L (translated by Zeng P) 2008 The Finite Element Method: v.1: Basic Formulation and Linear Problems (Beijing: Tsinghua University Press) p41 (in Chinese)
[27] Rogers J M, Mcculloch A D 1994 IEEE Trans. Biomed. Eng. 41 743
Google Scholar
[28] 林府标, 张千宏, 张俊, 龙文 2017 应用数学 4 200
Ling F B, Zhang Q H, Zhang J, Long W 2017 Math. Appl. 4 200
[29] Griffiths G, Schiesser W E 2011 Traveling Wave Analysis of Partial Differential Equations (New York: Academic Press) pp67−110
[30] 陈自高, 张金辉 2010 河南工程学院学报(自然科学版) 22 57
Google Scholar
Cheng Z G, Zhang J H 2010 Journal of Henan Institute of Engineering 22 57
Google Scholar
[31] Geselowitz D B 1970 IEEE Trans. Magn. 6 346
Google Scholar
[32] Nattel S 2002 Nature 415 219
Google Scholar
[33] 李明, 张朝祥, 张树林, 陈威, 鲁丽, 王毅, 孔祥燕 2017 低温 3 4
Li M, Zhang C X, Zhang S L, Chen W, Lu L, Wang Y, Kong X Y 2017 Chin. J. Low Temp. Phys. 3 4
[34] Seemann G, Keller D U J, Weiss D L, Dosse O 2008 2006 Computers in Cardiology Valencia, Spain, September 17−20, 2006 p801
-
图 1 MRI切片的图像分割 (a) 心脏; (b) 躯干
Fig. 1. Image segmentation of MRI slices: (a) Heart; (b) torso
图 4 离散的心脏-躯干三维模型 (a) 心脏三维模型; (b) 心脏-躯干模型组合
Fig. 4. Discretized three-dimensional heart-torso model: (a) 3D cardiac model; (b) heart-torso model.
图 5 t = 20, 40, 60时, 激发电位u的变化 实线: FHN方程数值解; 虚线: FHN方程行波解
Fig. 5. Evolution of excitation potential u at t = 20, 40, 60. Solid line: numerical solution of FHN equation. Dotted line: analytical solution of FHN equation.
图 6 一个心动周期的TMP分布图 (a), (b), (c) 为去极化过程 (t = 30, 60, 90); (d), (e), (f)为复极化过程(t = 120, 150, 180)
Fig. 6. TMP distribution of a cardiac cycle: (a), (b), (c) Depolarization process (t = 30, 60, 90); (d), (e), (f) repolarization process (t = 120, 150, 180).
图 7 TMP随时间变化曲线 实线: 右心室TMP变化; 虚线: 左心室TMP变化
Fig. 7. TMP time evolution curve, solid line: right ventricular TMP; dashed line: left ventricular TMP.
图 8 (a) 直导线模型; (b)中轴面磁感应强度的分布, 实线: 解析解; 虚线: 数值解
Fig. 8. (a) Straight wire model; (b) distribution of magnetic field on the axial plane. Solid line: analytical solution; dotted line: numerical solution.
图 10 体表外磁感应强度
${{{B}}_z}$ 分布 (a)−(h) 模拟的心磁分布图(t = 40, 60, 80, 100, 120, 140, 160, 180)；(i) 文献中的实测心磁图分布图[26]Fig. 10. Extracorporeal magnetic field distribution: (a)−(h) Simulated cardiac magnetic distribution (t = 40, 60, 80, 100, 120, 140, 160, 180); (i) measured human MCG in the literature.
表 1 FHN方程相对均方根误差RRMSE
Table 1. Relative root mean squared error of FHN equation.
时间 t = 20 t = 40 t = 60 RRMSE 0.39% 0.53% 0.79% 
下载: 导出CSV
-
[1] 李勇, 石曦, 陈伟宁 2008 中国医疗设备 23 46
Google Scholar
Li Y, Shi X, Chen W N 2008 Chin. Med. Equip. 23 46
Google Scholar
[2] 周志文, 郑宏超, 缪培智, 朱建民 2016 中国心血管杂志 21 60
Google Scholar
Zhou Z W, Zheng H C, Miao P Z, Zhu J M 2016 Chin. J. Cardiovasc. Med. 21 60
Google Scholar
[3] 张义红, 田青 2012 四川医学 33 452
Google Scholar
Zhang Y Q, Tian Q 2012 Sichuan Medical Journal 33 452
Google Scholar
[4] Wang L M, Wong K C L , Zhang H Y, Liu H F, Shi P C 2011 IEEE Trans. Biomed. Eng. 58 1033
Google Scholar
[5] Dang H B, Maloof A C, Romalis M V 2010 Appl. Phys. Lett. 97 227
[6] 何祥, 黄宇翔, 李曙光, 苏圣然, 郑文强, 胡正珲, 林强 2017 中国医学物理学杂志 34 1167
Google Scholar
He X, Huang Y X, Li S G, Shu S R, Zheng W Q, Hu Z H, Li Q 2017 Chin. J. Med. Phys. 34 1167
Google Scholar
[7] Hodgkin A L, Huxley A F 1952 J. Physiol. 117 500
Google Scholar
[8] Moore J W 2010 Front Comput. Neurosci. 4 20
[9] Schmitt O H 1969 Biological Information Processing Using the Concept of Interpenetrating Domains Information Processing in the Nervous System (New York: Springer) pp21−35
[10] Muler A L, Markin V S 1977 Biofizika 22 671
[11] Nielsen P M, Le G I, Smaill B H, Hunter P J 1991 Am. J. Physiol. 260 H1365
[12] Guo Y L , Heng P A , Zhang S X , Liu Z J 2005 Surg. Radiol. Anat. 27 113
Google Scholar
[13] Xia L, Zhang Y, Zhang H G, Wei Q 2006 Physiol. Meas. 27 1125
Google Scholar
[14] 李心雅, 许亮, 杨啸林, 彭屹 2011 中国生物医学工程学报 30 240
Google Scholar
Li X Y, Xu L, Yang X F, Peng Y 2011 Chin. J. Biomed. Eng. 30 240
Google Scholar
[15] 赵莉娜 2008 硕士学位论文 (山东: 山东大学)
Zhao L N 2008 M. S. Thesis (Shandong: Shangdong University) (in Chinese)
[16] 江贵平, 秦文健, 周寿军, 王昌淼 2015 计算机学报 38 1222
Google Scholar
Jiang G P, Qin W J, Zhou S J, Wang C M 2015 Chin. J. Comput. 38 1222
Google Scholar
[17] 齐丽娜, 张博, 王战凯 2006 无线电工程 36 25
Google Scholar
Qi LN, Zhang B, Wang Z K 2006 Chin. Radio. Eng. 36 25
Google Scholar
[18] Fitzhugh R 1961 Biophys. J. 1 445
Google Scholar
[19] Siregar P, Sinteff J P, Julen N, Beux P L 1998 Comput. Biomed. Res. 31 323
Google Scholar
[20] Aliev R R, Panfilov A V 1996 Chaos, Solitons Fractals 7 293
Google Scholar
[21] Wang L W, Zhang H Y, Wong K C, Liu H F, Shi P C 2010 IEEE Trans. Biomed. Eng. 57 296
Google Scholar
[22] Scher A M , Young A C 1960 Circ. Res. 8 344
Google Scholar
[23] 寿国法 2009 博士学位论文 (杭州: 浙江大学)
Shou G F 2009 Ph. D. Dissertation (Hangzhou: Zhejiang University) (in Chinese)
[24] Fischer G, Tilg B, Wach P, Lafer G, Rucker W 1998 Comput. Methods Programs Biomed. 55 99
Google Scholar
[25] Zhang H Y, Shi P C 2006 IEEE Engineering in Medicine and Biology 27th Annual Conference Shanghai, China, January 17−18, 2006 p349
[26] Zienkiewicz O C, Taylor R L著 (曾攀译 ) 2008 有限元方法 (第一卷)基本原理 (北京: 清华大学出版社)
Zienkiewicz O C, Taylor R L (translated by Zeng P) 2008 The Finite Element Method: v.1: Basic Formulation and Linear Problems (Beijing: Tsinghua University Press) p41 (in Chinese)
[27] Rogers J M, Mcculloch A D 1994 IEEE Trans. Biomed. Eng. 41 743
Google Scholar
[28] 林府标, 张千宏, 张俊, 龙文 2017 应用数学 4 200
Ling F B, Zhang Q H, Zhang J, Long W 2017 Math. Appl. 4 200
[29] Griffiths G, Schiesser W E 2011 Traveling Wave Analysis of Partial Differential Equations (New York: Academic Press) pp67−110
[30] 陈自高, 张金辉 2010 河南工程学院学报(自然科学版) 22 57
Google Scholar
Cheng Z G, Zhang J H 2010 Journal of Henan Institute of Engineering 22 57
Google Scholar
[31] Geselowitz D B 1970 IEEE Trans. Magn. 6 346
Google Scholar
[32] Nattel S 2002 Nature 415 219
Google Scholar
[33] 李明, 张朝祥, 张树林, 陈威, 鲁丽, 王毅, 孔祥燕 2017 低温 3 4
Li M, Zhang C X, Zhang S L, Chen W, Lu L, Wang Y, Kong X Y 2017 Chin. J. Low Temp. Phys. 3 4
[34] Seemann G, Keller D U J, Weiss D L, Dosse O 2008 2006 Computers in Cardiology Valencia, Spain, September 17−20, 2006 p801
下载:
计量
- 文章访问数: 9611
- PDF下载量: 93
- 被引次数: 0
