-
Fermi-Hubbard model is a fundamental lattice model describing correlated electron systems in condensed matter physics and is closely related to high-temperature superconductivity. In recent years, cold-atom quantum simulations have become an important paradigm for studying the Fermi-Hubbard model, and advances in quantum many-body computations have contributed to our understanding of its fundamental properties. Notably, a recent ultracold-atom experiment achieving the well-known antiferromagnetic (AFM) phase transition in the three-dimensional (3D) Hubbard model represents a key step in quantum simulation, laying a foundation for exploring the link between the quantum magnetism and high-temperature superconductivity. In this paper, the experimental and theoretical research progress of Fermi-Hubbard model in 3D systems is reviewed, the development history and present status in this field are discussed, and the future development direction is also prospected. The paper is organized as follows. To begin with, recent progress of observing AFM phase transitions in the 3D Hubbard model is reviewed, focusing on an ultracold-atom experiment conducted by the research group at the University of Science and Technology of China (USTC). Next, a theoretical introduction to the fundamental properties of the 3D Hubbard model is provided, in which prior theoretical studies is summarized, the current research status is outlined, and some unresolved or under-explored problems are discussed. In Section 3, the quantum simulation of the Hubbard model using ultracold atoms in optical lattices is discussed, and the basic principle, historical developments and key challenges are outlined. The USTC team overcame these challenges through innovative techniques such as atom cooling, large-scale uniform box traps, and precise measurements of the AFM structure factor. Their work successfully confirms the AFM phase transition via the critical scaling analysis. Finally, the significance of this achievement is emphasized, and the future research prospects of the 3D Hubbard model are discussed, including experimental studies on the doped regions and related theoretical benchmarks. -
Keywords:
- Fermi-Hubbard model /
- quantum simulation based on ultracold atoms /
- quantum many-body computation /
- antiferromagnetic phase transition
[1] Shao H J, Wang Y X, Zhu D Z, Zhu Y S, Sun H N, Chen S Y, Zhang C, Fan Z J, Deng Y, Yao X C, Chen Y A, Pan J W 2024 Nature 632 267
Google Scholar
[2] Arovas D P, Berg E, Kivelson S A, Raghu S 2022 Annu. Rev. Conden. Ma. P. 13 239
Google Scholar
[3] Qin M, Schäfer T, Andergassen S, Corboz P, Gull E 2022 Annu. Rev. Conden. Ma. P 13 275
Google Scholar
[4] Hubbard J, Flowers B H 1963 Proc. R. Soc. Lond. Ser. A 276 238
Google Scholar
[5] Kanamori J 1963 Prog. Theor. Phys. 30 275
Google Scholar
[6] Gutzwiller M C 1963 Phys. Rev. Lett. 10 159
Google Scholar
[7] Chen Q J, Wang Z Q, Rufus Boyack, Yang S L, Levin K 2024 Rev. Mod. Phys. 96 025002
Google Scholar
[8] Campostrini M, Hasenbusch M, Pelissetto A, Rossi P, Vicari E 2002 Phys. Rev. B 65 144520
Google Scholar
[9] Mermin N D, Wagner H 1966 Phys. Rev. Lett. 17 1133
Google Scholar
[10] Staudt R, Dzierzawa M, Muramatsu A 2000 Eur. Phys. J. B 17 411
Google Scholar
[11] Ibarra-García-Padilla E, Mukherjee R, Hulet R G, Hazzard K R A, Paiva T, Scalettar R T 2020 Phys. Rev. A 102 033340
Google Scholar
[12] Sun F, Xu X Y 2024 arXiv: 2404.09989 [cond-mat. str-el]
[13] Song Y F, Deng Y, He Y Y 2024 arXiv: 2407.08603 [cond-mat. str-el]
[14] Werner F, Parcollet O, Georges A, Hassan S R 2005 Phys. Rev. Lett. 95 056401
Google Scholar
[15] Fuchs S, Gull E, Troyer M, Jarrell M, Pruschke T 2011 Phys. Rev. B 83 235113
Google Scholar
[16] Schäfer T, Katanin A A, Held K, Toschi A 2017 Phys. Rev. Lett. 119 046402
Google Scholar
[17] Rampon L, Simkovic F, Ferrero M 2024 arXiv: 2409.08848 [cond-mat. str-el]
[18] Kozik E, Burovski E, Scarola V W, Troyer M 2013 Phys. Rev. B 87 205102
Google Scholar
[19] Lenihan C, Kim A J, Simkovic F, Kozik E 2022 Phys. Rev. Lett. 129 107202
Google Scholar
[20] Garioud R, Šimkovic F, Rossi R, Spada G, Schäfer T, Werner F, Ferrero M 2024 Phys. Rev. Lett. 132 246505
Google Scholar
[21] He Y Y, Qin M P, Shi H, Lu Z Y, Zhang S W 2019 Phys. Rev. B 99 045108
Google Scholar
[22] Zheng B X, Chung C M, Corboz P, Ehlers G, Qin M P, Noack R M, Shi H, White S R, Zhang S W, Chan G K L 2017 Science 358 1155
Google Scholar
[23] Xu H, Chung C M, Qin M P, Schollwöck U, White S R, Zhang S W 2024 Science 384 7691
Google Scholar
[24] Feynman R P 1982 Int. J. Theor. Phys. 21 467
Google Scholar
[25] Anderson M H, Ensher J R, Matthews M R, Wieman C E, Cornell E A 1995 Science 269 198
Google Scholar
[26] Jaksch D, Bruder C, Cirac J I, Gardiner C W, Zoller P 1998 Phys. Rev. Lett. 81 3108
Google Scholar
[27] DeMarco B, Jin D S 1999 Science 285 1703
Google Scholar
[28] Greiner M, Mandel O, Esslinger T, Hansch T W, Bloch I 2002 Nature 415 39
Google Scholar
[29] Jordens R, Strohmaier N, Gunter K, Moritz H, Esslinger T 2008 Nature 455 204
Google Scholar
[30] Schneider U, Hackermuller L, Will S, Best Th, Bloch I, Costi T A, Helmes R W, Rasch D, Rosch A 2008 Science 322 1520
Google Scholar
[31] Ho T L, Zhou Q 2009 arXiv: 0911.5506 [cond-mat. quant-gas]
[32] Lee P A, Nagaosa N, Wen X G 2006 Rev. Mod. Phys. 78 17
Google Scholar
[33] Duan L M, Demler E, Lukin M D 2003 Phys. Rev. Lett. 91 090402
Google Scholar
[34] Trotzky S, Cheinet P, Fölling S, et al. 2008 Science 319 295
Google Scholar
[35] Bakr W S, Gillen J I, Peng A, Folling S, Greiner M 2009 Nature 462 74
Google Scholar
[36] Haller E, Hudson J, Kelly A, Cotta D A, Peaudecerf B, Bruce G D, Kuhr S 2015 Nat. Phys. 11 738
Google Scholar
[37] Greif D, Parsons M F, Mazurenko A, Chiu C S, Blatt S, Huber F, Ji G, Greiner M 2016 Science 351 953
Google Scholar
[38] Hart R A, Duarte P M, Yang T L, Liu X X, Paiva T, Khatami E, Scalettar R T, Trivedi N, Huse D A, Hulet R G 2015 Nature 519 211
Google Scholar
[39] Mazurenko A, Chiu C S, Ji G, et al. 2017 Nature 545 462
Google Scholar
[40] Yang B, Sun H, Huang C J, Wang H Y, Deng Y J, Dai H N, Yuan Z S, Pan J W 2020 Science 369 550
Google Scholar
[41] Sun H, Yang B, Wang H Y, et al. 2021 Nat. Phys. 17 990
Google Scholar
-
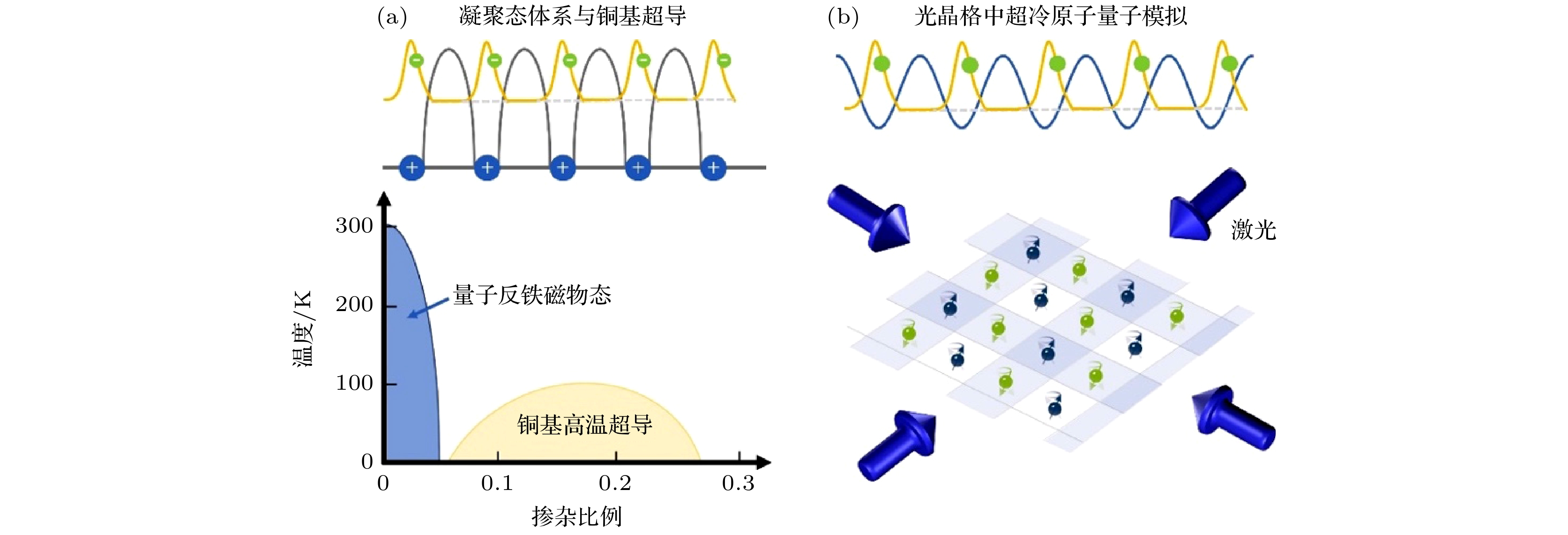
图 1 超冷原子模拟费米-哈伯德模型的示意图, 采用超冷原子等效于凝聚态体系中的电子, 而利用光学驻波构建出晶格结构, 可构筑哈伯德模型中的量子反铁磁物态, 进而通过调控体系的相互作用、温度和掺杂浓度等来研究反铁磁相变, 以及探究高温超导态的微观机理
Fig. 1. Illustration of the ultracold atom simulation for the Fermi-Hubbard model, ultracold atoms serve as analogues of electrons in condensed matter systems, with optical standing waves forming lattice structures, this setup allows for the creation of the Hubbard antiferromagnetic (AFM) state, by controlling parameters such as interaction strength, temperature and doping, the system can be utilized to study AFM phase transitions and explore the microscopic mechanism of high-temperature superconductivity.
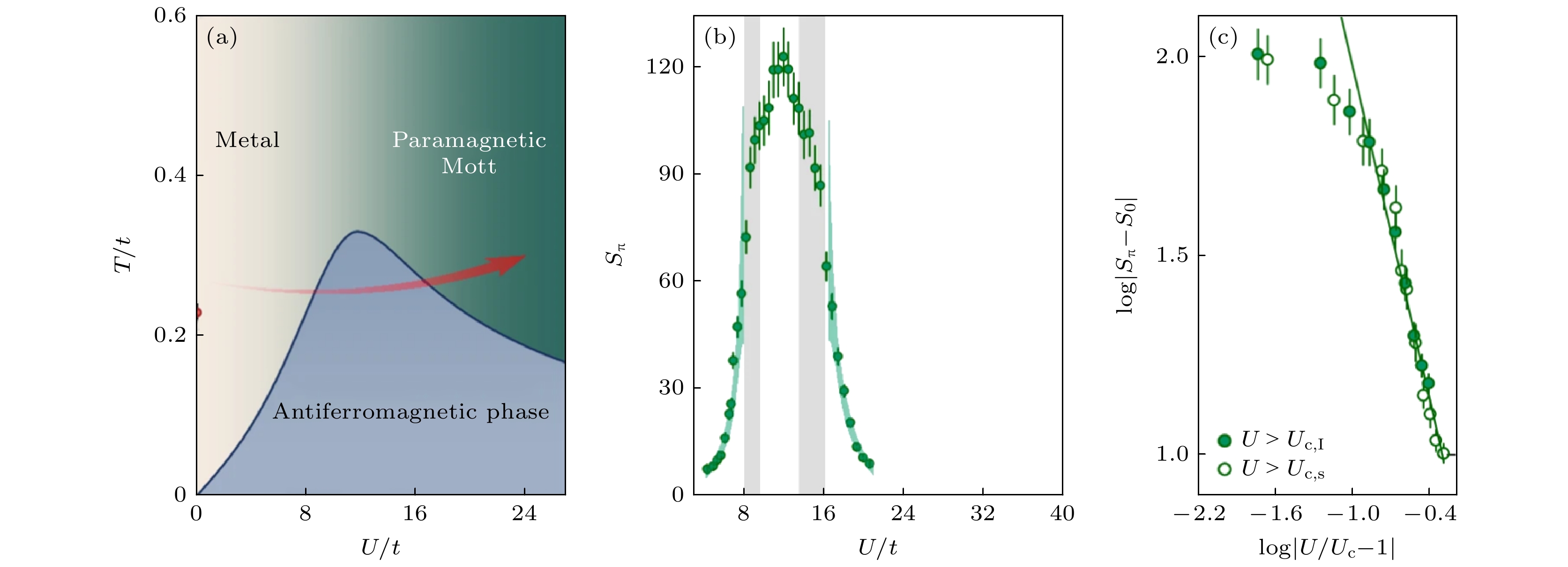
图 2 (a)半满填充下的费米-哈伯德模型大致相图[1]; (b)随着$ U $增大实验测量的反铁磁结构因子结果[1]; (c)关于$ U $的临界标度行为[1]
Fig. 2. (a) Schematic phase diagram of the 3D half-filled Fermi-Hubbard model[1]; (b) experimental results of antiferromagnetic structure factor as a function of interaction strength $ U $[1]; (c) the critical scaling behavior of antiferromagnetic structure factor[1].
图 3 使用DMFT和$ {\mathrm{D}}{{\Gamma }}{\mathrm{A}} $算法得到的掺杂三维哈伯德模型在$ U=9.8 t $的磁性相图[16], 其中AF是奈儿反铁磁序, SDW是非公度的自旋密度波序, QCP是量子临界点
Fig. 3. Magnetic phase diagram for the 3D doped Hubbard model with $ U=9.8 t $, based on DMFT and $ {\mathrm{D}}{{\Gamma }}{\mathrm{A}} $ simulations[16], AF denotes the antiferromagnetic order, and SDW represents incommensurate spin density wave order, and QCP marks the quantum critical point.
-
[1] Shao H J, Wang Y X, Zhu D Z, Zhu Y S, Sun H N, Chen S Y, Zhang C, Fan Z J, Deng Y, Yao X C, Chen Y A, Pan J W 2024 Nature 632 267
Google Scholar
[2] Arovas D P, Berg E, Kivelson S A, Raghu S 2022 Annu. Rev. Conden. Ma. P. 13 239
Google Scholar
[3] Qin M, Schäfer T, Andergassen S, Corboz P, Gull E 2022 Annu. Rev. Conden. Ma. P 13 275
Google Scholar
[4] Hubbard J, Flowers B H 1963 Proc. R. Soc. Lond. Ser. A 276 238
Google Scholar
[5] Kanamori J 1963 Prog. Theor. Phys. 30 275
Google Scholar
[6] Gutzwiller M C 1963 Phys. Rev. Lett. 10 159
Google Scholar
[7] Chen Q J, Wang Z Q, Rufus Boyack, Yang S L, Levin K 2024 Rev. Mod. Phys. 96 025002
Google Scholar
[8] Campostrini M, Hasenbusch M, Pelissetto A, Rossi P, Vicari E 2002 Phys. Rev. B 65 144520
Google Scholar
[9] Mermin N D, Wagner H 1966 Phys. Rev. Lett. 17 1133
Google Scholar
[10] Staudt R, Dzierzawa M, Muramatsu A 2000 Eur. Phys. J. B 17 411
Google Scholar
[11] Ibarra-García-Padilla E, Mukherjee R, Hulet R G, Hazzard K R A, Paiva T, Scalettar R T 2020 Phys. Rev. A 102 033340
Google Scholar
[12] Sun F, Xu X Y 2024 arXiv: 2404.09989 [cond-mat. str-el]
[13] Song Y F, Deng Y, He Y Y 2024 arXiv: 2407.08603 [cond-mat. str-el]
[14] Werner F, Parcollet O, Georges A, Hassan S R 2005 Phys. Rev. Lett. 95 056401
Google Scholar
[15] Fuchs S, Gull E, Troyer M, Jarrell M, Pruschke T 2011 Phys. Rev. B 83 235113
Google Scholar
[16] Schäfer T, Katanin A A, Held K, Toschi A 2017 Phys. Rev. Lett. 119 046402
Google Scholar
[17] Rampon L, Simkovic F, Ferrero M 2024 arXiv: 2409.08848 [cond-mat. str-el]
[18] Kozik E, Burovski E, Scarola V W, Troyer M 2013 Phys. Rev. B 87 205102
Google Scholar
[19] Lenihan C, Kim A J, Simkovic F, Kozik E 2022 Phys. Rev. Lett. 129 107202
Google Scholar
[20] Garioud R, Šimkovic F, Rossi R, Spada G, Schäfer T, Werner F, Ferrero M 2024 Phys. Rev. Lett. 132 246505
Google Scholar
[21] He Y Y, Qin M P, Shi H, Lu Z Y, Zhang S W 2019 Phys. Rev. B 99 045108
Google Scholar
[22] Zheng B X, Chung C M, Corboz P, Ehlers G, Qin M P, Noack R M, Shi H, White S R, Zhang S W, Chan G K L 2017 Science 358 1155
Google Scholar
[23] Xu H, Chung C M, Qin M P, Schollwöck U, White S R, Zhang S W 2024 Science 384 7691
Google Scholar
[24] Feynman R P 1982 Int. J. Theor. Phys. 21 467
Google Scholar
[25] Anderson M H, Ensher J R, Matthews M R, Wieman C E, Cornell E A 1995 Science 269 198
Google Scholar
[26] Jaksch D, Bruder C, Cirac J I, Gardiner C W, Zoller P 1998 Phys. Rev. Lett. 81 3108
Google Scholar
[27] DeMarco B, Jin D S 1999 Science 285 1703
Google Scholar
[28] Greiner M, Mandel O, Esslinger T, Hansch T W, Bloch I 2002 Nature 415 39
Google Scholar
[29] Jordens R, Strohmaier N, Gunter K, Moritz H, Esslinger T 2008 Nature 455 204
Google Scholar
[30] Schneider U, Hackermuller L, Will S, Best Th, Bloch I, Costi T A, Helmes R W, Rasch D, Rosch A 2008 Science 322 1520
Google Scholar
[31] Ho T L, Zhou Q 2009 arXiv: 0911.5506 [cond-mat. quant-gas]
[32] Lee P A, Nagaosa N, Wen X G 2006 Rev. Mod. Phys. 78 17
Google Scholar
[33] Duan L M, Demler E, Lukin M D 2003 Phys. Rev. Lett. 91 090402
Google Scholar
[34] Trotzky S, Cheinet P, Fölling S, et al. 2008 Science 319 295
Google Scholar
[35] Bakr W S, Gillen J I, Peng A, Folling S, Greiner M 2009 Nature 462 74
Google Scholar
[36] Haller E, Hudson J, Kelly A, Cotta D A, Peaudecerf B, Bruce G D, Kuhr S 2015 Nat. Phys. 11 738
Google Scholar
[37] Greif D, Parsons M F, Mazurenko A, Chiu C S, Blatt S, Huber F, Ji G, Greiner M 2016 Science 351 953
Google Scholar
[38] Hart R A, Duarte P M, Yang T L, Liu X X, Paiva T, Khatami E, Scalettar R T, Trivedi N, Huse D A, Hulet R G 2015 Nature 519 211
Google Scholar
[39] Mazurenko A, Chiu C S, Ji G, et al. 2017 Nature 545 462
Google Scholar
[40] Yang B, Sun H, Huang C J, Wang H Y, Deng Y J, Dai H N, Yuan Z S, Pan J W 2020 Science 369 550
Google Scholar
[41] Sun H, Yang B, Wang H Y, et al. 2021 Nat. Phys. 17 990
Google Scholar
下载:
计量
- 文章访问数: 1546
- PDF下载量: 144
- 被引次数: 0
