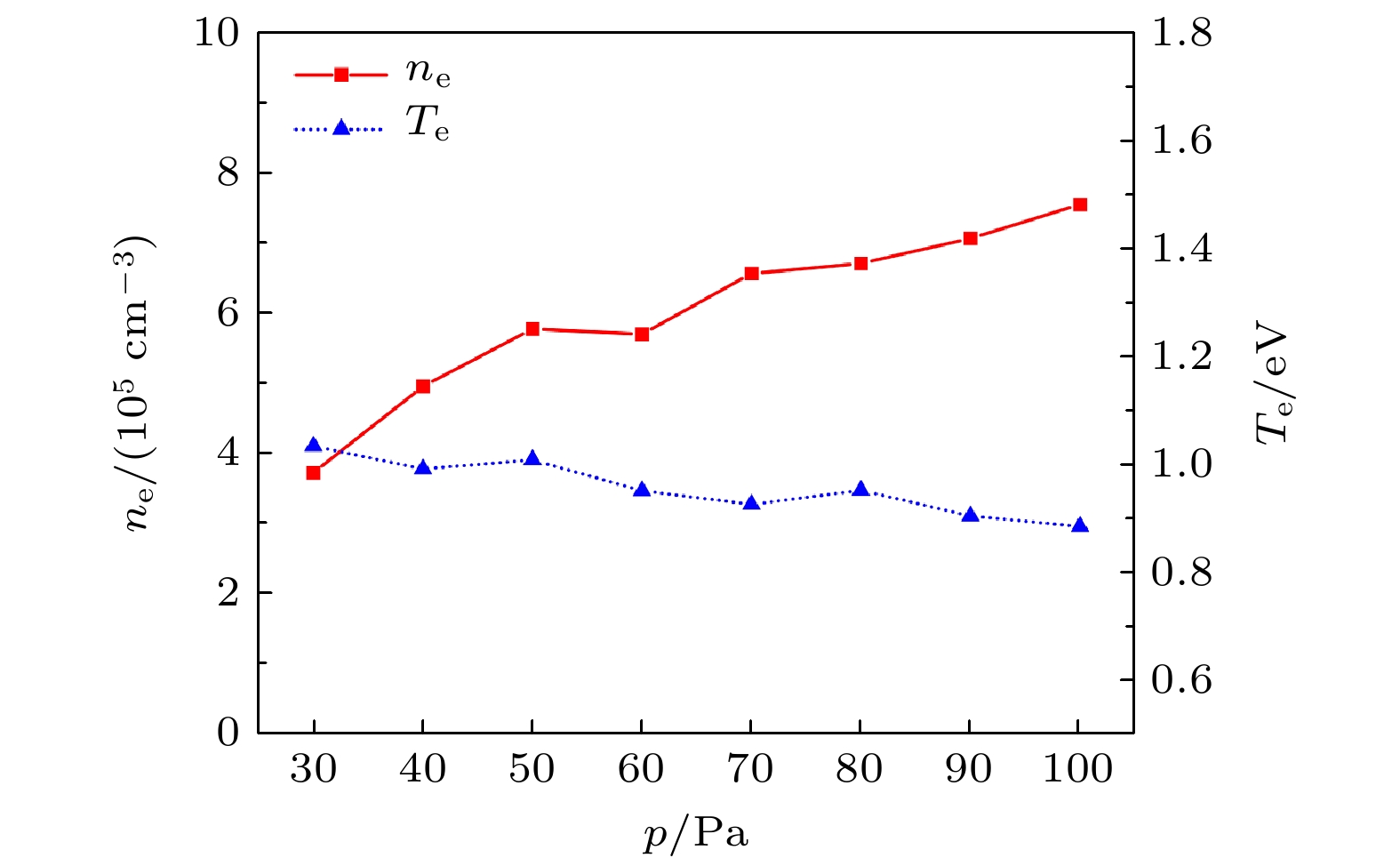
-
低电子密度等离子体源在电离层等离子体的实验室模拟中具有重要的应用. 本文利用高能电子束透射氮化硅薄膜窗口, 在低气压条件下激发低密度等离子体. 采用蒙特卡罗方法对氮化硅薄膜的电子束透射特性进行模拟; 利用CCD相机和静电探针对产生的电子束等离子体进行诊断. 电子束等离子体呈现出顶点位于氮化硅薄膜窗口的锥状结构. 在相同气压下, 电子初始能量越高, 锥状等离子体锥角越小, 等离子体更加向轴线集中, 这与氮化硅薄膜的电子透射特性蒙特卡罗模拟定性一致. 随着工作气压增大, 高能电子与中性粒子碰撞频率变大, 电子束散射效应增强导致等离子体锥角变大. 等离子体锥状结构是高能电子透射特性以及高能电子与气体分子散射特征的反映. 在40 keV条件下, 当电子束流从10 μA减小到 0.5 μA时, 电子密度减小, 变化在105—106 cm–3范围; 而电子温度没有明显的变化, 接近1 eV. 随气压增大, 电子密度有增大趋势. 研究工作表明, 在工作腔尺寸足够大的情况下, 可得到沿电子入射方向随距离增加逐渐衰减的105 cm–3以下更低的电子密度; 这是由于射程增加, 透射的高能电子与中性气体碰撞产生能量损失增大; 同时电子束散射增强导致了等离子体更加发散.In general, more attention is paid to how to improve the characteristic parameters of plasma in plasma applications. However, in some cases, it is necessary to produce plasma with low-electron density, such as in the laboratory simulation of ionospheric plasma in space science. In this study, a low-density plasma is generated by electron beams passing through a silicon nitride transmission window under low pressure condition. The transmission properties of electron beam passing through silicon nitride films are investigated by Monte Carlo simulation, and the plasma feature is studied by a planar Langmuir probe and a digital camera. It is found that the plasma exhibits a conical structure with its apex located at the transmission window. At a constant pressure, the cone angle of conical plasma decreases with the electron energy increasing. This is qualitatively consistent with the Monte Carlo simulation result. The frequency of electron-neutral collisions increases as the working pressure rising, which leads the plasma cone angle to increase. When the beam current is reduced from 10 μA to 0.5 μA at 40 keV, the electron density decreases, in a range between 105 and 106 cm–3, while the electron temperature does not change significantly but approaches 1 eV. It can be inferred that the electron density decreases with the distance z from the transmission window in the incident direction of the electron beam. A low-density plasma of less than 105 cm–3 can be obtained further away from the transmission window.
-
Keywords:
- electron beam /
- plasma generation /
- plasma source /
- electron density
[1] Donnelly V M, Kornblit A 2013 J. Vac. Sci. Technol. A 31 050825
Google Scholar
[2] Wang L, Cui L, Zhu X D, Wen X H 2007 Phys. Plasmas 14 123501
Google Scholar
[3] Chen F F, Evans J D, Tynan G R 2001 Plasma Sources Sci. Technol. 10 236
Google Scholar
[4] Fang H K, Oyama K I, Chen A B 2017 Plasma Sources Sci. Technol. 26 115010
Google Scholar
[5] Pigache D R 1973 AIAA J. 11 129
Google Scholar
[6] Stone N H, Rehmann W K 1970 The Simulation of Ionospheric Conditions for Space Vehicles TN D-5894 NASA
[7] Kelley M 2009 The Earth’s Ionosphere (2nd Ed.) (Amsterdam: Elsevier
[8] Morozov A, Heindl T, Skrobol C, Wieser J, Krücken R, Ulrich A, 2008 Eur. Phys. J. D 48 383
Google Scholar
[9] Masoud N, Martus K, Murnick D 2019 Plasma Sources Sci. Technol. 28 045010
Google Scholar
[10] Zhu B L, Yan S Q, Chen Y, Zhu X D 2022 Plasma Sources Sci. Technol. 31 025012
Google Scholar
[11] Pei X K, Zhang P, Chen K, Lu X P 2013 IEEE Trans. Plasma Sci. 41 494
Google Scholar
[12] Yan S Q, Chen Y, Ma Y, Gao J K, Zhu X D 2023 Phys. Plasmas 30 073504
Google Scholar
[13] Shimizu R, Ding Z J 1992 Rep. Prog. Phys. 55 487
Google Scholar
[14] Zhu B L, Ma Y, Yan S Q, Ke W, Yang K, Zhu X D 2020 J. Appl. Phys. 128 135302
Google Scholar
[15] 蒲其荣, 丁泽军, 孙霞, 吴自勤 2004 电子显微学报 23 571
Google Scholar
Pu Q R, Ding Z J, Sun X, Wu Z Q 2004 J. Chin. Electr. Microsc. Soc. 23 571
Google Scholar
[16] Seltzer S M, Berger M J 1982 Int. J. Appl. Radiat. Isot. 33 1189
Google Scholar
[17] Hoang H, Røed K, Bekkeng T A, Moen J I, Spicher A, Clausen L B N, Miloch W J, Trondsen E, Pedersen A 2018 Meas. Sci. Technol. 29 065906
Google Scholar
[18] Morozov A, Krücken R, Ulrich A, Wieser J 2006 J. Appl. Phys. 100 093305
Google Scholar
[19] Walton S G, Boris D R, Hernández S C, Lock E H, Petrova Tz B, Petrov G M, Fernsler R F 2015 ECS J. Solid State Sci. Technol. 4 N5033
Google Scholar
[20] Cohn A, Caledonia G 1970 J. Appl. Phys. 41 3767
Google Scholar
[21] Soloviev V, Konchakov A, Krivstov V, Malmuth N 2001 32nd AIAA Plasmadynamics and Lasers Conference Anaheim, CA, U. S. A. June 2001 AIAA 2001–3089
[22] Bai X Y, Chen C, Li H, Liu W D, Chen W 2017 Phys. Plasmas 24 103509
Google Scholar
-
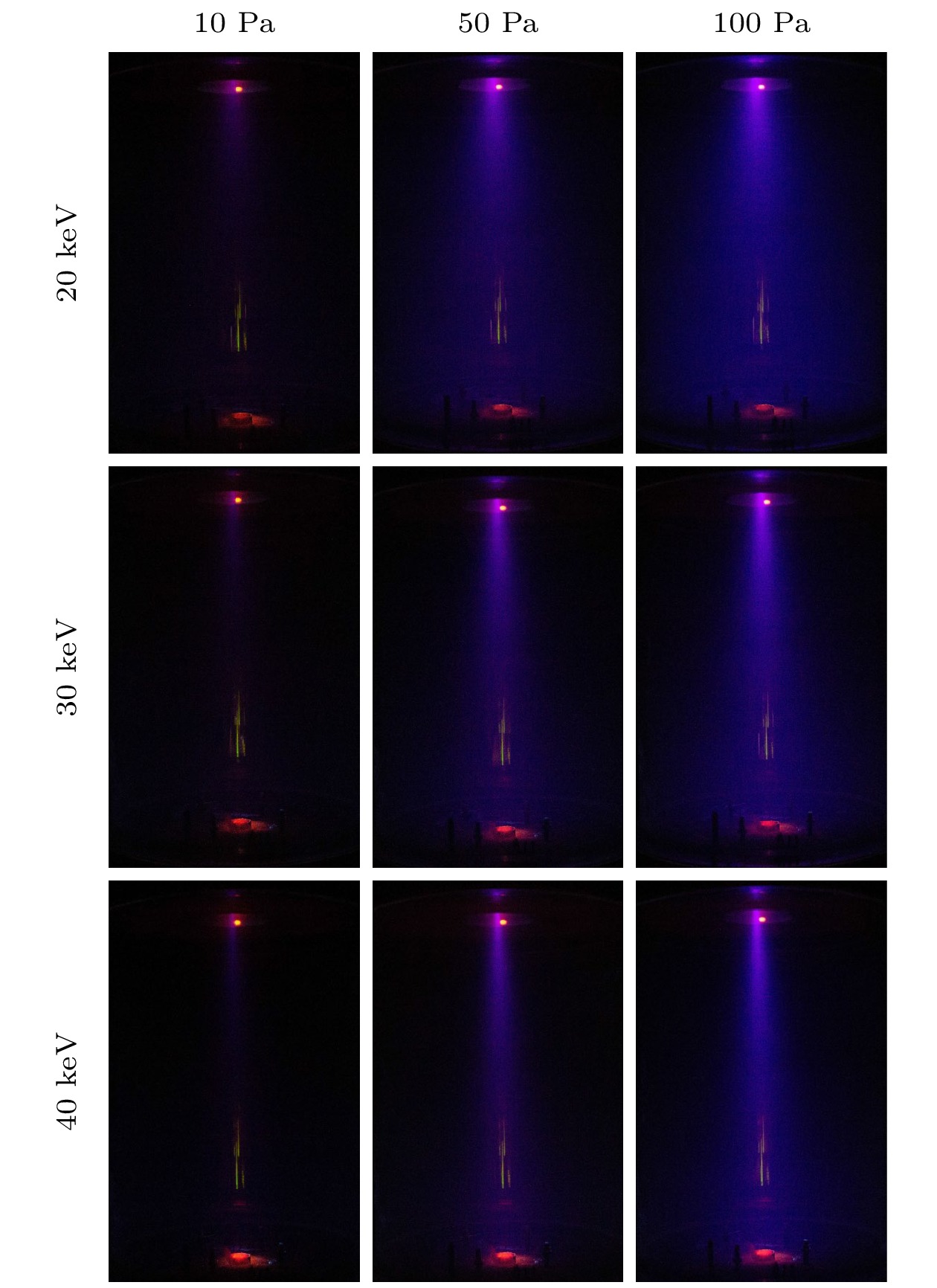
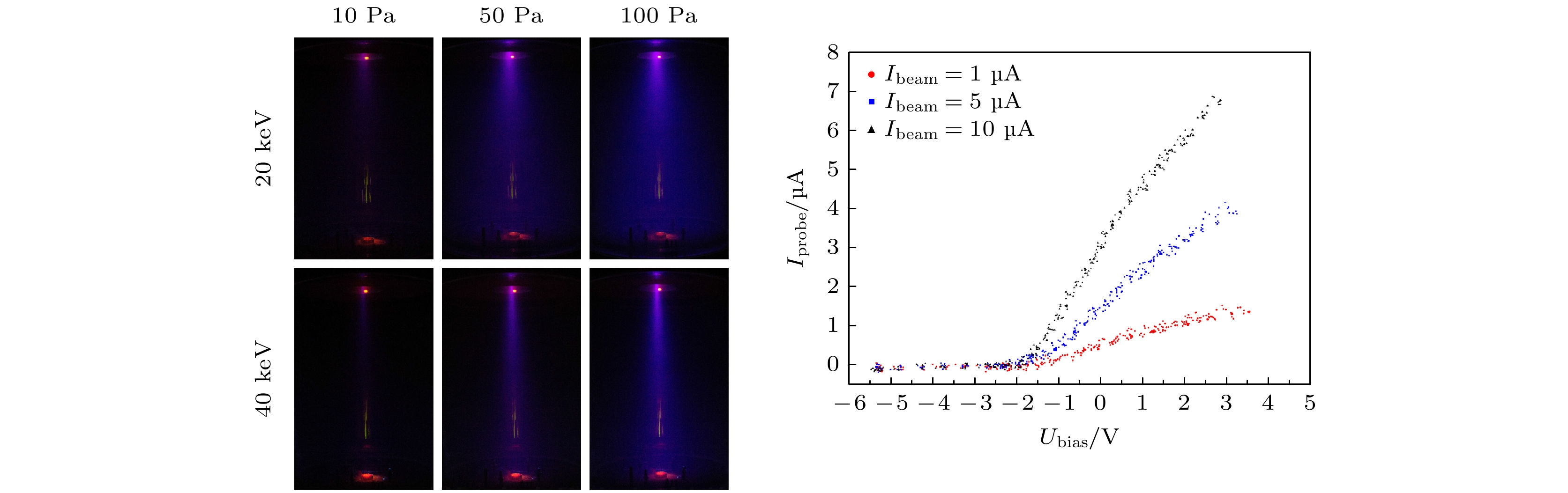
图 2 氮气等离子体放电图像, 电子束的束流为 5 μA, 横列表示不同气压, 纵列表示不同电子束能量
Fig. 2. Photos of nitrogen plasma taken by CCD digital camera. The electron beam current is controlled at 5 μA. Each column shows the change of plasma shape with electron energy when the gas pressure is fixed, and each row gives the plasma shapes at different pressures under constant electron energy.
图 3 束流传输效率TMC和能量传输效率EMC的模拟结果, 电子束能量为20—40 keV, 氮化硅薄膜厚度400 nm
Fig. 3. The simulation results of the transmittance of the electron beam TMC and energy transmission efficiency EMC with electron beam energy from 20 to 40 keV. The thickness of the silicon nitride film is 400 nm.
图 4 不同能量的入射高能电子束透射400 nm薄膜后的散射角度统计分布 (a) 透射电子的散射角分布; (b) N(θ)/Nz随θ的变化
Fig. 4. Statistical distribution of electron scattering angle after passing through the 400 nm film with different energy: (a) Distribution of electron scattering angle; (b) N(θ)/Nz ratio with θ.
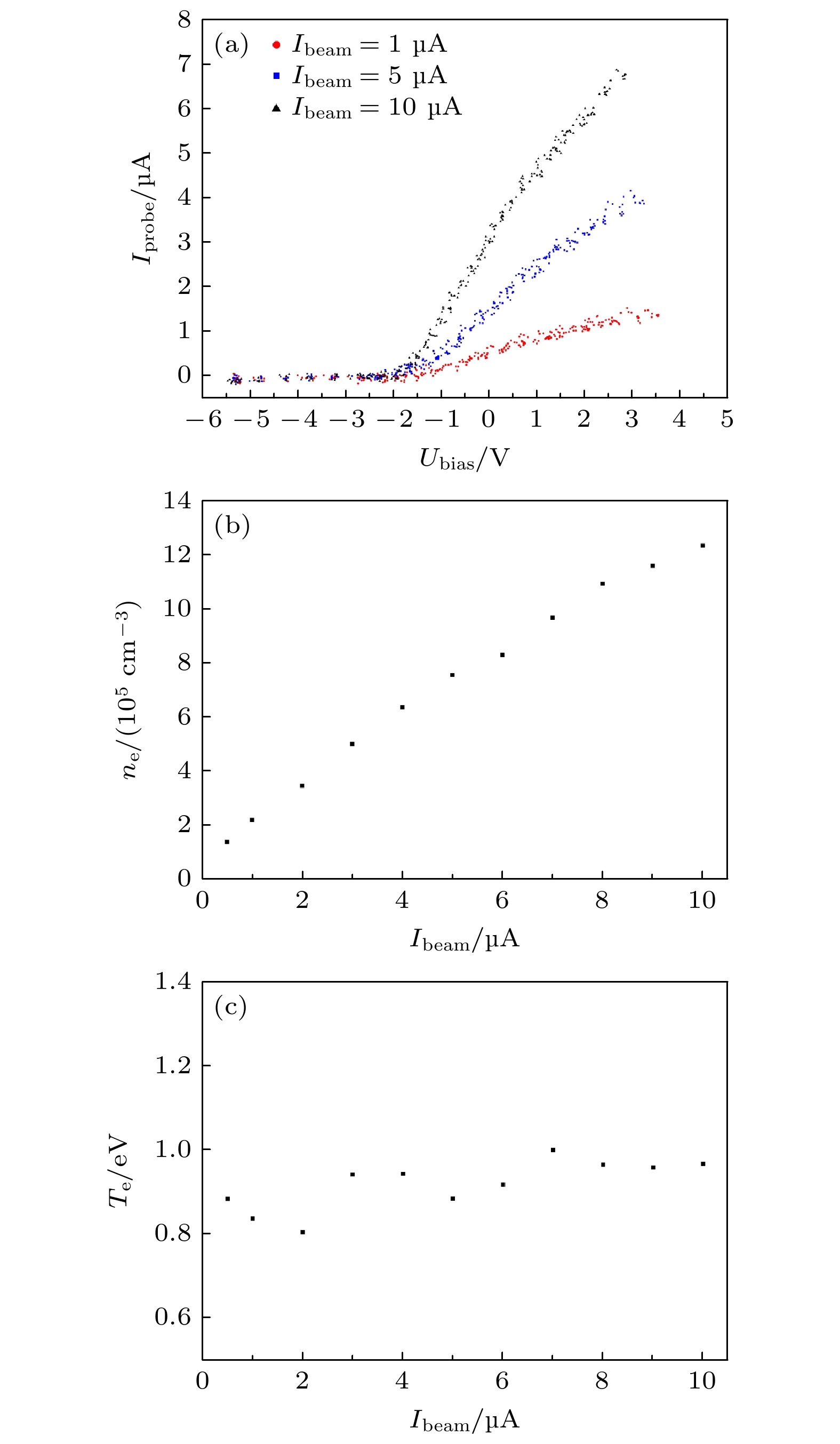
图 5 静电探针的测量结果 (a) 3个束流下典型的探针I-V曲线; (b) 电子密度随束流的变化; (c) 电子温度随束流的变化; 电子束能量为40 keV, 工作气压为100 Pa
Fig. 5. The results of the langmuir probe measurement: (a) Typical I-V curves under different beam currents; (b) the electron density variation with beam currents; (c) the electron temperature variation with beam currents. The energy of the electron beam is 40 keV, and the working pressure is 100 Pa.
图 6 电子密度和电子温度随工作气压的变化, 电子束能量为40 keV, 电子束电流为5 μA
Fig. 6. The variation of electron density and electron temperature with working pressure. The electron beam energy is 40 keV, and the electron beam current is 5 μA.
-
[1] Donnelly V M, Kornblit A 2013 J. Vac. Sci. Technol. A 31 050825
Google Scholar
[2] Wang L, Cui L, Zhu X D, Wen X H 2007 Phys. Plasmas 14 123501
Google Scholar
[3] Chen F F, Evans J D, Tynan G R 2001 Plasma Sources Sci. Technol. 10 236
Google Scholar
[4] Fang H K, Oyama K I, Chen A B 2017 Plasma Sources Sci. Technol. 26 115010
Google Scholar
[5] Pigache D R 1973 AIAA J. 11 129
Google Scholar
[6] Stone N H, Rehmann W K 1970 The Simulation of Ionospheric Conditions for Space Vehicles TN D-5894 NASA
[7] Kelley M 2009 The Earth’s Ionosphere (2nd Ed.) (Amsterdam: Elsevier
[8] Morozov A, Heindl T, Skrobol C, Wieser J, Krücken R, Ulrich A, 2008 Eur. Phys. J. D 48 383
Google Scholar
[9] Masoud N, Martus K, Murnick D 2019 Plasma Sources Sci. Technol. 28 045010
Google Scholar
[10] Zhu B L, Yan S Q, Chen Y, Zhu X D 2022 Plasma Sources Sci. Technol. 31 025012
Google Scholar
[11] Pei X K, Zhang P, Chen K, Lu X P 2013 IEEE Trans. Plasma Sci. 41 494
Google Scholar
[12] Yan S Q, Chen Y, Ma Y, Gao J K, Zhu X D 2023 Phys. Plasmas 30 073504
Google Scholar
[13] Shimizu R, Ding Z J 1992 Rep. Prog. Phys. 55 487
Google Scholar
[14] Zhu B L, Ma Y, Yan S Q, Ke W, Yang K, Zhu X D 2020 J. Appl. Phys. 128 135302
Google Scholar
[15] 蒲其荣, 丁泽军, 孙霞, 吴自勤 2004 电子显微学报 23 571
Google Scholar
Pu Q R, Ding Z J, Sun X, Wu Z Q 2004 J. Chin. Electr. Microsc. Soc. 23 571
Google Scholar
[16] Seltzer S M, Berger M J 1982 Int. J. Appl. Radiat. Isot. 33 1189
Google Scholar
[17] Hoang H, Røed K, Bekkeng T A, Moen J I, Spicher A, Clausen L B N, Miloch W J, Trondsen E, Pedersen A 2018 Meas. Sci. Technol. 29 065906
Google Scholar
[18] Morozov A, Krücken R, Ulrich A, Wieser J 2006 J. Appl. Phys. 100 093305
Google Scholar
[19] Walton S G, Boris D R, Hernández S C, Lock E H, Petrova Tz B, Petrov G M, Fernsler R F 2015 ECS J. Solid State Sci. Technol. 4 N5033
Google Scholar
[20] Cohn A, Caledonia G 1970 J. Appl. Phys. 41 3767
Google Scholar
[21] Soloviev V, Konchakov A, Krivstov V, Malmuth N 2001 32nd AIAA Plasmadynamics and Lasers Conference Anaheim, CA, U. S. A. June 2001 AIAA 2001–3089
[22] Bai X Y, Chen C, Li H, Liu W D, Chen W 2017 Phys. Plasmas 24 103509
Google Scholar
下载:
计量
- 文章访问数: 1277
- PDF下载量: 34
- 被引次数: 0
