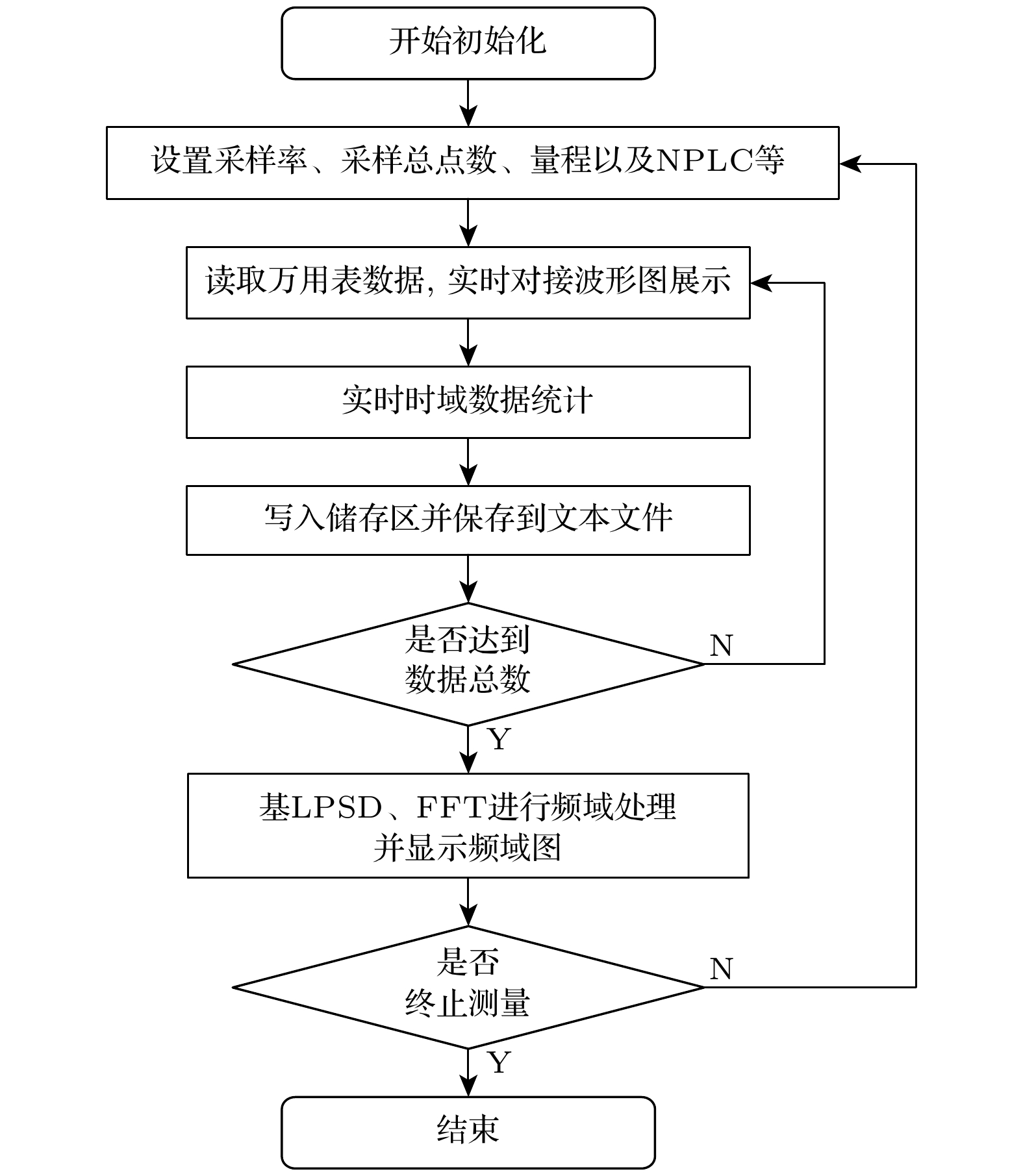
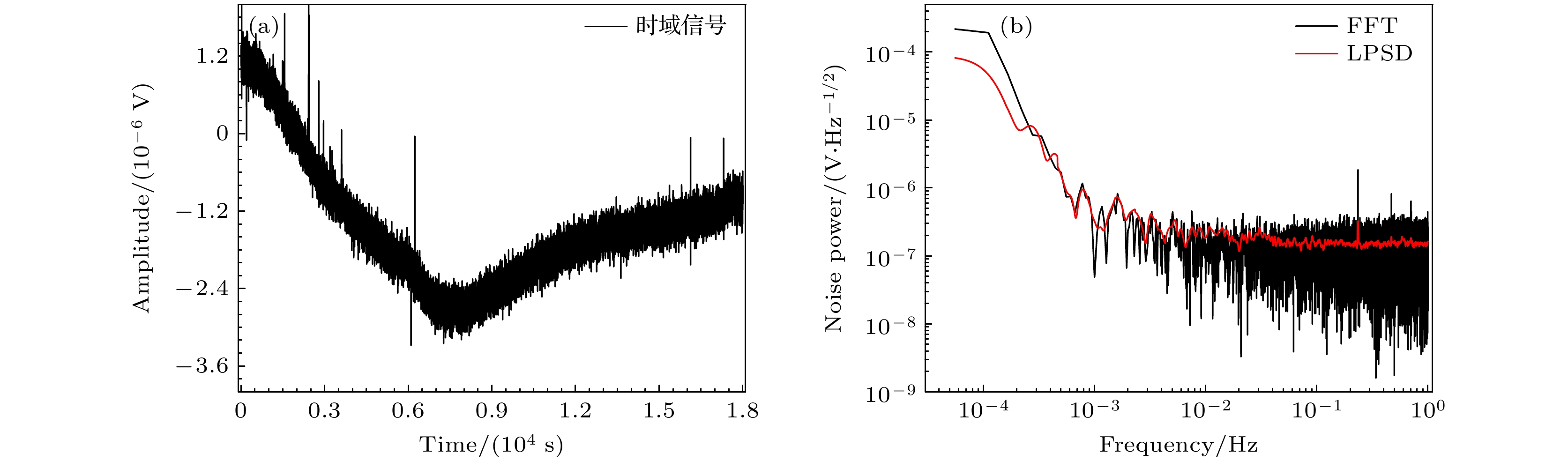
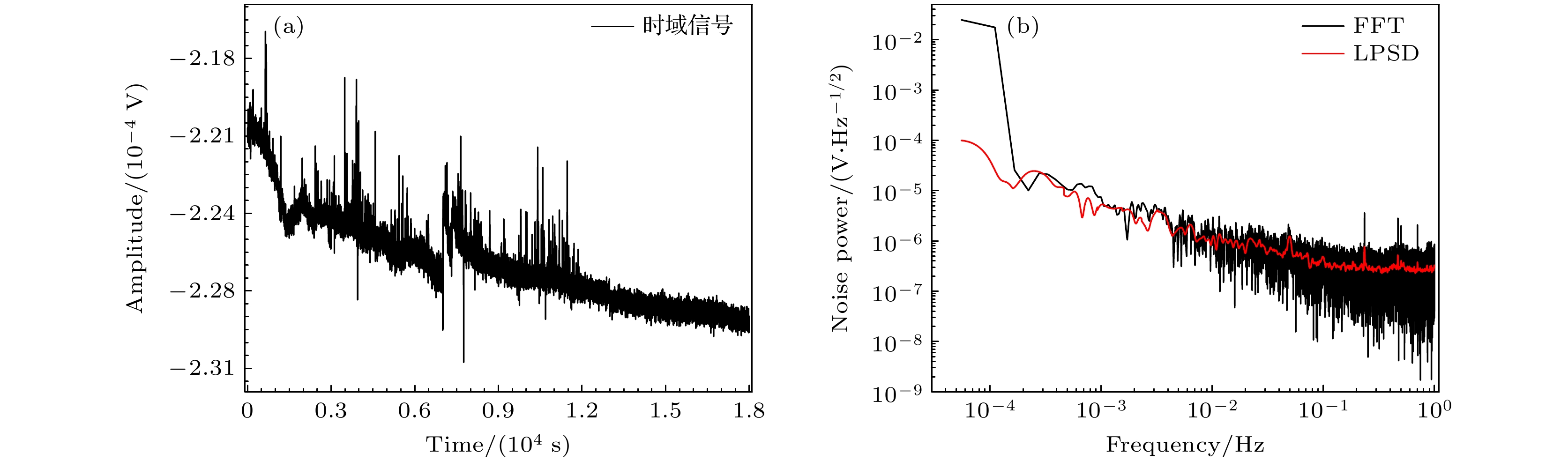
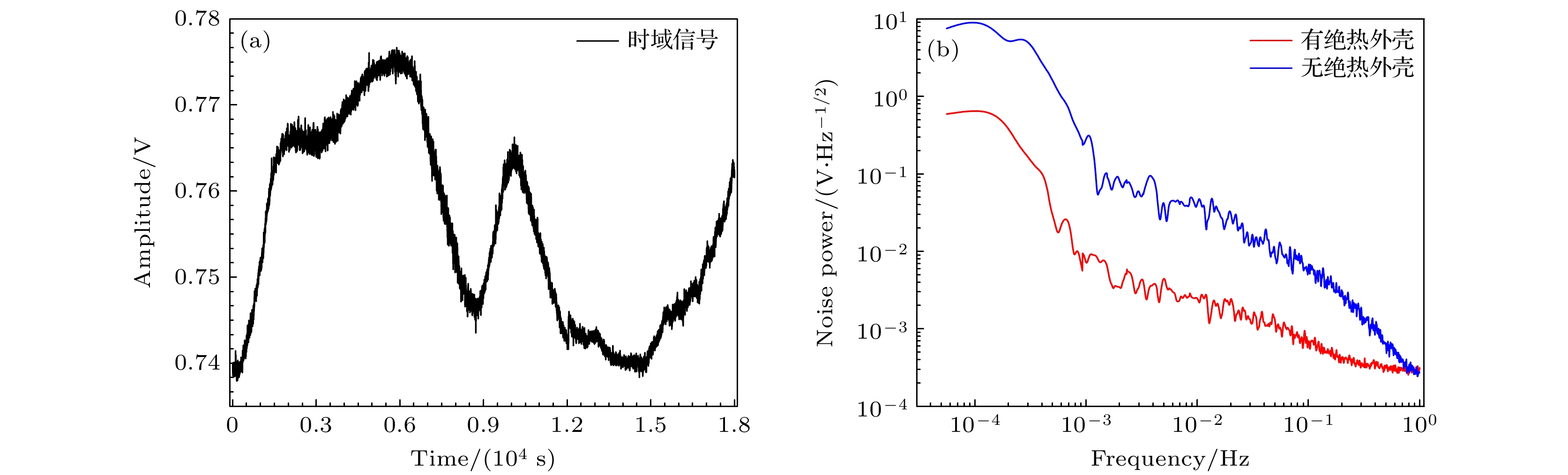
-
空间引力波探测的波源特征面向更大特征质量和尺度的引力波源信息, 与地基引力波探测、原初引力波探测、脉冲星引力波探测等形成互补探测方案. 空间引力波探测基于长距离激光干涉装置, 主要探测0.1 mHz—1 Hz频段范围内的引力波信号, 由于空间引力波探测装置的灵敏度直接受到激光光源噪声的影响, 为满足空间引力波探测的要求, 就需要对极低频段激光强度噪声进行评估与表征. 本文基于低噪声光电探测、高精度数字万用表操控以及对数频率轴功率谱密度估计算法编程, 构建极低频段激光强度噪声测试评估系统. 实验结果表明, 在0.1 mHz—1 Hz频段高精度万用表的电子学噪声低于5×10–5 V/Hz1/2, 探测器电子学噪声低于4×10–5 V/Hz1/2, 高精度万用表及探测器的电子学噪声均低于我国空间引力波探测计划中对激光光源强度噪声的要求. 本文中构建的0.1 mHz—1 Hz频段激光强度噪声评估系统满足了我国空间引力波探测计划对激光强度噪声评估的需求, 为空间引力波探测中激光光源噪声评估及噪声抑制奠定了重要基础.
-
关键词:
- 空间引力波探测 /
- 激光强度噪声 /
- 对数频率轴功率谱密度估计算法 /
- 噪声评估系统
The space-based gravitational wave detection can acquire the gravitational wave source information with larger characteristic mass and scale, forming a complementary detection scheme with ground-based gravitational wave detection, primordial gravitational wave detection, and pulsar gravitational wave detection. The space-based gravitational wave detection is based on a long-distance laser interference device, which mainly detects gravitational wave signals in a frequency range of 0.1 mHz–1 Hz. The noise evaluation and noise suppression of the laser light source system directly affect the detection sensitivity. In this work, based on low-noise photoelectric detection, a very low-frequency laser intensity noise test and evaluation system is constructed with high-precision digital multimeter, software control and algorithm programming of the host computer. The laser intensity noise can be converted into the fluctuation of the current signal by utilizing the photodiode, and the current signal is converted into the voltage signal and amplified by the transimpedance circuit. Thus the high-frequency interference components are filtered out by a passive low-pass filtering, and the extremely low-frequency noise components are retained. According to the definition of shot noise, it can be known that the photocurrent injected into the detector is inversely proportional to the shot noise, so at least 5 mW laser is chosen for photoelectric detection. After controlling the high-precision digital multimeter through LabVIEW software programming, the acquisition is detected. The output voltage signal by the laser is subjected to the fast Fourier transform and logarithmic frequency axis power spectral density estimation algorithm for noise evaluation in the frequency domain, forming a complete laser intensity noise evaluation and measurement system. The 0.1 mHz–1 Hz frequency band laser intensity noise evaluation is finally obtained. The experimental results show that the noise of the high-precision multimeter in a frequency band of 0.1 mHz–1 Hz is lower than 5×10–5 V/Hz1/2; the noise of the detector electronics ina frequency band of 0.1 mHz–1 Hz is lower than 4×10–5 V/Hz1/2. The electronic noise of the high-precision multimeters and the detectors meet the requirements for space gravitational wave detection. The experimental results show that the 0.1 mHz–1 Hz frequency band laser intensity noise evaluation system we built meets the needs of space-based gravitational wave detection program, and provides an important foundation for building a laser source that meets the needs of space-based gravitational wave detection.-
Keywords:
- space-based gravitational wave detection /
- laser intensity noise /
- logarithmic frequency axis power spectral density /
- noise evaluation system
[1] Sathyaprakash B S, Schutz B F 2009 Living Rev. Relativ. 12 2
Google Scholar
[2] Abbott B P, Abbott R, Abbott T D, et al. 2016 Phys. Rev. Lett. 116 061102
Google Scholar
[3] ESA-SCI 2000 LISA: System and Technology Study Report. ESA-SCI 11 2
[4] Black E D, Gutenkunst R N 2003 Am. J. Phys. 71 365
Google Scholar
[5] ESA-SCI 2000 LISA: System and Technology Study Report. ESA-SCI 11 76
[6] Jennrich O 2009 Classical Quantum Gravity 26 153001
Google Scholar
[7] Bender P, Brillet A, Ciufolini I, Cruise A M, Cutler C, Danzmann K, Fidecaro F, Folkner W M, Hough J, McNamara P, Peterseim M, Robertson D, Rodrigues M, Rüdiger A, Sandford M, Schäfer G, Schilling R, Schutz B, Speake C, Stebbins R T, Sumner T, Touboul P, Vinet J Y, Vitale S, Ward H, Winkler W 1998 LISA pre-phase a report. Max Planck Institute for Quantum Optics, Garching 1998 p1
[8] Armano M, Audley H, Auger G, et al. 2016 Phys. Rev. Lett. 116 231101
Google Scholar
[9] Araújo H, Boatella C, Chmeissani M, Conchillo A, García-Berro E, Grimani C, Hajdas W, Lobo A, Martínez Ll, Nofrarias M, Ortega J A, Puigdengoles C, Ramos-Castro J, Sanjuán J, Wass P, Xirgu X 2007 J. Phys. Conf. Ser. 66 012003
Google Scholar
[10] 罗子人, 白姗, 边星, 陈葛瑞, 董鹏, 董玉辉, 高伟, 龚雪飞, 贺建武, 李洪银, 李向前, 李玉琼, 刘河山, 邵明学, 宋同消, 孙保三, 唐文林, 徐鹏, 徐生年, 杨然, 靳刚 2013 力学进展 43 415
Google Scholar
Luo Z R, Bai S, Bian X, Chen G R, Dong P, Dong Y H, Gao W, Gong X F, He J W, Li H Y, Li X Q, Li Y Q, Liu H S, Shao M X, Song T X, Sun B S, Tang W L, Xu P, Xu S N, Yang R, Jin G 2013 Adv. Mech. 43 415
Google Scholar
[11] 罗子人, 张敏, 靳刚, 吴岳良, 胡文瑞 2020 深空探测学报 7 3
Luo Z R, Zhang M, Jin G, Wu Y L, Hu W R 2020 J. Deep Space Explor. 7 3
[12] 王璐钰, 李玉琼, 蔡榕 2021 中国光学 14 1426
Google Scholar
Wang L Y, Li Y Q, Cai R 2021 Chin. Opt. 14 1426
Google Scholar
[13] 王智, 沙巍, 陈哲, 王永宪, 康玉思, 罗子人, 黎明, 李钰鹏 2018 中国光学 11 131
Google Scholar
Wang Z, Sha W, Chen Z, Wang Y X, Kang Y S, Luo Z R, Li M, Li Y P 2018 Chin. Opt. 11 131
Google Scholar
[14] 刘河山, 高瑞弘, 罗子人, 靳刚 2019 中国光学 12 486
Google Scholar
Liu H S, Gao R H, Luo Z R, Jin G 2019 Chin. Opt. 12 486
Google Scholar
[15] Dahl K, Cebeci P, Fitzau O, Giesberts M, Greve C, Krutzik M, Peters A, Pyka S A, Sanjuan J, Schiemangk M, Schuldt T, Voss K, Wicht A 2018 International Conference on Space Optics—ICSO 2018, Chania Greece, October 9–12, 2018 111800C-2
[16] 刘宝洲 2022 电子测量技术 43 76
Liu B Z 2022 Electron. Meas. Technol. 43 76
[17] Cooley J W, Tukey J W 1965 Math. Comput. 19 297
Google Scholar
[18] Welch P D 1967 IEEE Trans. Audio Electroacoust. 15 70
Google Scholar
[19] Tröbs M, Heinzel G 2006 Measurement 39 120
Google Scholar
[20] Zhou H J, Wang W Z, Chen C Y, Zheng Y H 2015 IEEE Sens. J. 15 2101
Google Scholar
[21] 刘奎, 杨荣国, 张海龙, 白云飞, 张俊香, 郜江瑞 2009 中国激光 36 1852
Google Scholar
Liu K, Yang R G, Zhang H L 2009 Chinese Journal of Lasers 36 1852
Google Scholar
[22] 王雅君, 高丽, 张晓莉 2020 红外与激光工程 49 20201073
Google Scholar
Wang Y J, Gao L, Zhang X L 2020 Infrared Laser Eng. 49 20201073
Google Scholar
[23] Goßler S, Bertolini A, Born M, Chen Y, Dahl K, Gering D, Gräf C, Heinzel G, Hild S, Kawazoe F, Kranz O, Kühn G, Lück H, Mossavi K, Schnabel R, Somiya K, Strain K A, Taylor J R, Wanner A, Westphal T, Willke B, Danzmann K 2010 Classical Quantum Gravity 27 084023
Google Scholar
[24] Tröbs M 2005 Ph. D. Dissertation (Hannover: Leibniz University Hannover)
[25] Jerri A J 1977 Proc. IEEE 65 1565
Google Scholar
[26] Higgins J R 1985 Bull. Amer. Math. Soc. 12 45
Google Scholar
[27] 曹敏, 毕志周, 李波, 李毅, 王昕, 石少岩, 梁钻仁, 刘畅 2013 电子器件 36 371
Google Scholar
Cao M, Bi Z Z, Li B, Wang X, Shi S Y, Liang Z R, Liu C 2013 Chin. Electron Devices 36 371
Google Scholar
[28] 王俊璞, 金志华 2008 计量技术 12 24
Wang J P, Jin Z H 2008 Meas. Tech. 12 24
[29] Junker J, Oppermann P, Willke B 2017 Opt. Lett. 42 755
Google Scholar
-
图 1 极低频段激光强度噪声评估系统架构
Fig. 1. Architecture of laser intensity noise measurement and evaluation system for space-based gravitational wave detection.
图 2 激光强度噪声评估系统程序流程图
Fig. 2. Program flow chart of laser intensity noise evaluation system.
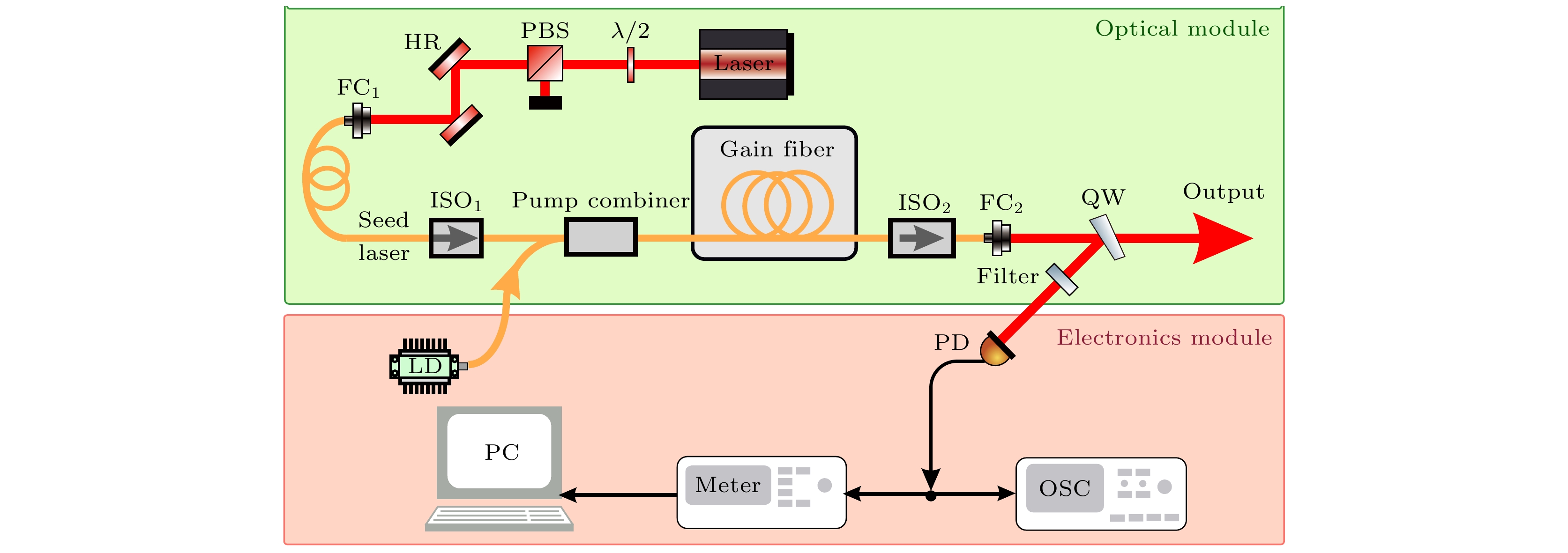
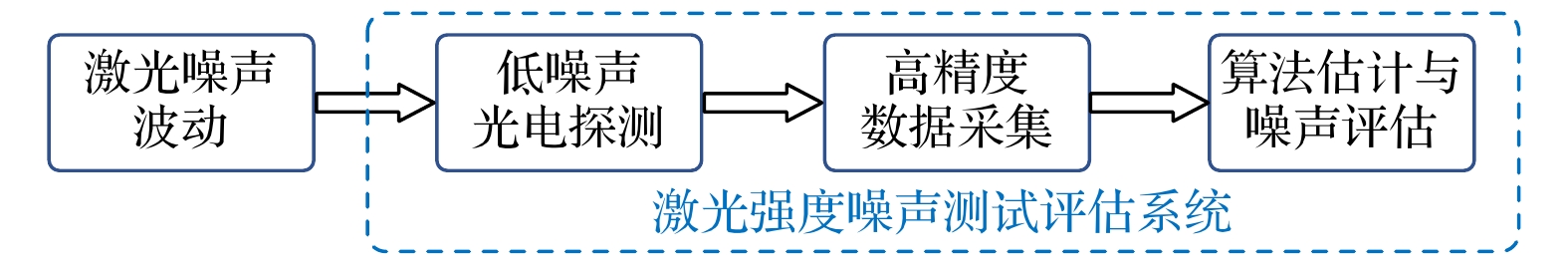
图 4 极低频段激光强度噪声评估系统(laser, 全固态激光器; pump combiner, 泵浦合束器; gain fiber, 增益光纤; LD, 半导体泵浦模块; λ/2, 半波片; PBS, 偏振分束器; ISO, 光隔离器; HR, 高反镜; FC1, 光纤耦合器; FC2, 光纤准直器; QW, 楔形分光镜; Filter, 衰减片; OSC, 示波器; PD, 光电探测器; meter, 高精度数字万用表)
Fig. 4. Evaluation system for laser intensity noise at ultra low frequency band. Laser, soild state laser; pump combiner, pump combiner; gain fiber, gain fiber; LD, semiconductor pump module; λ/2, half-wave-plate; PBS, polarization beam splitter; ISO, optical isolator; HR, high reflection mirror; FC, fiber coupler; QW, wedge beamsplitter; Filter, optical attenuator; OSC, oscilloscope; PD, photodetector; Meter, high-precision digital multimeter.
图 5 高精度万用表电子学噪声测试表征 (a) 时域数据结果; (b) 利用LPSD及FFT算法得到的噪声功率谱结果
Fig. 5. Electronic noise of the high-precision multimeter in the time domain (a) and spectral domain (b). The red and black lines in Figure (b) are spectrum estimations obtained by LPSD and FFT, respectively.
图 6 探测器电子学噪声测试表征 (a)时域数据结果; (b) 利用LPSD及FFT算法得到的噪声功率谱结果
Fig. 6. Electronic noise of the photodetector in the time domain (a) and spectral domain (b). The red and black lines in Figure (b) are spectrum estimations obtained by LPSD and FFT, respectively.
图 7 激光放大器自由运转时激光强度噪声测试表征 (a) 时域数据结果; (b) 有无绝热罩子情况下激光放大器输出激光的强度噪声功率谱结果
Fig. 7. Intensity noise of laser amplifier in the time domain (a) and spectral domain (b). The blue and red lines in Figure (b) are spectrum estimations with and without using adiabatic tank, respectively.
-
[1] Sathyaprakash B S, Schutz B F 2009 Living Rev. Relativ. 12 2
Google Scholar
[2] Abbott B P, Abbott R, Abbott T D, et al. 2016 Phys. Rev. Lett. 116 061102
Google Scholar
[3] ESA-SCI 2000 LISA: System and Technology Study Report. ESA-SCI 11 2
[4] Black E D, Gutenkunst R N 2003 Am. J. Phys. 71 365
Google Scholar
[5] ESA-SCI 2000 LISA: System and Technology Study Report. ESA-SCI 11 76
[6] Jennrich O 2009 Classical Quantum Gravity 26 153001
Google Scholar
[7] Bender P, Brillet A, Ciufolini I, Cruise A M, Cutler C, Danzmann K, Fidecaro F, Folkner W M, Hough J, McNamara P, Peterseim M, Robertson D, Rodrigues M, Rüdiger A, Sandford M, Schäfer G, Schilling R, Schutz B, Speake C, Stebbins R T, Sumner T, Touboul P, Vinet J Y, Vitale S, Ward H, Winkler W 1998 LISA pre-phase a report. Max Planck Institute for Quantum Optics, Garching 1998 p1
[8] Armano M, Audley H, Auger G, et al. 2016 Phys. Rev. Lett. 116 231101
Google Scholar
[9] Araújo H, Boatella C, Chmeissani M, Conchillo A, García-Berro E, Grimani C, Hajdas W, Lobo A, Martínez Ll, Nofrarias M, Ortega J A, Puigdengoles C, Ramos-Castro J, Sanjuán J, Wass P, Xirgu X 2007 J. Phys. Conf. Ser. 66 012003
Google Scholar
[10] 罗子人, 白姗, 边星, 陈葛瑞, 董鹏, 董玉辉, 高伟, 龚雪飞, 贺建武, 李洪银, 李向前, 李玉琼, 刘河山, 邵明学, 宋同消, 孙保三, 唐文林, 徐鹏, 徐生年, 杨然, 靳刚 2013 力学进展 43 415
Google Scholar
Luo Z R, Bai S, Bian X, Chen G R, Dong P, Dong Y H, Gao W, Gong X F, He J W, Li H Y, Li X Q, Li Y Q, Liu H S, Shao M X, Song T X, Sun B S, Tang W L, Xu P, Xu S N, Yang R, Jin G 2013 Adv. Mech. 43 415
Google Scholar
[11] 罗子人, 张敏, 靳刚, 吴岳良, 胡文瑞 2020 深空探测学报 7 3
Luo Z R, Zhang M, Jin G, Wu Y L, Hu W R 2020 J. Deep Space Explor. 7 3
[12] 王璐钰, 李玉琼, 蔡榕 2021 中国光学 14 1426
Google Scholar
Wang L Y, Li Y Q, Cai R 2021 Chin. Opt. 14 1426
Google Scholar
[13] 王智, 沙巍, 陈哲, 王永宪, 康玉思, 罗子人, 黎明, 李钰鹏 2018 中国光学 11 131
Google Scholar
Wang Z, Sha W, Chen Z, Wang Y X, Kang Y S, Luo Z R, Li M, Li Y P 2018 Chin. Opt. 11 131
Google Scholar
[14] 刘河山, 高瑞弘, 罗子人, 靳刚 2019 中国光学 12 486
Google Scholar
Liu H S, Gao R H, Luo Z R, Jin G 2019 Chin. Opt. 12 486
Google Scholar
[15] Dahl K, Cebeci P, Fitzau O, Giesberts M, Greve C, Krutzik M, Peters A, Pyka S A, Sanjuan J, Schiemangk M, Schuldt T, Voss K, Wicht A 2018 International Conference on Space Optics—ICSO 2018, Chania Greece, October 9–12, 2018 111800C-2
[16] 刘宝洲 2022 电子测量技术 43 76
Liu B Z 2022 Electron. Meas. Technol. 43 76
[17] Cooley J W, Tukey J W 1965 Math. Comput. 19 297
Google Scholar
[18] Welch P D 1967 IEEE Trans. Audio Electroacoust. 15 70
Google Scholar
[19] Tröbs M, Heinzel G 2006 Measurement 39 120
Google Scholar
[20] Zhou H J, Wang W Z, Chen C Y, Zheng Y H 2015 IEEE Sens. J. 15 2101
Google Scholar
[21] 刘奎, 杨荣国, 张海龙, 白云飞, 张俊香, 郜江瑞 2009 中国激光 36 1852
Google Scholar
Liu K, Yang R G, Zhang H L 2009 Chinese Journal of Lasers 36 1852
Google Scholar
[22] 王雅君, 高丽, 张晓莉 2020 红外与激光工程 49 20201073
Google Scholar
Wang Y J, Gao L, Zhang X L 2020 Infrared Laser Eng. 49 20201073
Google Scholar
[23] Goßler S, Bertolini A, Born M, Chen Y, Dahl K, Gering D, Gräf C, Heinzel G, Hild S, Kawazoe F, Kranz O, Kühn G, Lück H, Mossavi K, Schnabel R, Somiya K, Strain K A, Taylor J R, Wanner A, Westphal T, Willke B, Danzmann K 2010 Classical Quantum Gravity 27 084023
Google Scholar
[24] Tröbs M 2005 Ph. D. Dissertation (Hannover: Leibniz University Hannover)
[25] Jerri A J 1977 Proc. IEEE 65 1565
Google Scholar
[26] Higgins J R 1985 Bull. Amer. Math. Soc. 12 45
Google Scholar
[27] 曹敏, 毕志周, 李波, 李毅, 王昕, 石少岩, 梁钻仁, 刘畅 2013 电子器件 36 371
Google Scholar
Cao M, Bi Z Z, Li B, Wang X, Shi S Y, Liang Z R, Liu C 2013 Chin. Electron Devices 36 371
Google Scholar
[28] 王俊璞, 金志华 2008 计量技术 12 24
Wang J P, Jin Z H 2008 Meas. Tech. 12 24
[29] Junker J, Oppermann P, Willke B 2017 Opt. Lett. 42 755
Google Scholar
下载:
计量
- 文章访问数: 5116
- PDF下载量: 211
- 被引次数: 0
