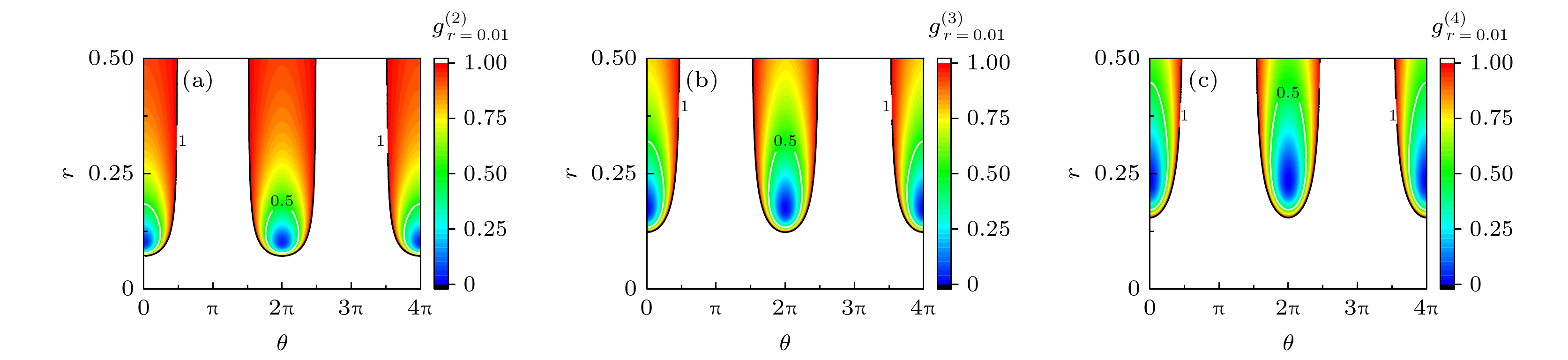
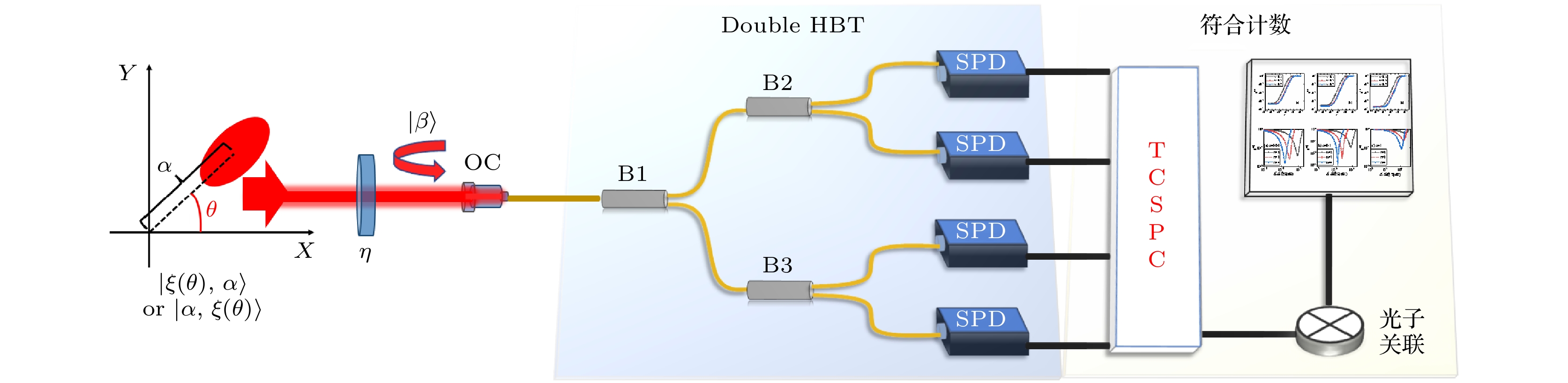
-
基于扩展的Hanbury Brown-Twiss方案研究相位可变压缩相干态的高阶光子关联及反聚束效应. 通过调控压缩参数r、平移α和压缩相位θ, 压缩相干态的高阶光子关联呈明显的反聚束效应. 在压缩相位θ∈[0,π/2]范围内, 较大α-r参数区间都可获得光场的高阶反聚束效应, 理想情况下最小的反聚束值为g(4) = 6.6352 × 10–5. 研究了背景噪声γ和系统探测效率η对高阶光子反聚束的影响, 在较低探测效率η = 0.1, 背景噪声γ = 10–6时, 仍可获得明显的高阶反聚束效应g(4) = 0.0149, 验证了更高阶光子关联的反聚束效应对实验环境具有较强的鲁棒性. 此外, 研究了相位可变压缩相干态的反聚束效应随探测平均光子数
$\langle$ n$\rangle $ 和压缩度S的变化, 在探测平均光子数远小于1、压缩参数10–4以下时, 仍可得到g(n)$\ll $ 0.5的显著的光子反聚束效应. 结果表明利用对压缩相位θ的调控可制备具有明显反聚束效应的压缩相干态, 在量子精密测量及保密通信领域有着潜在的重要应用.Squeezed state has important applications in quantum communication, quantum computing, and precision measurement. It has been used to improve the sensitivity and measurement accuracy of gravitational wave detectors. Currently, squeezed state can be prepared by optical parametric oscillators, four-wave mixing, and atom–optomechanical coupling. As a typical non-classical light, the photon statistics of squeezed state usually shows obvious bunching effect, but it can also present photon antibunching effect through interference or photon subtraction operation. More importantly, squeezed coherent state is prepared by performing displacement operation on the squeezed state. In the case of certain displacement and squeezing operations, squeezed coherent state with obvious antibunching effect can be produced. The squeezed coherent state with photon antibunching effect can be employed to achieve super-resolution imaging beyond the diffraction limit, and the state exhibits good particle features which can suppress the multiphoton emission. Then it has become a focus for studying the antibunching effect and quantum statistical properties of squeezed coherent state at a single-photon level. The photon antibunching effect can be characterized by the second-order photon correlation g(2)(τ), which is introduced by Glauber to determine the non-classical properties of the light field. Namely, the second-order photon correlation g(2) can be used as a metric to distinguish different lights. Hanbury Brown-Twiss (HBT) scheme is used to measure the second-order photon correlation experimentally. However, the second-order photon correlation g(2) can reflect only the variance of the photon-number statistical distribution. In order to obtain more information about the photon statistical distribution and non-classical features, it is necessary to measure higher-order photon correlations. Then the higher-order photon correlations for different light fields are studied by extending the traditional HBT scheme and combining with multiplex single-photon detection technology. This method can be applied to ghost imaging, characterization of single-photon detectors, research of exciton dynamics, and analysis of NV center fluorescence emission. However, the research on photon statistics of the squeezed state focuses mainly on the second-order photon correlation and the effect of displacement amplitude on the statistical properties. The effect of squeezed phase on photon antibunching and higher-order photon correlation of squeezed coherent states, with background noise and detection efficiency taken into consideration, have not been investigated. In this paper, we study high-order photon correlations and antibunching effect of phase-variable squeezed coherent state based on an extended HBT scheme. The photon statistics of the squeezed coherent state manifests prominent antibunching effect by adjusting the squeezing parameter r, displacement amplitude α and squeezing phase θ. The antibunching effect of the state can be obtained in a wide range of α-r parameter space when squeezing phase θ∈[0,π/2]. In an ideal case, the minimum antibunching values of the squeezed coherent state are g(2) = 4.006 × 10–4, g(3) = 1.3594 × 10–4 and g(4) = 6.6352 × 10–5. When the detection efficiency η = 0.1 and background noise γ = 10–6, the strong antibunching effect can still be observed, specifically, g(2) = 0.1740, g(3) = 0.0432, g(4) = 0.0149. The results indicate that the antibunching effect of higher-order photon correlation has strong robustness against the experimental environment. In addition, the antibunching effect of the phase-variable squeezed coherent state is studied as a function of the measured mean photon number <n> and the squeezing degree S. When the measured mean photon number is much less than 1 and the squeezing parameter is less than 10–4, a prominent photon anti-bunching effect of g(n) $\ll $ 0.5 can still be obtained. The results show that the control of the squeezing phase θ can be used to prepare the squeezed coherent state with obvious antibunching effect, which has potentially important applications in quantum metrology and secure communication.-
Keywords:
- high-order photon correlation /
- squeezed coherent state /
- squeezing phase /
- antibunching effect
[1] Tian C X, Han D M, Wang Y, and Su X L 2018 Opt. Express 26 29159
Google Scholar
[2] Su X L, Wang M H, Yan Z H, Jia X J, Xie C D, Peng K C 2020 Sci. China Inf. Sci. 63 180503
Google Scholar
[3] Zhong H S, Wang H, Deng Y H, et al. 2020 Science 370 1460
Google Scholar
[4] Hamilton C S, Kruse R, Sansoni L, Barkhofen S, Silberhorn C, Jex L 2017 Phys. Rev. Lett. 119 170501
Google Scholar
[5] 孙恒信, 刘奎, 张俊香, 郜江瑞 2015 64 234210
Google Scholar
Sun H X, Liu K, Zhang J X, Gao J R 2015 Acta Phys. Sin. 64 234210
Google Scholar
[6] Casacio C A, Madsen L S, Terrasson A, Waleed M, Barnscheidt K, Hage B, Taylor M A, Bowen W P 2021 Nature 594 201
Google Scholar
[7] McCuller L, Whittle C, Ganapathy D, et al. 2020 Phys. Rev. Lett. 124 171102
Google Scholar
[8] Lough J, Schreiber E, Bergamin F, et al. 2021 Phys. Rev. Lett. 126 041102
Google Scholar
[9] Vahlbruch H, Mehmet M, Danzmann K, Schnabel R 2016 Phys. Rev. Lett. 117 110801
Google Scholar
[10] Guerrero A. M, Nussenzveig P, Martinelli M, Marino A M, Florez H M 2020 Phys. Rev. Lett. 125 083601
Google Scholar
[11] Ma L, Guo H, Sun H X, Liu K, Su B D, Gao J R 2020 Photon. Res. 8 1422
Google Scholar
[12] 左冠华, 杨晨, 赵俊祥, 田壮壮, 朱诗尧, 张玉驰, 张天才 2020 69 014207
Google Scholar
Zuo G H, Yang C, Zhao J X, Tian Z Z, Zhu S Y, Zhang Y C, Zhang T C 2020 Acta Phys. Sin. 69 014207
Google Scholar
[13] 李庆回, 姚文秀, 李番, 田龙, 王雅君, 郑耀辉 2021 70 154203
Google Scholar
Li Q H, Yao W X, Li F, Tian L, Wang Y J, Zheng Y H 2021 Acta Phys. Sin. 70 154203
Google Scholar
[14] Liu S S, Lou Y B, Jing J T 2019 Phys. Rev. Lett. 123 113602
Google Scholar
[15] Zhao Y, Okawachi Y, Jang J K, Ji X C, Lipson M, Gaeta A L 2020 Phys. Rev. Lett. 124 193601
Google Scholar
[16] Guo Y Q, Guo X M, Li P, Shen H, Zhang J, Zhang T C 2018 Ann. Phys. 530 1800138
Google Scholar
[17] Zhang Y, Menotti M, Tan K, Vaidya V D, Mahler D H, Helt L G, Zatti L, Liscidini M, Morrison B, Vernon Z 2021 Nat. Commun. 12 2233
Google Scholar
[18] Lemonde M A, Didier N, Clerk A A 2014 Phys. Rev. A 90 063824
Google Scholar
[19] Jiang K, Wei L F 2021 Phys. Lett. A 403 127396
Google Scholar
[20] Grosse N B, Symul T, Stobinska M, Ralph T C, Lam P K, 2007 Phys. Rev. Lett. 98 153603
Google Scholar
[21] Han Y S, Wu D H, Kasai K, Wang L R, Watanabe M, Zhang Y 2020 J. Opt. 22 025202
Google Scholar
[22] Schwartz O, Oron D 2012 Phys. Rev. A 85 033812
Google Scholar
[23] Schwartz, O, Levitt J M, Tenne, Ron, Itzhakov S, Deutsch, Z, Oron D 2013 Nano Lett. 13 5832
Google Scholar
[24] Matsuoka M, Hirano T 2003 Phys. Rev. A 67 042307
Google Scholar
[25] Wang Q, Wang X B, Guo G C 2007 Phys. Rev. A 75 012312
Google Scholar
[26] Glauber R J 1963 Phys. Rev. 130 2529
Google Scholar
[27] Glauber R J 1963 Phys. Rev. 131 2766
Google Scholar
[28] 兰豆豆, 郭晓敏, 彭春生, 姬玉林, 刘香莲, 李璞, 郭龑强 2017 66 120502
Google Scholar
Lan D D, Guo X M, Peng C S, Ji Y L, Liu X L, Li P, Guo Y Q 2017 Acta Phys. Sin. 66 120502
Google Scholar
[29] Guo Y Q, Peng C S, Ji Y L, Li P, Guo Y Y, Guo X M 2018 Opt. Express 26 5991
Google Scholar
[30] Guo X M, Cheng C, Liu T, Fang X, Guo Y Q 2019 Appl. Sci. 9 4907
Google Scholar
[31] Luo S, Zhou Y, Zheng H B, Liu J B, Chen H, He Y C, Xu W T, Zhang S H, Li F L, Xu Z 2021 Phys. Rew. A 103 013723
Google Scholar
[32] Li J M, Su J, Cui L, Xie T Q, Ou Z Y, Li X Y 2020 Appl. Phys. Lett. 116 204002
[33] Guo Y Q, Yang R C, Li G, Zhang P F, Zhang Y C, Wang J M, Zhang T C 2011 J. Phys. B At. Mol. Opt. Phys. 44 205502
Google Scholar
[34] Guo Y Q, Wang L J, Wang Y, Fang X, Zhao T, Guo X M, Zhang T C 2020 J. Opt. 22 095202
Google Scholar
[35] Hong P L, Li L M, Liu J J, Zhang G Q 2016 Sci. Rep. 6 23614
Google Scholar
[36] Nieves Y, Muller A 2020 Phys. Rev. B 102 155418
Google Scholar
[37] Zhou Y, Simon J, Liu J B, Shih Y H 2010 Phys. Rev. A 81 043831
Google Scholar
[38] Chen X H, Wu S S, Wu W, Guo W Y, Meng S Y, Sun Z B, Zhai G J, Li M F, Wu L A 2014 J. Opt. Soc. Am. A 31 2105
Google Scholar
[39] Hodgman S S, Bu W, Mann S B, Khakimov R I, Truscott A G 2019 Phys. Rew. Lett. 122 233601
Google Scholar
[40] Wayne M A, Bienfang J C, Polyakov S V 2017 Opt. Express 25 20352
Google Scholar
[41] Amgar D, Yang G L, Tenne R, Oron D 2019 Nano Lett. 19 8741
Google Scholar
[42] Tang H J, Ahmed I, Puttapirat P, Wu T H, Lan Y W, Zhang Y P, Li E L 2018 Phys. Chem. Chem. Phys. 20 5721
Google Scholar
[43] Lu Y J, Ou Z Y 2002 Phys. Rev. Lett. 88 023601
[44] Boddeda R, Glorieux Q, Bramati A, Pigeon S 2019 J. Phys. B:At. Mol. Opt. Phys. 52 215401
Google Scholar
[45] Shih C C 1986 Phys. Rev. D 34 2720
Google Scholar
[46] Campos R A, Saleh B E A, Teich M C 1989 Phys. Rev. A 40 1371
Google Scholar
-
图 1 测量相位可变压缩相干态高阶光子关联的双HBT原理示意图
Fig. 1. Schematic diagram of double HBT model for measuring high-order photon correlation of phase-variable squeezed state.
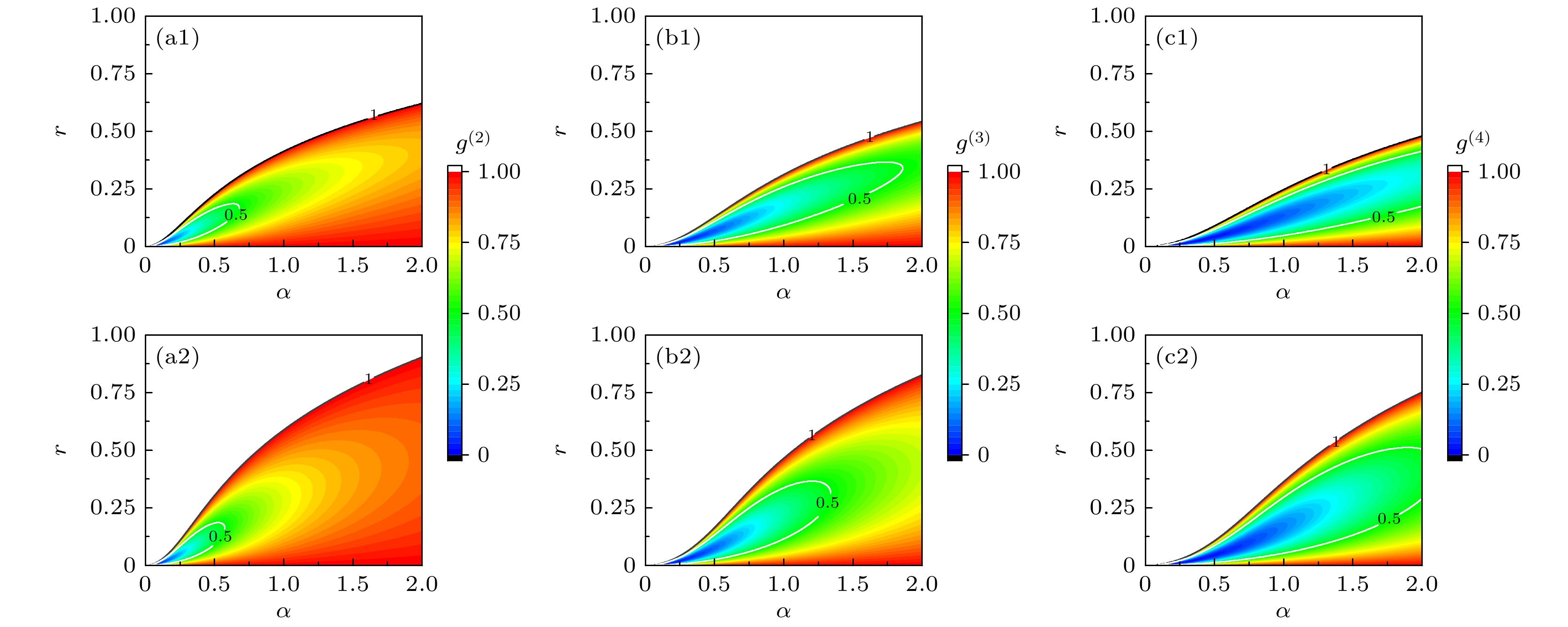
图 2 压缩相位为θ = 0时, 压缩相干态 (a1)—(c1) |ξ(θ), α
$\rangle $ 和(a2)—(c2) |α, ξ(θ)$\rangle $ 的高阶光子关联随平移振幅α和压缩参数r变化的反聚束结果Fig. 2. High-order photon antibunching of squeezed coherent states (a1)–(c1) |ξ(θ), α
$\rangle $ and (a2)–(c2) |α, ξ(θ)$\rangle $ versus displacement amplitude α and squeezing parameter r for squeezing phase θ = 0.
图 3 当平移振幅α = 0.1时, 相位可变压缩相干态高阶光子关联g(n)随压缩参数r和压缩相位θ变化的反聚束结果
Fig. 3. Photon antibunching results of high-order correlation g(n) of phase-variable squeezed coherent state versus squeezing parameter r and squeezing phase θ when displacement amplitude α = 0.1.
图 4 当压缩参数r = 0.01时, 相位可变压缩相干态的高阶光子关联g(n)随平移振幅α和压缩相位θ变化的反聚束结果
Fig. 4. Photon antibunching results of g(n) of phase-variable squeezed coherent state versus displacement amplitude α and squeezing phase θ when squeezing parameter r = 0.01.
图 5 探测效率分别为η = 0.1, 0.5和1时, 压缩态的(a)二阶、(b)三阶和(c)四阶光子关联最小值随背景噪声γ变化的结果
Fig. 5. Minimum second-order (a), third-order (b), and fourth-order (c) photon correlations of squeezed coherent state |ξ, α
$\rangle $ versus background noise γ when detection efficiencies η = 0.1, 0.5, and 1.
图 6 背景噪声γ = 10–6, 探测效率η = 0.5, 压缩度分别为 (a) r = 10–8 (8.6859 × 10–8 dB); (b) r = 10–4 (8.6859 × 10–4 dB); (c) r = 10–1 (8.6859 × 10–1 dB)时, 压缩相干态的g(n)随平均光子数
$\langle $ n$\rangle $ 的变化Fig. 6. g(n) of squeezed coherent state versus measured mean photon number
$\langle $ n$\rangle $ when background noise is γ = 10–6, detection efficiency is η = 0.5, and squeezing degrees are (a) r = 10–8 (8.6859 × 10–8 dB); (b) r = 10–4 (8.6859 × 10–4 dB); (c) r = 10–1 (8.6859 × 10–1 dB) respectively.
图 7 背景噪声γ = 10–6, 探测效率η = 0.5, 位移分别为 (a) α = 0.01, (b) α = 0.1和(c) α = 1时, 相位可变压缩相干态高阶光子关联g(n)随压缩度S的变化
Fig. 7. g(n) of phase-variable squeezed coherent state versus squeezing degree S when background noise is γ = 10–6, detection efficiency is η = 0.5, and displacement amplitudes are (a) α = 0.01, (b) α = 0.1, and (c) α = 1, respectively.
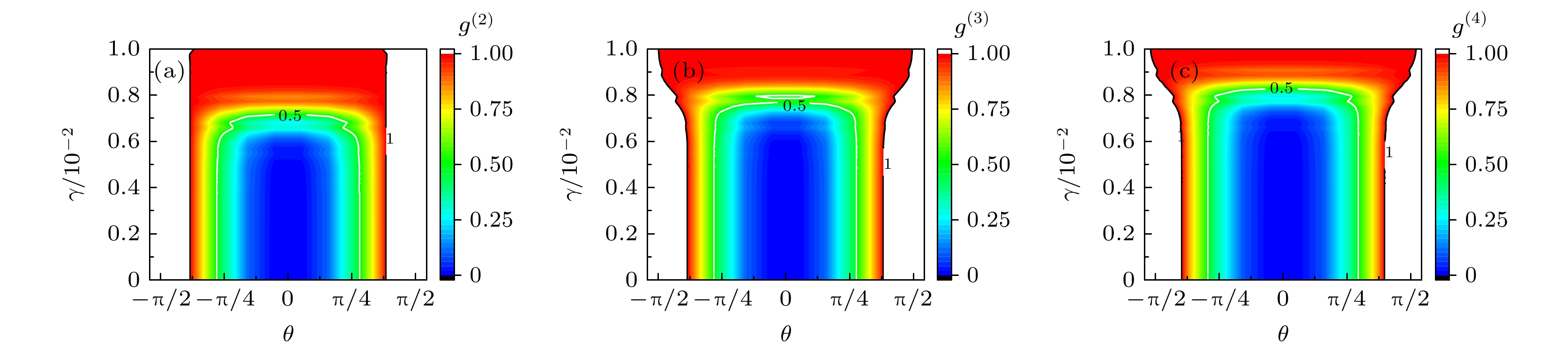
图 8 探测效率η = 0.5, 压缩参数r = 10–4, 平移振幅分别为 (a) α = 0.01, (b) α = 0.0173和(c) α = 0.0233时, 相位可变压缩相干态的高阶光子关联随压缩相位θ和背景噪声γ变化的分布图
Fig. 8. Maps of high-order photon correlations g(n) of phase-variable squeezed coherent state versus squeezing phase θ and background noise γ when detection efficiency is η = 0.5, squeezing parameter is r = 10–4 and displacement amplitudes are (a) α = 0.01, (b) α = 0.0173, and (c) α = 0.0233 respectively.
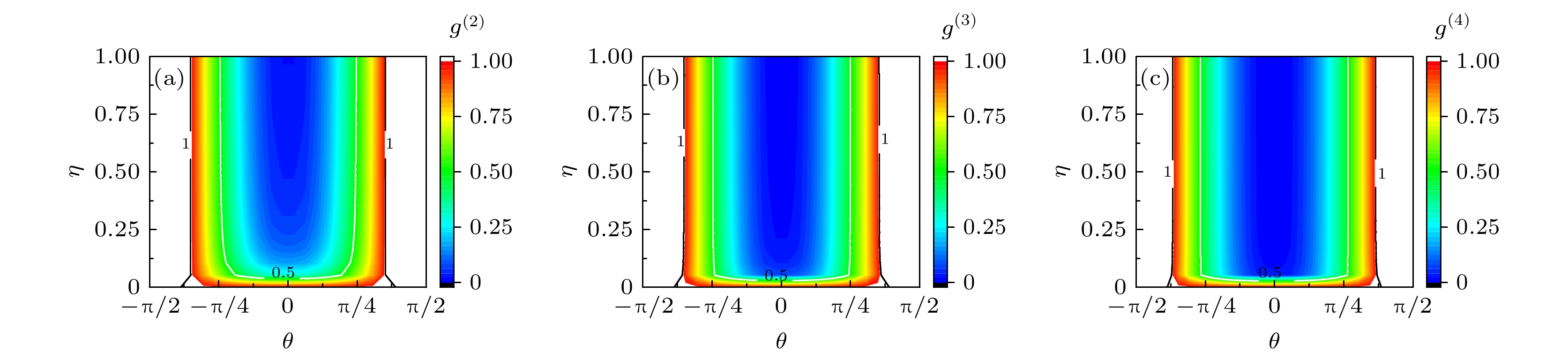
图 9 背景噪声γ = 10–6, 压缩参数r = 10–4, 平移振幅分别为 (a) α = 0.01, (b) α = 0.0173和(c) α = 0.0233时, 压缩相干态的高阶光子关联随压缩相位θ和探测效率η变化的分布图
Fig. 9. Maps of g(n) of the squeezed coherent state versus squeezing phase θ and detection efficiency η when background noise is γ = 10–6, squeezing parameter is r = 10–4 and displacement amplitudes are (a) α = 0.01, (b) α = 0.0173, and (c) α = 0.0233, respectively.
-
[1] Tian C X, Han D M, Wang Y, and Su X L 2018 Opt. Express 26 29159
Google Scholar
[2] Su X L, Wang M H, Yan Z H, Jia X J, Xie C D, Peng K C 2020 Sci. China Inf. Sci. 63 180503
Google Scholar
[3] Zhong H S, Wang H, Deng Y H, et al. 2020 Science 370 1460
Google Scholar
[4] Hamilton C S, Kruse R, Sansoni L, Barkhofen S, Silberhorn C, Jex L 2017 Phys. Rev. Lett. 119 170501
Google Scholar
[5] 孙恒信, 刘奎, 张俊香, 郜江瑞 2015 64 234210
Google Scholar
Sun H X, Liu K, Zhang J X, Gao J R 2015 Acta Phys. Sin. 64 234210
Google Scholar
[6] Casacio C A, Madsen L S, Terrasson A, Waleed M, Barnscheidt K, Hage B, Taylor M A, Bowen W P 2021 Nature 594 201
Google Scholar
[7] McCuller L, Whittle C, Ganapathy D, et al. 2020 Phys. Rev. Lett. 124 171102
Google Scholar
[8] Lough J, Schreiber E, Bergamin F, et al. 2021 Phys. Rev. Lett. 126 041102
Google Scholar
[9] Vahlbruch H, Mehmet M, Danzmann K, Schnabel R 2016 Phys. Rev. Lett. 117 110801
Google Scholar
[10] Guerrero A. M, Nussenzveig P, Martinelli M, Marino A M, Florez H M 2020 Phys. Rev. Lett. 125 083601
Google Scholar
[11] Ma L, Guo H, Sun H X, Liu K, Su B D, Gao J R 2020 Photon. Res. 8 1422
Google Scholar
[12] 左冠华, 杨晨, 赵俊祥, 田壮壮, 朱诗尧, 张玉驰, 张天才 2020 69 014207
Google Scholar
Zuo G H, Yang C, Zhao J X, Tian Z Z, Zhu S Y, Zhang Y C, Zhang T C 2020 Acta Phys. Sin. 69 014207
Google Scholar
[13] 李庆回, 姚文秀, 李番, 田龙, 王雅君, 郑耀辉 2021 70 154203
Google Scholar
Li Q H, Yao W X, Li F, Tian L, Wang Y J, Zheng Y H 2021 Acta Phys. Sin. 70 154203
Google Scholar
[14] Liu S S, Lou Y B, Jing J T 2019 Phys. Rev. Lett. 123 113602
Google Scholar
[15] Zhao Y, Okawachi Y, Jang J K, Ji X C, Lipson M, Gaeta A L 2020 Phys. Rev. Lett. 124 193601
Google Scholar
[16] Guo Y Q, Guo X M, Li P, Shen H, Zhang J, Zhang T C 2018 Ann. Phys. 530 1800138
Google Scholar
[17] Zhang Y, Menotti M, Tan K, Vaidya V D, Mahler D H, Helt L G, Zatti L, Liscidini M, Morrison B, Vernon Z 2021 Nat. Commun. 12 2233
Google Scholar
[18] Lemonde M A, Didier N, Clerk A A 2014 Phys. Rev. A 90 063824
Google Scholar
[19] Jiang K, Wei L F 2021 Phys. Lett. A 403 127396
Google Scholar
[20] Grosse N B, Symul T, Stobinska M, Ralph T C, Lam P K, 2007 Phys. Rev. Lett. 98 153603
Google Scholar
[21] Han Y S, Wu D H, Kasai K, Wang L R, Watanabe M, Zhang Y 2020 J. Opt. 22 025202
Google Scholar
[22] Schwartz O, Oron D 2012 Phys. Rev. A 85 033812
Google Scholar
[23] Schwartz, O, Levitt J M, Tenne, Ron, Itzhakov S, Deutsch, Z, Oron D 2013 Nano Lett. 13 5832
Google Scholar
[24] Matsuoka M, Hirano T 2003 Phys. Rev. A 67 042307
Google Scholar
[25] Wang Q, Wang X B, Guo G C 2007 Phys. Rev. A 75 012312
Google Scholar
[26] Glauber R J 1963 Phys. Rev. 130 2529
Google Scholar
[27] Glauber R J 1963 Phys. Rev. 131 2766
Google Scholar
[28] 兰豆豆, 郭晓敏, 彭春生, 姬玉林, 刘香莲, 李璞, 郭龑强 2017 66 120502
Google Scholar
Lan D D, Guo X M, Peng C S, Ji Y L, Liu X L, Li P, Guo Y Q 2017 Acta Phys. Sin. 66 120502
Google Scholar
[29] Guo Y Q, Peng C S, Ji Y L, Li P, Guo Y Y, Guo X M 2018 Opt. Express 26 5991
Google Scholar
[30] Guo X M, Cheng C, Liu T, Fang X, Guo Y Q 2019 Appl. Sci. 9 4907
Google Scholar
[31] Luo S, Zhou Y, Zheng H B, Liu J B, Chen H, He Y C, Xu W T, Zhang S H, Li F L, Xu Z 2021 Phys. Rew. A 103 013723
Google Scholar
[32] Li J M, Su J, Cui L, Xie T Q, Ou Z Y, Li X Y 2020 Appl. Phys. Lett. 116 204002
[33] Guo Y Q, Yang R C, Li G, Zhang P F, Zhang Y C, Wang J M, Zhang T C 2011 J. Phys. B At. Mol. Opt. Phys. 44 205502
Google Scholar
[34] Guo Y Q, Wang L J, Wang Y, Fang X, Zhao T, Guo X M, Zhang T C 2020 J. Opt. 22 095202
Google Scholar
[35] Hong P L, Li L M, Liu J J, Zhang G Q 2016 Sci. Rep. 6 23614
Google Scholar
[36] Nieves Y, Muller A 2020 Phys. Rev. B 102 155418
Google Scholar
[37] Zhou Y, Simon J, Liu J B, Shih Y H 2010 Phys. Rev. A 81 043831
Google Scholar
[38] Chen X H, Wu S S, Wu W, Guo W Y, Meng S Y, Sun Z B, Zhai G J, Li M F, Wu L A 2014 J. Opt. Soc. Am. A 31 2105
Google Scholar
[39] Hodgman S S, Bu W, Mann S B, Khakimov R I, Truscott A G 2019 Phys. Rew. Lett. 122 233601
Google Scholar
[40] Wayne M A, Bienfang J C, Polyakov S V 2017 Opt. Express 25 20352
Google Scholar
[41] Amgar D, Yang G L, Tenne R, Oron D 2019 Nano Lett. 19 8741
Google Scholar
[42] Tang H J, Ahmed I, Puttapirat P, Wu T H, Lan Y W, Zhang Y P, Li E L 2018 Phys. Chem. Chem. Phys. 20 5721
Google Scholar
[43] Lu Y J, Ou Z Y 2002 Phys. Rev. Lett. 88 023601
[44] Boddeda R, Glorieux Q, Bramati A, Pigeon S 2019 J. Phys. B:At. Mol. Opt. Phys. 52 215401
Google Scholar
[45] Shih C C 1986 Phys. Rev. D 34 2720
Google Scholar
[46] Campos R A, Saleh B E A, Teich M C 1989 Phys. Rev. A 40 1371
Google Scholar
下载:
计量
- 文章访问数: 4388
- PDF下载量: 99
- 被引次数: 0
