-
本文基于声光效应和Gladstone–Dale关系, 推导了在平面声场扰动下, 各向同性均匀大气介质和非均匀大气介质的折射率随声压变化关系式, 建立了平面光波和拉盖尔-高斯(Laguerre-Gaussian, LG)光束通过经平面声波扰动的均匀大气和非均匀大气介质的传输模型. 结果表明, 经平面声场扰动后, 均匀大气介质折射率分布呈层均匀的周期性分布. 对于大气压强纵向变化的大尺度角度, 平面声场对非均匀大气折射率的分布情况影响不明显; 而对于小尺度角度, 非均匀大气折射率会随高度的增加逐渐减小, 并且随声压的影响而产生波动. 平面声波扰动均匀大气介质时, 会使平面光波的等相位面因声波的影响产生明显波动; LG光束相位会发生旋转, 且总会回到初始相位. 平面声波扰动非均匀大气介质时, 会使平面光波的相位变化会随着声波的变化规律产生周期性的变化, 光程整体为倾斜的平面, 但由于声波的扰动, 光程会产生波动; LG光束的相位仍会发生旋转, 但与均匀介质不同的是, 由于其折射率随高度的变化, 其相位不会回到初始相位.Based on the acousto-optic effect and the Gladstone–Dale relationship, the relationship about variations of the refractive index of the isotropic homogeneous atmospheric medium and the inhomogeneous atmospheric medium with the sound pressure under the disturbance of the plane sound field is derived. Models for the transmission of plane light waves and Laguerre-Gaussian beams through homogeneous atmospheric medium and inhomogeneous atmospheric medium disturbed by plane acoustic waves are established. The results show that the refractive index distribution of the homogeneous atmospheric medium exhibits a homogeneous periodic distribution after being disturbed by the plane sound field. For large-scale angles of longitudinal variation of atmospheric pressure, the plane sound field has little effect on the distribution of the refractive index of the inhomogeneous atmosphere. For small-scale angles, the inhomogeneous atmospheric refractive index gradually decreases with height and fluctuates with the influence of sound pressure. When the plane acoustic wave disturbs the homogeneous atmospheric medium, the isophase plane of the plane light wave will fluctuate significantly due to the influence of the acoustic wave. The phase of the LG beam rotates and always returns to the original phase. When the plane acoustic wave disturbs the inhomogeneous atmospheric medium, the phase change of the plane light wave will change periodically with the change law of the sound wave. The overall optical path is an inclined plane, but due to the disturbance of the sound wave, the optical path will fluctuate. The phase of the LG beam still rotates, but unlike the homogeneous medium, its phase does not return to its original phase due to the change of its refractive index with height.
-
Keywords:
- atmospheric media /
- sound waves /
- light wave /
- acousto-optic effect /
- phase
[1] Adler R 1967 IEEE spectr. 4 42
Google Scholar
[2] Torras-Rosell A, Barrera-Figueroa S, Jacobsen F 2012 J. Acoust. Soc. Am. 131 3786
Google Scholar
[3] Certon D, Ferin G, Matar O B, Guyonvarch J, Remenieras J P, Patat F 2004 Ultrasonics 42 465
Google Scholar
[4] Wang J, Yang J Y, Fazal I M, Ahmed N, Yan Y, Huang H, Ren Y, Yue Y, Dolinar S, Tur M 2012 Nat. Photonics 6 488
Google Scholar
[5] Ramachandran S, Kristensen P, Yan M F 2009 Opt. Lett. 34 2525
Google Scholar
[6] Weisbuch G, Garbay F 1979 Am. J. Phys. 47 355
Google Scholar
[7] Pitts T A, Greenleaf J F 2000 J. Acoust. Soc. Am. 108 2873
Google Scholar
[8] Yamaguchi K, Choi P K 2006 Jpn. J. Appl. Phys. 45 4621
Google Scholar
[9] 周慧婷, 吕朋, 廖长义, 王华, 沈勇 2012 光学学报 32 6
Google Scholar
Zhou H T, Lv P, Liao C Y, Wang H, Shen Y 2012 Acta Opt. Sin. 32 6
Google Scholar
[10] Farhat M, Guenneau S, Bagci H 2013 Phys. Rev. Lett. 111 237404
Google Scholar
[11] Ishikawa K, Yatabe K, Chitanont N, Ikeda Y, Oikawa Y, Onuma T, Niwa H, Yoshii M 2016 Opt. Express 24 12922
Google Scholar
[12] Gladstone J H, Dale T P 1863 Philos. Trans. R. Soc. London 153 317
[13] Ishikawa K, Yatabe K, Ikeda Y, Oikawa Y 2015 12 th Western Pacific Acoustics Conference Singapore, December 6-10, 2015 pp165–169
[14] 程建春 2012 声学原理 (北京: 科学出版社) 第341页
Cheng J C 2012 Principles of Acoustic (Beijing: Science Press) p32 (in Chinese)
[15] Gong S H, Yan D, Wang X 2015 Radio Sci. 50 983
Google Scholar
[16] Gong S H, Liu Y, Hou M Y, Guo L X 2017 Computational and Experimental Studies of Acoustic Waves (New York: IntechOpen) p124
[17] Abdullah-Al-Mamun M, Voelz D 2020 Opt. Eng. 59 081802
Google Scholar
[18] Rüeger J M 2002 Refractive indices of light, infrared and radio waves in the atmosphere (Sydney: School of Surveying and Spatial Information Systems, University of New South Wales)
[19] Luo H, Wen S, Shu W, Tang Z, Zou Y, Fan D 2008 Phys. Rev. A 78 1
Google Scholar
[20] 丁攀峰, 蒲继雄 2011 60 094204
Google Scholar
Ding P F, Pu J X 2011 Acta Phys. Sin. 60 094204
Google Scholar
[21] Yang S, Wang J, Guo M, Qin Z, Li J 2020 Opt. Commun. 465 125559
Google Scholar
-
图 1 参考坐标系及不同类型声源所导致均匀介质折射率分布示意图
Fig. 1. Reference coordinate system and schematic diagram of refractive index distribution of uniform medium caused by different types of sound sources.
图 2 不同声源扰动均匀介质折射率分布三维图 (a) 平面波声源; (b) 球面波声源; (c) 柱面波声源
Fig. 2. Three-dimensional diagram of refractive index distribution of homogeneous medium perturbed by different sound sources: (a) Plane wave sound source; (b) spherical wave sound source; (c) cylindrical wave sound source.
图 3 不同声源扰动均匀介质折射率分布二维图 (a) 平面波声源; (b) 球面波声源; (c) 柱面波声源
Fig. 3. Two-dimensional diagram of the refractive index distribution of homogeneous medium perturbed by different sound sources: (a) Plane wave sound source; (b) spherical wave sound source; (c) cylindrical wave sound source.
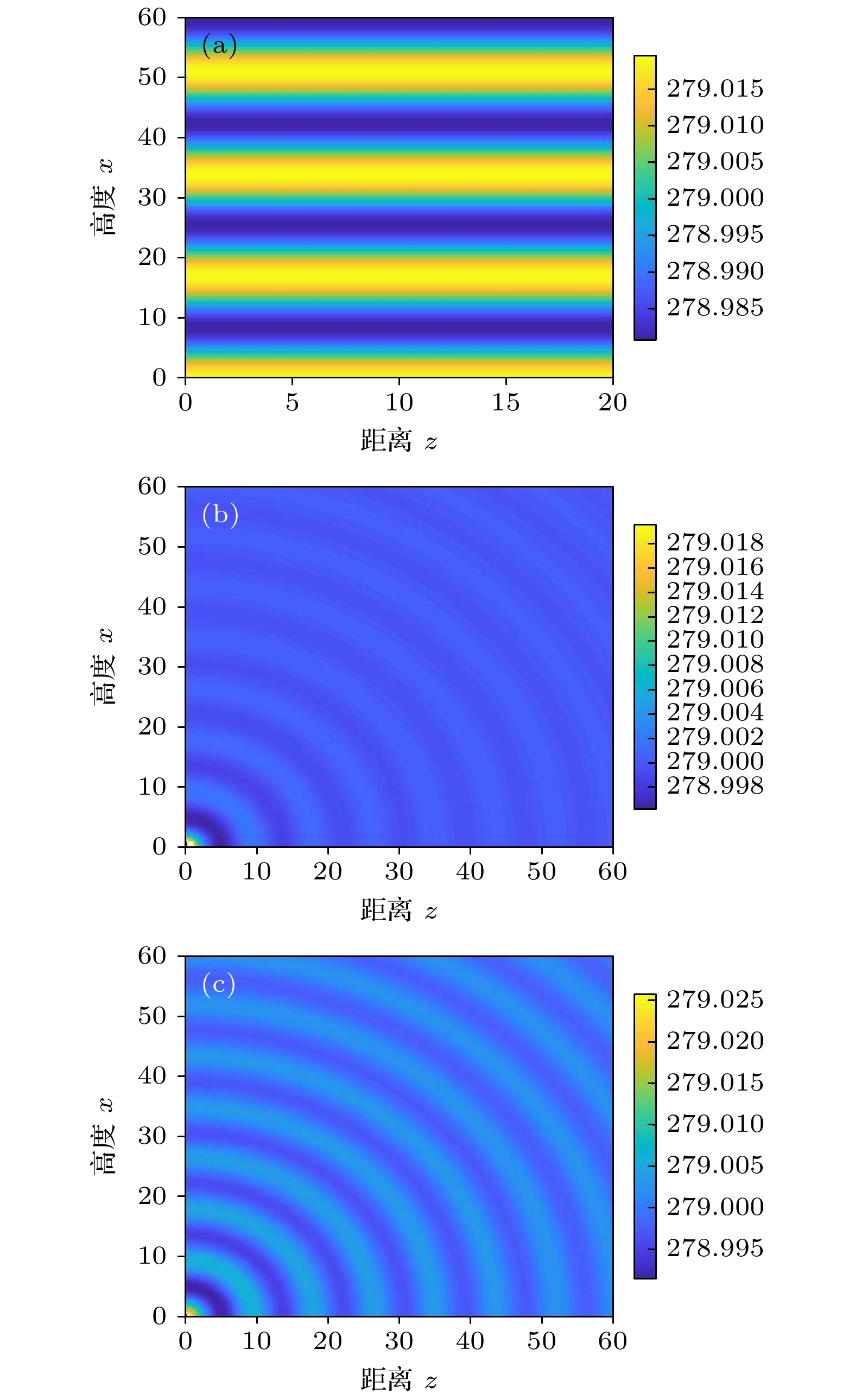
图 4 声波扰动非均匀大气介质折射率随高度和距离变化 (a) 大气压强纵向变化大尺度; (b) 大气压强纵向变化小尺度
Fig. 4. Variation of refractive index with height and distance of inhomogeneous atmospheric medium perturbed by acoustic waves: (a) Large-scale longitudinal variation of atmospheric pressure; (b) small-scale longitudinal variation of atmospheric pressure.
图 5 非均匀介质折射率随高度变化曲线 (a) 不同声波频率; (b) 不同声压
Fig. 5. Variation curve of refractive index of inhomogeneous medium with height: (a) Different sound wave frequencies; (b) different sound pressures
图 6 平面光波通过平面声场扰动的大气介质模型
Fig. 6. The atmospheric medium model of plane light wave perturbed by plane sound field.
图 7 均匀大气介质中平面光波的相位变化 (a) 未进入声场(z = 0 m, p = 10 Pa); (b) 通过声场后(z = 20 m, p = 10 Pa)
Fig. 7. Phase change of plane light waves in homogeneous atmospheric medium: (a) Without entering the sound field (z = 0 m, p = 10 Pa); (b) after passing through the sound field (z = 20 m, p = 10 Pa).
图 8 均匀大气介质中平面光波的光程 (a)无声场(z = 20 m, p = 0 Pa); (b) 有声场(z = 20 m, p = 10 Pa)
Fig. 8. Optical path of plane light waves in homogeneous atmospheric medium: (a) Without sound field (z = 20 m, p = 0 Pa); (b) with sound field (z = 20 m, p = 10 Pa).
图 9 非均匀大气介质中平面光波的相位变化 (a) 未进入声场(z = 0 m, p = 10 Pa); (b) 通过声场后(z = 20 m, p = 10 Pa)
Fig. 9. Phase change of plane light waves in inhomogeneous atmospheric medium: (a) Without entering the sound field (z = 0 m, p = 10 Pa); (b) after passing through the sound field (z = 20 m, p = 10 Pa)
图 10 非均匀大气介质中平面光波的光程 (a) 无声场(z = 20 m, p = 0 Pa); (b) 有声场(z = 20 m, p = 10 Pa)
Fig. 10. Optical path of plane light waves in inhomogeneous atmospheric medium: (a) Without sound field (z = 20 m, p = 0 Pa); (b) with sound field (z = 20 m, p = 10 Pa).
图 11 LG光束通过平面声场扰动的大气介质模型
Fig. 11. The atmospheric medium model of LG beam perturbed by the plane sound field.
图 12 均匀介质折射率随高度变化曲线
Fig. 12. Variation curve of refractive index of homogeneous medium with height
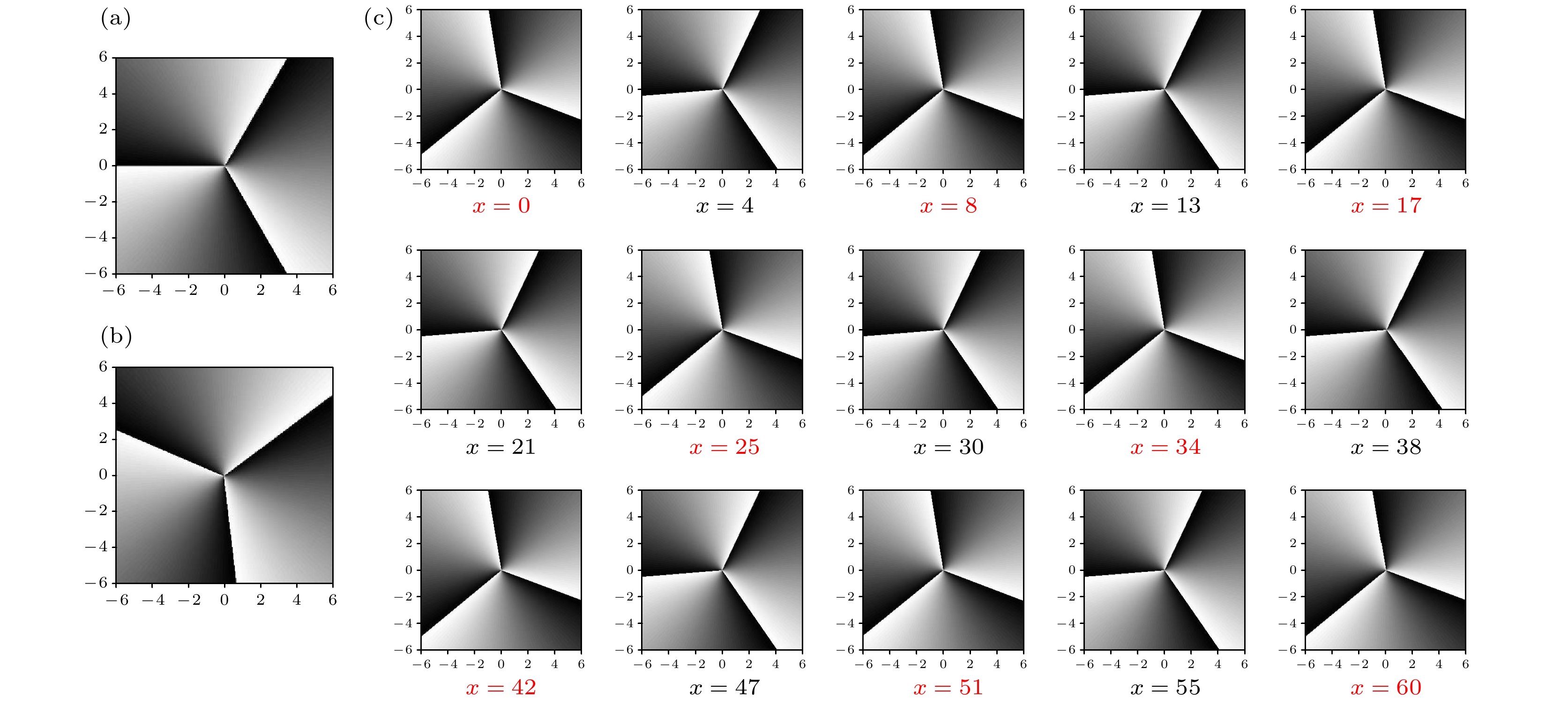
图 13 LG光束进入声场扰动的均匀介质相位随高度变化情况 (a) 未进入声场; (b) 进入无声场介质; (c) 进入平面声场扰动的均匀介质
Fig. 13. The phase variation of LG beam entering the homogeneous medium disturbed by the sound field: (a) Without entering the sound field; (b) entering the medium without sound field; (c) entering the homogeneous medium disturbed by the plane sound field.
图 14 LG光束进入声场扰动的非均匀介质相位随高度变化情况 (a) 进入无声场非均匀介质; (b) 进入平面声场扰动的非均匀介质
Fig. 14. The phase variation of the LG beam entering the inhomogeneous medium disturbed by the sound field: (a) Entering the inhomogeneous medium without sound field; (b) entering the inhomogeneous medium with plane sound field disturbance.
-
[1] Adler R 1967 IEEE spectr. 4 42
Google Scholar
[2] Torras-Rosell A, Barrera-Figueroa S, Jacobsen F 2012 J. Acoust. Soc. Am. 131 3786
Google Scholar
[3] Certon D, Ferin G, Matar O B, Guyonvarch J, Remenieras J P, Patat F 2004 Ultrasonics 42 465
Google Scholar
[4] Wang J, Yang J Y, Fazal I M, Ahmed N, Yan Y, Huang H, Ren Y, Yue Y, Dolinar S, Tur M 2012 Nat. Photonics 6 488
Google Scholar
[5] Ramachandran S, Kristensen P, Yan M F 2009 Opt. Lett. 34 2525
Google Scholar
[6] Weisbuch G, Garbay F 1979 Am. J. Phys. 47 355
Google Scholar
[7] Pitts T A, Greenleaf J F 2000 J. Acoust. Soc. Am. 108 2873
Google Scholar
[8] Yamaguchi K, Choi P K 2006 Jpn. J. Appl. Phys. 45 4621
Google Scholar
[9] 周慧婷, 吕朋, 廖长义, 王华, 沈勇 2012 光学学报 32 6
Google Scholar
Zhou H T, Lv P, Liao C Y, Wang H, Shen Y 2012 Acta Opt. Sin. 32 6
Google Scholar
[10] Farhat M, Guenneau S, Bagci H 2013 Phys. Rev. Lett. 111 237404
Google Scholar
[11] Ishikawa K, Yatabe K, Chitanont N, Ikeda Y, Oikawa Y, Onuma T, Niwa H, Yoshii M 2016 Opt. Express 24 12922
Google Scholar
[12] Gladstone J H, Dale T P 1863 Philos. Trans. R. Soc. London 153 317
[13] Ishikawa K, Yatabe K, Ikeda Y, Oikawa Y 2015 12 th Western Pacific Acoustics Conference Singapore, December 6-10, 2015 pp165–169
[14] 程建春 2012 声学原理 (北京: 科学出版社) 第341页
Cheng J C 2012 Principles of Acoustic (Beijing: Science Press) p32 (in Chinese)
[15] Gong S H, Yan D, Wang X 2015 Radio Sci. 50 983
Google Scholar
[16] Gong S H, Liu Y, Hou M Y, Guo L X 2017 Computational and Experimental Studies of Acoustic Waves (New York: IntechOpen) p124
[17] Abdullah-Al-Mamun M, Voelz D 2020 Opt. Eng. 59 081802
Google Scholar
[18] Rüeger J M 2002 Refractive indices of light, infrared and radio waves in the atmosphere (Sydney: School of Surveying and Spatial Information Systems, University of New South Wales)
[19] Luo H, Wen S, Shu W, Tang Z, Zou Y, Fan D 2008 Phys. Rev. A 78 1
Google Scholar
[20] 丁攀峰, 蒲继雄 2011 60 094204
Google Scholar
Ding P F, Pu J X 2011 Acta Phys. Sin. 60 094204
Google Scholar
[21] Yang S, Wang J, Guo M, Qin Z, Li J 2020 Opt. Commun. 465 125559
Google Scholar
下载:
计量
- 文章访问数: 3605
- PDF下载量: 65
- 被引次数: 0
