-
In order to study the decoherence process of quantum system and find the ways to delay it, in this paper we investigate the decoherence process under correlated depolarizing channels by calculating the evolution of norm coherence, relative entropy coherence and basis-independent coherence of the three initial states: special initial state, maximum coherent state and isotropic state. From the analytic results, numerical results and evolution images, it can be found that 1) completely correlated channels can suppress decoherence to a greatest extent,. 2) the time point at which maximum decoherence occurs is irrelevant to the degree of correlation, but system’s decoherence evolution behavior depends on it. 3)the correlation of depolarizing channels can reinforce the collective quantum coherence between subsystems, and an inequality relation of basis-independent coherence is proved in this system.
-
Keywords:
- depolarization channel /
- correlation /
- quantum coherence /
- decoherence evolution
[1] Hu M L, Zhou W 2019 Laser Phys. Lett. 10 1088
Google Scholar
[2] Baumgratz T, Cramer M, Plenio M B 2014 Phys. Rev. Lett. 113 140401
Google Scholar
[3] Li B M, Hu M L, Fan H 2019 Acta Phys. Sin. 68 030304
Google Scholar
[4] Girolami D 2014 Phys. Rev. Lett. 113 170401
Google Scholar
[5] Streltsov A, Singh U, Dhar H S 2015 Phys. Rev. Lett. 115 020403
Google Scholar
[6] Winter A, Yang D 2016 Phys. Rev. Lett. 116 120404
Google Scholar
[7] Yao Y, Xiao X, Ge L 2015 Phys. Rev. A 92 022112
Google Scholar
[8] Shao L H, Xi Z J, Fan H 2015 Phys. Rev. A 91 042120
Google Scholar
[9] Napoli C, Bromley T R, Clanciaruso M 2016 Phys. Rev. Lett. 116 150502
Google Scholar
[10] Hu M L, Hu X Y, Wang J C, Peng Y, Zhang Y R, Fan H 2018 Phys. Rep. 762 1
Google Scholar
[11] Chen J J, Cui J, Zhang Y R 2016 Phys. Rev. A 94 022112
Google Scholar
[12] Hu M L, Fan H 2016 Sci. Rep. 6 29260
Google Scholar
[13] Osterloh A, Amico L, Falci G, 2002 Nature 416 608
Google Scholar
[14] Li C M, Lambert N, Chen Y N, Chen G Y, Nori F 2012 Sci. Rep. 2 885
Google Scholar
[15] Tan K C, Volkoff T, Kwon H, Jeong H 2017 Phys. Rev. Lett. 119 190405
Google Scholar
[16] Zhang Y D 2005 Principles of Quantum Information Physics (Beijing: Science Press) pp147–151
[17] Macchiavello C, Palma G M 2002 Phys. Rev. A 65 050301
Google Scholar
[18] Wang S, Yao L, Long G L 2013 Phys. Rev. A 87 062305
Google Scholar
[19] Moreva E V, Maslennikov S S, Straupe S P 2006 Phys. Rev. Lett. 97 023602
Google Scholar
[20] Radhakrishnan C, Ding Z, Shi F, Du J, Byrnes T 2019 Ann. Phys. 409 167906
Google Scholar
[21] Napoli C, Bromley T R, CianciarusoM 2016 Physical Review Letters 116 150502
[22] Xue G H, Qiu L 2020 Entropy. 22 827
Google Scholar
[23] Khan S, Khan M K 2011 J. Mod. Opt. 58 918
Google Scholar
[24] Doustimotlagh N, Guo J L, Wang S H 2015 IJTP 54 1784
-
图 1 图中的球为Bloch球, 绿色线段标示的距离衡量的是基矢无关量子相干性, 红色线段标示的距离衡量的是基矢相关的量子相干性
Fig. 1. This is a Bloch ball, the distance marked by the green line represents basis-independent quantum coherence and the distance marked by the red line represents basis-dependent quantum coherence.
图 2 蓝色、红色、绿色、紫色、黑色曲线依次对应关联度
$ \mu $ 为1, 0.5,$ 1/6 $ , 0.1, 纵坐标表示范数相干性Fig. 2. Blue, red, green, purple and black lines correspond to
$ \mu $ as 1, 0.5,$ 1/6 $ , 0.1, and Y-axis represents$ {l}_{1} $ norm coherence.
图 3 关联度满足
$1/5\leqslant \mu \leqslant 1$ 或$ \mu =0 $ 时第一种演化方式($ \mu =1 $ ).Fig. 3. The first caseof evolution when
$1/5\leqslant \mu \leqslant 1$ or$ \mu =0 $ ($ \mu =1 $ ).
图 5 关联度满足
$ 0 < \mu < 1/9 $ 时第3种演化方式($ \mu = $ $ 1/10 $ )Fig. 5. The third case of evolution when
$ 0 < \mu < 1/9 $ ($ \mu =1/10 $ ).
图 4 关联度满足
$1/9\leqslant \mu < 1/5$ 时第二种演化方式($ \mu = $ $ 1/7 $ )Fig. 4. The second case of evolution when
$1/9\leqslant \mu < 1/5$ ($ \mu =1/7 $ ) .
图 6 稳态的相对熵相干性随退极化信道关联度的变化
Fig. 6. The change of relative entropy coherence of the steady state with the correlation degree of depolarizing channels.
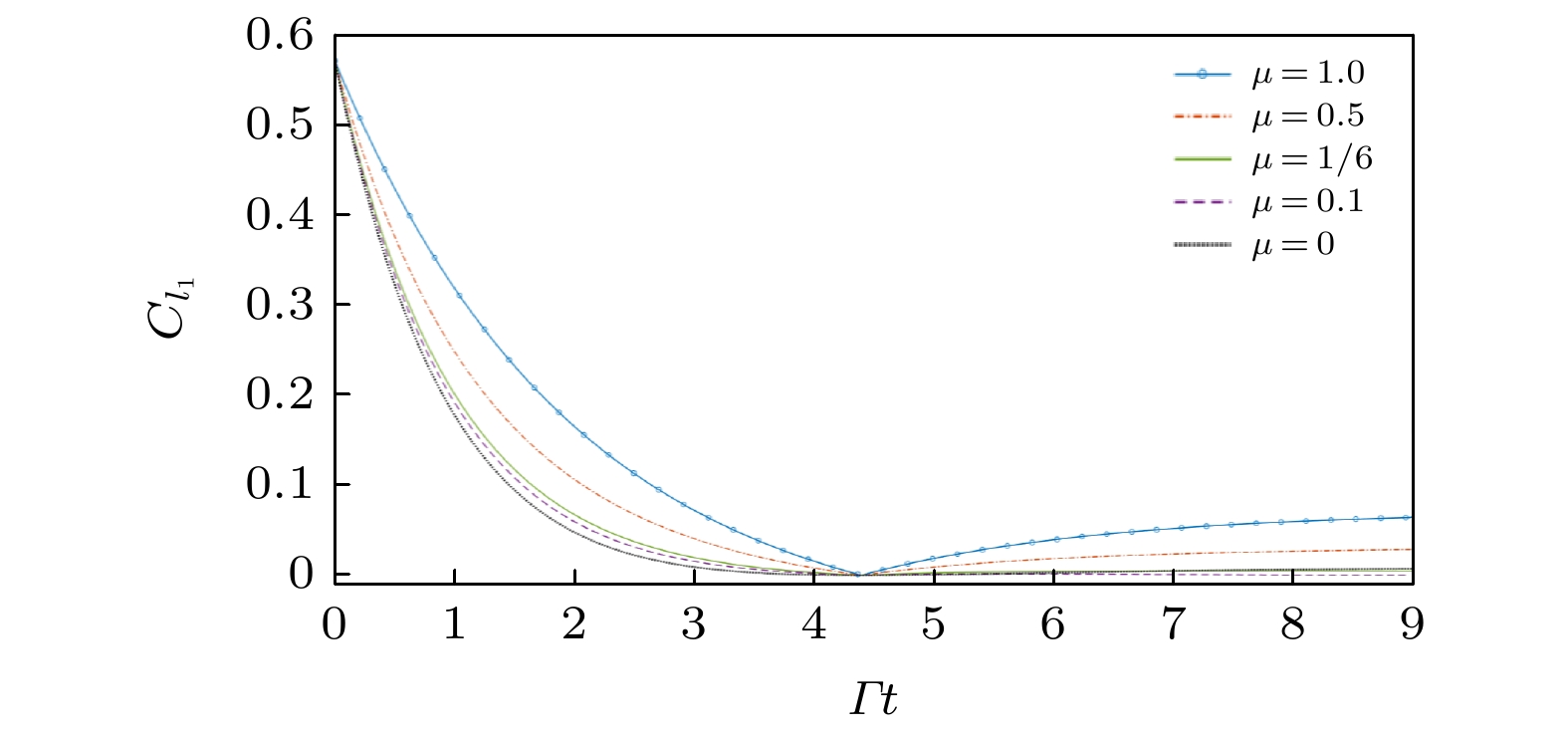
图 7 相对熵相干性随时间的演化, 纵坐标为相对熵相干性数值, 蓝色、紫色、绿色、红色和黑色曲线分别对应关联度
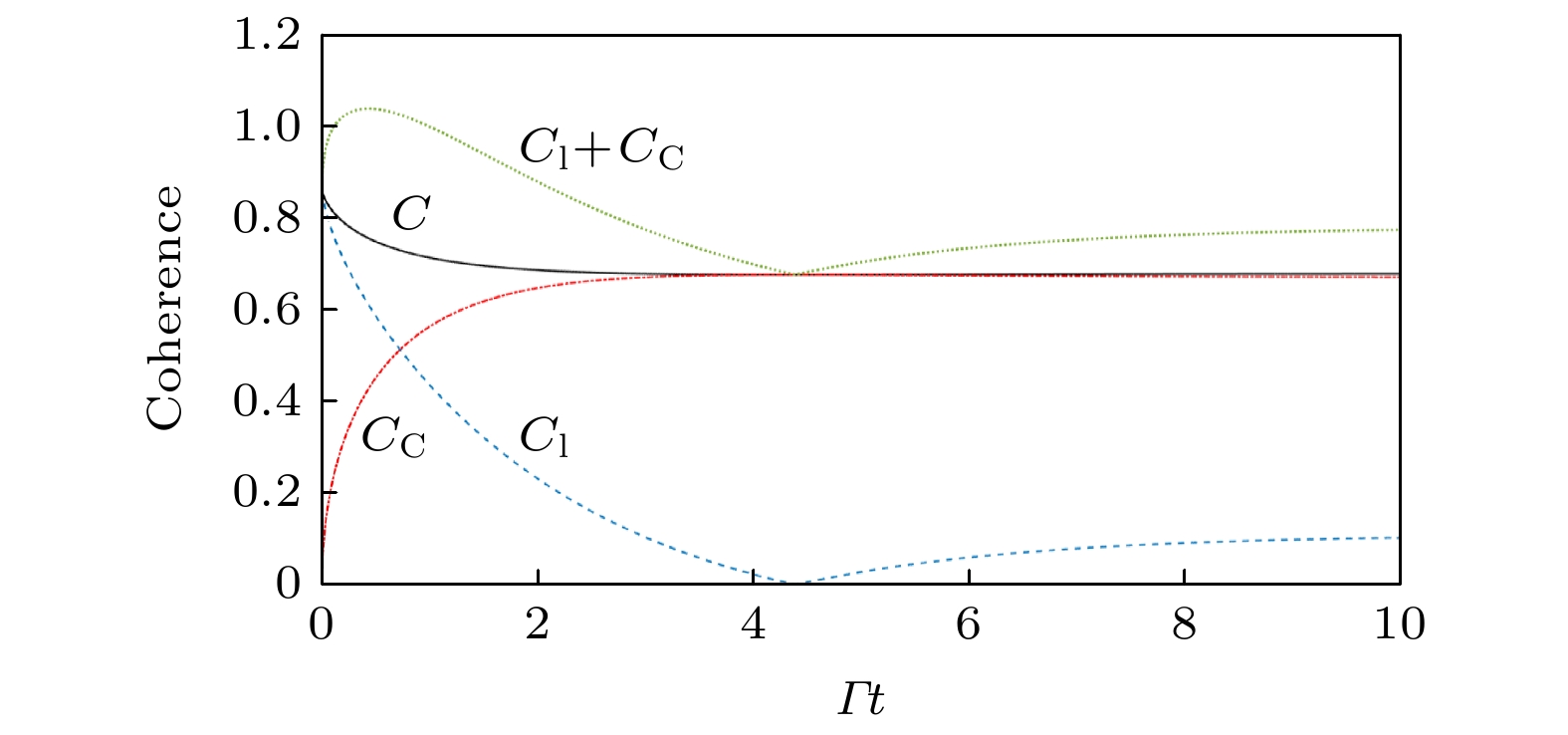
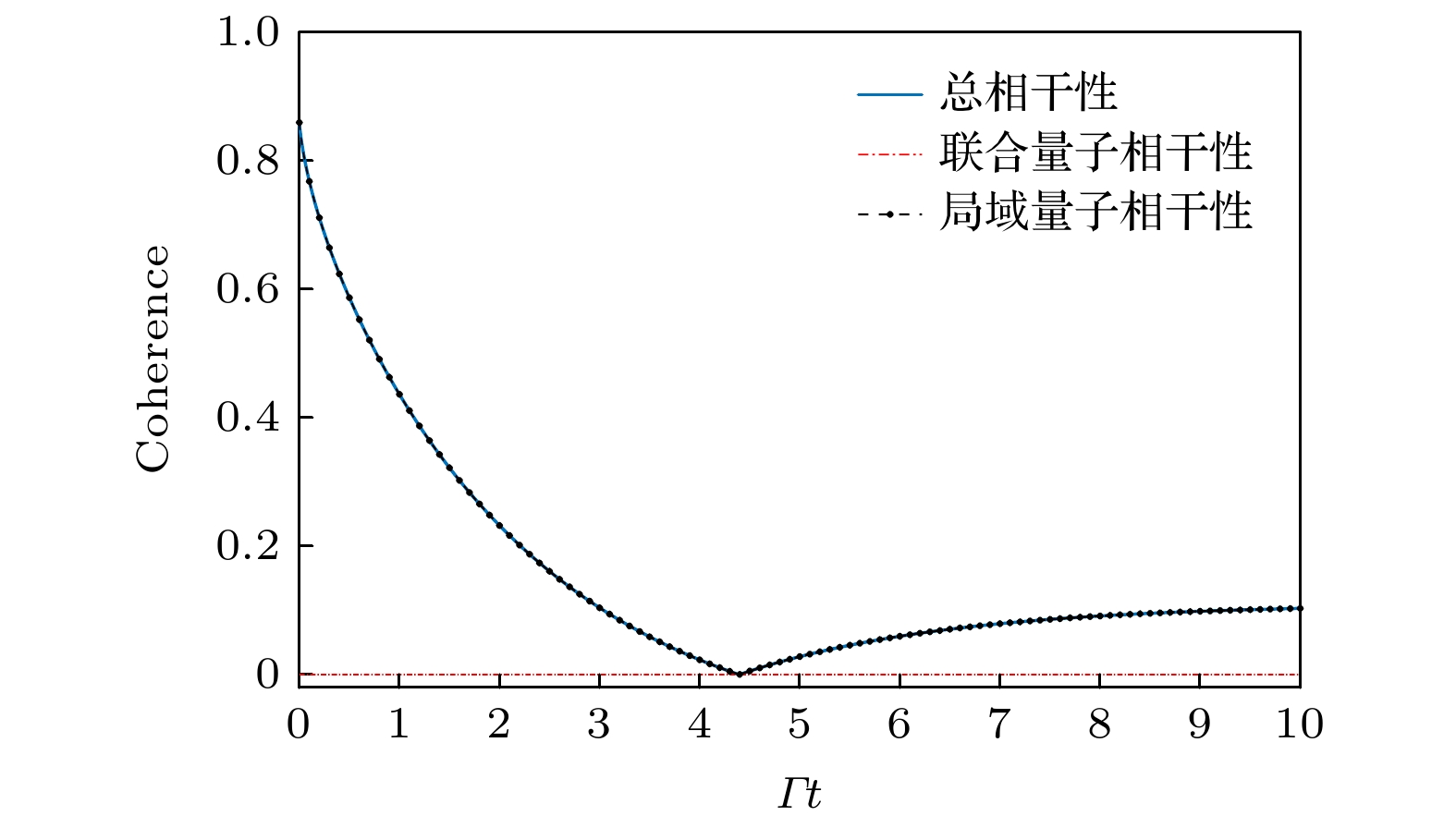
$ \mu $ =1, 0.5,$ 1/6 $ , 0.1, 0的情况, 发现不同关联度下相对熵相干性在同一时间点衰减到0, 零点满足$\varGamma t=2\ln 9$ .Fig. 7. The relative entropy coherence evolving over time, Y-axis represents the value of relative entropy coherence. Blue, purple, green, red and black lines correspond to
$ \mu $ as 1, 0.5,$ 1/6, $ 0.1 and 0. It can be found that the relative entropy coherence decays to 0 at the same time under different correlation degrees, and the zero point is$\varGamma t=2\ln 9$ .
图 8 相对熵相干性在固定零点
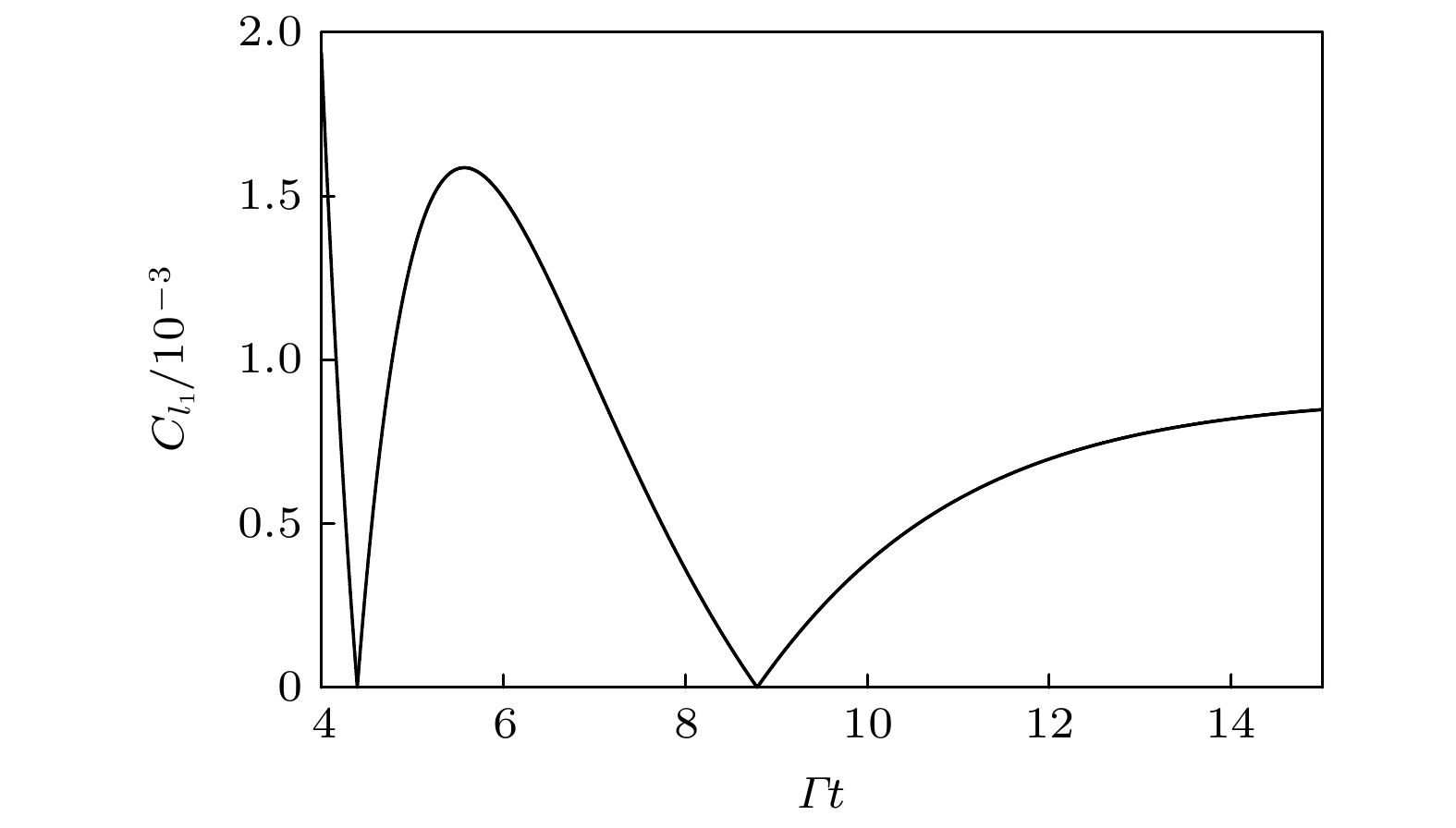
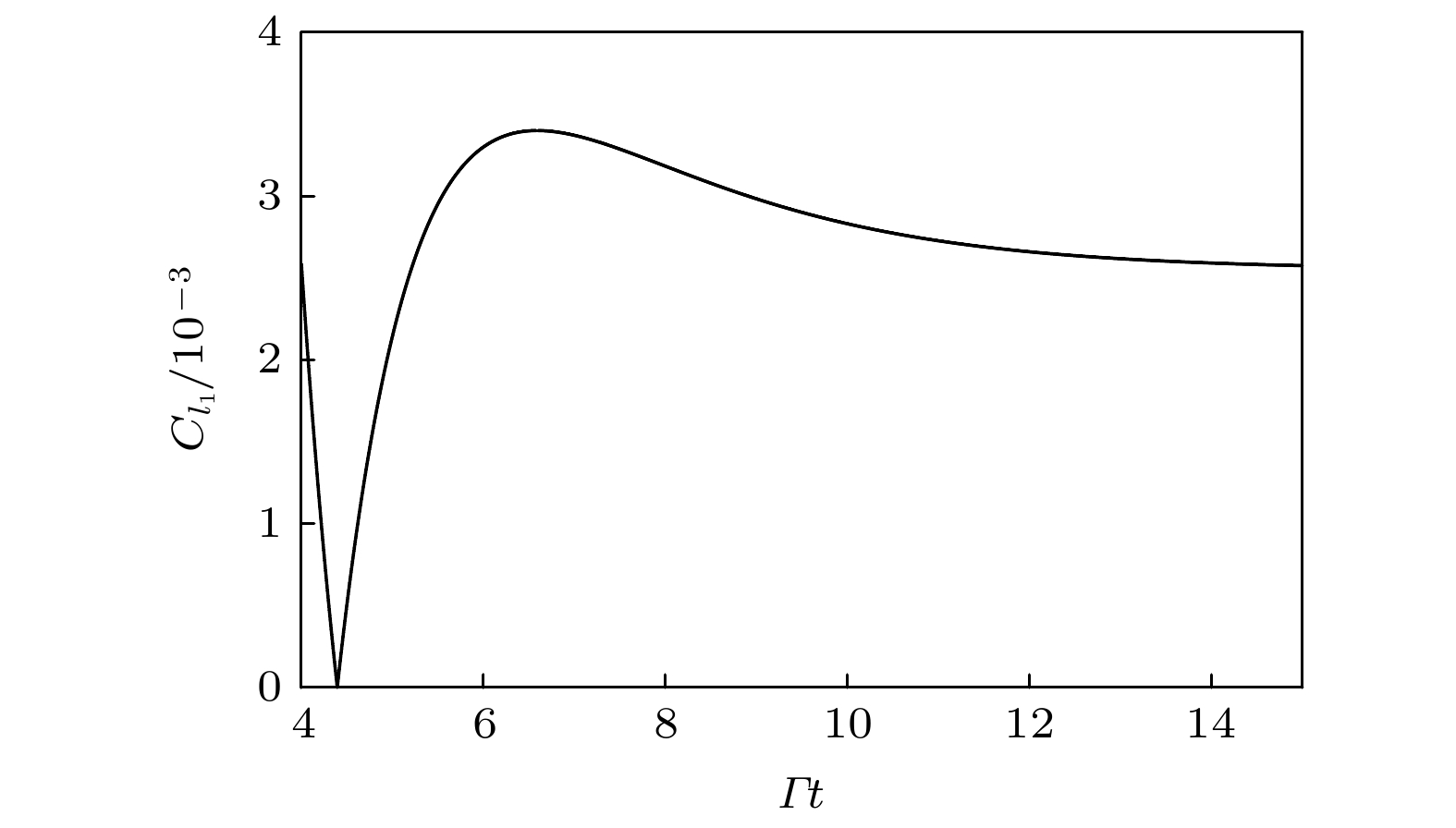
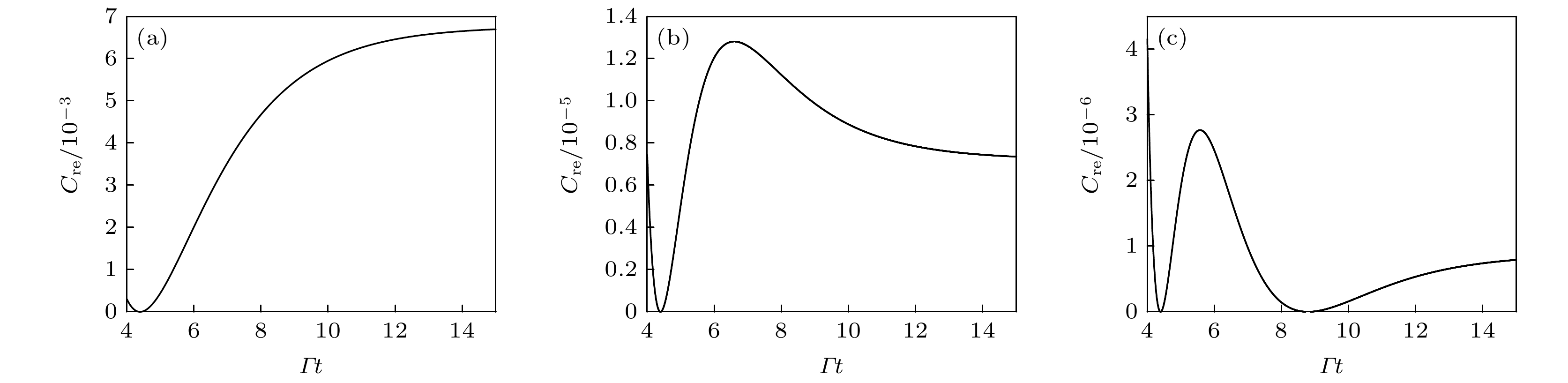
$\varGamma t=2\ln 9$ 后的3种演化方式 (a)$ \mu =0 $ 或$1/2\leqslant \mu \leqslant 1$ ; (b)$ 1/9\leqslant \mu < 1/5 $ ; (c)$ 0 < \mu < 1/9 $ (图中依次取$ \mu =1, 1/7 $ ,$ 0.1 $ ).Fig. 8. Three ways of evolution of relative entropy coherence after fixed time point
$\varGamma t=2 \ln 9$ : (a)$ \mu =0 $ or$1/5\leqslant \mu \leqslant 1$ , (b)$1/9\leqslant \mu < 1/5$ ; (c)$ 0 < \mu < 1/9 $ ($ \mu $ takes to$ 1, 1/7 $ ,$ 0.1 $ respectively) .
图 9 最大相干初态的范数相干性随时间演化, 纵坐标表示其
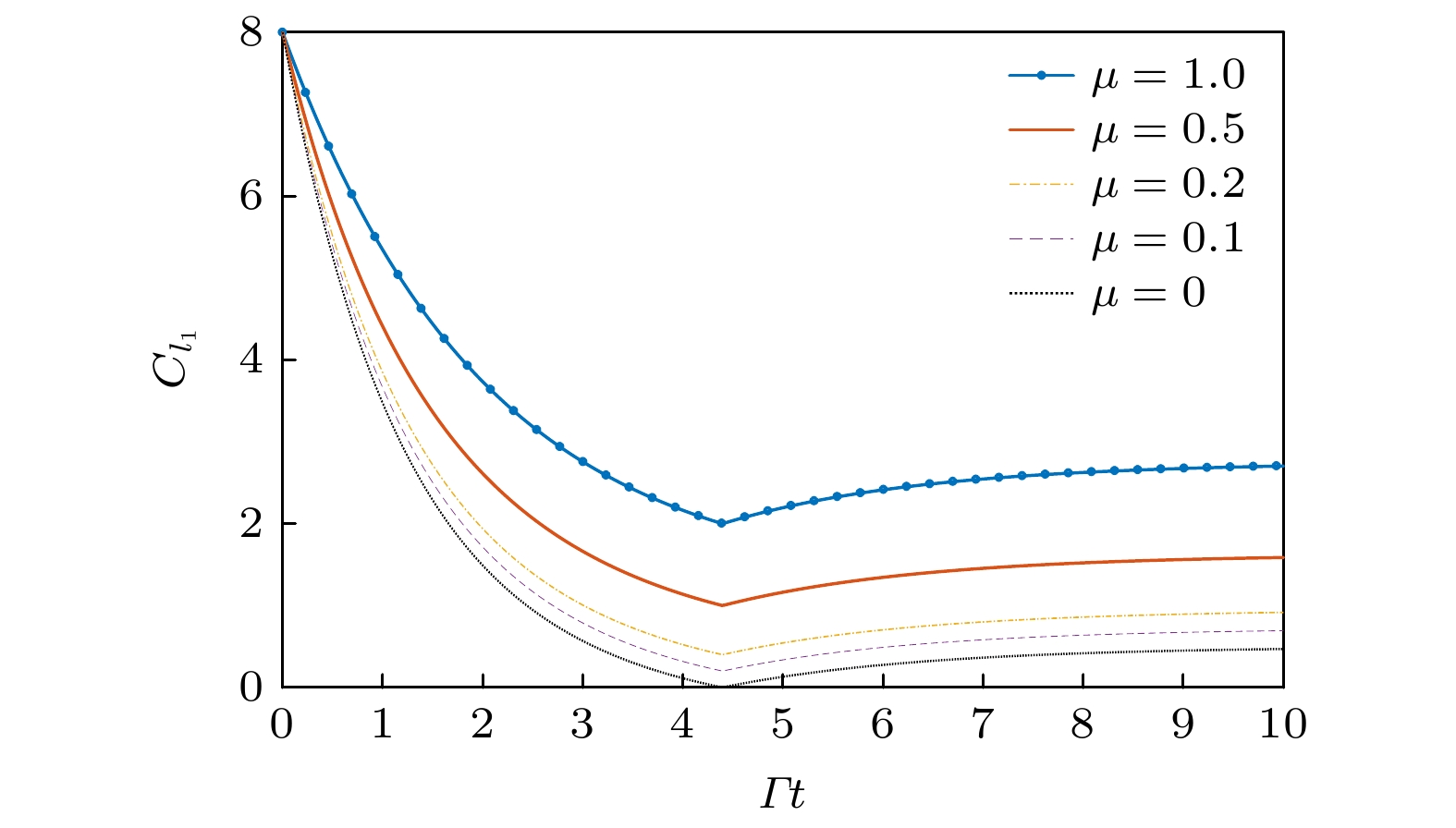
$ {l}_{1} $ 范数相干性, 蓝色、红色、黄色、紫色、黑色曲线分别对应关联度$ \mu $ 为1, 0.5, 0.2, 0.1, 0的情况.Fig. 9. The
$ {l}_{1} $ norm coherence evolution figure of maximum coherent initial tate, Y-axis represents$ {l}_{1} $ norm coherence. Blue, red, yellow, purple and black lines correspond to$ \mu $ as 1, 0.5, 0.2, 0.1, 0.
图 10 基矢无关总相干性, 联合量子相干性与局域量子相干性演化图像(
$ \mu =1 $ , 局域量子相干性)Fig. 10. The basis-independent quantum coherence , collective quantum coherence and localized quantum coherence evolving over time(
$ \mu =1 $ , l) .
图 11 基矢无关总相干性, 联合量子相干性与局域量子相干性演化图像(
$ \mu =0.5 $ )Fig. 11. The basis-independent quantum coherence , collective quantum coherence and localized quantum coherence evolving over time(
$ \mu =0.5 $ ).
图 12 基矢无关总相干性, 联合量子相干性与局域量子相干性演化图像(
$ \mu =0 $ ).Fig. 12. The basis-independent quantum coherence, collective quantum coherence and localized quantum coherence evolving over time (
$ \mu =0 $ ). -
[1] Hu M L, Zhou W 2019 Laser Phys. Lett. 10 1088
Google Scholar
[2] Baumgratz T, Cramer M, Plenio M B 2014 Phys. Rev. Lett. 113 140401
Google Scholar
[3] Li B M, Hu M L, Fan H 2019 Acta Phys. Sin. 68 030304
Google Scholar
[4] Girolami D 2014 Phys. Rev. Lett. 113 170401
Google Scholar
[5] Streltsov A, Singh U, Dhar H S 2015 Phys. Rev. Lett. 115 020403
Google Scholar
[6] Winter A, Yang D 2016 Phys. Rev. Lett. 116 120404
Google Scholar
[7] Yao Y, Xiao X, Ge L 2015 Phys. Rev. A 92 022112
Google Scholar
[8] Shao L H, Xi Z J, Fan H 2015 Phys. Rev. A 91 042120
Google Scholar
[9] Napoli C, Bromley T R, Clanciaruso M 2016 Phys. Rev. Lett. 116 150502
Google Scholar
[10] Hu M L, Hu X Y, Wang J C, Peng Y, Zhang Y R, Fan H 2018 Phys. Rep. 762 1
Google Scholar
[11] Chen J J, Cui J, Zhang Y R 2016 Phys. Rev. A 94 022112
Google Scholar
[12] Hu M L, Fan H 2016 Sci. Rep. 6 29260
Google Scholar
[13] Osterloh A, Amico L, Falci G, 2002 Nature 416 608
Google Scholar
[14] Li C M, Lambert N, Chen Y N, Chen G Y, Nori F 2012 Sci. Rep. 2 885
Google Scholar
[15] Tan K C, Volkoff T, Kwon H, Jeong H 2017 Phys. Rev. Lett. 119 190405
Google Scholar
[16] Zhang Y D 2005 Principles of Quantum Information Physics (Beijing: Science Press) pp147–151
[17] Macchiavello C, Palma G M 2002 Phys. Rev. A 65 050301
Google Scholar
[18] Wang S, Yao L, Long G L 2013 Phys. Rev. A 87 062305
Google Scholar
[19] Moreva E V, Maslennikov S S, Straupe S P 2006 Phys. Rev. Lett. 97 023602
Google Scholar
[20] Radhakrishnan C, Ding Z, Shi F, Du J, Byrnes T 2019 Ann. Phys. 409 167906
Google Scholar
[21] Napoli C, Bromley T R, CianciarusoM 2016 Physical Review Letters 116 150502
[22] Xue G H, Qiu L 2020 Entropy. 22 827
Google Scholar
[23] Khan S, Khan M K 2011 J. Mod. Opt. 58 918
Google Scholar
[24] Doustimotlagh N, Guo J L, Wang S H 2015 IJTP 54 1784
下载:
计量
- 文章访问数: 4528
- PDF下载量: 110
- 被引次数: 0
