-
非线性相干光谱是一种测量材料非线性光学响应的谱学手段. 相比线性光谱, 非线性相干光谱具有多个时间变量, 能够提供材料的更多信息. 作为非线性相干光谱的代表之一, 二维相干光谱在众多领域取得了丰硕的成果. 在化学、生物学等领域, 二维相干光谱已经展现出相比线性谱学的优越性. 随着太赫兹技术的发展, 二维相干光谱在强关联体系也显现出巨大潜力, 相关的理论与实验工作正在开展. 本文简要概括了强关联体系二维相干光谱的发展现状, 介绍了二维相干光谱的基本概念与理论工具, 分析了二维相干光谱的主要特点, 并重点总结了我们研究组近几年关于强关联体系二维相干光谱的理论研究工作.Two-dimensional coherent spectroscopy (2DCS) diagnoses a material’s nonlinear optical response with multiple time variables, thus offering information that is inaccessible with conventional linear optical spectroscopy. The 2DCS in the infrared, visible, and ultraviolet frequency range has yielded fruitful results in chemistry and biology. In the terahertz (THz) frequency window, 2DCS has shown its promise in the study of strongly-correlated electronic systems. As a guide to this rapidly developing field, we survey the current status of the theory of THz-2DCS in strongly-correlated electronic systems. We then introduce the basic concepts and theoretical methods of 2DCS, and analyze the main characteristics of the two-dimensional spectra. Finally, we summarize our latest theoretical research in this field.
[1] Mukamel S 1999 Principle of Nonlinear Optical Spectroscopy (Oxford: Oxford University Press) pp3–139
[2] Hamm P, Zanni M 2011 Concepts and Methods of 2D Infrared Spectroscopy (Cambridge: Cambridge University Press) pp1–86
[3] 翁宇翔, 陈海龙 2018 超快激光光谱原理与技术基础 (北京: 化学工业出版社)
Weng Y X, Chen H L 2018 Ultrafast Spectroscopy-Principles and Techniques (Beijing : Chemical Industry Press) (in Chinese)
[4] Cundiff S, Mukamel S 2013 Phys. Today 66 44
Google Scholar
[5] Hüfner S, Hossain M A, Damascelli A, Sawatzky1 G A 2008 Rep. Prog. Phys. 71 062501
Google Scholar
[6] Breunig O, Garst M, Sela E, Buldmann B, Becker P, Bohaty L, Muller R, Lorenz T 2013 Phys. Rev. Lett. 111 187202
Google Scholar
[7] Bitko D, Rosenbaum T F, Aeppli G 1996 Phys. Rev. Lett. 77 940
Google Scholar
[8] Coldea R, Tennant D A, Wheeler E M, Wawrzynska E, Prabhakaran D, Telling M, Habicht K, Smeibidl P, Kiefer K 2010 Science 327 177
Google Scholar
[9] Chen Y L, Chu J H, Analytis J G, Liu Z K, Igarashi K, Kuo H H, Qi X L, Mo S K, Moore R G, Lu D H, Hashimoto M, Sasagawa T, Zhang S C, Fisher I R, Hussain Z, Shen Z X 2010 Science 329 5992
Google Scholar
[10] Woerner M, Kuehn W, Bowlan P, Reimann K, Elsaesser T 2013 New J. Phys. 15 025039
Google Scholar
[11] Lu J, Li X, Hwang H Y, Ofori-Okai B K, Kurihara T, Suemoto T, Nelson K A 2017 Phys. Rev. Lett. 118 207204
Google Scholar
[12] Mahmood F, Chaudhuri D, Gopalakrishnan S, Nandkishore R, Armitage N P 2021 Nat. Phys. 17 627
Google Scholar
[13] Wan Y, Armitage N P 2018 Phys. Rev. Lett. 122 257401
Google Scholar
[14] Choi W, Lee K H, Kim Y B 2020 Phys. Rev. Lett. 124 117205
Google Scholar
[15] Nandkishore R M, Choi W, Kim Y B 2021 Phys. Rev. Res. 3 013254
Google Scholar
[16] Parameswaran S A, Gopalakrishnan S 2020 Phys. Rev. Lett. 125 237601
Google Scholar
[17] Phuc N T, Trung P Q 2021 Phys. Rev. B 104 115105
Google Scholar
[18] Li Z L, Oshikawa M, Wan Y 2021 Phys. Rev. X 11 031035
Google Scholar
[19] Hamm P, Meuwl M, Johnson S L, Beaud P 2017 Struc. Dyn. 4 061601
Google Scholar
[20] Hahn E L 1950 Phys. Rev. 80 580
Google Scholar
[21] Carr H Y, Purcell E M 1954 Phys. Rev. 94 630
Google Scholar
[22] Abella I D, Kurnit N A, Hartmann S R 1966 Phys. Rev. 141 391
Google Scholar
[23] Pfeuty P 1970 Ann. Phys. 57 79
Google Scholar
[24] Anderson P W 1984 Basic Notations of Condensed Matter Physics (Benjamin Cummings) pp92–104
[25] Zvezdin A, Kotov V 1997 Modern Magnetooptics and Magnetooptical Materials (New York: Taylor & Francis)
[26] Lieb E, Schultz T, Mattis D 1961 Ann. Phys. 16 407
Google Scholar
[27] Affleck I 1988 Les Houches Summer School in Theoretical Physics: Fields, Strings, Critical Phenomena pp563–640
[28] Giamarchi T 2003 Quantum Physics in One Dimension (Oxford: Oxford University Press)
[29] von Delft J, Schoeller H 1998 Ann. Phys. 7 225
Google Scholar
[30] Shankar R 1993 Acta Phys. Polonica B 26 12
Google Scholar
[31] Pham K V, Gabay M, Lederer P 2000 Phys. Rev. B 61 16397
Google Scholar
[32] Kamenev A 2011 Field Theory of Non-Equilibrium Systems (Cambridge: Cambridge University Press)
[33] Sachdev S, Young A P 1997 Phys. Rev. Lett. 78 2220
Google Scholar
[34] Damle K, Sachdev S 1998 Phys. Rev. B 57 8307
Google Scholar
[35] Damle K, Sachdev S 1998 Phys. Rev. Lett. 95 187201
Google Scholar
-
图 1 线性响应的刘维尔路径.
$R_1$ 对应的路径为图中的红色实线Fig. 1. Liouville paths of linear responce.
$R_1$ is illustrated by the solid red line.
图 3 非线性响应的刘维尔路径.
$R_1$ 由图中红色实线表示Fig. 3. Liouville paths of non-linear responce.
$R_1$ is illustrated by the solid red line.
图 4 横场伊辛模型铁磁相[
$h/(h+J)=0.3$ ]的一维和二维光谱. 从上到下, (a)−(e)无耗散的结果$(1/T_{1, 2}=0)$ ; (f)−(j)有耗散的结果$(1/T_{1, 2}=0.2(J+h))$ ; (k)−(o)添加无序后的结果. 从左到右, 每列分别是${\boldsymbol{\chi}}^{(1)}_{xx}(\omega)$ , 以及${\boldsymbol{\chi}}^{(2)}_{xxx}(t, t+\tau), \; {\boldsymbol{\chi}}^{(3)}_{xxxx}\times $ $ (t, t+\tau, t+\tau),\; {\boldsymbol{\chi}}^{(3)}_{xxxx}(t, t, t+\tau)$ 的傅里叶变换, 以及沿着黑色箭头方向的信号轮廓(本图来自文献[13])Fig. 4. One dimensional (1D) and two dimensional (2D) spectra in the FM phase [
$h/(h+J)=0.3$ ] of the TFIC. From the top to bottom, the rows show (a)−(e) the case with no dissipation$(1/T_{1, 2}=0)$ , (f)−(j) with dissipation$(1/T_{1, 2}=0.2(J+h))$ , and (k)−(o) with quenched disorder. From the left to right, the columns show, respectively,${\boldsymbol{\chi}}^{(1)}_{xx}(\omega)$ , and the FTs of${\boldsymbol{\chi}}^{(2)}_{xxx}(t, t+\tau)$ ,${\boldsymbol{\chi}}^{(3)}_{xxxx}(t, t+\tau, t+\tau), \;{\boldsymbol{\chi}}^{(3)}_{xxxx}(t, t, t+\tau)$ , and its profile along a cut indicated by the black arrow. (This figure is reprinted from ref. [13])
图 5 脉冲序列 (a)
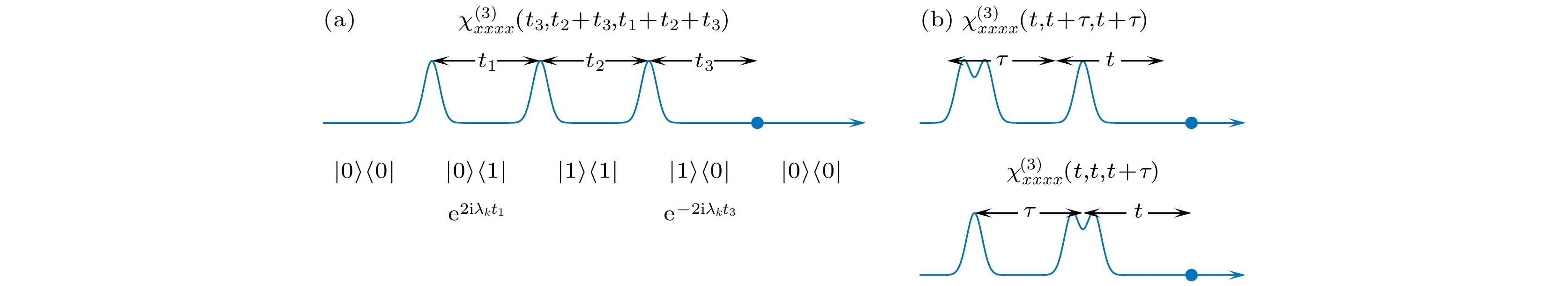
${\boldsymbol{\chi}}_{xxxx}^{(3)}(t_3, t_3+t_2, t_3+t_2+t_1)$ 对应的三脉冲过程以及“自旋子”回波过程$A_k^{(4)}$ 对应的刘维尔路径; (b) 作为三脉冲极限的两脉冲序列下的三阶响应${\boldsymbol{\chi}}^{(3)}$ (本图来自文献[13])Fig. 5. Pulse sequences: (a) Three-pulse process associated with
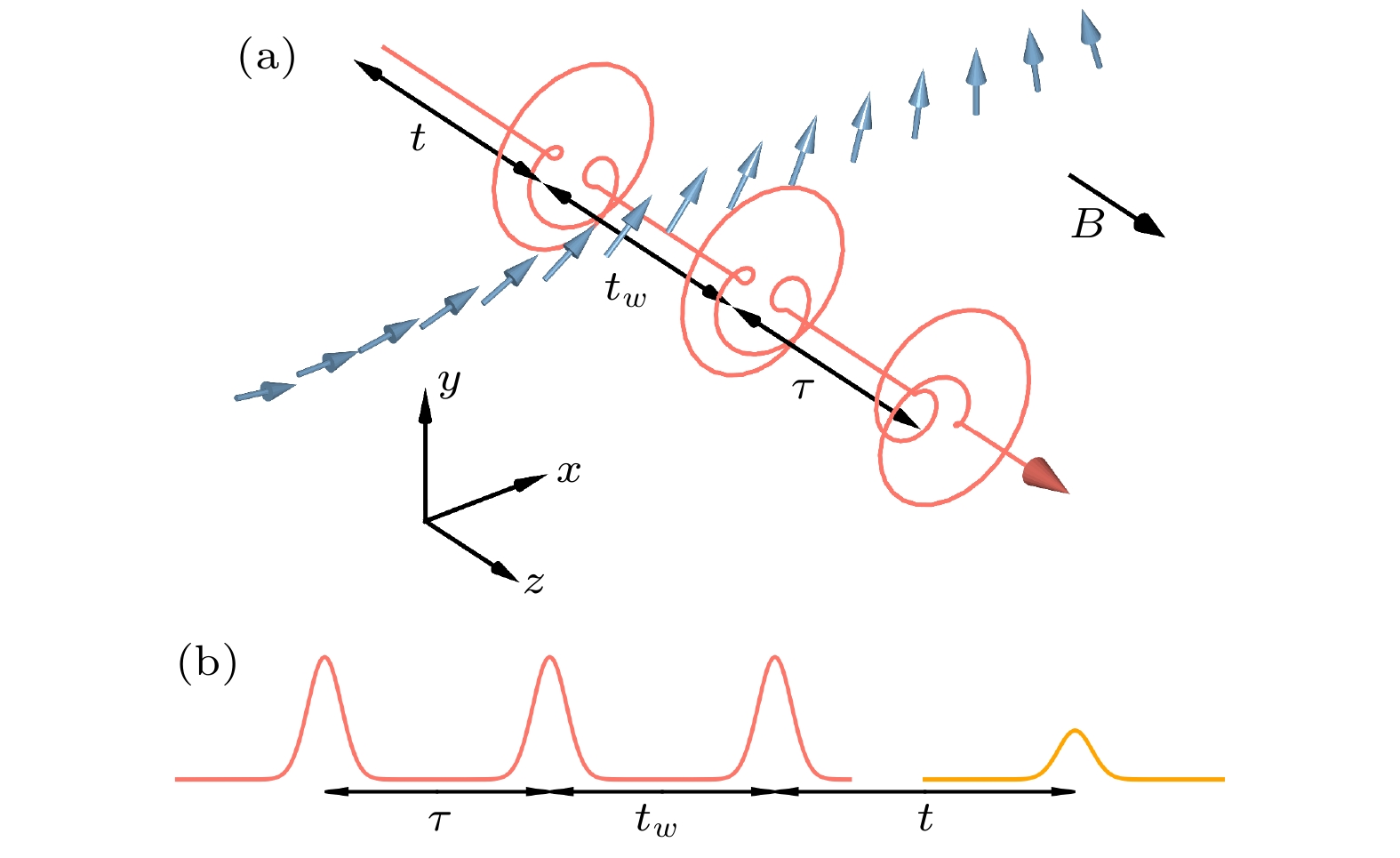
${\boldsymbol{\chi}}_{xxxx}^{(3)}(t_3, t_3+t_2, t_3+t_2+t_1)$ . The spinon echo process that produces the rephasing signal$A_k^{(4)}$ is also shown. (b) The${\boldsymbol{\chi}}^{(3)}$ terms measured in the two-pulse setup are special limits of the three-pulse process. (This figure is reprinted from ref. [13])
图 6 (a) 法拉第构型示意图. 磁场沿
$z$ 方向. 3个圆偏振短光脉冲通过自旋模型, 传播方向平行于$z$ 方向. 第1个光脉冲为右旋偏振, 第2和第3个光脉冲为左旋偏振. 第1和第2个光脉冲的时间间隔为$\tau$ , 第2和第3个光脉冲的时间间隔为$t_w$ , 第三个光脉冲和测量时间的时间间隔为$t$ . (b)$t\approx \tau$ 时, 光子回波信号出现(本图来自文献[18])Fig. 6. (a) The Faraday configuration. A magnetic field B is applied in the z axis. Three short electromagnetic pulses with circular polarizations pass through the S = 1/2 spin chain. The propagation direction is parallel with the spin z axis. The first pulse is right-handed, whereas the second and the third are left-handed. The time delay between the first and the second pulse is denoted by
$\tau$ , the second and the third by$t_w$ , and the third pulse and the time of detection by$t$ . (b) When$ t\approx\tau $ , photon echo appears. (This figure is reprinted from ref. [18])
图 7 (a) 以
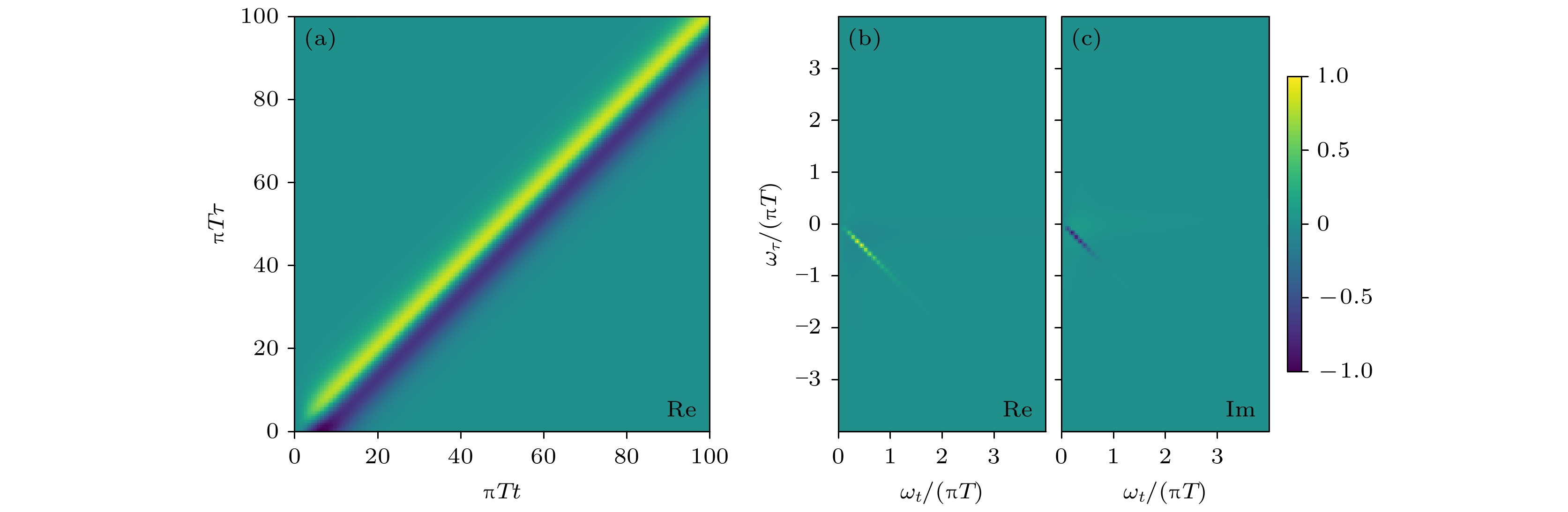
$\pi Tt, \pi T \tau$ 为自变量, 铁磁链的三阶非线性响应${\boldsymbol{\chi}}_{+--+}^{(3)}$ . 固定$\pi Tt_w=1$ , 拉廷格参数$K=1$ . (b), (c) 分别是图(a)中数据傅里叶变换后二维光谱的实部和虚部(本图来自文献[18])Fig. 7. (a) Nonlinear magnetic susceptibility
${\boldsymbol{\chi}}_{+--+}^{(3)}$ of a ferromagnetic chain as function of$\pi Tt$ and$\pi T\tau$ . The waiting time$\pi T t_w=1$ . The Luttinger parameter is$K=1$ . (b), (c) The real and imaginary parts of two dimensional spectrum, obtained by Fourier transforming the data of panel (a). (This figure is reprinted from Ref. [18])
图 8 (a), (b) 以
$\pi Tt, \;\pi T \tau$ 为自变量, 反铁磁链的三阶非线性响应${\boldsymbol{\chi}}_{+--+}^{(3)}$ 的实部和虚部. 固定$\pi Tt_w=1$ , 拉廷格参数$K=1$ , 磁化密度$2 mu/T=1.15$ . (c), (d) 分别是二维相干光谱的实部和虚部(本图来自文献[18])Fig. 8. (a), (b) The real and imaginary parts of Nonlinear magnetic susceptibility
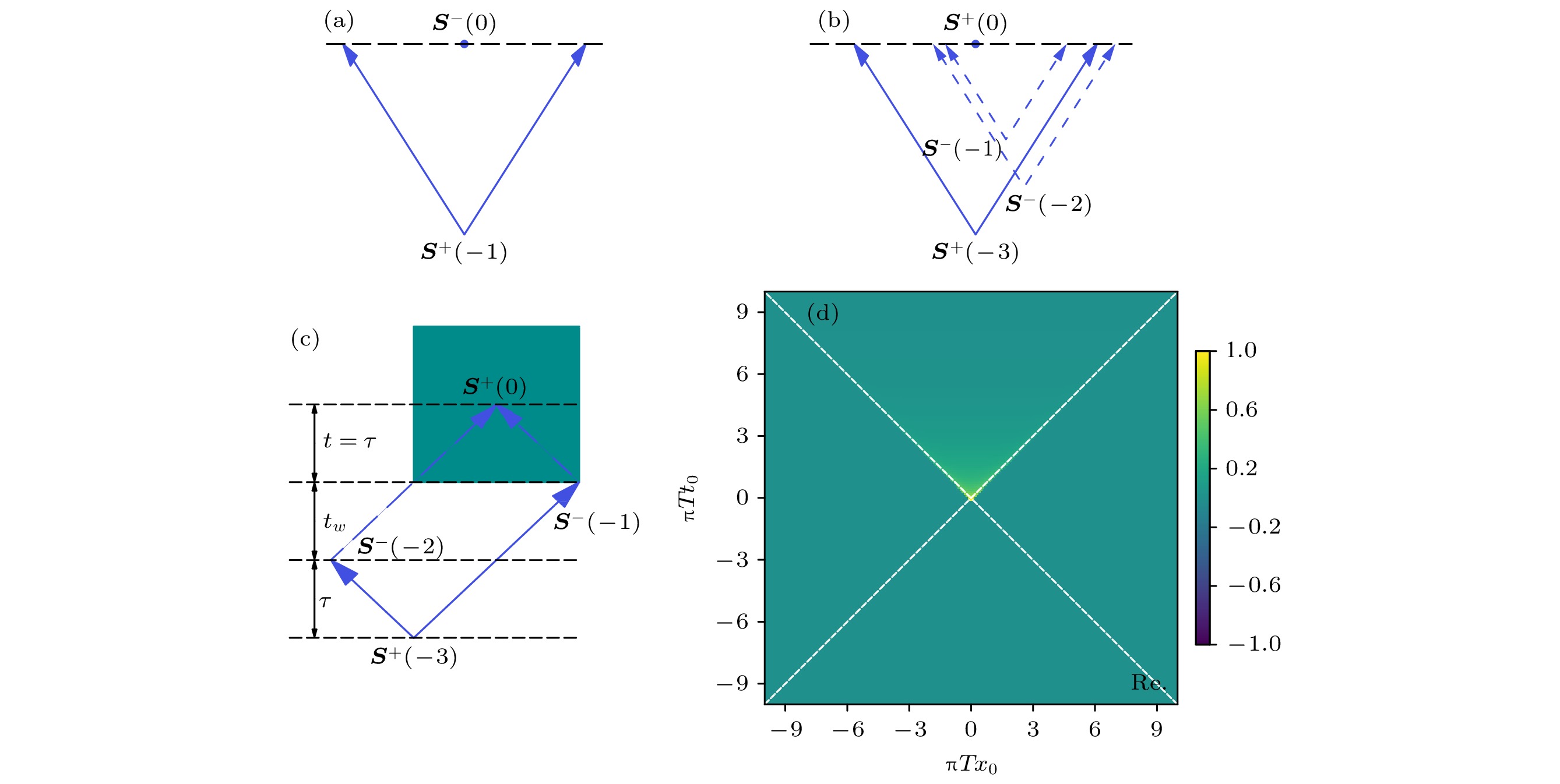
${\boldsymbol{\chi}}_{+--+}^{(3)}$ of an antiferromagnetic chain as function of$\pi Tt$ and$\pi T\tau$ . The waiting time$\pi T t_w=1$ . The Luttinger parameter is$K=1$ . The magnetization density$2 mu/T=1.15$ . (c), (d) The real and imaginary parts of the two-dimensional spectrum. (This figure is reprinted from Ref. [18])
图 9 (a) 两点关联函数中的“自旋子”产生湮灭过程. 实线代表“自旋子”的动力学过程. 虚线代表“反自旋子”的动力学过程. (b) 四点关联函数中的“自旋子”产生湮灭过程. (c) 四点关联函数中的“透镜效应”构型. (d)
$\tilde{{\boldsymbol{\chi}}}_{+--+}^{(3)}$ 在图(c)阴影部分的行为(本图来自文献[18])Fig. 9. (a) The spinon creation/annihilation process in two-point correlation function. Solid and dashed lines represent dynamical processes of spinon and antispinon respectively. (b) The spinon creation/annihilation process in four-point correlation function. (c) The “Lensing” configuration in four-point correlation function. (d) The behavior of the shaded area in panel (c). (This figure is reprinted from ref. [18])
-
[1] Mukamel S 1999 Principle of Nonlinear Optical Spectroscopy (Oxford: Oxford University Press) pp3–139
[2] Hamm P, Zanni M 2011 Concepts and Methods of 2D Infrared Spectroscopy (Cambridge: Cambridge University Press) pp1–86
[3] 翁宇翔, 陈海龙 2018 超快激光光谱原理与技术基础 (北京: 化学工业出版社)
Weng Y X, Chen H L 2018 Ultrafast Spectroscopy-Principles and Techniques (Beijing : Chemical Industry Press) (in Chinese)
[4] Cundiff S, Mukamel S 2013 Phys. Today 66 44
Google Scholar
[5] Hüfner S, Hossain M A, Damascelli A, Sawatzky1 G A 2008 Rep. Prog. Phys. 71 062501
Google Scholar
[6] Breunig O, Garst M, Sela E, Buldmann B, Becker P, Bohaty L, Muller R, Lorenz T 2013 Phys. Rev. Lett. 111 187202
Google Scholar
[7] Bitko D, Rosenbaum T F, Aeppli G 1996 Phys. Rev. Lett. 77 940
Google Scholar
[8] Coldea R, Tennant D A, Wheeler E M, Wawrzynska E, Prabhakaran D, Telling M, Habicht K, Smeibidl P, Kiefer K 2010 Science 327 177
Google Scholar
[9] Chen Y L, Chu J H, Analytis J G, Liu Z K, Igarashi K, Kuo H H, Qi X L, Mo S K, Moore R G, Lu D H, Hashimoto M, Sasagawa T, Zhang S C, Fisher I R, Hussain Z, Shen Z X 2010 Science 329 5992
Google Scholar
[10] Woerner M, Kuehn W, Bowlan P, Reimann K, Elsaesser T 2013 New J. Phys. 15 025039
Google Scholar
[11] Lu J, Li X, Hwang H Y, Ofori-Okai B K, Kurihara T, Suemoto T, Nelson K A 2017 Phys. Rev. Lett. 118 207204
Google Scholar
[12] Mahmood F, Chaudhuri D, Gopalakrishnan S, Nandkishore R, Armitage N P 2021 Nat. Phys. 17 627
Google Scholar
[13] Wan Y, Armitage N P 2018 Phys. Rev. Lett. 122 257401
Google Scholar
[14] Choi W, Lee K H, Kim Y B 2020 Phys. Rev. Lett. 124 117205
Google Scholar
[15] Nandkishore R M, Choi W, Kim Y B 2021 Phys. Rev. Res. 3 013254
Google Scholar
[16] Parameswaran S A, Gopalakrishnan S 2020 Phys. Rev. Lett. 125 237601
Google Scholar
[17] Phuc N T, Trung P Q 2021 Phys. Rev. B 104 115105
Google Scholar
[18] Li Z L, Oshikawa M, Wan Y 2021 Phys. Rev. X 11 031035
Google Scholar
[19] Hamm P, Meuwl M, Johnson S L, Beaud P 2017 Struc. Dyn. 4 061601
Google Scholar
[20] Hahn E L 1950 Phys. Rev. 80 580
Google Scholar
[21] Carr H Y, Purcell E M 1954 Phys. Rev. 94 630
Google Scholar
[22] Abella I D, Kurnit N A, Hartmann S R 1966 Phys. Rev. 141 391
Google Scholar
[23] Pfeuty P 1970 Ann. Phys. 57 79
Google Scholar
[24] Anderson P W 1984 Basic Notations of Condensed Matter Physics (Benjamin Cummings) pp92–104
[25] Zvezdin A, Kotov V 1997 Modern Magnetooptics and Magnetooptical Materials (New York: Taylor & Francis)
[26] Lieb E, Schultz T, Mattis D 1961 Ann. Phys. 16 407
Google Scholar
[27] Affleck I 1988 Les Houches Summer School in Theoretical Physics: Fields, Strings, Critical Phenomena pp563–640
[28] Giamarchi T 2003 Quantum Physics in One Dimension (Oxford: Oxford University Press)
[29] von Delft J, Schoeller H 1998 Ann. Phys. 7 225
Google Scholar
[30] Shankar R 1993 Acta Phys. Polonica B 26 12
Google Scholar
[31] Pham K V, Gabay M, Lederer P 2000 Phys. Rev. B 61 16397
Google Scholar
[32] Kamenev A 2011 Field Theory of Non-Equilibrium Systems (Cambridge: Cambridge University Press)
[33] Sachdev S, Young A P 1997 Phys. Rev. Lett. 78 2220
Google Scholar
[34] Damle K, Sachdev S 1998 Phys. Rev. B 57 8307
Google Scholar
[35] Damle K, Sachdev S 1998 Phys. Rev. Lett. 95 187201
Google Scholar
下载:
计量
- 文章访问数: 5592
- PDF下载量: 247
- 被引次数: 0
