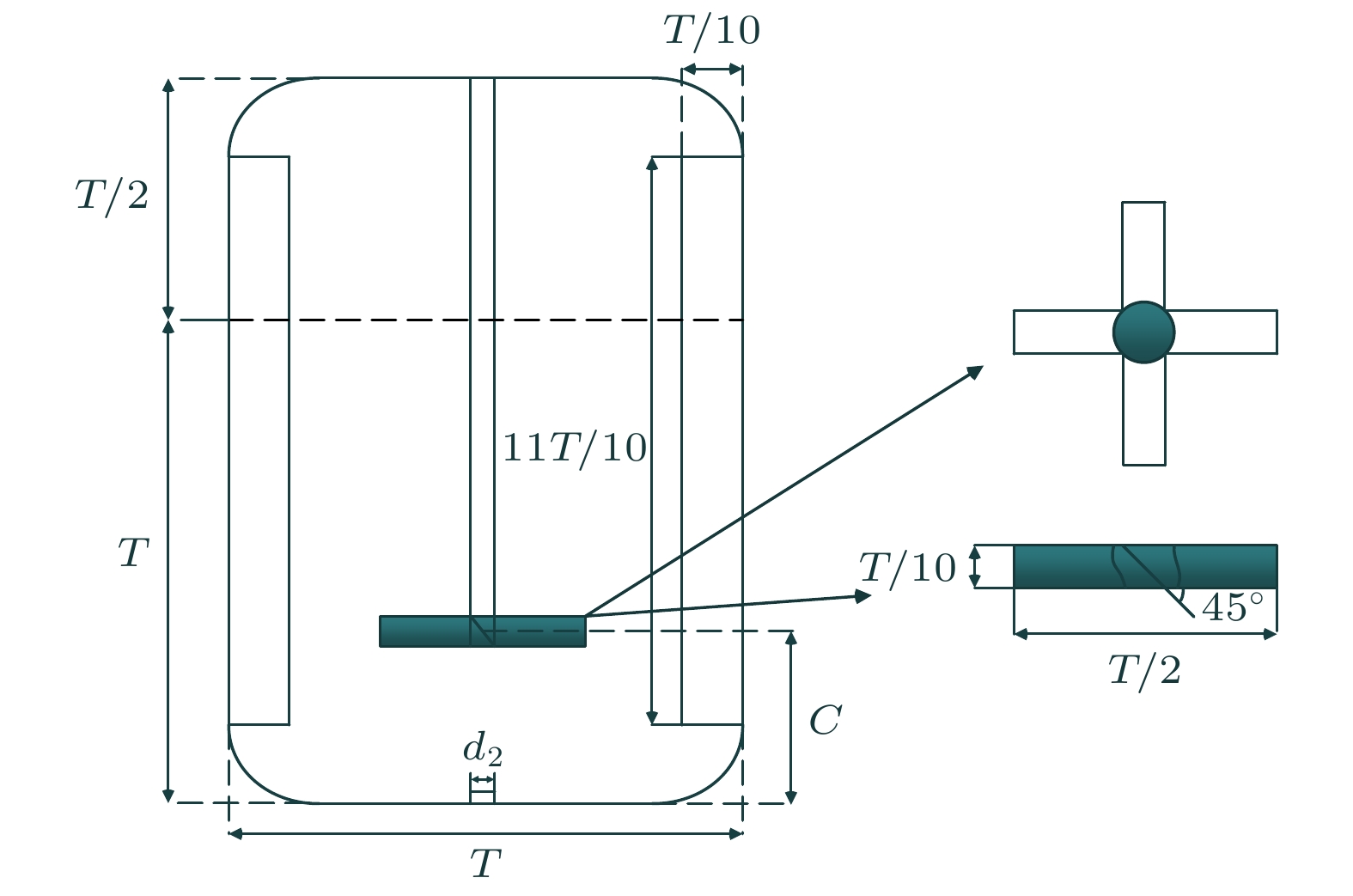
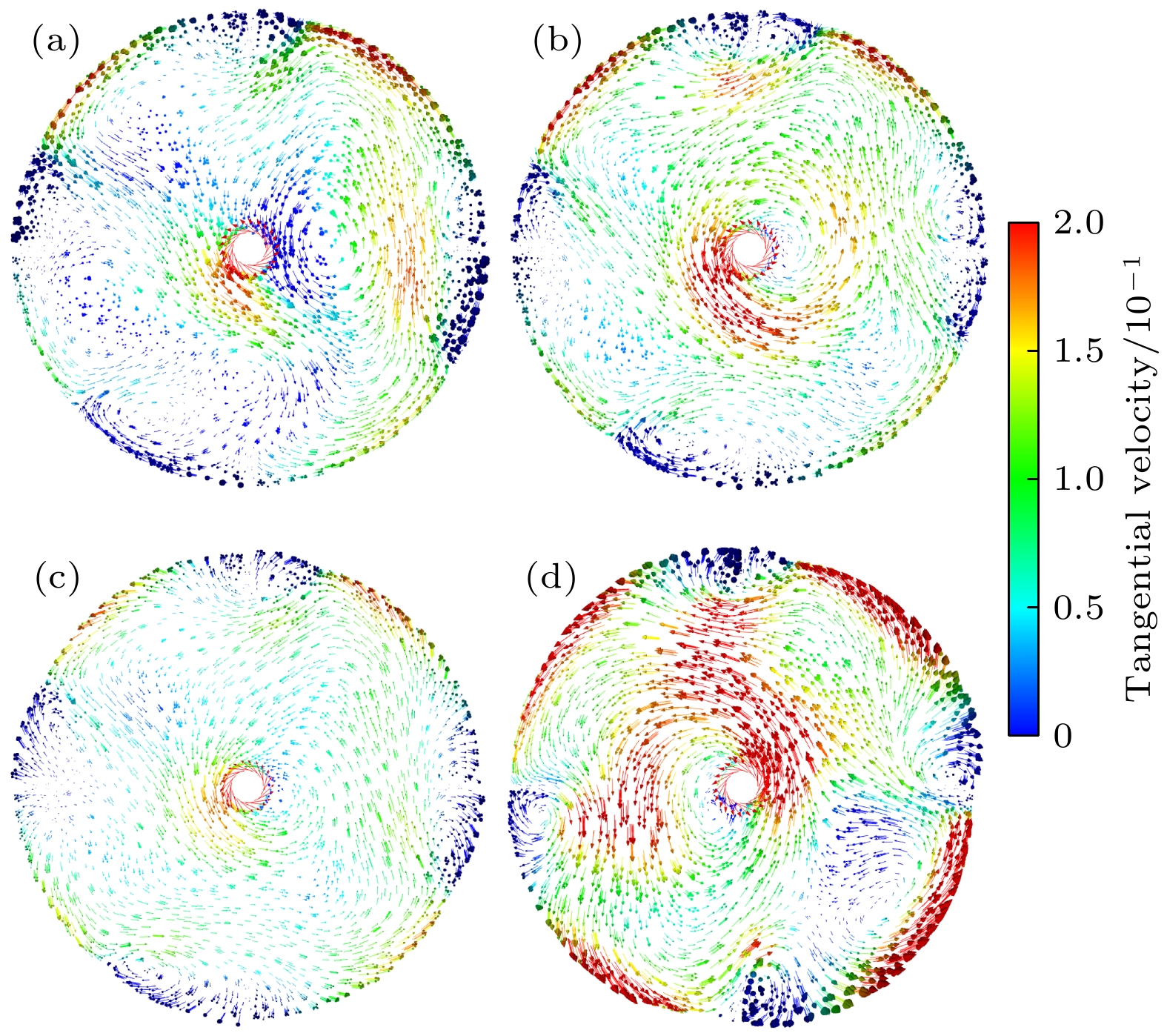
-
气-液-固三相流混合过程是一个复杂的多重流固耦合动力学问题, 颗粒参数与流道物理空间尺度之间的关系直接影响计算收敛性, 强剪切区域的流固双向耦合作用数值建模与网格处理具有较高难度. 针对上述问题, 提出了一种气-液-固三相流混合的建模与求解方法. 基于流体体积-离散单元耦合模型, 建立考虑颗粒运动的三相动力学模型, 通过求解动量方程, 实现两相流体与颗粒的双向耦合. 自主开发用户自定义函数(UDF)通信接口, 得到流体与颗粒间的相互作用力, 提出了一种多孔相间耦合解法来描述颗粒运动轨迹. 以带强剪切的三相流混合过程为例, 使用该方法研究了不同充气条件对流道物理空间内自由表面、速度分布和颗粒悬浮特性的影响规律. 结果表明, 强剪切和壁面作用可以将流体的切向速度转化为轴向和径向的速度; 选择合适的充气速度可以消除自由液面的不稳定性; 增加流体的流动速度, 对于部分区域颗粒的悬浮提升作用有限. 研究结果可为复杂多相流相间作用机理研究提供有益借鉴, 也可为气-液-固三相颗粒混合生产调控提供技术支持.
-
关键词:
- 三相流 /
- 固体颗粒 /
- 流体体积-离散单元耦合 /
- 强剪切
The mixing process of gas-liquid-solid three-phase flow is a complex multi-fluid-structure coupling dynamic problem. The relationship between the particle parameters and the physical spatial scale of the flow channel directly affects the calculation convergence. Numerical modeling and mesh processing of fluid-structure bidirectional interaction in the strong shear zone are difficult. Aiming at the above problems, a method of modeling and solving the gas-liquid-solid three-phase flow mixing is proposed. Based on the volume-of-fluid coupled with discrete-element-method model, a three-phase dynamic model considering particle motion is established. By solving the momentum equation, the bidirectional coupling of two-phase fluid and particle is realized. The user-defined function communication interface is developed independently to obtain the interaction force between fluid and particles, and a porous-interphase coupling solution is proposed to describe the trajectory of particles. Taking the mixing process of three-phase flow with strong shear for example, this method is used to study the influence of different aeration conditions on the free surface, velocity distribution and particle suspension characteristics in the physical space of the flow channel. The results show that strong shear and wall action can convert the tangential velocity of the fluid into axial and radial velocity; choosing an appropriate inflation velocity can eliminate the instability of the free surface and increasing the flow velocity of the fluid has a limited effect on the suspension of particles in some areas. The research results can provide a useful reference for studying the interaction mechanism of complex multiphase flow, and also provide technical support for the mixing production control of gas-liquid-solid three-phase particles.-
Keywords:
- three-phase flow /
- solid particles /
- volume of fluid-discrete element method coupling /
- strong shear
[1] Delvigne F, Destain J, Thonart P 2005 Chem. Eng. J. 113 1
Google Scholar
[2] Ge M, Ji S M, Tan D P, Cao H Q 2021 Int. J. Adv. Manuf. Tech. 114 3419
Google Scholar
[3] Hosseini S, Patel D, Ein-Mozaffari F, Mehrvar M 2010 Ind. Eng. Chem. Res. 49 4426
Google Scholar
[4] Wang S Y, Jiang X X, Wang R C, Wang X, Yang S W, Zhao J, Liu Y 2017 Adv. Powder Technol. 28 1611
Google Scholar
[5] Panneerselvam R, Savithri S, Surender G D 2009 Ind. Eng. Chem. Res. 48 1611
Google Scholar
[6] Li L, Tan D P, Yin Z C, Wang T, Fan X H, Wang R H 2021 Renew. Energy 175 887
Google Scholar
[7] Li L, Tan D P, Wang T, Yin Z C, Fan X H, Wang R H 2021 Energy 216 119136
Google Scholar
[8] Kasat G R, Khopkar A R, Ranade V V, Pandit A B 2008 Chem. Eng. Sci. 63 3877
Google Scholar
[9] Qi H, Qin S K, Cheng Z C, Teng Q, Hong T, Xie Y 2021 J. Manuf. Processes 64 585
Google Scholar
[10] Tan D P, Li L, Yin Z C, Li D F, Zhu Y L, Zheng S 2020 Int. J. Heat Mass Transfer 150 119250
Google Scholar
[11] Alexander S, Alexander D, Markku N, Jan M, Falah A, Maximilian V B, Jochen S, Bernd E 2019 Chem. Eng. Sci. 196 37
Google Scholar
[12] Musango L, John S, Lloyd M 2021 Powder Technol. 378 85
Google Scholar
[13] Li L, Lu J F, Fang H, Yin Z C, Wang T, Wang R H, Fan X H, Zhao L J, Tan D P, Wan Y H 2020 IEEE Access 8 27649
Google Scholar
[14] Han Y, Cundall P A 2013 Int. J. Numer. Anal. Methods Geomech. 37 10
Google Scholar
[15] Bastien D, Juliane R, Louis F, Francois B, Bruno B 2021 Chem. Eng. Sci. 230 116137
Google Scholar
[16] 邵婷, 胡银玉, 王文坦, 金涌, 程易 2013 中国化学工程学报 21 1069
Google Scholar
Shao T, Hu Y Y, Wang W T, Jin Y, Cheng Y 2013 Chin. J. Chem. Eng. 21 1069
Google Scholar
[17] Blais B, Lassaigne M, Goniva C, Fradette L 2016 J. Comput. Phys. 318 201
Google Scholar
[18] Blais B, Bertrand F 2017 Chem. Eng. Res. Des. 118 270
Google Scholar
[19] Blais B, Bertrand O, Fradette L, Bertrand F 2017 Chem. Eng. Res. Des. 123 388
Google Scholar
[20] Xu W T, Tan Y B, Li M, Sun J L, Xie D, Liu Z 2020 Particuology 49 159
Google Scholar
[21] Sun X, Sakai M 2015 Chem. Eng. Sci. 134 531
Google Scholar
[22] Wu L, Gong M, Wang J T 2018 Ind. Eng. Chem. Res. 57 1714
Google Scholar
[23] Kang Q Q, He D P, Zhao N, Feng X, Wang J T 2019 Chem. Eng. J. 386 122846
Google Scholar
[24] 谭大鹏, 杨涛, 赵军, 计时鸣 2016 65 054701
Google Scholar
Tan D P, Yang T, Zhao J, Ji S M 2016 Acta Phys. Sin. 65 054701
Google Scholar
[25] 刘扬, 韩燕龙, 贾富国, 姚丽娜, 王会, 史宇菲 2015 64 114501
Google Scholar
Liu Y, Han Y L, Jia F G, Yao L N, Wang H, Shi Y F 2015 Acta Phys. Sin. 64 114501
Google Scholar
[26] Ergun S 1952 Chem. Eng. Prog. 48 89
Google Scholar
[27] Ji S M, Xiao F Q, Tan D P 2010 Sci. China Technol. Sc. 53 100
Google Scholar
[28] Saffman P G 1965 J. Fluid Mech. 22 385
Google Scholar
[29] Tan D P, Ji S M, Fu Y Z 2016 Int. J. Adv. Manuf. Technol. 85 1261
Google Scholar
[30] Li L, Qi H, Yin Z C, Li D F, Zhu Z L, Tangwarodomnukun V, Tan D P 2019 Powder Technol. 360 462
Google Scholar
[31] Lu J F, Wang T, Li L, Yin Z C, Wang R H, Fan X H, Tan D P 2020 Processes 8 760
Google Scholar
[32] Tamburini A, Cipollina A, Micale G, Brucato A, Ciofalo M 2012 Chem. Eng. J. 193 234
Google Scholar
[33] Jahoda M, Tomaskova L, Mostek M 2009 Chem. Eng. Res. Des. 87 460
Google Scholar
[34] Wang J J, Han Y, Gu X P, Feng L F, Hu G H 2013 AIChE J. 59 1066
Google Scholar
[35] Xie L, Luo Z H 2017 Chem. Eng. Sci. 176 439
Google Scholar
[36] Jovanovic A, Pezo M, Pezo L, Levic L 2014 Powder Technol. 266 240
Google Scholar
-
图 3 网格划分 (a) 静子区域网格; (b) 转子区域网格; (c) 叶轮网格
Fig. 3. Grids division: (a) Grids of stator region; (b) grids of rotor region; (c) grids of impeller.
图 4 四种网格尺寸在t = 5 s时的轴向速度分布
Fig. 4. Axial velocity distribution of four grid sizes at t = 5 s
图 5 t = 5 s时四种充气条件下混合空间内的自由液面 (a) v = 0 m/s; (b) v = 0.01 m/s; (c) v = 0.05 m/s; (d) v = 1 m/s
Fig. 5. Free surface under four aeration conditions at t = 5 s: (a) v = 0 m/s; (b) v = 0.01 m/s; (c) v = 0.05 m/s; (d) v = 1 m/s.
图 6 t = 5 s时, 四种充气条件下混合空间内z = 0.15 m高度截面的切向速度矢量图 (a) v = 0 m/s; (b) v = 0.01 m/s; (c) v = 0.05 m/s; (d) v = 1 m/s
Fig. 6. Tangential velocity vector in height z = 0.15 m under four aeration conditions at t = 5 s: (a) v = 0 m/s; (b) v = 0.01 m/s; (c) v = 0.05 m/s; (d) v = 1 m/s.
图 7 径向位置r = 60 mm处的轴向高度速度分布 (a) 总速度; (b) 轴向速度; (c) 径向速度; (d) 切向速度
Fig. 7. Axial velocity distribution at radial position r = 60 mm: (a) Total velocity; (b) axial velocity; (c) radial velocity; (d) tangential velocity.
图 8 v = 0 m/s时不同时刻的三相混合系统模拟结果
Fig. 8. Simulation results of three-phase stirred system at different time when v = 0 m/s.
图 9 v = 0.01 m/s时不同时刻的三相混合系统模拟结果
Fig. 9. Simulation results of three-phase stirred system at different time when v = 0.01 m/s.
图 10 v = 0.05 m/s时不同时刻的三相混合系统模拟结果
Fig. 10. Simulation results of three-phase stirred system at different time when v = 0.05 m/s.
图 11 v = 1 m/s时不同时刻的三相混合系统模拟结果
Fig. 11. Simulation results of three-phase stirred system at different time when v = 1 m/s.
图 12 不同充气工作条件下的RSD随时间的变化
Fig. 12. RSD changes with time under different aeration conditions.
表 1 流体和颗粒特性设置
Table 1. Characteristics settings of fluid and particle.
参数 值 空气密度 ${\rho _{\rm{a}}}$/(${\rm{kg}} \cdot {{\rm{m}}^{{ - 3}}}$) 1 空气黏度 ${\mu _{\rm{a}}}$/(${\rm{Pa}} \cdot {\rm{s}}$) 1 × 10–5 水密度 ${\rho _{\rm{w}}}$/(${\rm{kg}} \cdot {{\rm{m}}^{{ - 3}}}$) 1000 水黏度 ${\mu _{\rm{w}}}$/(${\rm{Pa}} \cdot {\rm{s}}$) 0.001 颗粒密度 ${\rho _{\rm{p}}}$/(${\rm{kg}} \cdot {{\rm{m}}^{{ - 3}}}$) 1100 颗粒直径 ${d_{\rm{p}}}$/mm 1 颗粒数目 ${n_{\rm{p}}}$ 10000 颗粒杨氏模量 ${Y_{\rm{P}}}$/${\rm{MPa}}$ 1 颗粒泊松比 ${\nu _{\rm{P}}}$ 0.25 壁面杨氏模量 ${Y_{\rm{w}}}$/${\rm{MPa}}$ 70000 壁面泊松比 ${\nu _{\rm{w}}}$ 0.3 滚动摩擦系数 ${\mu _{\rm{r}}}$ 0.01 静摩擦系数 ${\mu _{\rm{s}}}$ 0.5 恢复系数 ${e_{\rm{r}}}$ 0.5 搅拌桨速度 $\omega $/(${\rm r} \cdot {\rm min }^{ - 1 }$) 400 CFD时间步 $\Delta {t_{\rm{CFD}}}$/${\rm{s}}$ $2 \times {10^{ - 4}}$ DEM时间步 $\Delta {t_{\rm{DEM}}}$/${\rm{s}}$ $2 \times {10^{ - 5}}$ 耦合时间步 $\Delta {t_{{\rm{coupling}}}}$/${\rm{s}}$ $2 \times {10^{ - 4}}$ 
下载: 导出CSV
-
[1] Delvigne F, Destain J, Thonart P 2005 Chem. Eng. J. 113 1
Google Scholar
[2] Ge M, Ji S M, Tan D P, Cao H Q 2021 Int. J. Adv. Manuf. Tech. 114 3419
Google Scholar
[3] Hosseini S, Patel D, Ein-Mozaffari F, Mehrvar M 2010 Ind. Eng. Chem. Res. 49 4426
Google Scholar
[4] Wang S Y, Jiang X X, Wang R C, Wang X, Yang S W, Zhao J, Liu Y 2017 Adv. Powder Technol. 28 1611
Google Scholar
[5] Panneerselvam R, Savithri S, Surender G D 2009 Ind. Eng. Chem. Res. 48 1611
Google Scholar
[6] Li L, Tan D P, Yin Z C, Wang T, Fan X H, Wang R H 2021 Renew. Energy 175 887
Google Scholar
[7] Li L, Tan D P, Wang T, Yin Z C, Fan X H, Wang R H 2021 Energy 216 119136
Google Scholar
[8] Kasat G R, Khopkar A R, Ranade V V, Pandit A B 2008 Chem. Eng. Sci. 63 3877
Google Scholar
[9] Qi H, Qin S K, Cheng Z C, Teng Q, Hong T, Xie Y 2021 J. Manuf. Processes 64 585
Google Scholar
[10] Tan D P, Li L, Yin Z C, Li D F, Zhu Y L, Zheng S 2020 Int. J. Heat Mass Transfer 150 119250
Google Scholar
[11] Alexander S, Alexander D, Markku N, Jan M, Falah A, Maximilian V B, Jochen S, Bernd E 2019 Chem. Eng. Sci. 196 37
Google Scholar
[12] Musango L, John S, Lloyd M 2021 Powder Technol. 378 85
Google Scholar
[13] Li L, Lu J F, Fang H, Yin Z C, Wang T, Wang R H, Fan X H, Zhao L J, Tan D P, Wan Y H 2020 IEEE Access 8 27649
Google Scholar
[14] Han Y, Cundall P A 2013 Int. J. Numer. Anal. Methods Geomech. 37 10
Google Scholar
[15] Bastien D, Juliane R, Louis F, Francois B, Bruno B 2021 Chem. Eng. Sci. 230 116137
Google Scholar
[16] 邵婷, 胡银玉, 王文坦, 金涌, 程易 2013 中国化学工程学报 21 1069
Google Scholar
Shao T, Hu Y Y, Wang W T, Jin Y, Cheng Y 2013 Chin. J. Chem. Eng. 21 1069
Google Scholar
[17] Blais B, Lassaigne M, Goniva C, Fradette L 2016 J. Comput. Phys. 318 201
Google Scholar
[18] Blais B, Bertrand F 2017 Chem. Eng. Res. Des. 118 270
Google Scholar
[19] Blais B, Bertrand O, Fradette L, Bertrand F 2017 Chem. Eng. Res. Des. 123 388
Google Scholar
[20] Xu W T, Tan Y B, Li M, Sun J L, Xie D, Liu Z 2020 Particuology 49 159
Google Scholar
[21] Sun X, Sakai M 2015 Chem. Eng. Sci. 134 531
Google Scholar
[22] Wu L, Gong M, Wang J T 2018 Ind. Eng. Chem. Res. 57 1714
Google Scholar
[23] Kang Q Q, He D P, Zhao N, Feng X, Wang J T 2019 Chem. Eng. J. 386 122846
Google Scholar
[24] 谭大鹏, 杨涛, 赵军, 计时鸣 2016 65 054701
Google Scholar
Tan D P, Yang T, Zhao J, Ji S M 2016 Acta Phys. Sin. 65 054701
Google Scholar
[25] 刘扬, 韩燕龙, 贾富国, 姚丽娜, 王会, 史宇菲 2015 64 114501
Google Scholar
Liu Y, Han Y L, Jia F G, Yao L N, Wang H, Shi Y F 2015 Acta Phys. Sin. 64 114501
Google Scholar
[26] Ergun S 1952 Chem. Eng. Prog. 48 89
Google Scholar
[27] Ji S M, Xiao F Q, Tan D P 2010 Sci. China Technol. Sc. 53 100
Google Scholar
[28] Saffman P G 1965 J. Fluid Mech. 22 385
Google Scholar
[29] Tan D P, Ji S M, Fu Y Z 2016 Int. J. Adv. Manuf. Technol. 85 1261
Google Scholar
[30] Li L, Qi H, Yin Z C, Li D F, Zhu Z L, Tangwarodomnukun V, Tan D P 2019 Powder Technol. 360 462
Google Scholar
[31] Lu J F, Wang T, Li L, Yin Z C, Wang R H, Fan X H, Tan D P 2020 Processes 8 760
Google Scholar
[32] Tamburini A, Cipollina A, Micale G, Brucato A, Ciofalo M 2012 Chem. Eng. J. 193 234
Google Scholar
[33] Jahoda M, Tomaskova L, Mostek M 2009 Chem. Eng. Res. Des. 87 460
Google Scholar
[34] Wang J J, Han Y, Gu X P, Feng L F, Hu G H 2013 AIChE J. 59 1066
Google Scholar
[35] Xie L, Luo Z H 2017 Chem. Eng. Sci. 176 439
Google Scholar
[36] Jovanovic A, Pezo M, Pezo L, Levic L 2014 Powder Technol. 266 240
Google Scholar
下载:
计量
- 文章访问数: 10176
- PDF下载量: 378
- 被引次数: 0
